Quantum Monte Carlo approaches for strongly correlated systems

The quantum many-body problem and Monte Carlo

|\psi\rangle=

Quantum Monte Carlo: sample properties without storing full wave functions

Exact simulations \(\rightarrow\) fermion sign problem (exponential decay of signal to noise ratio)

\hat{H}|\psi\rangle = E|\psi\rangle
i\frac{d |\psi\rangle}{d t} = \hat{H}|\psi\rangle
\langle \hat{O}\rangle = \frac{\langle\psi|\hat{O}|\psi\rangle}{\langle\psi|\psi\rangle}

Lattice models:  

\hat{H}_{\text{Hubbard}} = -t\sum_{\langle ij\rangle,\sigma}(\hat{a}_{i\sigma}^{\dagger}\hat{a}_{j\sigma}+\hat{a}_{j\sigma}^{\dagger}\hat{a}_{i\sigma}) + U\sum_i\hat{n}_{i\uparrow}\hat{n}_{i\downarrow}

Ab initio models: 

\hat{H}_{\textit{Ab initio}} = \sum t_{pr} \hat{a}_{p}^{\dagger}\hat{a}_r + \sum v_{prqs}\hat{a}_{p}^{\dagger}\hat{a}_r\hat{a}_{q}^{\dagger}\hat{a}_{s}

Variational Monte Carlo

Stochastic multireference perturbation theory

Auxiliary field QMC

Outline

  • Sampling and the sign problem in AFQMC 
  • Reducing noise using selected CI wave functions 
  • Benchmark results
  • Jastrow symmetry projected states in VMC
  • Auxiliary field QMC
  • Variational MC
  • Use in projection QMC

Projection QMC

e^{-\tau (\hat{H}-E_0)}|\psi_r\rangle = c_0|\Psi_0\rangle + c_1 e^{-\Delta E_1\tau}|\Psi_1\rangle+\dots
|\psi_r\rangle = c_0|\Psi_0\rangle + c_1|\Psi_1\rangle +\dots
  • Free projection: try to manage sign problem, numerically exact and exponentially scaling

Noise in QMC sampling worsens exponentially with \(\tau\) and system size

Imaginary time propagation:

Two flavors:

  • Constrained: use trial state to constrain projection, trial dependent bias but polynomially scaling
E(\tau)=\dfrac{\langle\psi_l|\hat{H}e^{-\tau \hat{H}}|\psi_r\rangle}{\langle\psi_l|e^{-\tau \hat{H}}|\psi_r\rangle}

Sampling in Auxiliary Field QMC

\hat{H} = \hat{K} + \hat{V} = t_{pr} \hat{a}_{p}^{\dagger}\hat{a}_r + \frac{1}{2}\sum_{\gamma} \left(L^{\gamma}_{pr}\hat{a}_p^{\dagger}\hat{a}_r\right)^2
  • Exponentiating \(\hat{K}\):  
e^{t_{pr}\hat{a}_p^{\dagger}\hat{a}_r}|\phi\rangle=|\phi'\rangle
  • Exponentiating  \(\hat{V}\): coupling to a scalar field
e^{-\frac{\hat{L}_{\gamma}^2}{2}} = \int \frac{dx_{\gamma}}{\sqrt{2\pi}}\ e^{\frac{-x_{\gamma}^2}{2}}e^{ix_{\gamma}\hat{L}_{\gamma}}

\(x_{\gamma}\): auxiliary field

(Thouless, 1960)

(Stratonovich, 1957)

E(\tau) = \dfrac{\langle\psi_l|\hat{H}e^{-\tau \hat{H}}|\psi_r\rangle}{\langle\psi_l|e^{-\tau \hat{H}}|\psi_r\rangle} \approx \dfrac{\int\ dX p(X)\langle\psi_l|\hat{H}\hat{\mathcal{B}}(X)|\psi_r\rangle}{\int\ dX p(X)\langle\psi_l|\hat{\mathcal{B}}(X)|\psi_r\rangle}

Free projection:

Zhang, Krakauer, Reichman, Freisner, Rubenstein, ...

Phaseless:

w_{\phi(\mathbf{x})} = \Bigg\vert\dfrac{\langle\psi_T|\phi(\mathbf{x})\rangle}{\langle\psi_T|\phi\rangle}e^{\mathbf{x}.\bar{\mathbf{x}}-\bar{\mathbf{x}}^2/2}\Bigg\vert\max(0, \cos(\Delta\theta))

CCSD as \(|\psi_r\rangle\) in free projection: 

|\psi_r\rangle = \exp\left(t_{ikjl}\hat{a}_i^{\dagger}\hat{a}_k\hat{a}_j^{\dagger}\hat{a}_l\right)\exp\left(t_{ik}\hat{a}_i^{\dagger}\hat{a}_k\right)|\phi_0\rangle

Benzene (30e, 102o)

E(\tau)=\dfrac{\langle\psi_l|\hat{H}e^{-\tau \hat{H}}|\psi_r\rangle}{\langle\psi_l|e^{-\tau \hat{H}}|\psi_r\rangle}

The sign problem in free projection

\text{Var}\left(\dfrac{\overline{N}}{\overline{D}}\right) \approx \dfrac{\text{Var}(\overline{N})}{\overline{D}^2} + \dfrac{\overline{N}^2\text{Var}(\overline{D})}{\overline{D}^4} - 2\dfrac{\overline{N}\text{Cov}(\overline{N},\overline{D})}{\overline{D}^3}
E(\tau)\approx\dfrac{\sum_i\langle\psi_l|\hat{H}|\phi_i\rangle}{\sum_i\langle\psi_l|\phi_i\rangle} = \dfrac{\overline{N}}{\overline{D}}

Contour shift:

e^{-\frac{y^2}{2}} = \int \frac{dx}{\sqrt{2\pi}}\ e^{\frac{-x^2}{2}+ixy}
x\rightarrow x+iy
x_{\gamma} \rightarrow x_{\gamma} + i \sqrt{\tau}\langle\hat{L}_{\gamma}\rangle

In AFQMC:

Baer, Head-Gordon, Neuhauser (1998)

Zero variance principle

If \(|\psi_l\rangle\) is the exact ground state, then \(N\) and \(D\) are perfectly correlated, \(\langle\psi_0|\hat{H}|\phi_i\rangle = E_0 \langle\psi_0|\phi_i\rangle\), and the energy estimator has zero variance. More accurate \(|\psi_l\rangle\ \rightarrow\ \) higher \(\text{Cov}(N, D)\).

E(\tau)\approx\dfrac{\sum_i\langle\psi_l|\hat{H}|\phi_i\rangle}{\sum_i\langle\psi_l|\phi_i\rangle} = \dfrac{\overline{N}}{\overline{D}}
\text{Var}\left(\dfrac{\overline{N}}{\overline{D}}\right) \approx \dfrac{\text{Var}(\overline{N})}{\overline{D}^2} + \dfrac{\overline{N}^2\text{Var}(\overline{D})}{\overline{D}^4} - 2\dfrac{\overline{N}\text{Cov}(\overline{N},\overline{D})}{\overline{D}^3}

Selected configuration interaction: put the most important configurations in the state using particle-hole excitations and diagonalize

|\psi_l\rangle = \sum_i^{N_c} c_i |\psi_i\rangle

Benzene (30e, 102o)

E(\tau)=\dfrac{\langle\psi_l|\hat{H}e^{-\tau \hat{H}}|\psi_r\rangle}{\langle\psi_l|e^{-\tau \hat{H}}|\psi_r\rangle}

Selected CI local energy algorithm

E_L[\phi]=\dfrac{\langle\psi_l|\hat{H}|\phi\rangle}{\langle\psi_l|\phi\rangle},\ \text{two-body part: }\ L^{\gamma}_{pr}L^{\gamma}_{qs}\dfrac{\langle\psi_l|\hat{a}_p^{\dagger}\hat{a}_q^{\dagger}\hat{a}_s\hat{a}_r|\phi\rangle}{\langle\psi_l|\phi\rangle}

Generalized Wick's theorem:  consider \(|\psi_l\rangle = c_{ptqu}\hat{a}_t^{\dagger}\hat{a}_p\hat{a}_u^{\dagger}\hat{a}_q|\psi_0\rangle\)

Benzene (30e, 102o)

\([\text{Cu}_2\text{O}_2]^{2+}\) isomerization

\(\Delta E = E(\text{bis}) - E(\text{peroxo})\)

Method
DFT (UBLYP) 36.0
DFT (UB3LYP) 52.9
DFT (UMPW1K) 74.0
CCSD(T) 30.6
CR-CCSD(TQ) 33.8
DMRG-CT 27.1
ph-AFQMC (NOCI) 32.1
fp-AFQMC 24.1(6)

kcal/mol

(32e, 108o)

\(\text{H}_{50}\) (50e, 50o)

Systematically improving phaseless AFQMC

Ni

Excited states in phaseless AFQMC

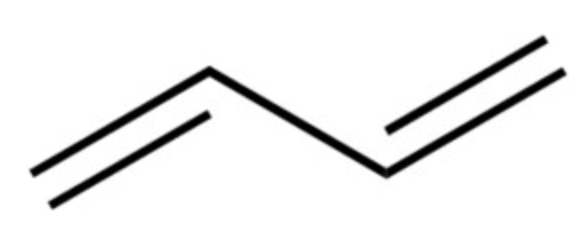
Butadiene: (22e, 142o)

Nickel porphyrin:  (122e, 406o)

Method
SC-NEVPT2  6.72 6.74
CCSD 6.31 7.08
AFQMC  6.46(5) 6.67(5)
TBE 6.2 6.5

eV

Method
CASSCF (4e, 4o) 3.8
CCSD 2.55
AFQMC / sCI (50k) 3.0(1)
AFQMC / sCI (100k) 2.8(1)
Experiment 2.3-2.4

eV

1\ ^1B_{2u}
1\ ^1B_{u}
2\ ^1A_{g}

(4e, 8o) active space

Other properties

Species Exact  CCSD ph-AFQMC
0.986 0.991 0.985(2)
0.990 0.992 0.986(2)
CO 0.090 0.099 0.086(3) 
  • Can be evaluated as derivatives of energy
  • Derivatives can be calculated just as efficiently as energy (JAX implementation)

a.u.

Small molecule dipole moment calculations (6-31g basis):

NH\(_3\)

H\(_2\)O

Outline

  • Sampling and the sign problem in AFQMC 
  • Reducing noise using selected CI wave functions 
  • Benchmark results
  • Jastrow symmetry projected states in VMC
  • Auxiliary field QMC
  • Variational MC
  • Use in projection QMC

Variational Monte Carlo (VMC)

E = \dfrac{\langle \psi|H|\psi\rangle }{\langle \psi|\psi\rangle}

Strategy:

  • Parametrize the wave function: \(|\psi(\mathbf{p})\rangle\), choose initial \(\mathbf{p}\)
  • Calculate energy and gradient: Markov chain Monte Carlo
\ \dfrac{\langle \psi(\mathbf{p})|H|\psi(\mathbf{p})\rangle }{\langle \psi(\mathbf{p})|\psi(\mathbf{p})\rangle} = \sum_{\mathbf{n}} \rho_{\mathbf{n}} E_L[\mathbf{n}]
  • Optimize: smart gradient descent to change parameters
\propto|\langle \mathbf{n}|\psi(\mathbf{p})\rangle|^2

Ground state minimizes

\text{walker}: |\mathbf{n}\rangle

McMillan (1965)

Symmetry projection in VMC

Symmetry breaking \(\rightarrow\) more variational freedom

Break the symmetry under a projector, to retain good quantum numbers

|\psi\rangle=\hat{P}|\phi\rangle

Projection in VMC by restricting random walk to the symmetry sector

Symmetries: spin, number, complex conjugation, ...

Example: complex conjugation in \(\text{H}_2\) near dissociation

|\text{RHF}\rangle = (a_{1\uparrow}^{\dagger}+a_{2\uparrow}^{\dagger})(a_{1\downarrow}^{\dagger}+a_{2\downarrow}^{\dagger})|0\rangle = (a_{1\uparrow}^{\dagger}a_{1\downarrow}^{\dagger}+a_{1\uparrow}^{\dagger}a_{2\downarrow}^{\dagger}+a_{2\uparrow}^{\dagger}a_{1\downarrow}^{\dagger}+a_{2\uparrow}^{\dagger}a_{2\downarrow}^{\dagger})|0\rangle
|\text{cRHF}\rangle = (e^{i\pi/4}a_{1\uparrow}^{\dagger}+e^{-i\pi/4}a_{2\uparrow}^{\dagger})(e^{i\pi/4}a_{1\downarrow}^{\dagger}+e^{-i\pi/4}a_{2\downarrow}^{\dagger})|0\rangle\\ \quad = (ia_{1\uparrow}^{\dagger}a_{1\downarrow}^{\dagger}+a_{1\uparrow}^{\dagger}a_{2\downarrow}^{\dagger}+a_{2\uparrow}^{\dagger}a_{1\downarrow}^{\dagger}-ia_{2\uparrow}^{\dagger}a_{2\downarrow}^{\dagger})|0\rangle
\hat{K}|\text{cRHF}\rangle = (a_{1\uparrow}^{\dagger}a_{2\downarrow}^{\dagger}+a_{2\uparrow}^{\dagger}a_{1\downarrow}^{\dagger})|0\rangle

Imada, Sorella, Neuscamman, ...

Jastrow factor

\hat{\mathcal{J}}|\psi\rangle = \exp\left(\sum_{p\sigma,q\gamma} v_{p\sigma,q\gamma}\hat{n}_{p\sigma}\hat{n}_{q\gamma}\right)|\psi\rangle
d (Bohr) Exact (DMRG) Jastrow-KSzPfaffian Green's function MC
1.6 -0.5344 -0.5337 -0.5342
1.8 -0.5408 -0.5400 -0.5406
2.5 -0.5187 -0.5180 -0.5185

H\( _{50} \) linear chain (50e, 50o)

Hartree/particle

Correlates doublons and holons, can describe Mott insulating behavior

U Benchmark energy Jastrow-
KSzGHF		 Green's function MC
2 -1.1962 -1.1920 -1.1939
4 -0.8620 -0.8566 -0.8598
8 -0.5237 -0.5183 -0.5221

2D Hubbard: 98 sites (half filling)

Density-density correlation function: 18 site 2D Hubbard model (\(U/t=4\))

Thank you!