DESI 2024: Survey overview and cosmological constraints from DR1 BAO and Full Shape measurements
Arnaud de Mattia
CEA Saclay

CPPM
November 2024


Thanks to our sponsors and
72 Participating Institutions!


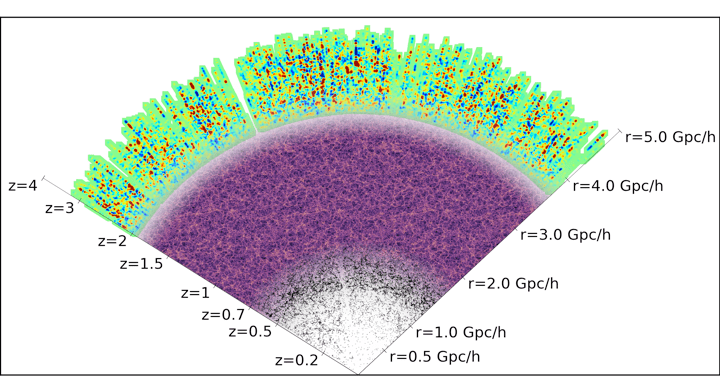
DESI 3D Map

Physics program
- Galaxy and quasar clustering
- Lyman-alpha forest
- Clusters and cross-correlations
- Galaxy and quasar physics
- Milky Way Survey
- Transients and low-z

DESI 3D Map

Physics program
- Galaxy and quasar clustering
- Lyman-alpha forest
- Clusters and cross-correlations
- Galaxy and quasar physics
- Milky Way Survey
- Transients and low-z

DESI: a stage IV survey
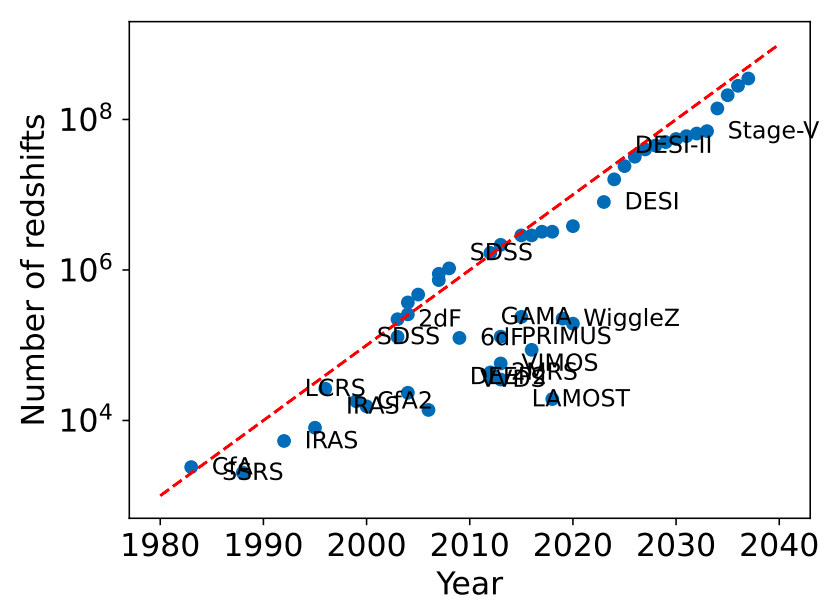
10 years = \(10 \times \)

DESI Y5 galaxy samples
Bright Galaxies: 14M (SDSS: 600k)
0 < z < 0.4
LRG: 8M (SDSS: 1M)
0.4 < z < 0.8
ELG: 16M (SDSS: 200k)
0.6 < z < 1.6
QSO: 3M (SDSS: 500k)
Lya \(1.8 < z\)
Tracers \(0.8 < z < 2.1\)
Y5 \(\sim 40\)M galaxy redshifts!
\(z = 0.4\)
\(z = 0.8\)
\(z = 0\)
\(z = 1.6\)
\(z = 2.0\)
\(z = 3.0\)

From images to redshifts





imaging surveys (2014 - 2019) + WISE (IR)
target selection
spectroscopic observations
spectra and redshift measurements

Mayall Telescope
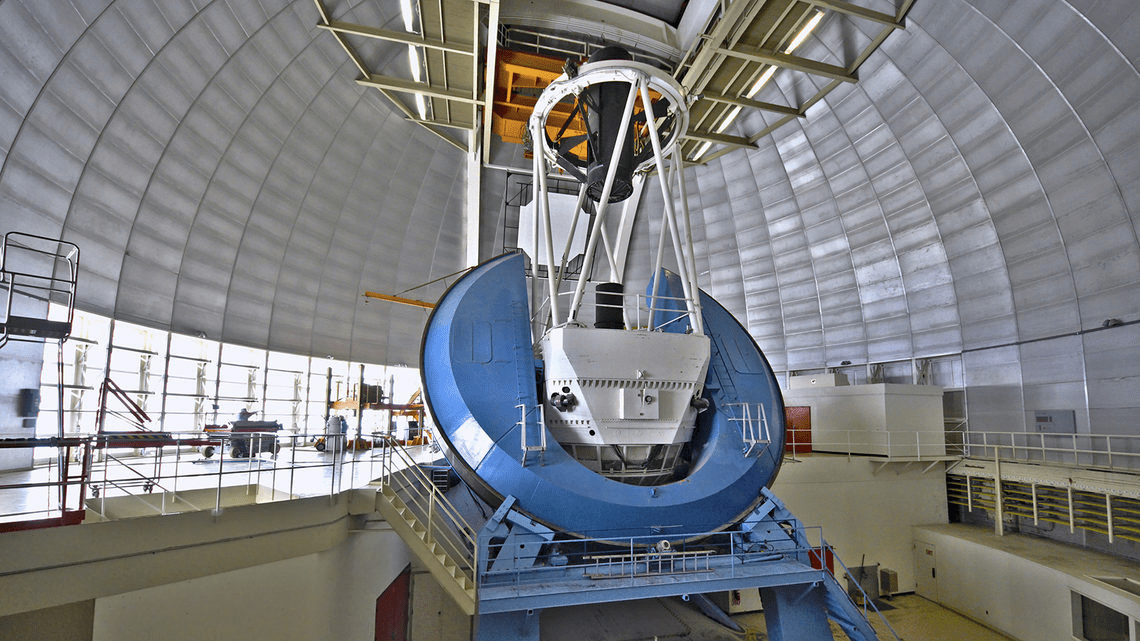

focal plane 5000 fibers
wide-field corrector
6 lenses, FoV \(\sim 8~\mathrm{deg}^{2}\)
Kitt Peak, AZ
4 m mirror

Mayall Telescope

focal plane 5000 fibers
fiber view camera
ten 3-channel spectrographs
49 m, 10-cable fiber run
Kitt Peak, AZ


Focal plane: 5000 robotic positioners



Focal plane: 5000 robotic positioners


Exposure time (dark): 1000 s
Configuration of the focal plane
CCD readout
Go to next pointing
140 s
0.1 mm

Spectroscopic pipeline

wavelength
fiber number


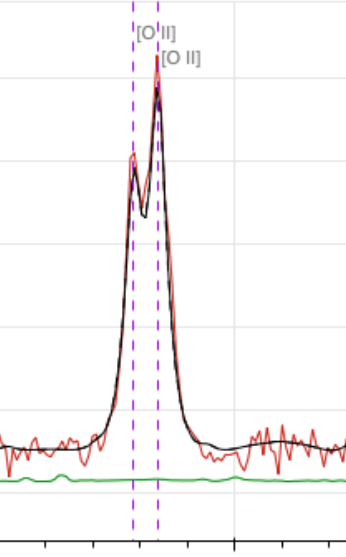
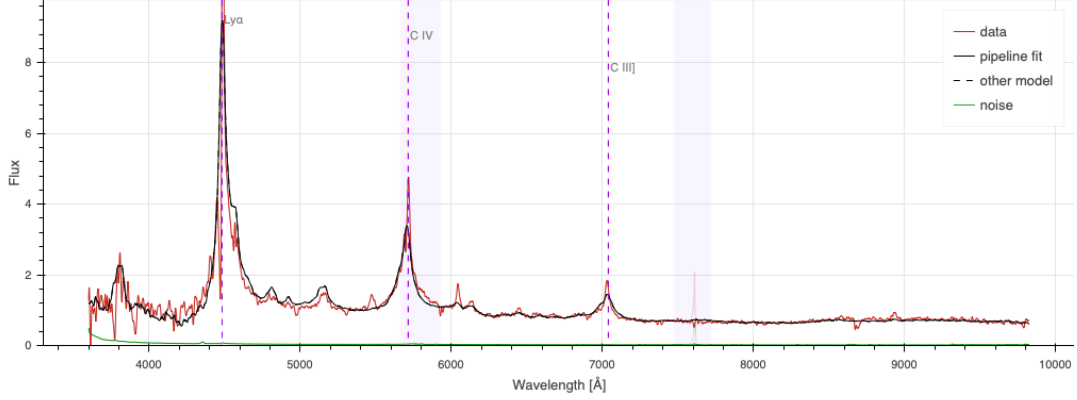
\(z = 2.1\) QSO
\(z = 0.9\) ELG
Ly\(\alpha\)
CIV
CIII
[OII] doublet at \(2727 \AA\) up to \(z = 1.6\)
[OII]
Ly\(\alpha\) at \(1216 \AA\) down to \(z = 2.0\)

DESI data release 1 (DR1)
Observations from May 14th 2021 to June 12th 2022

Final survey
- dark time (LRG, ELG, QSO): 7 layers
- bright time (BGS): 5 layers
- 14,000 \(\mathrm{deg}^2\)


Release of DESI DR1 (BAO) results
April 4th 2024
First batch of DESI DR1 cosmological analyses
https://data.desi.lbl.gov/doc/papers/
• DESI 2024 I: First year data release
• DESI 2024 II: Sample definitions and two-point clustering statistics
• DESI 2024 III: BAO from Galaxies and Quasars
• DESI 2024 IV: BAO from the Lyman-Forest
• DESI 2024 V: Full Shape measurements from Galaxies and Quasars
• DESI 2024 VI: Cosmological constraints from BAO measurements
• DESI 2024 VII: Constraints from the Full Shape measurements

Release of DESI DR1 (FS) results
November 17th 2024
Second batch of DESI DR1 cosmological analyses
https://data.desi.lbl.gov/doc/papers/
• DESI 2024 I: First year data release
• DESI 2024 II: Sample definitions and two-point clustering statistics
• DESI 2024 III: BAO from Galaxies and Quasars
• DESI 2024 IV: BAO from the Lyman-Forest
• DESI 2024 V: Full Shape measurements from Galaxies and Quasars
• DESI 2024 VI: Cosmological constraints from BAO measurements
• DESI 2024 VII: Constraints from the Full Shape measurements

Clustering analysis

galaxy catalog
galaxy power spectrum (or correlation function)
cosmological constraints
compression = "we measure specific features"

"variance of the density field as a function of scale"
Full Shape (baseline)
BAO
ShapeFit (alternative Full Shape)

Baryon acoustic oscillations
Sound waves in primordial plasma
At recombination (\(z \sim 1100\))
- plasma changes to optically thin
- baryons decouple from photons
- sound wave stalls
spherical shell in the distribution of galaxies, of radius the distance that sound waves travelled
= sound horizon scale at the drag epoch \( r_\mathrm{d} \sim 150 \; \mathrm{Mpc} \sim 100 \; \mathrm{Mpc}/h \)
standard ruler

BAO measurements
- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)



transverse comoving distance
sound horizon \(r_d\)

BAO measurements
- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)



Hubble distance
sound horizon \(r_d\)

- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)
At multiple redshifts \(z\)
BAO measurements







Probes the expansion history, hence the energy content
Absolute size at \(z = 0\): \(H_0 r_d\)

Release of DESI DR1 (BAO) results
April 4th 2024
First batch of DESI DR1 cosmological analyses
https://data.desi.lbl.gov/doc/papers/
• DESI 2024 I: First year data release
• DESI 2024 II: Sample definitions and two-point clustering statistics
• DESI 2024 III: BAO from Galaxies and Quasars
• DESI 2024 IV: BAO from the Lyman-Forest
• DESI 2024 V: Full Shape measurements from Galaxies and Quasars
• DESI 2024 VI: Cosmological constraints from BAO measurements
• DESI 2024 VII: Constraints from the Full Shape measurements

Correlation functions




BAO peak
Excess probability to find 2 galaxies separated by a distance s

Power spectra



BAO wiggles
Fourier transform of the correlation function

Some fits: correlation function

isotropic measurement
anisotropic measurement

Density field reconstruction
Non-linear structure growth and peculiar velocities blur and shrink (slightly) the ruler
Reconstruction: estimate Zeldovich displacements from observed field and moves galaxies back \(\rightarrow\) refurbishes the ruler (improves precision and accuracy)





reconstruction

Density field reconstruction


DR1 BAO analysis: what's new?
- Biggest ever spectroscopic BAO dataset (\(N_\mathrm{tracer}\) and \(V\))

5.7 million unique redshifts
Effective volume \(V_\mathrm{eff} = 18 \; \mathrm{Gpc}^{3}\)
\(3 \times \) bigger than SDSS!

- Biggest ever spectroscopic BAO dataset (\(N_\mathrm{tracer}\) and \(V\))
- Blind analysis to mitigate observer / confirmation biases (catalog-level blinding)
- Theory developments in BAO fitting code
- New and improved reconstruction methods
- New combined tracer method used for overlapping galaxy samples (LRG and ELG in \(0.8 < z < 1.1\))
- Unified BAO pipeline applied to all (discrete) tracer / redshift bins consistently
DR1 BAO analysis: what's new?

Tests of systematic errors
Considered many possible sources of systematic errors using simulations and data:
- observational effects (imaging systematics, fiber collisions)
- BAO reconstruction (2 algorithms compared)
- covariance matrix construction
- incomplete theory modelling
- choice of fiducial cosmology
- galaxy-halo (HOD) model uncertainties
no systematics detected
systematics << statistics
Maximum effect: \(\sigma_\mathrm{stat. + syst.} < 1.05 \sigma_\mathrm{stat.}\)

Release of DESI DR1 (BAO) results
April 4th 2024
First batch of DESI DR1 cosmological analyses
https://data.desi.lbl.gov/doc/papers/
• DESI 2024 I: First year data release
• DESI 2024 II: Sample definitions and two-point clustering statistics
• DESI 2024 III: BAO from Galaxies and Quasars
• DESI 2024 IV: BAO from the Lyman-Forest
• DESI 2024 V: Full Shape measurements from Galaxies and Quasars
• DESI 2024 VI: Cosmological constraints from BAO measurements
• DESI 2024 VII: Constraints from the Full Shape measurements

Ly\(\alpha\) forest
Absorption in QSO spectra by neutral hydrogen in the intergalactic medium: \(\lambda_\mathrm{abs} = (1 + z_\mathrm{HI}) \times 1215.17 \; \AA \)
Transmitted flux fraction \(F = e^{-\tau}\) probes the fluctuation in neutral hydrogen density, \(\tau \propto n_\mathrm{HI} \)
credit: Andrew Pontzen

Ly\(\alpha\) correlation functions in DESI DR1




Ly\(\alpha\) - Ly\(\alpha\)
Ly\(\alpha\) - QSO
QSO
QSO
HI cloud
HI cloud
HI cloud
QSO



DR1 Ly\(\alpha\) BAO analysis: what's new?
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
>420,000 Ly\(\alpha\) QSO at z > 2.1
\(2 \times \) more than SDSS!


- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
DR1 Ly\(\alpha\) BAO analysis: what's new?

- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function: cosmological signal, and many contaminants!
- Very stable results, systematic uncertainty neglected
DR1 Ly\(\alpha\) BAO analysis: what's new?

- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)
- low S/N, isotropic average: \( D_\mathrm{V}(z) / r_\mathrm{d} = (z D_{\mathrm{M}}^{2}(z) D_\mathrm{H}(z))^{1/3} / r_\mathrm{d}\)
BAO measurements
Let's factor out the \(h\) terms:
- \(\color{blue}{[D_\mathrm{M}(z) h] (\Omega_\mathrm{m}, f_\mathrm{DE}, \Omega_\mathrm{K}, ...)} \color{black}{/} \color{orange}{[r_\mathrm{d}(\Omega_\mathrm{m} h^{2}, \Omega_\mathrm{b} h^{2}) h]} \)
- \( \color{blue}{[D_\mathrm{H}(z) h] (\Omega_\mathrm{m}, f_\mathrm{DE}, \Omega_\mathrm{K}, ...)} \color{black}{/} \color{orange}{[r_\mathrm{d}(\Omega_\mathrm{m} h^{2}, \Omega_\mathrm{b} h^{2}) h]} \)
BAO measurements at different \(z\) constrain:
- energy content \( \color{blue}{(\Omega_\mathrm{m}, f_\mathrm{DE}, ...)} \)
- constant-over-\(z\) product \(\color{orange}{r_\mathrm{d} h}\) i.e. \(\color{orange}{H_{0} r_\mathrm{d}}\)


DESI DR1 BAO

DESI DR1 BAO measurements



DESI DR1 BAO
DESI DR1 BAO measurements



DESI DR1 BAO
DESI DR1 BAO measurements



DESI DR1 BAO
DESI DR1 BAO measurements



DESI DR1 BAO
DESI DR1 BAO measurements



DESI DR1 BAO
DESI DR1 BAO measurements

Consistent with each other,
and complementary

DESI DR1 BAO
DESI DR1 BAO measurements
Consistency with other probes


DESI DR1 BAO consistent with:
Consistency with other probes


DESI DR1 BAO consistent with:
Consistency with other probes

DESI DR1 BAO consistent with:
- SDSS eBOSS Collaboration, 2020
- primary CMB: Planck Collaboration, 2018 and CMB lensing: Planck PR4 + ACT DR6 lensing ACT Collaboration, 2023, Carron, Mirmelstein, Lewis, 2022


- BAO constrains \( r_\mathrm{d}(\blue{\Omega_\mathrm{m}} h^{2}, \orange{\Omega_\mathrm{b} h^{2}}) h \)
- \( \blue{\Omega_\mathrm{m}} \) constrained by BAO at different \(z\)
- \(\orange{\Omega_\mathrm{b}h^2}\) can be constrained by light element abundance from Big Bang Nucleosynthesis (BBN): Schöneberg 2024
\(\implies\) constraints on \(h\) i.e. \(H_0 = 100 h \; \mathrm{km} / \mathrm{s} / \mathrm{Mpc}\)
Hubble constant

Hubble constant
\(\theta_\ast\) CMB angular acoustic scale


- Consistency with SDSS
Hubble constant


- Consistency with SDSS
- In agreement with CMB
- In \(3.7 \sigma\) tension with SH0ES
Hubble constant


Release of DESI DR1 (FS) results
November 17th 2024
Second batch of DESI DR1 cosmological analyses
https://data.desi.lbl.gov/doc/papers/
• DESI 2024 I: First year data release
• DESI 2024 II: Sample definitions and two-point clustering statistics
• DESI 2024 III: BAO from Galaxies and Quasars
• DESI 2024 IV: BAO from the Lyman-Forest
• DESI 2024 V: Full Shape measurements from Galaxies and Quasars
• DESI 2024 VI: Cosmological constraints from BAO measurements
• DESI 2024 VII: Constraints from the Full Shape measurements

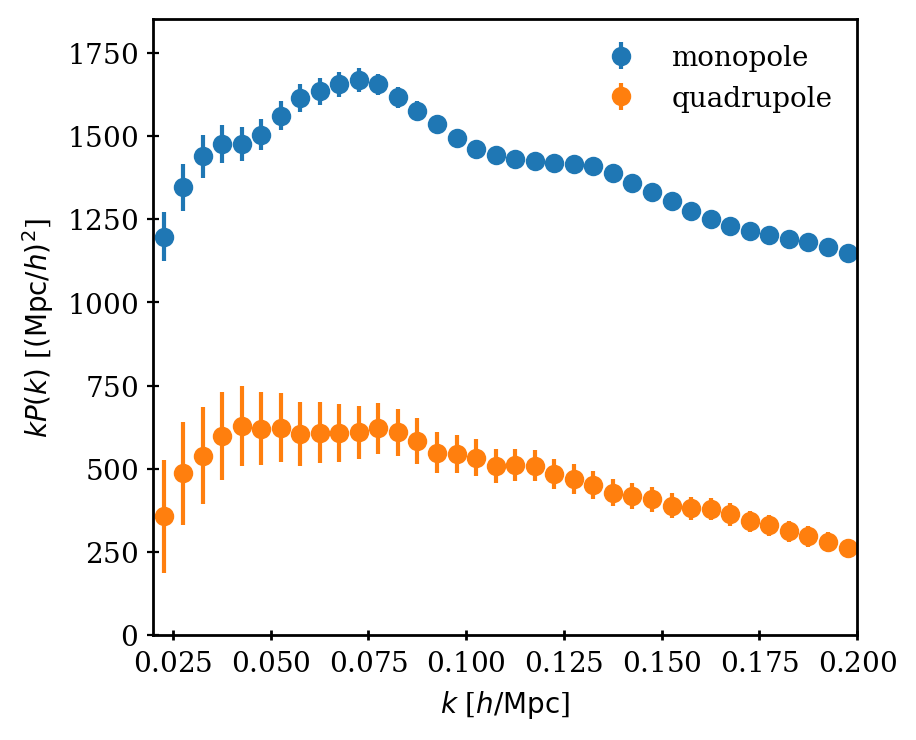
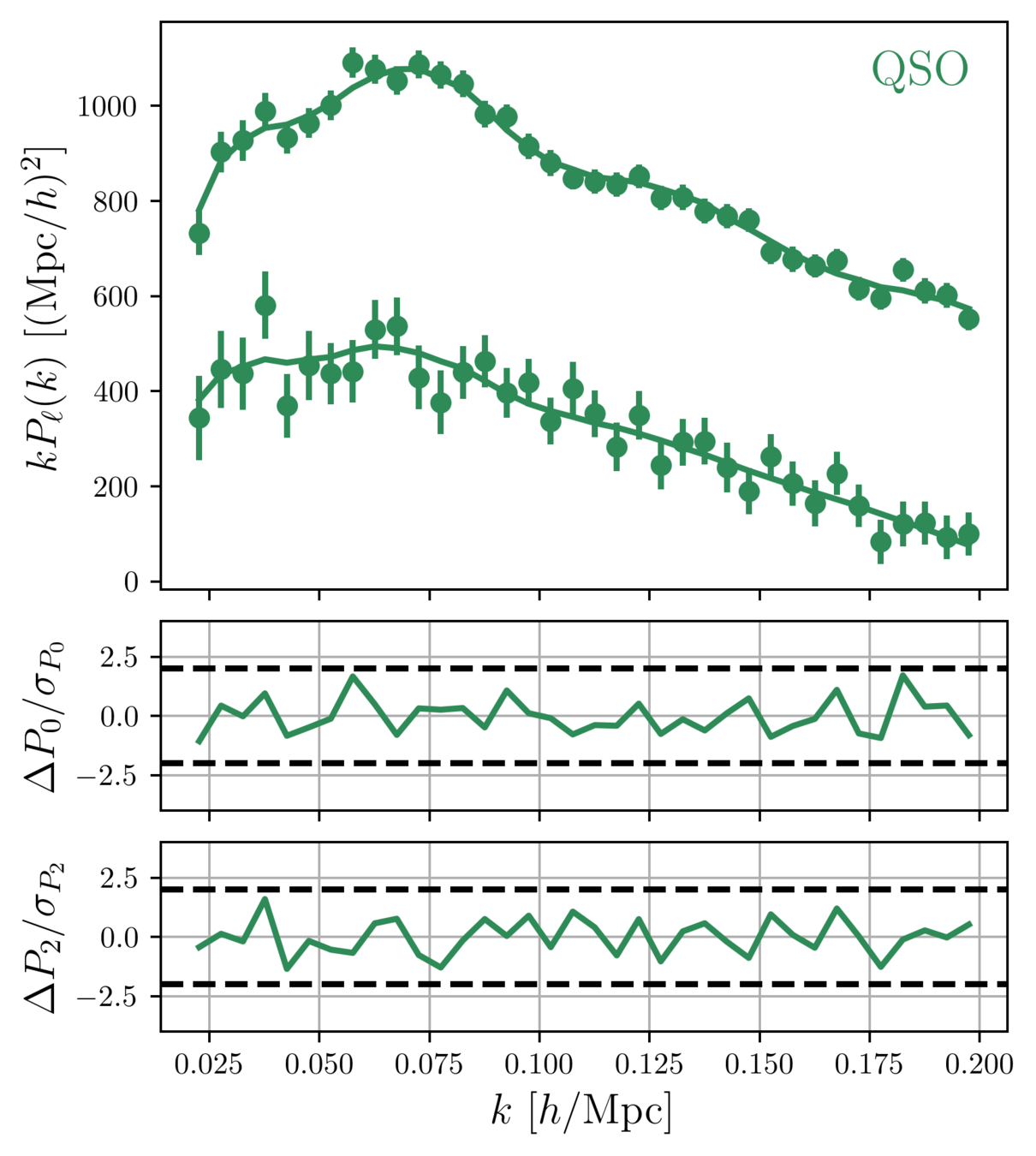
Full Shape measurements
clustering



We fit the "full shape" (FS) of the galaxy power spectrum multipoles


Full Shape measurements






RSD
observed redshift = Hubble flow and peculiar velocities (RSD = "redshift space distortions")
We fit the "full shape" (FS) of the galaxy power spectrum multipoles
shape
(\( \Omega_\mathrm{cdm} h^2, \Omega_\mathrm{b} h^2, n_\mathrm{s}, \sum m_\nu \))
growth of structure \(f\sigma_8\) sensitive to the theory of gravity and dark energy

Full Shape models
Three power spectrum Effective Field Theory models considered:
- velocileptors Maus et al. 2024
- folps Noriega et al. 2024
- pybird Lai et al. 2024

credit: Mark Maus, Hernan Noriega, Yan Lai
Comparison paper:

Full Shape models
perturbation theory term
linear and quasi-linear physics
counter-terms contribution
truncation of perturbative series
stochastic-terms contribution
small-scale galaxy physics
The Effective Field Theory in a nutshell
- perturbation theory model + counter-terms and stochastic terms
- dependence on cosmology into \(P_\mathrm{lin}\), \(f\) and Alcock-Paczynski parameters (\(\mathrm{R.A.}, \mathrm{Dec.}, z \Rightarrow \mathrm{distance}\))

- Biggest ever spectroscopic dataset (\(N_\mathrm{tracer}\) and \(V\))
- Blind analysis to mitigate observer / confirmation biases (catalog-level blinding)
- Effective Field Theory models
- Full-Modelling (\(\Omega_\mathrm{cosmo}\)) and updated compression approach (ShapeFit)
- Improvements in the treatment of observational systematics (e.g. fiber assignment)
- Unified Full Shape pipeline applied to all (discrete) tracer / redshift bins consistently
DR1 Full Shape analysis: what's new?

Groups of galaxies too close to each other cannot all receive a fiber
\(0.05^\circ \simeq\) positioner patrol diameter
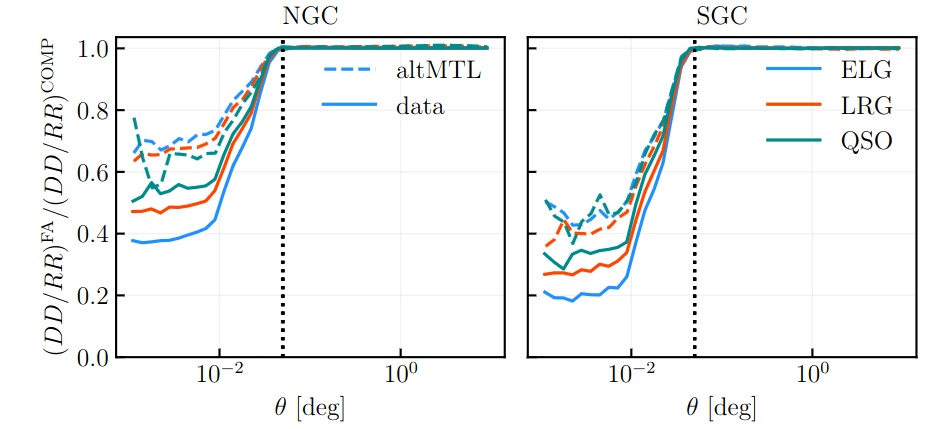

Fiber assignment
Fiber assignment

Impacts power spectrum measurements (altMTL vs complete)


Impacts power spectrum measurements (altMTL vs complete)
Solution: \(\theta\)-cut = remove all pairs \(< 0.05^\circ\)

Fiber assignment

New window matrix \(W^\mathrm{cut}\); \(\langle P_o(k) \rangle = W^\mathrm{cut}(k, k^\prime) P_t(k^\prime)\)

Fiber collisions

New window matrix \(W^\mathrm{cut}\); \(\langle P_o(k) \rangle = W^\mathrm{cut}(k, k^\prime) P_t(k^\prime)\)
Very non diagonal: let's "rotate" it


Fiber collisions

Systematic effects
-
Theoretical modelling (Maus et al. 2024ab, Lai et al. 2024, Noriega et al. 2024, Ramirez et al. 2024)
-
Galaxy-halo connection (Findlay et al. 2024)
-
Fiducial cosmology (Gsponer et al. 2024)
-
Fibre assignment (Pinon et al. 2024)
-
Inhomogeneities in the target selection (Zhao et al. 2024)
-
Spectroscopic redshift failures/uncertainties (Yu et al. 2024, Krowleski et al. 2024)
-
Covariance matrix: mock-based vs analytic (Forero-Sanchez et al. 2024, Alves et al. 2024, Rashkovetskyi et al. 2024)
Total systematic error = ⅖ of DR1 statistical uncertainty
no systematics detected

Full Shape (+ BAO) measurements
-
Observable: power spectrum monopole and quadrupole, post-reconstruction BAO
-
Model: Effective Field Theory
-
Covariance: mock-based
-
Systematic error: at the data vector level
-
Fitting range: \(0.02 < k\, [h/\mathrm{Mpc}] < 0.2\)
-
Fitting parameters:
-
7 nuisance parameters
-
5 \(\Lambda\)CDM parameters
-

External information
\(\Omega_\mathrm{b} h^2\): BBN from Schöneberg 2024
\(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\): "\(n_{\mathrm{s}10}\)" \(10\times\) wider than Planck posterior

Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
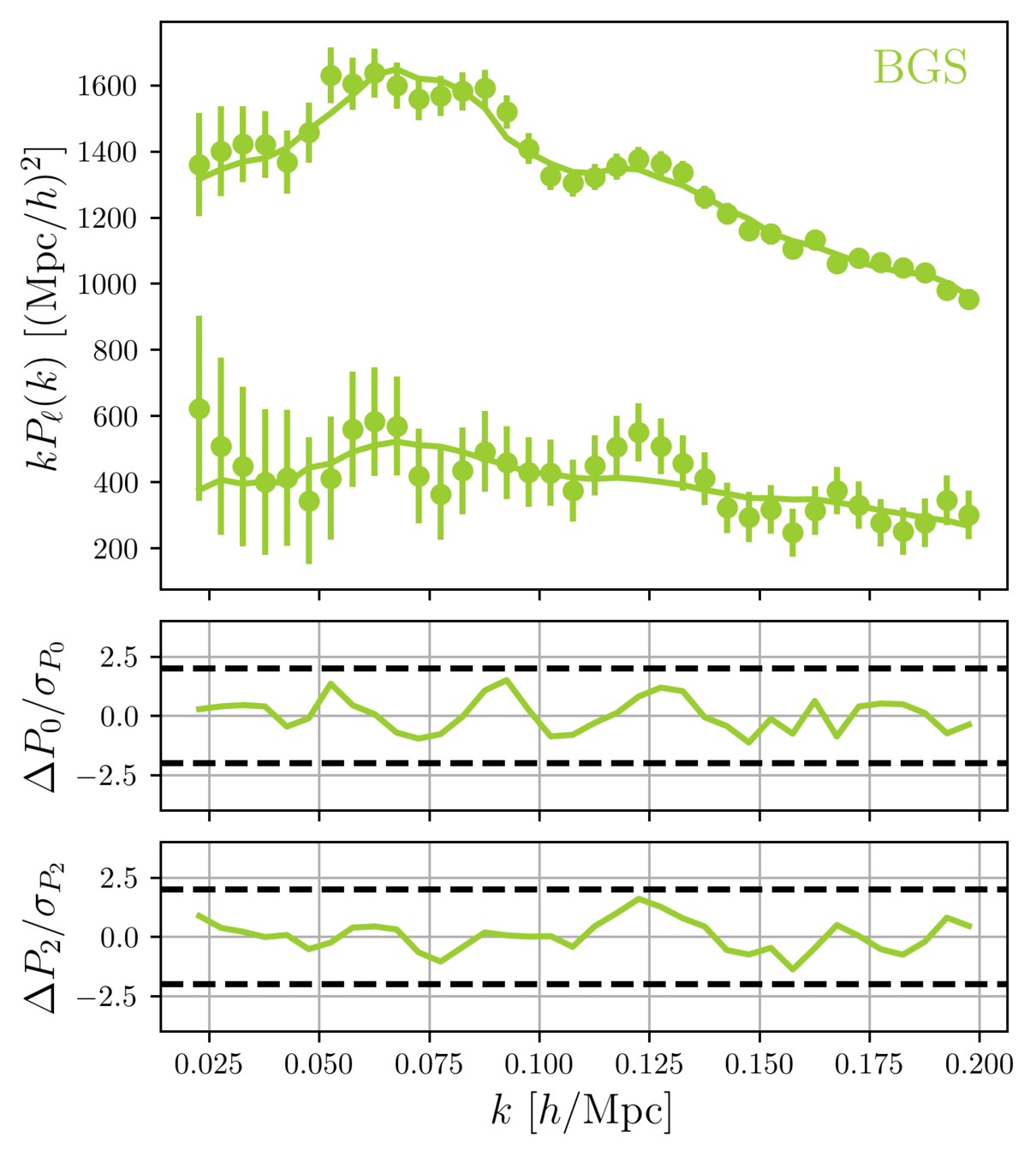
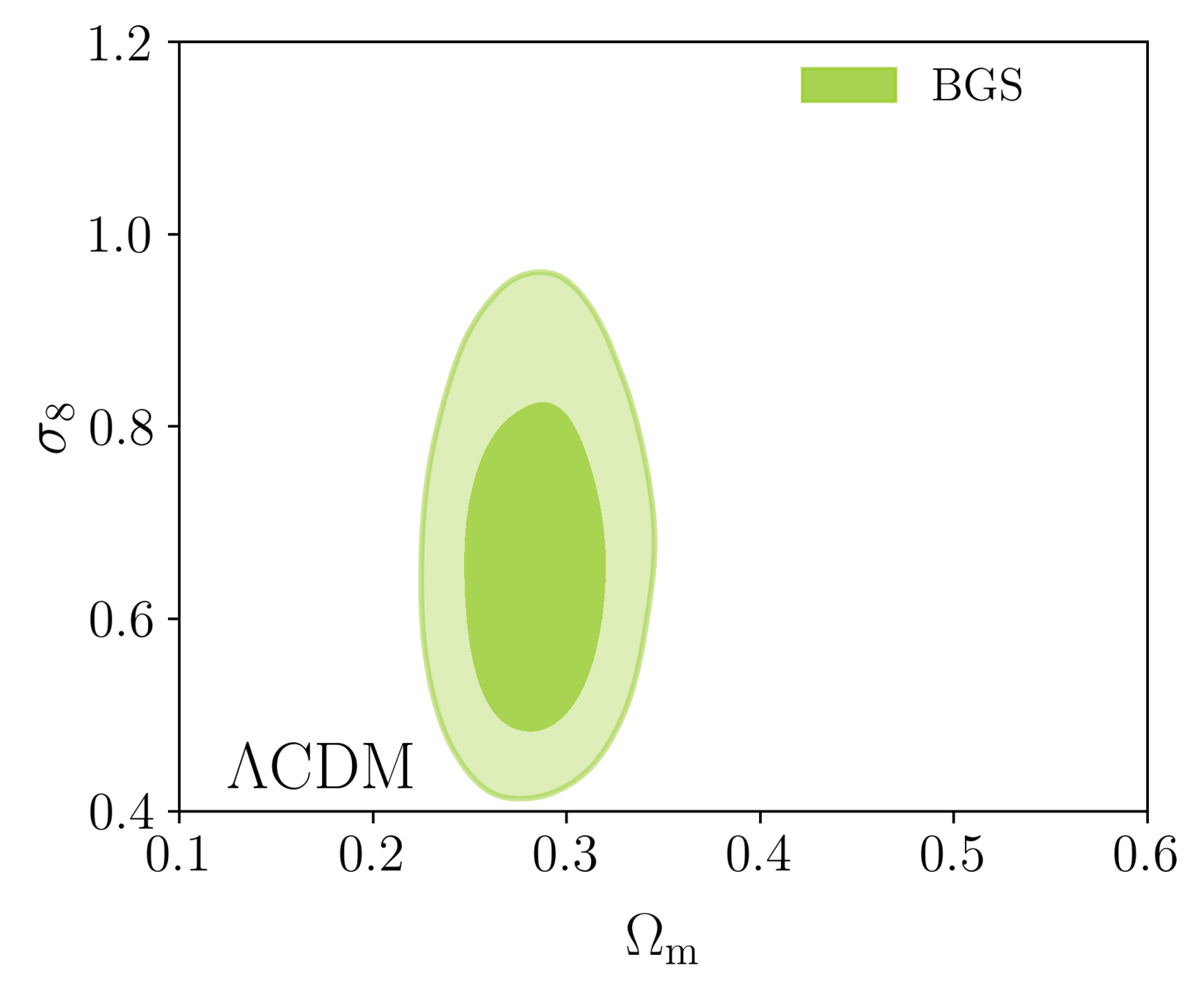


Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)




Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
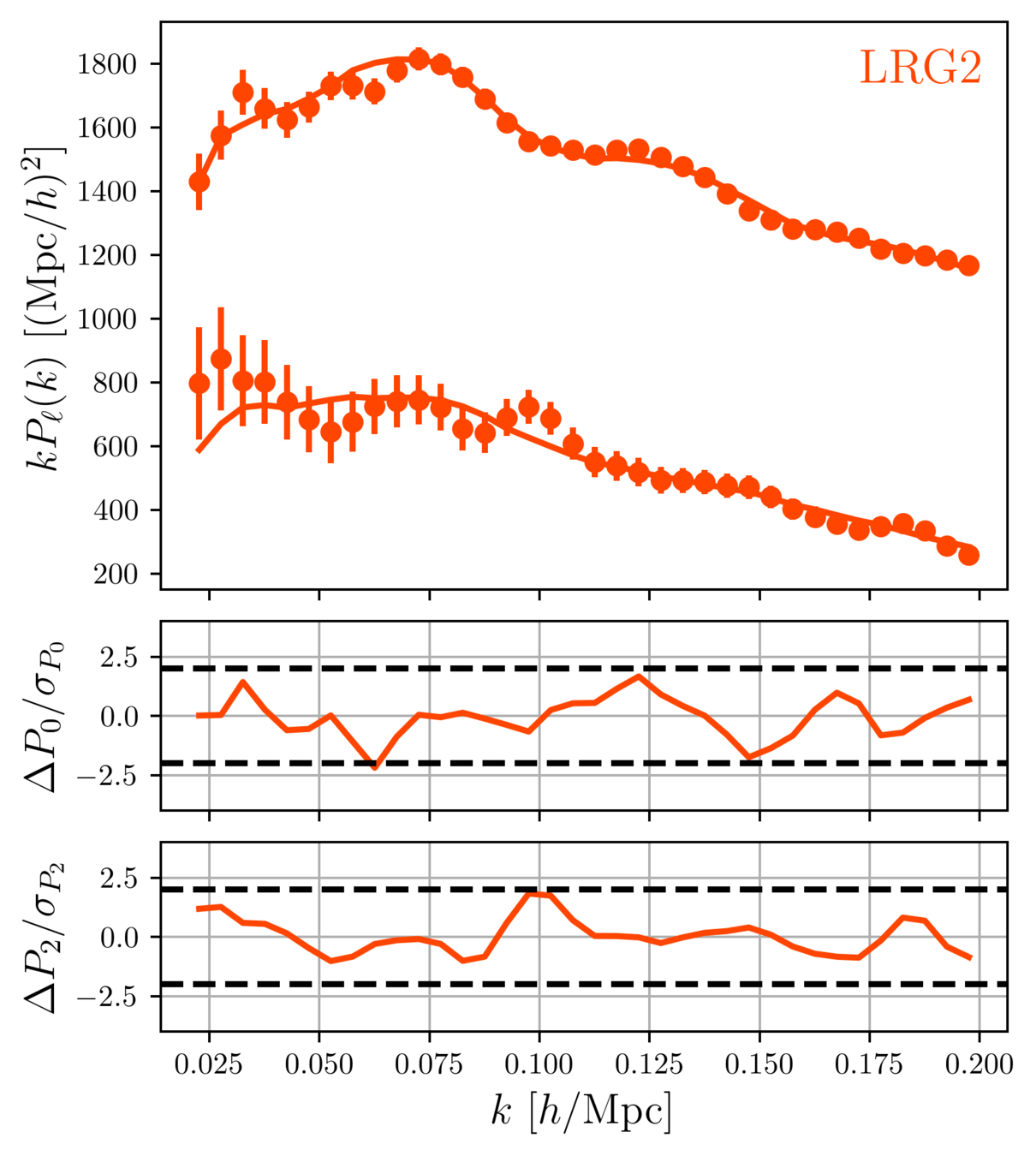
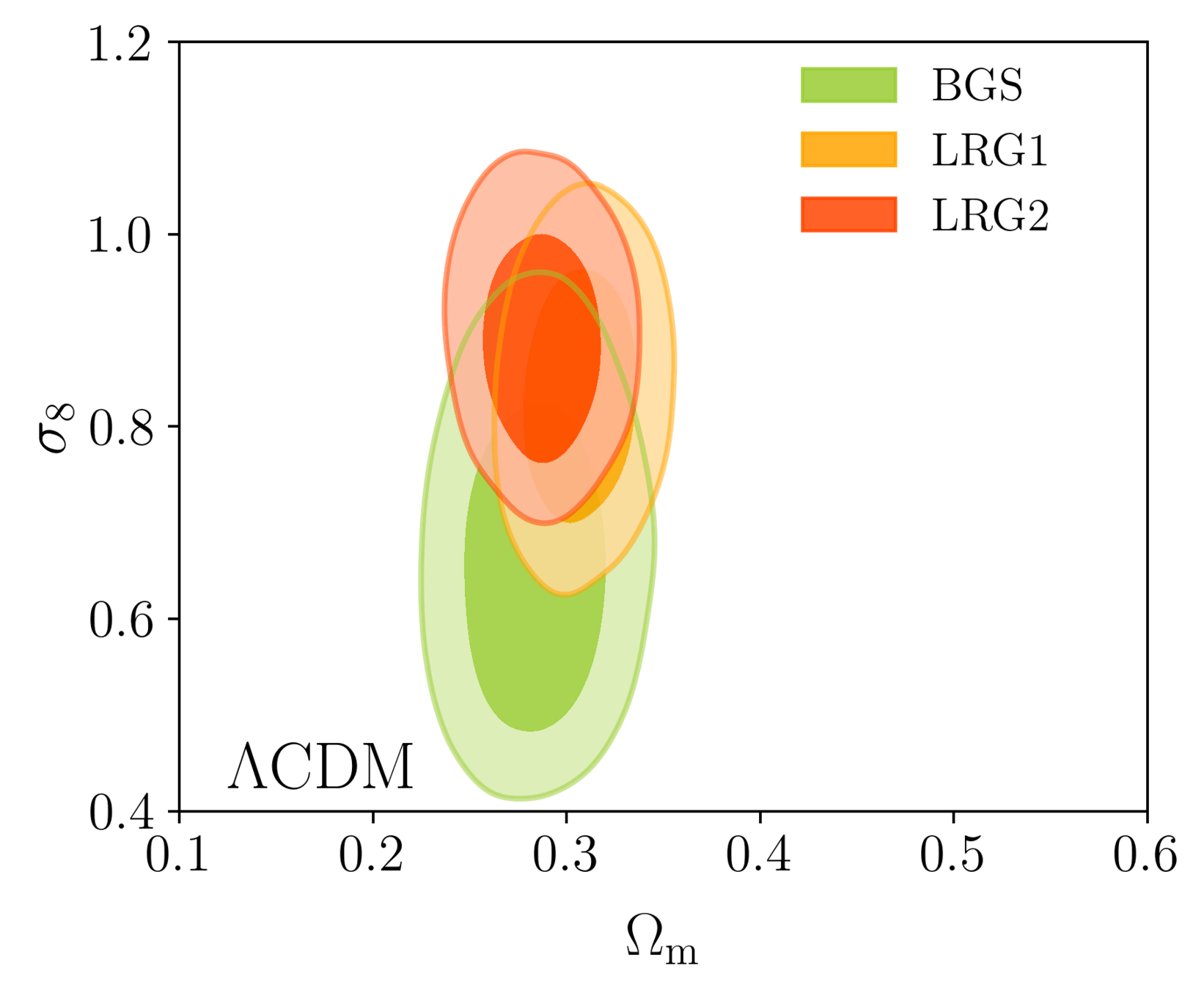


Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
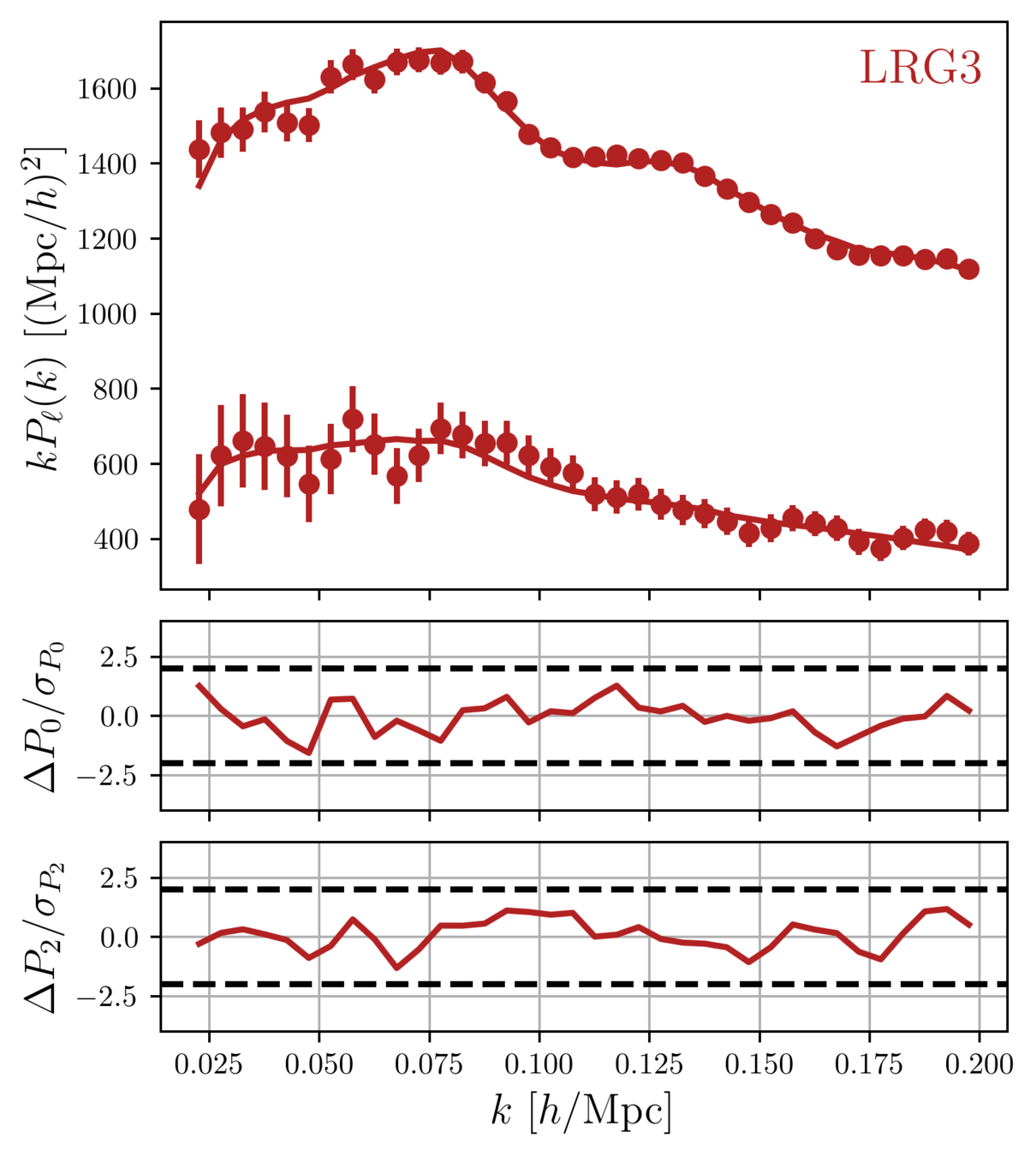


Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)

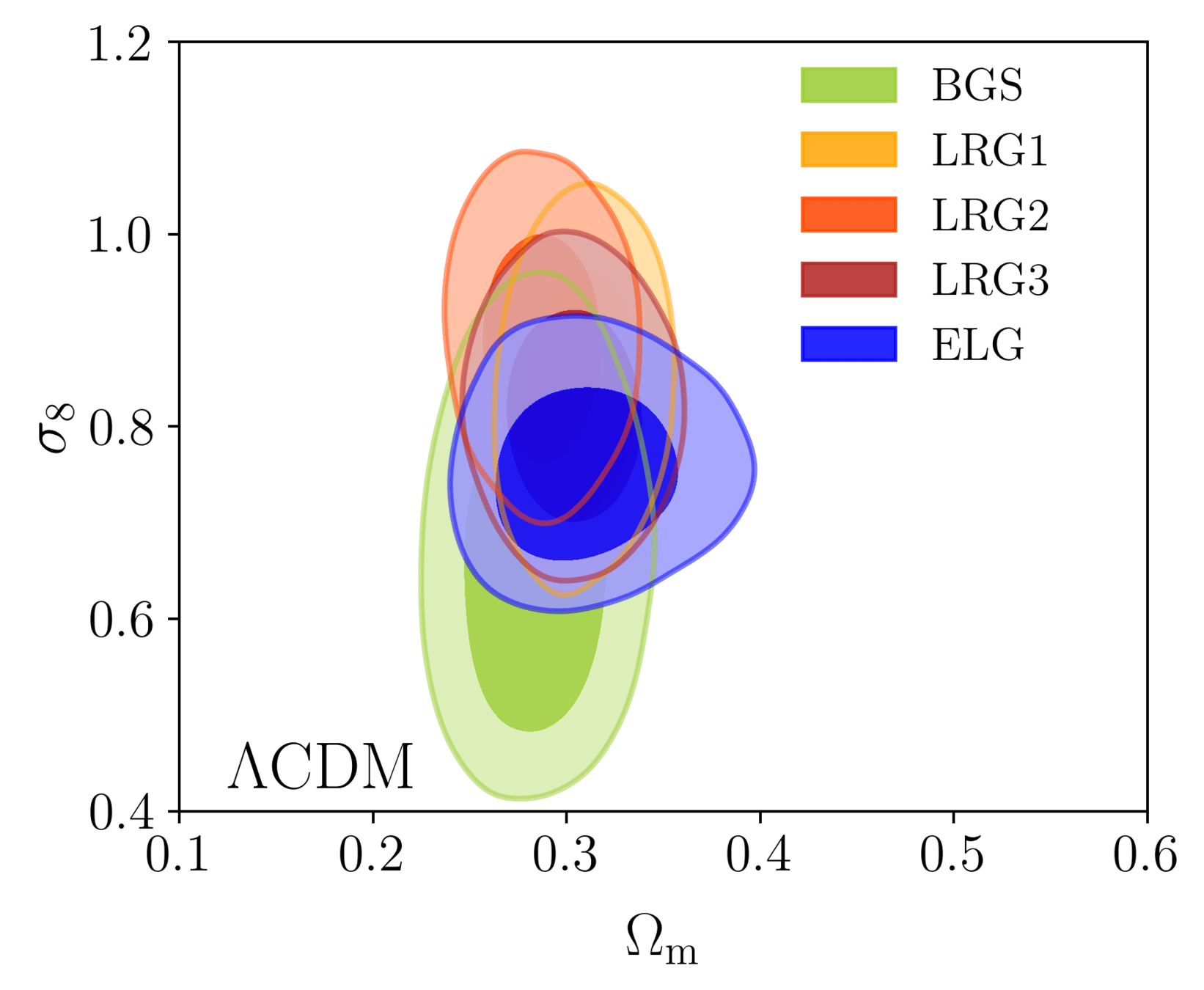

Full Shape + BAO measurements
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)

Full Shape + BAO measurements

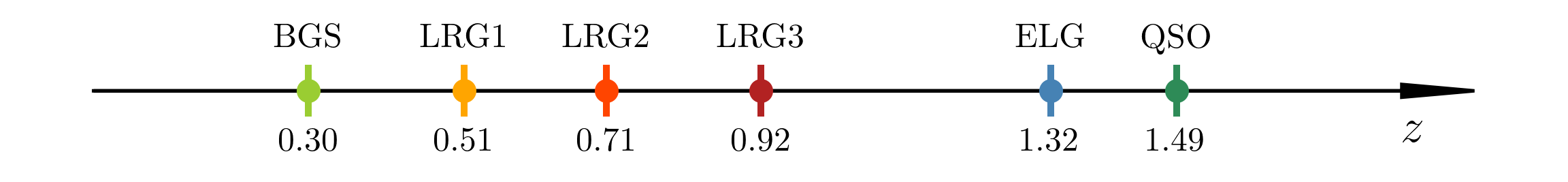
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)

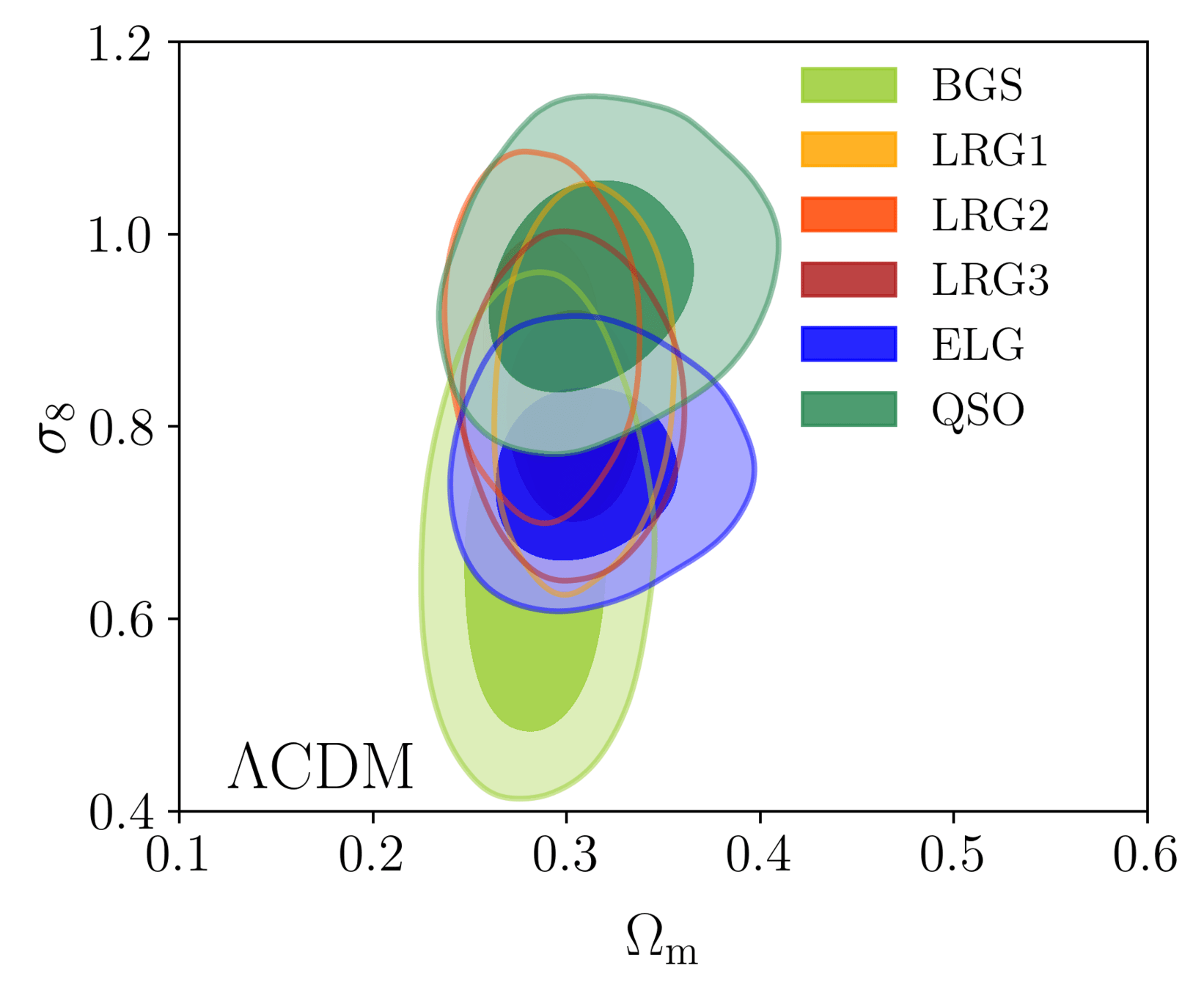
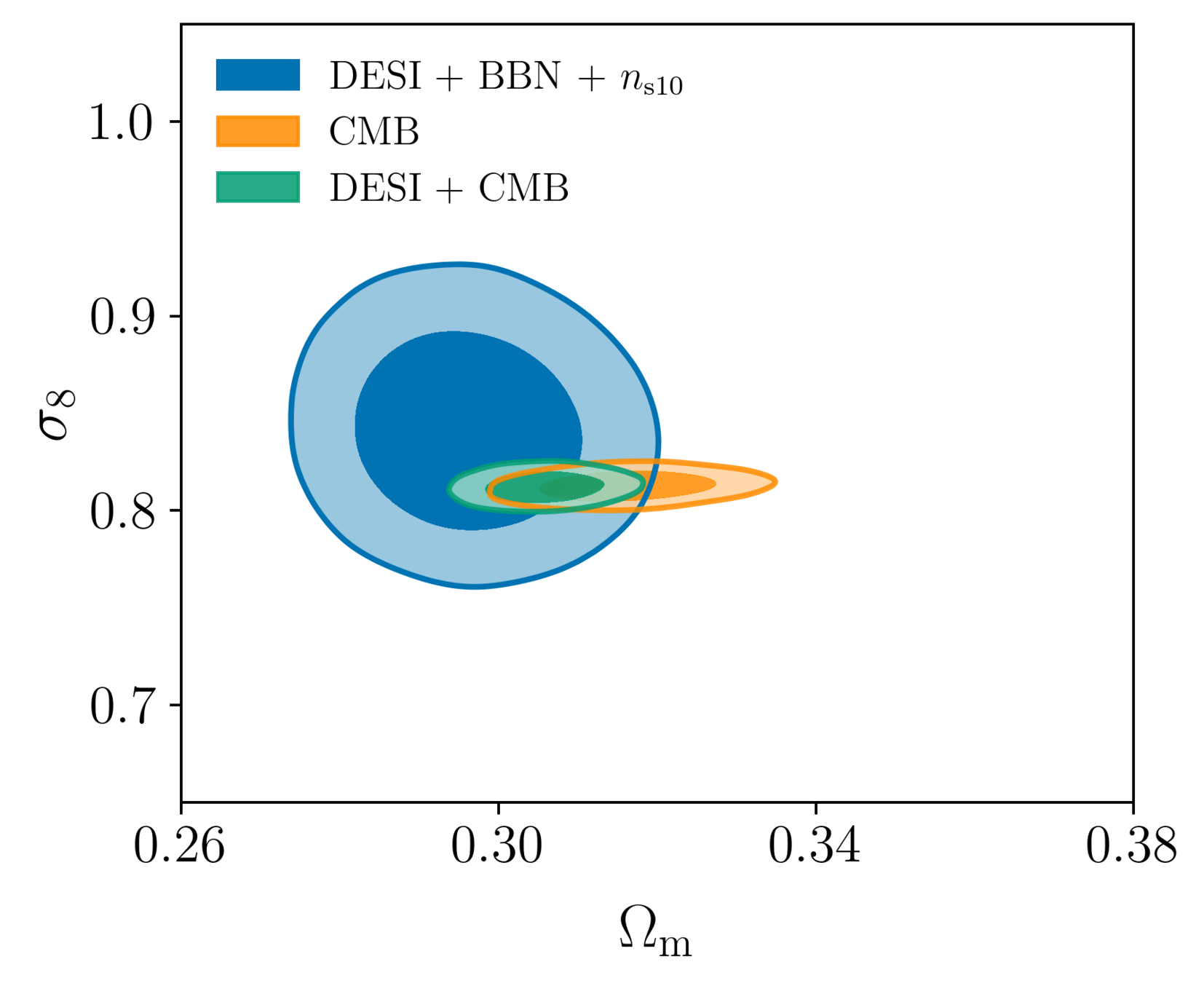
\(S_8\) constraints

\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

\(S_8\) constraints
- Consistency with SDSS
- In agreement with CMB
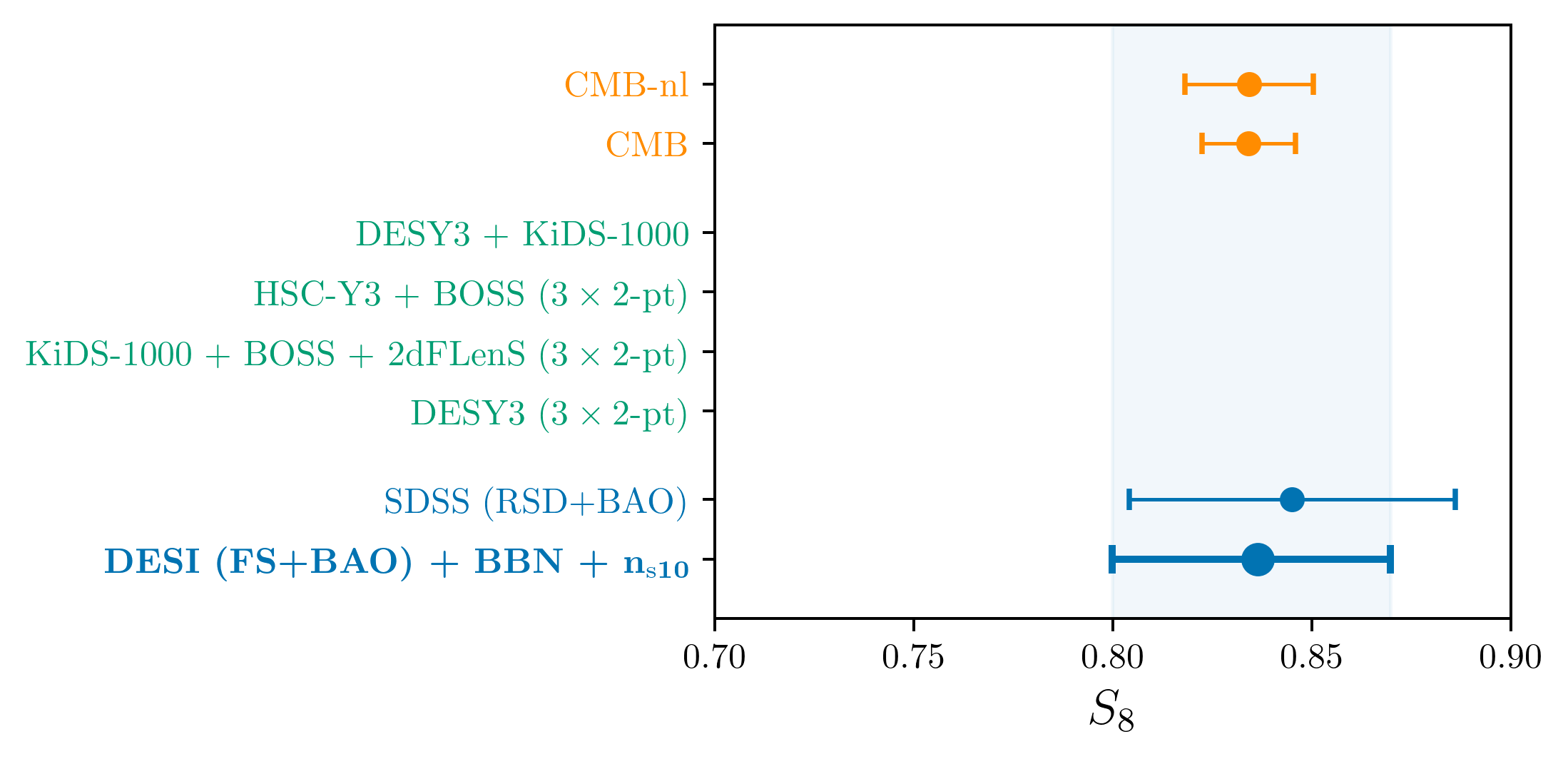
\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

\(S_8\) constraints
- Consistency with SDSS
- In agreement with CMB
- Weak lensing prefers lower \(S_8\), but still consistent
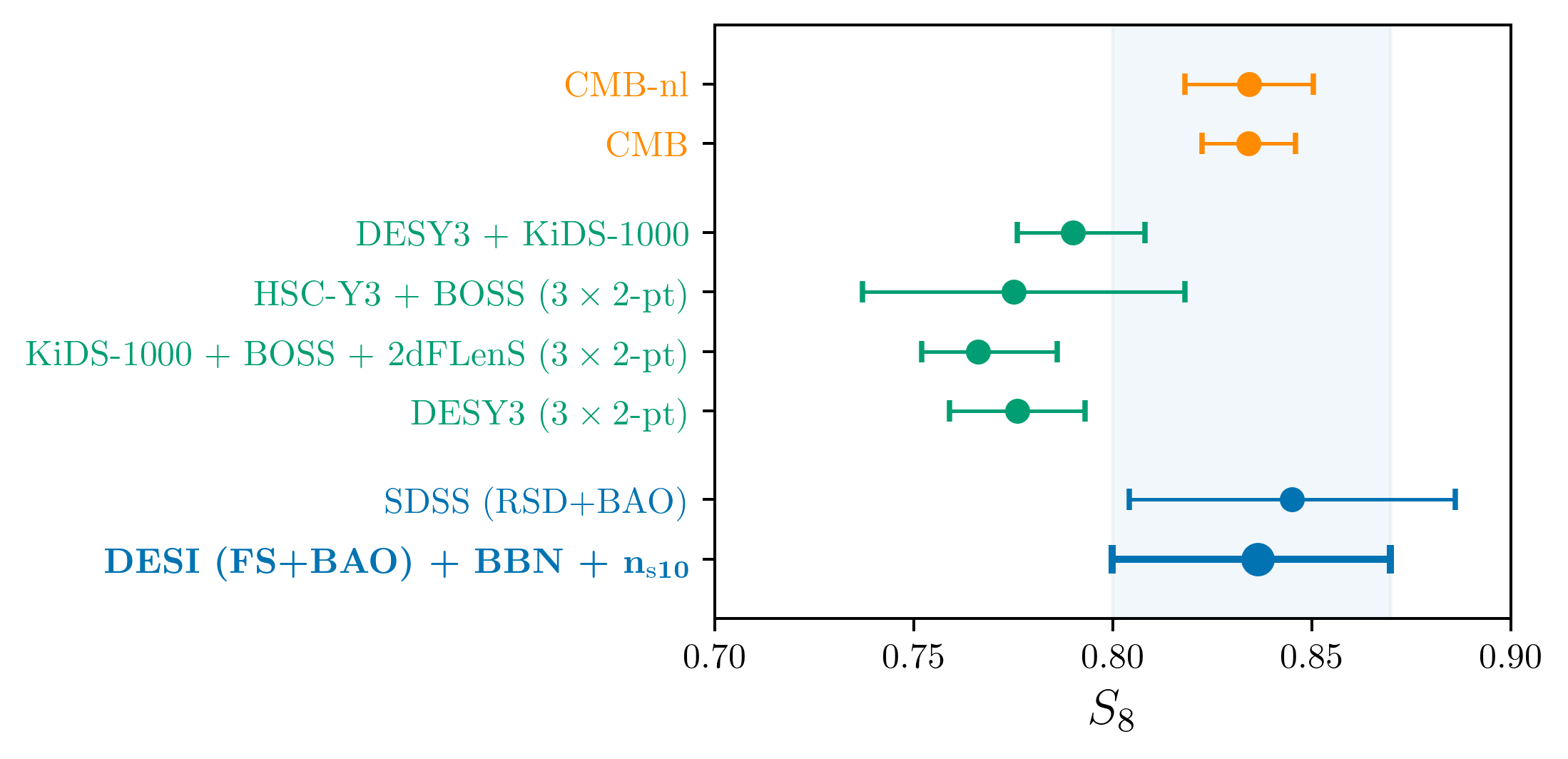
\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

Dark Energy fluid, pressure \(p\), density \(\rho\)
Equation of State parameter \(w = p / \rho\)
Linked to the evolution of Dark Energy \(w(z) = -1 + \frac{1}{3}\frac{d \ln f_\mathrm{DE}(z)}{d \ln (1 + z)}\)
Let's assume the CPL parameterization
Dynamical Dark Energy - \((w_{0}, w_{a})\)
\(\Lambda\)CDM: \((w_0, w_a) = (-1, 0)\)

Dynamical Dark Energy - \((w_{0}, w_{a})\)
DESI + CMB + SN (uncalibrated):

\(\Lambda\)CDM

Dynamical Dark Energy - \((w_{0}, w_{a})\)
- same preference for \(w_{0} > -1, w_{a} < 0\)
- similar significance for \(w_0w_a\)CDM vs \(\Lambda\)CDM
- \(20\%\) better constraints in \((w_0, w_a)\) than without FS
\(2.5\sigma\)
\(3.4\sigma\)
\(3.8\sigma\)


Sum of neutrino masses
Massive neutrinos impact:
i) the expansion history
ii) the growth of structure: \( \Delta P(k)/P(k) \propto -\sum m_\nu / \omega_\mathrm{m} \)
the \(k\)-fitting range


Sum of neutrino masses
Massive neutrinos impact:
i) the expansion history
ii) the growth of structure: \( \Delta P(k)/P(k) \propto -\sum m_\nu / \omega_\mathrm{m} \)
Taking \(n_\mathrm{s}\) prior from Planck:


Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by DESI, especially through \(H_{0}\)

Sum of neutrino masses
Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by DESI, especially through \(H_{0}\)

Low preferred value of \(H_{0}\) yields
\(\sum m_\nu < 0.071 \, \mathrm{eV} \; (95\%, \color{green}{\text{DESI + CMB})}\)
(\(15\%\) better than BAO-only: \(0.082 \, \mathrm{eV} \))
\(\sum m_\nu < 0.081 \, \mathrm{eV} \; (95\%, \color{green}{\text{DESI + CMB[hillipop]})}\)
In \(w_0w_a\mathrm{CDM}\), with DES-SN5YR: \(\sim 0.2\, \mathrm{eV} \; (95\%)\)

Modified gravity constraints
In general relativity, \(\green{\mu(a, k)} = \green{\Sigma(a, k)} = 1\)
To test GR, introduce \(\green{\mu_0, \Sigma_0}\)
Perturbed FLRW metric
\(ds^2=a(\tau)^2[-(1+2\orange{\Psi})d\tau^2+(1-2\orange{\Phi})\delta_{ij}dx^i dx^j]\)
At late times:
(mass) \(k^2\orange{\Psi} = -4\pi G a^2 \green{\mu(a,k)} \blue{\sum_i\rho_i\Delta_i}\)
(light) \(k^2(\orange{\Phi} + \orange{\Psi})=-8\pi G a^2 \green{\Sigma(a,k)} \blue{\sum_i\rho_i\Delta_i}\)
gravitational potentials
density perturbations
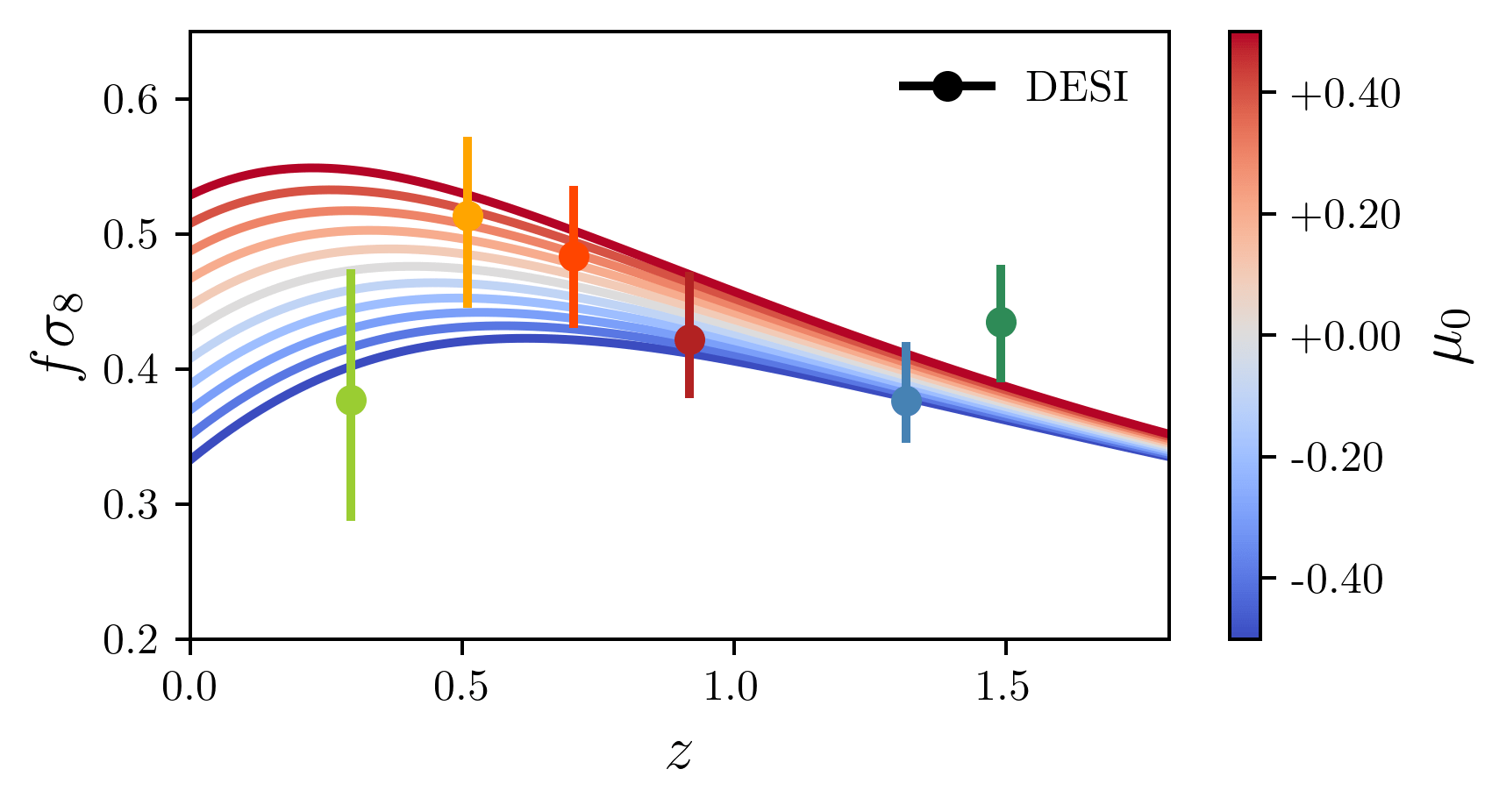

Modified gravity constraints

DESI constrains
GR

Modified gravity constraints
\(\Sigma_0\) constrained by
- CMB (ISW and lensing)
- galaxy lensing

DESI constrains

Modified gravity constraints
\(\Sigma_0\) constrained by
- CMB (ISW and lensing)
- galaxy lensing

compared to CMB-nl + DESY3 (3x2pt) only: \(\sigma(\mu_0) / 2.5\), \(\sigma(\Sigma_0) / 2\)
DESI constrains

Conclusions
BAO
- compared to Planck: low \(\Omega_\mathrm{m}\), high \(H_{0}\)
- hint of dynamical dark energy (depending on SN dataset)
Adding Full Shape
- \(\sigma_8, S_8\) consistent with Planck
- modified gravity \(\mu_0\) parameter consistent with GR
- small improvements in \(w_0, w_a\) and \(\sum m_\nu\) constraints

What to do next?
DR2 data (Y3 > Y1) on disk, DR2 BAO analysis on-going... stay tuned!
DR2 analyses will include joint 2-pt, 3-pt measurements: new challenges!
Possible improvements to the current analyses:
- more / improved mocks
- faster cosmological inference with emulators / JAX
- simplify \(P(k)\) and associated window measurements
\(f_\mathrm{NL}^\mathrm{loc}\) - mock constraints


Chaussidon et al. 2024, in prep
DR1: \(\sigma(f_\mathrm{NL}^\mathrm{loc}) \sim 10\)
SDSS: \(\sigma(f_\mathrm{NL}^\mathrm{loc}) \sim 20\)
LRG
QSO

Successfully removes the \( > 1 \sigma\) bias

credit: Ruiyang Zhao
Fiber collisions


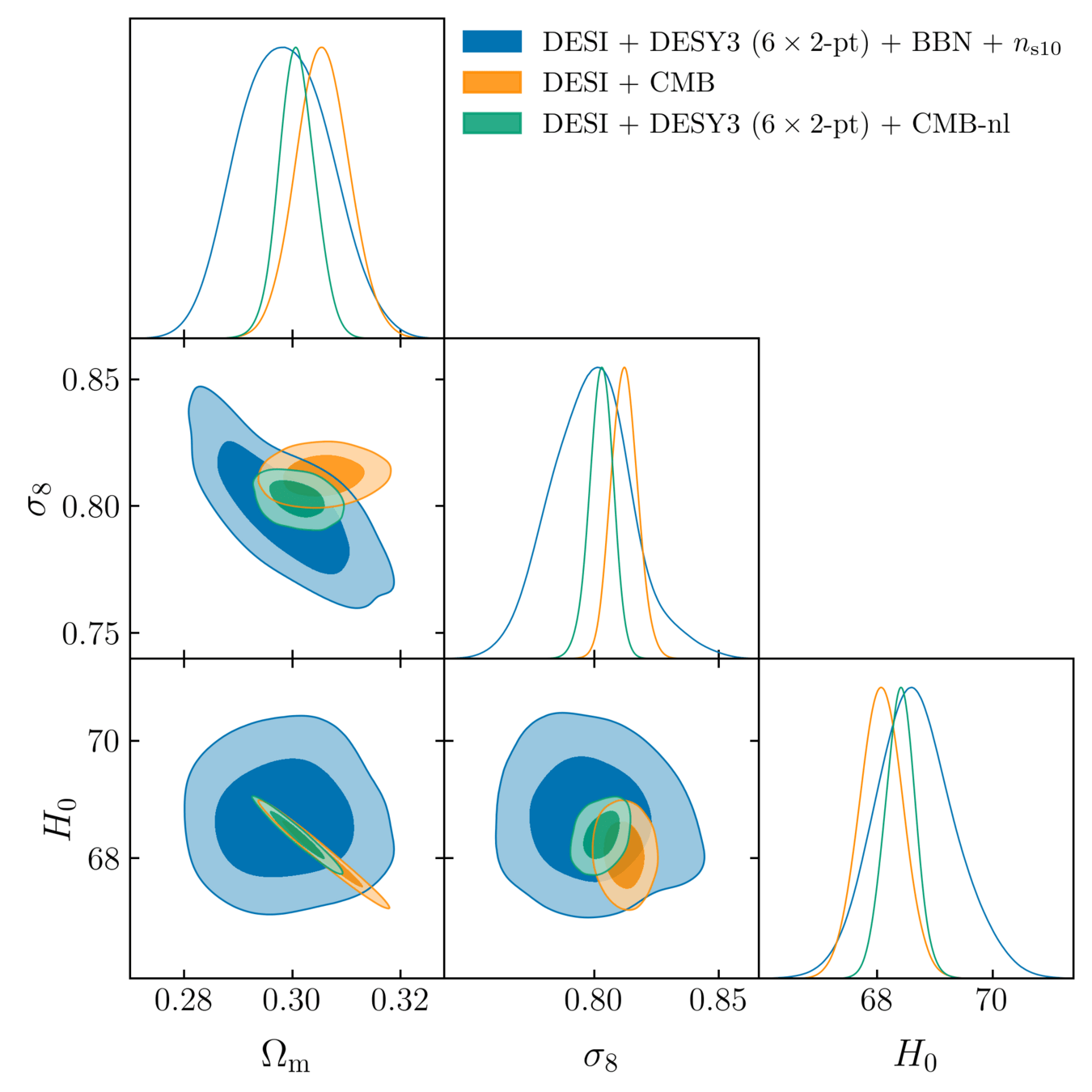
Combined constraints
*DES and SPT collaborations 2022
6x2pt = galaxy-galaxy, galaxy-shear, shear-shear, galaxy-CMB lensing, shear-CMB lensing, CMB lensing-CMB lensing
- Adding DESI to DESY3 6x2pt improves \(\sigma_8\) and \(\Omega_\mathrm{m}\) precision by \(\times 2\) (\(S_8\) by \(20\%\))

Combined constraints
- Adding DESI to DESY3 6x2pt improves \(\sigma_8\) and \(\Omega_\mathrm{m}\) precision by \(\times 2\) (\(S_8\) by \(20\%\))
- Adding DESI to CMB improves \(\Omega_\mathrm{m}\), \(H_{0}\) and \(S_{8}\) precision by \(30\%\)

Combined constraints
- Adding DESI to DESY3 6x2pt improves \(\sigma_8\) and \(\Omega_\mathrm{m}\) precision by \(\times 2\) (\(S_8\) by \(20\%\))
- Adding DESI to CMB improves \(\Omega_\mathrm{m}\), \(H_{0}\) and \(S_{8}\) precision by \(30\%\)

Dynamical Dark Energy - \((\Omega_\mathrm{m}, \sigma_{8})\)
(\(\Omega_\mathrm{m}, \sigma_{8})\) constraints remain stable when opening up to \(w_0w_a\text{CDM}\)


Dynamical Dark Energy - \((\Omega_\mathrm{m}, \sigma_{8})\)
(\(\Omega_\mathrm{m}, \sigma_{8})\) constraints remain stable when opening up to \(w_0w_a\text{CDM}\)
SN (uncalibrated):
- Pantheon+ Brout, Scolnic, Popovic et al., 2022
- Union3 Rubin, Aldering, Betoule et al. 2023
- DES-SN5YR DES Collaboration et al. 2024

Other datasets

- SDSS (for comparisons only): eBOSS Collaboration, 2020
- Primary CMB: Planck Collaboration, 2018
- CMB lensing: Planck PR4 + ACT DR6 lensing ACT Collaboration, 2023, Carron, Mirmelstein, Lewis, 2022
- BBN: Schöneberg 2024
- SN: Pantheon+ Brout, Scolnic, Popovic et al. 2022, Union3 Rubin, Aldering, Betoule et al. 2023, DES-SN5YR DES Collaboration
- DESY3 3x2pt DES collaboration 2021, 6x2pt DES and SPT collaborations 2022

DESI + CMB measurements favor a flat Universe

Spatial curvature
Dark Energy Equation of State


Constant EoS parameter \(w = p / \rho\)
Dark Energy Equation of State


Constant EoS parameter \(w = p / \rho\)
Dark Energy Equation of State


SNe (uncalibrated):
- Pantheon+ Brout, Scolnic, Popovic et al., 2022
Constant EoS parameter \(w = p / \rho\)
Dark Energy Equation of State


SNe (uncalibrated):
- Pantheon+ Brout, Scolnic, Popovic et al., 2022
- Union3 Rubin, Aldering, Betoule et al. 2023
Constant EoS parameter \(w = p / \rho\)
Dark Energy Equation of State

SNe (uncalibrated):
- Pantheon+ Brout, Scolnic, Popovic et al., 2022
- Union3 Rubin, Aldering, Betoule et al. 2023
- DES-SN5YR DES Collaboration et al. 2024

Constant EoS parameter \(w = p / \rho\)
Dark Energy Equation of State

Assuming a constant EoS, DESI BAO fully compatible with a cosmological constant...

Constant EoS parameter \(w = p / \rho\)

Dark Energy Equation of State

Varying EoS

Dark Energy Equation of State

Varying EoS

Dark Energy Equation of State

Varying EoS

Dark Energy Equation of State

Varying EoS

Dark Energy Equation of State

Varying EoS

Dark Energy Equation of State
Combining all DESI + CMB + SN


Dark Energy Equation of State
Combining all DESI + CMB + SN


Dark Energy Equation of State
Combining all DESI + CMB + SN


Dark Energy Equation of State
Combining all DESI + CMB + SN
\(w_{0} > -1, w_{a} < 0\) favored, level varying on the SN dataset

Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses

Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by BAO, especially through \(H_{0}\)
Low preferred value of \(H_{0}\) yields
\(\sum m_\nu < 0.072 \, \mathrm{eV} \; (95\%, \color{green}{\text{DESI + CMB})}\)

Limit relaxed for extensions to \(\Lambda\mathrm{CDM}\)
\(\sum m_\nu < 0.195 \, \mathrm{eV}\) for \(w_0w_a\mathrm{CDM}\)
Neutrino mass hierarchies


With \(> 0.059 \, \mathrm{eV}\) prior (NH)
Neutrino mass hierarchies


With \(> 0.059 \, \mathrm{eV}\) prior (NH)
With \(> 0.1 \, \mathrm{eV}\) prior (IH)
Neutrino mass hierarchies


With \(> 0.059 \, \mathrm{eV}\) prior (NH)
With \(> 0.1 \, \mathrm{eV}\) prior (IH)
Current constraints do not strongly favor normal over inverted hierarchy (\(\simeq 2 \sigma\))
Summary

DESI already has the most precise BAO measurements ever
Summary

DESI already has the most precise BAO measurements ever
DESI BAO is consistent (at the \(\sim 1.9\sigma\) level) with CMB in flat ΛCDM
Summary

DESI already has the most precise BAO measurements ever
DESI BAO is consistent (at the \(\sim 1.9\sigma\) level) with CMB in flat ΛCDM
In flat ΛCDM, DESI prefers "small \(\Omega_\mathrm{m}\), large \(H_0\) (though \(3.7\sigma\) away from SH0ES), small \(\sum m_\nu\)"
Summary

DESI already has the most precise BAO measurements ever
DESI BAO is consistent (at the \(\sim 1.9\sigma\) level) with CMB in flat ΛCDM
In flat ΛCDM, DESI prefers "small \(\Omega_\mathrm{m}\), large \(H_0\) (though \(3.7\sigma\) away from SH0ES), small \(\sum m_\nu\)"
Some hint of time-varying Dark Energy equation of state especially when combined with supernovae measurements

DESI data release 1 (DR1)


5.7 million unique redshifts at z < 2.1 and > 420,000 Ly\(\alpha\) QSO at z > 2.1

DESI Y5 forecasts

Survey Validation (arXiv:2306.06307)
BAO and RSD constraints at the end of the survey (\( \Delta z = 0.1 \))
Ly\(\alpha\)

DESI Y5 forecasts

Survey Validation (arXiv:2306.06307)
BAO and RSD constraints at the end of the survey (\( \Delta z = 0.1 \))
Ly\(\alpha\)

(w/ Planck)

Power spectra




Power spectra



BAO wiggles

Density field reconstruction


DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function:
- cosmo signal
linear bias + RSD
hydro-sim
BAO

DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function:
- cosmo signal
- high-column density
- metal absorbers
SiII

DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function:
- cosmo signal
- high-column density
- metal absorbers
- correlated noise (sky subtraction)

DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function:
- cosmo signal
- high-column density
- metal absorbers
- correlated noise (sky subtraction)

DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function

physical model fit
+ broadband polynomial

broadband: \(< 0.1\sigma\)

DESI DR1 Ly\(\alpha\) BAO analysis
- Biggest ever Ly\(\alpha\) dataset (\(N_\mathrm{tracer}\))
- First blind analysis to mitigate observer / confirmation biases (correlation function-level blinding)
- Modelling of the correlation function
- Covariance matrix

Correlation matrix
smoothed jackknife, validated with mocks

Tests of systematic errors




tests with same dataset (not red): shifts \(< \sigma_\mathrm{stat}/3\)
tests with varying datasets (red): shifts consistent with stat.

Tests of systematic errors


DESI DR1 BAO analysis
- Biggest ever spectroscopic BAO dataset (\(N_\mathrm{tracer}\) and \(V\))
- Blind analysis to mitigate observer / confirmation biases (catalog-level blinding)
fiducial cosmology
blinded cosmology (\(\Omega_\mathrm{m}, w_0, w_a\))
(random & unknown)
+ RSD blinding: change reconstructed peculiar velocities
+ \(f_\mathrm{NL}\) blinding: add clustering-dependent signal on large scales with weights

DESI DR1 BAO analysis
- Biggest ever spectroscopic BAO dataset (\(N_\mathrm{tracer}\) and \(V\))
- Blind analysis to mitigate observer / confirmation biases (catalog-level blinding)
- Theory developments in BAO fitting code

Chen, Howlett et al. 2024

Some fits: Fourier space


\(\sum m_\nu\)

credit: Christophe Yèche
\(w(z)\)


DESI - SDSS consistency (\(\Omega_\mathrm{m}\))



Perfectly consistent!
Using these 2 points alone moves \(\Omega_\mathrm{m}\) by \(< 2 \sigma\)
Are SN \(\Omega_\mathrm{m}\) consistent?


Not so much in flat \(\Lambda\mathrm{CDM}\)...
(so we do not combine them in this model!)
Are SN \(\Omega_\mathrm{m}\) consistent?


Consistent in \(w_0w_a\mathrm{CDM}\)!
plik vs PR4 Planck likelihoods



Appendix B
\(w_0 - w_a\) with \(\sum m_\nu\) free



\(w_0 - w_a\) with \(\Omega_\mathrm{K}\)
Preference for \(w_{0} > -1, w_{a} < 0\) persists when curvature is left free

DE constraints driven by low-\(z\) ?

Not that much!
DESI + SDSS swaps DESI measurements with SDSS for \(z < 0.6\)
\(- 0.4 \sigma\) compared to DESI only

\(w(z)\)



Dark energy equation of state:
\(P = w \rho\)
- \(w\) = constant

BAO measurements: dark energy


BAO measurements: dark energy
Full tables

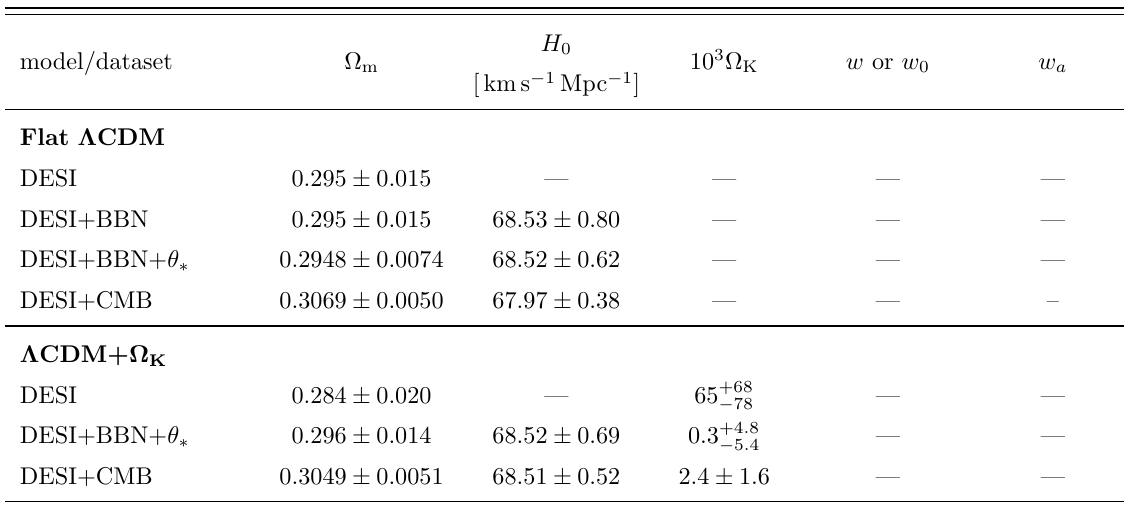
Full tables

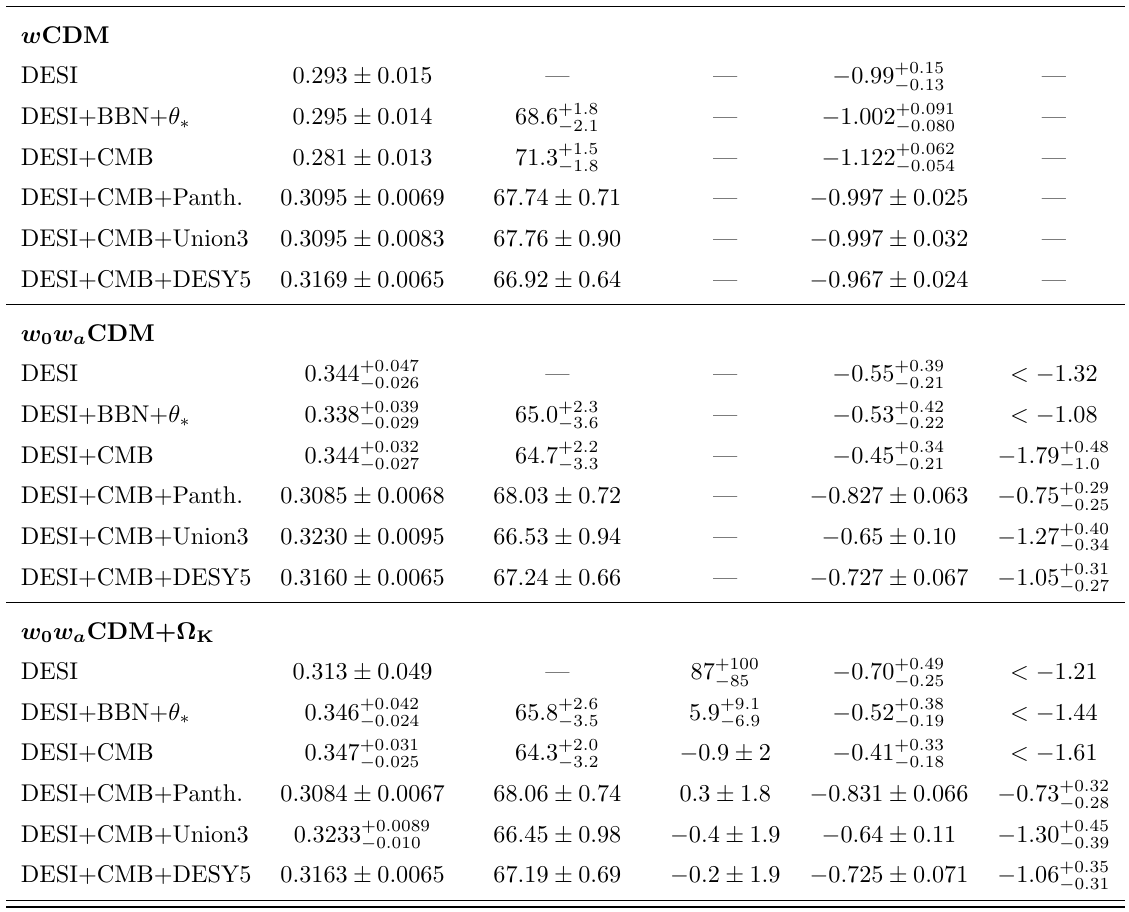
Full tables

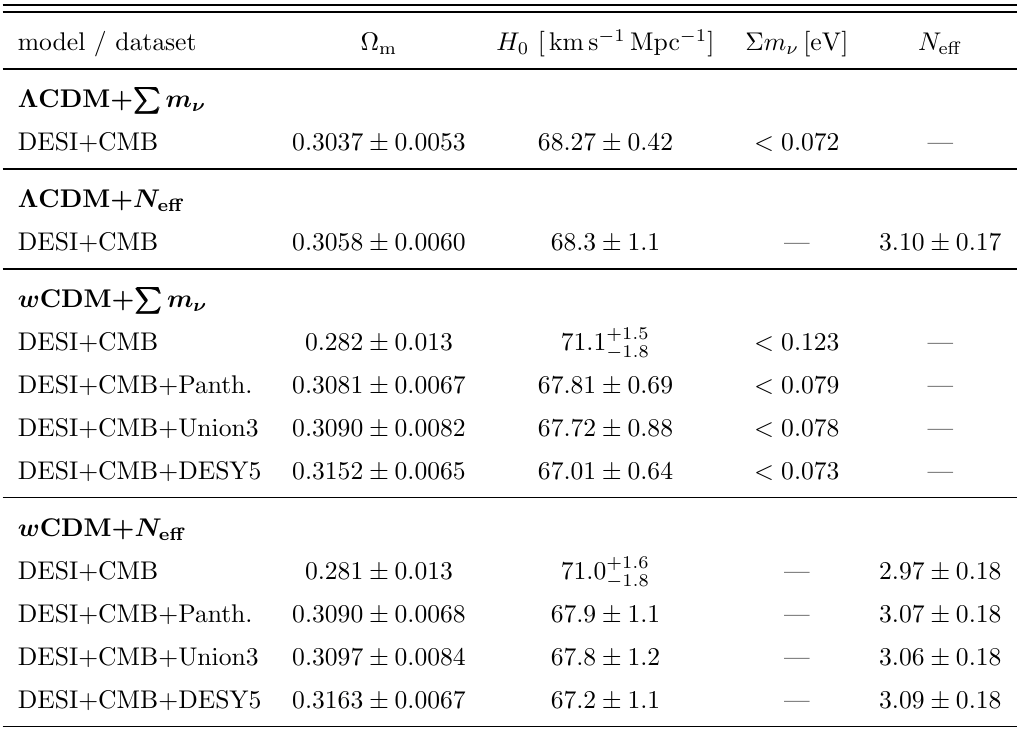
Full tables