


Corentin Cadiou
RUM | 18 April 2023

On the causal origin of dark matter halo and galaxy properties:
replacing galaxies in their cosmological context

Angular momentum: bridging galaxies to cosmology?
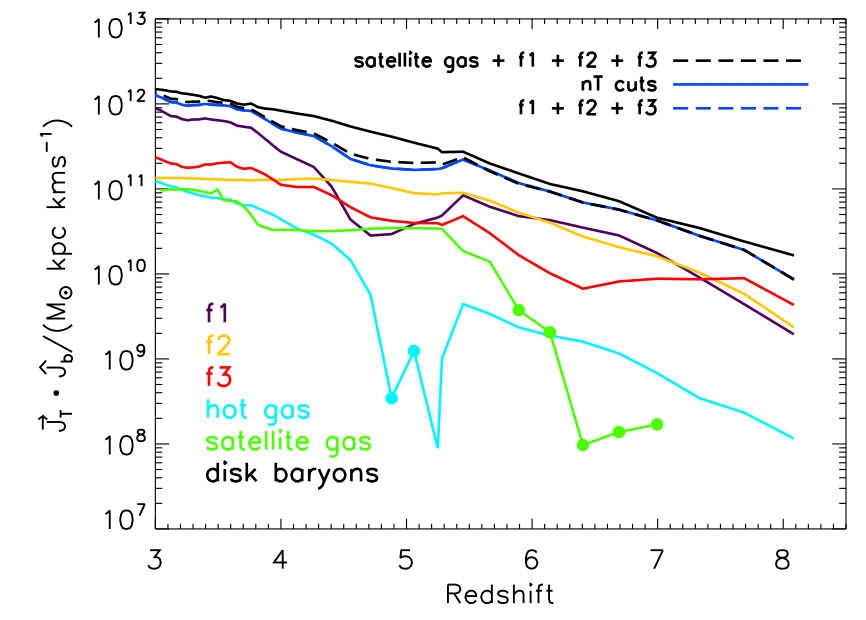
Tillson+15
[AM: Dekel & Birboim 06; Stewart+11; Kim+11; Pichon+11; Codis+12; Danovich+12,15; Stewart+13; Codis+15; Prieto+15; Tillson+15; Stewart+17, Cadiou+21,…]

Dekel&Birnboim 06
High-z:
most of mass + AM flow along filaments
Lower-zs:
intrinsic alignment problem

Tempel+13
Corentin Cadiou
Angular momentum: bridging galaxies to cosmology?

Tillson+15

Dekel&Birnboim 06
High-z:
most of mass + AM flow along filaments
Lower-zs:
intrinsic alignment problem

Tempel+13
How do we study these effects?
Large volumes
sample \(p(M_\star, M_\mathrm{DM},\mathbf{J}, d_\mathrm{fil}, \dots)\)
This talk
sample \(p(\mathbf{J}|M_\star, M_\mathrm{DM}, d_\mathrm{fil}, \dots)\)
Corentin Cadiou
Angular momentum: where are we?
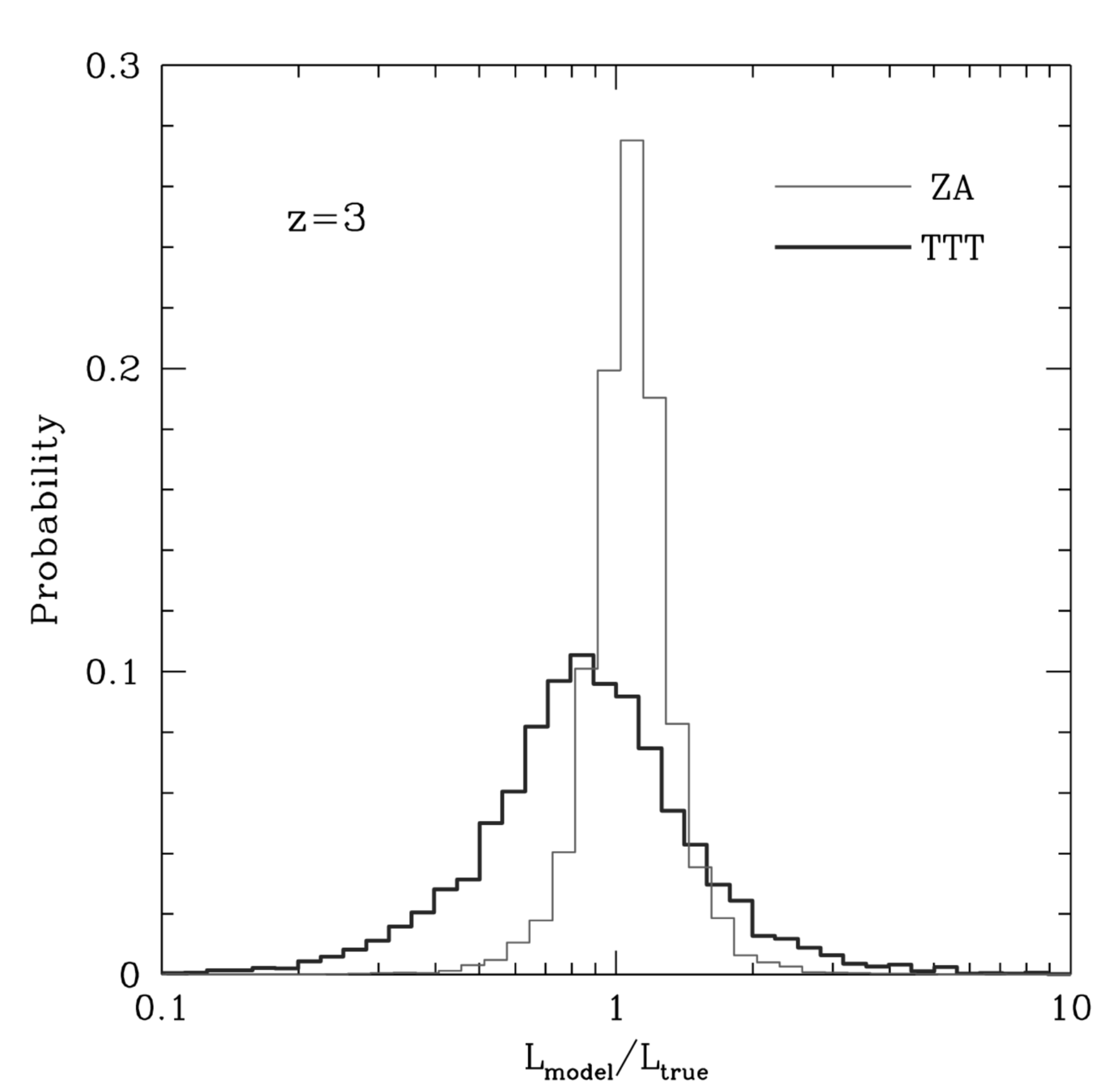
Porciani+02
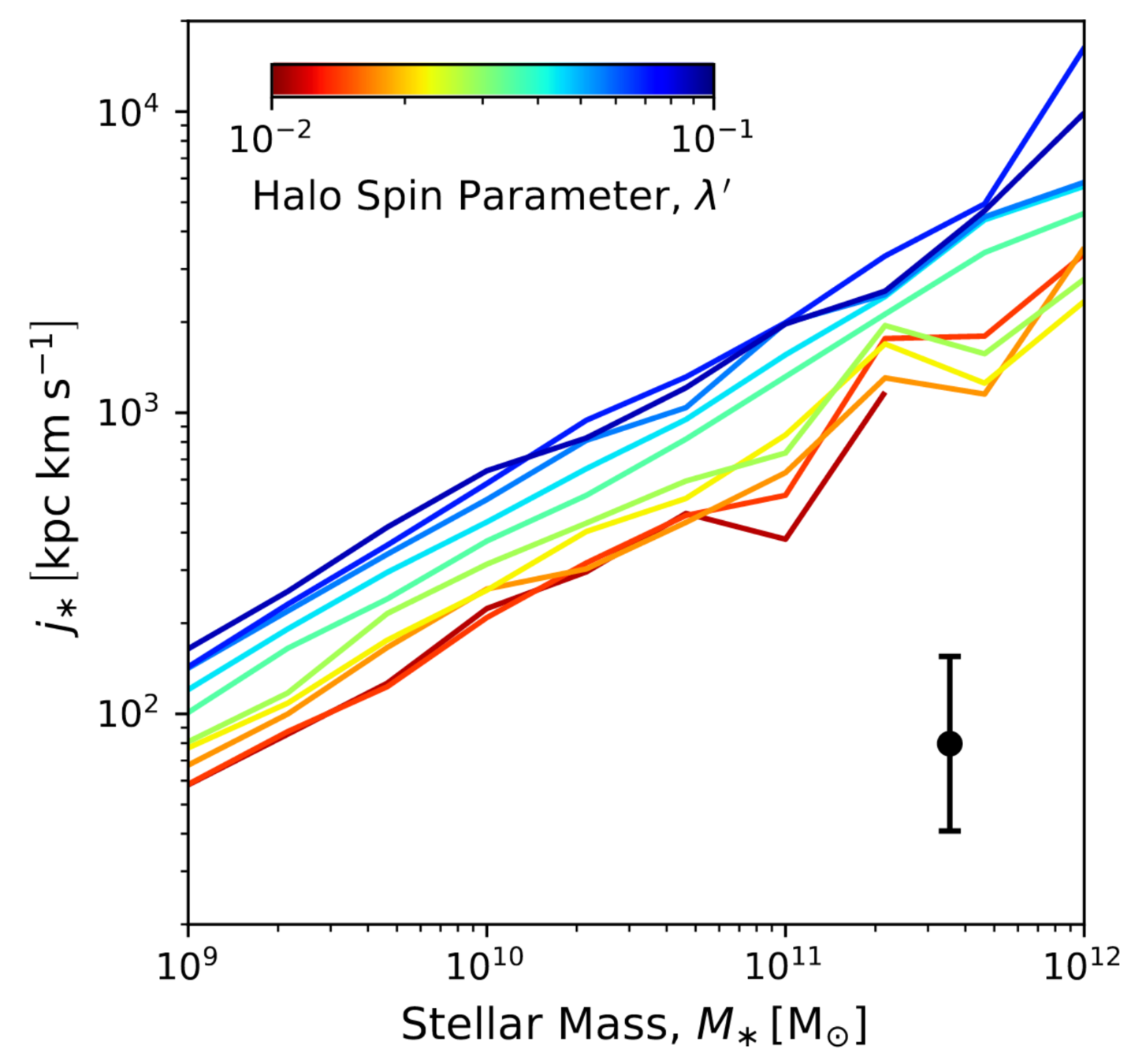
Rodriguez-Gomez+22
Predictions for \(j_\mathrm{DM}\) remain qualitative
\(j_\mathrm{DM}-j_\mathrm{\star}\)
weak correlation
(statistically strong)
- Is \(j_\mathrm{DM}\) chaotic or our theory poor?
Can we do better than statistical correlations? - Do \(j_\mathrm{gal}\) retain memory of their environment?
Can we spin a simulated galaxy up?


Corentin Cadiou
The effects of environment on halo properties
Kraljic+18 [see also Laigle15, Song+21,…]
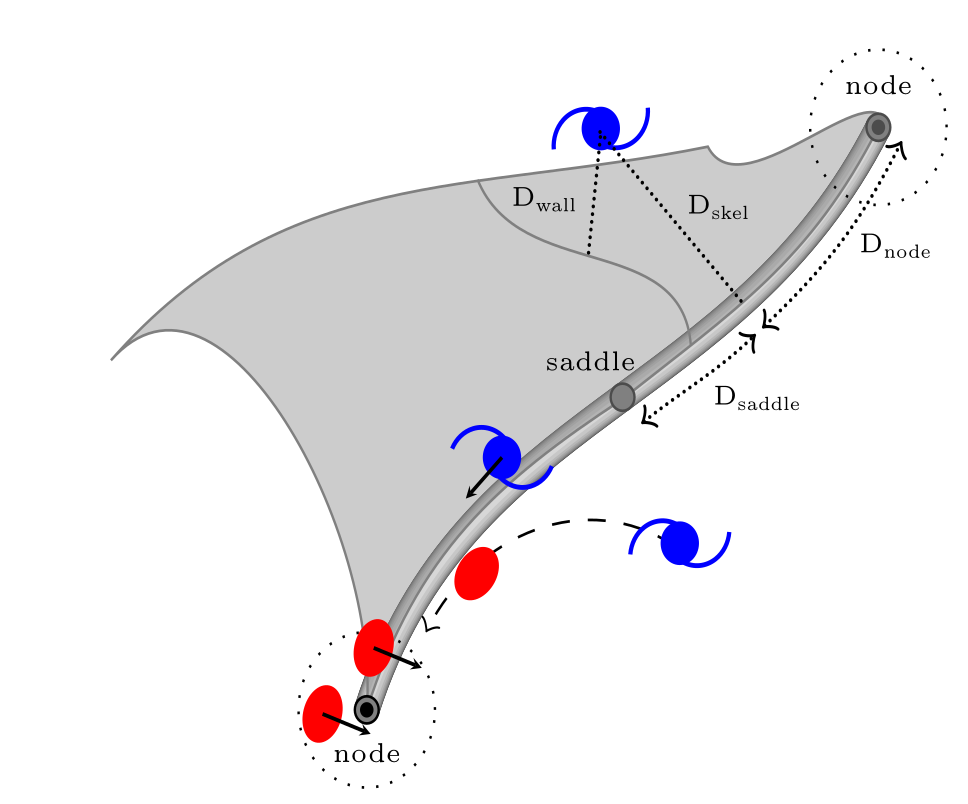


- \( M_\mathrm{DM}(\text{node}) \) > \(M_\mathrm{DM}(\text{fil}) \) >\(M_\mathrm{DM}(\text{void})\), higher clustering
- spins align with cosmic web ⇒ issue for weak lensing
- \(v/\sigma(\mathrm{fil})>v/\sigma(\mathrm{void})\) ⇒ bias in galaxy formation
- ….
The effects of environment on halo properties




Isotropic effects
Kaiser bias, cluster vs. groups, ...
From theory: \(M\propto \int\mathrm{d}^3R\rho\)
Mass regulated
An-isotropic effects
Intrinsic alignment, formation of disks?
From theory: \(J \propto \int\mathrm{d}^3R \nabla \phi\)
Angular momentum regulated?
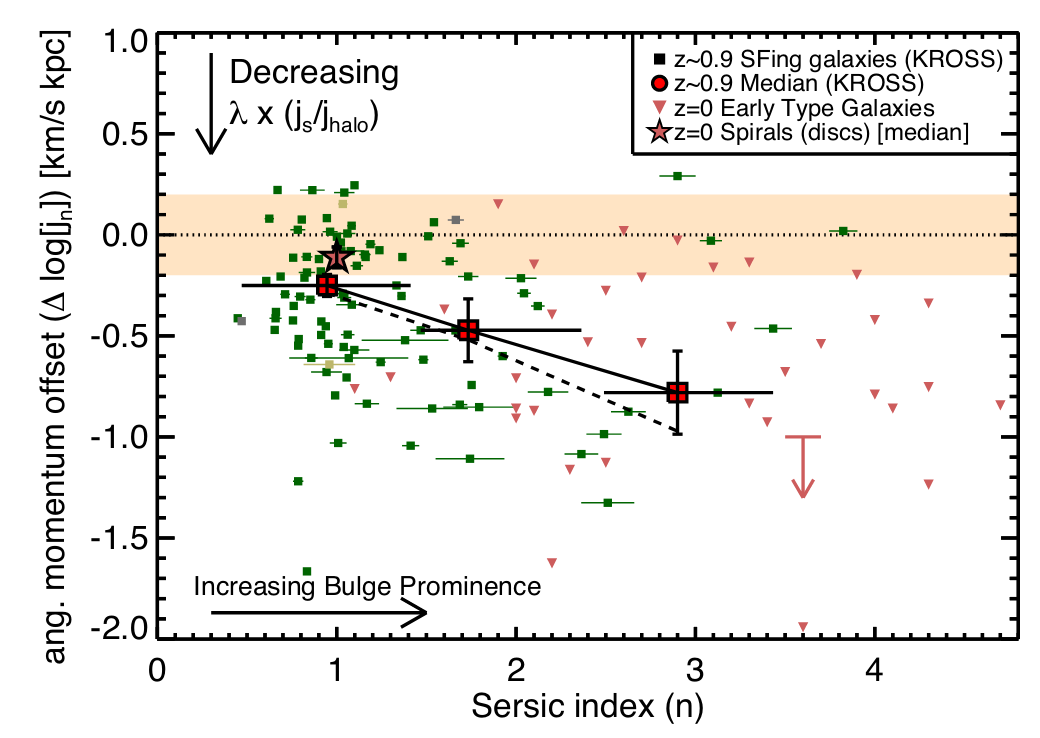
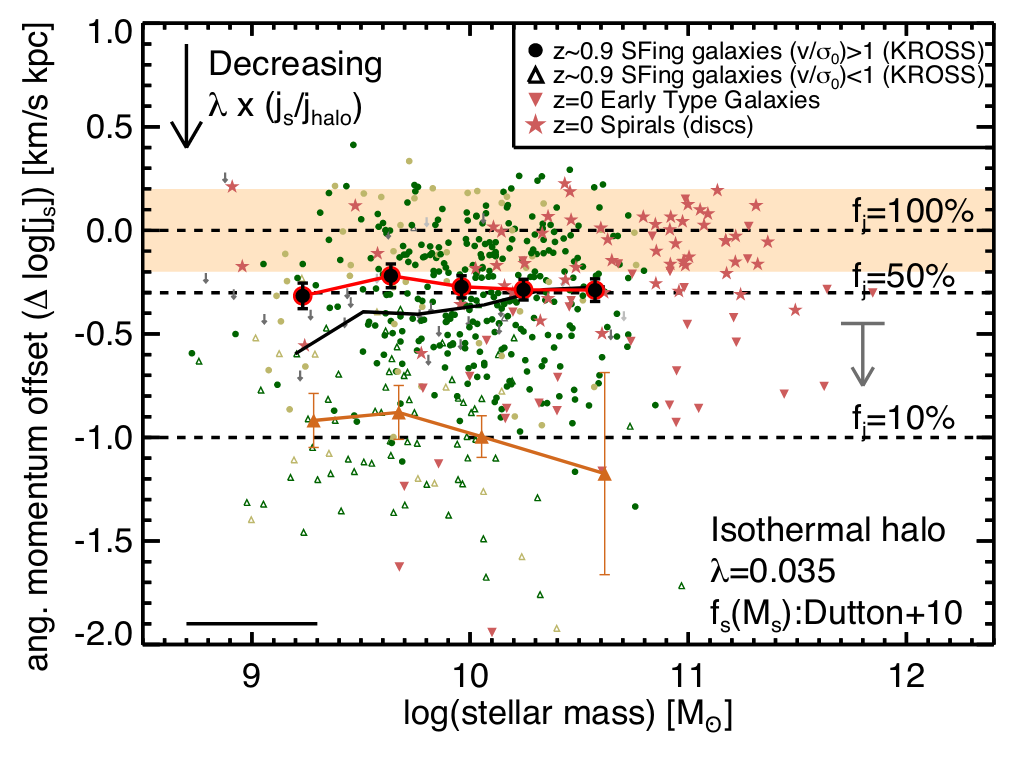
Harrison+17 (KMOS, \(z=1\))





Spiral galaxies \(\leftrightarrow\) high \(J_\star\)
What's the arrow of causality?
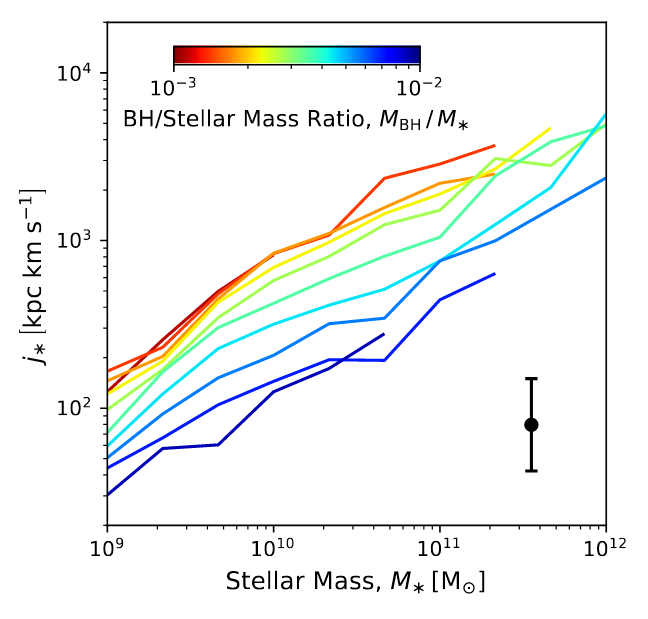
Rodriguez-Gomez+22 (TNG)


Is \(j_\mathrm{DM}\) chaotic or our theory poor?
First controlled experiment of testing tidal torque theory for individual halos
CC+21a, arXiv: 2012.02201
Corentin Cadiou
Predicting angular momentum



\(z=0\)
\( z = 100\)
Predicting angular momentum



\(z=0\)
\( z = 100\)
\[\mathbf{L}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
Position w.r.t. center
Velocity
[White 84]

Note: vanishes at 1st order in a sphere
\[ \int_\Gamma \mathrm{d}^3{q}(\mathbf{q}-\mathbf{\bar{q}}) \times\nabla\phi = \int_{\partial\Gamma}\phi(q)(\mathbf{q}-\mathbf{\bar{q}})\times\mathrm{d}\mathbf{S}\]
Note: the following is a (poor) approximation:
\[ \mathbf{L} \propto \epsilon_{ijk} T_{jl}I_{lk},\quad\text{with \textbf{T} the tidal tensor and \textbf{I} the inertia tensor}\]
Predicting angular momentum



\(z=0\)
\( z = 100\)
[Genetic modifications: Roth+16, see also Rey&Pontzen 18, Stopyra+20]



Corentin Cadiou
Predicting angular momentum










Time
Sampling \(p(\mathbf{J}|M_\mathrm{DM}, d_\mathrm{fil}, \dots)\)
Predicting angular momentum





Time
Time
“Poorer” predictions
“Good” predictions









Predicting angular momentum







✅ AM of fixed DM regions responds ~linearly (so is not chaotic!)
Improve theory?
Good model of Lagrangian patch boundaries (cf. M. Musso future talk)
or
Find more robust definition of AM?
Corentin Cadiou
Do \(j_\mathrm{gal}\) retain memory
of the environment?
First controlled experiment of angular momentum accretion on individual galaxies
CC+22, arXiv: 2206.11913
Main idea: stars are deeper in potential well so less sensitive to what happens at large scales
⇒ stellar Lagrangian patch should be more stable to perturbations
Corentin Cadiou



Same \(M_\mathrm{DM},\rho_\mathrm{5 Mpc},M_\star\)
but
different \(j_\star\)?
1/ Perturb initial conditions
here: add tides
2/ Run cosmological simulation
Angular momentum genetic modifications
\[\mathbf{j}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
Position w.r.t. center
Velocity



Same \(M_\mathrm{DM},\rho_\mathrm{5 Mpc},M_\star\)
but
different \(j_\star\)?
1/ Perturb initial conditions
here: add tides
2/ Run cosmological simulation
Angular momentum genetic modifications
\[\mathbf{j}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
Position w.r.t. center
Velocity
Corentin Cadiou
Baryon angular momentum
Full hydro simulations
(10Mh @ DiRAC):
- Resolve disk height
\(\Delta x = 35\ \mathrm{kpc}\) - \(z \geq 2\), \(M_\mathrm{200c} = 10^{12}\ \mathrm{M}_\odot\)
- SF + AGN & SN feedback
-
Tracer particles
CC+19 - 3 galaxies, 5× scenario each
\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)
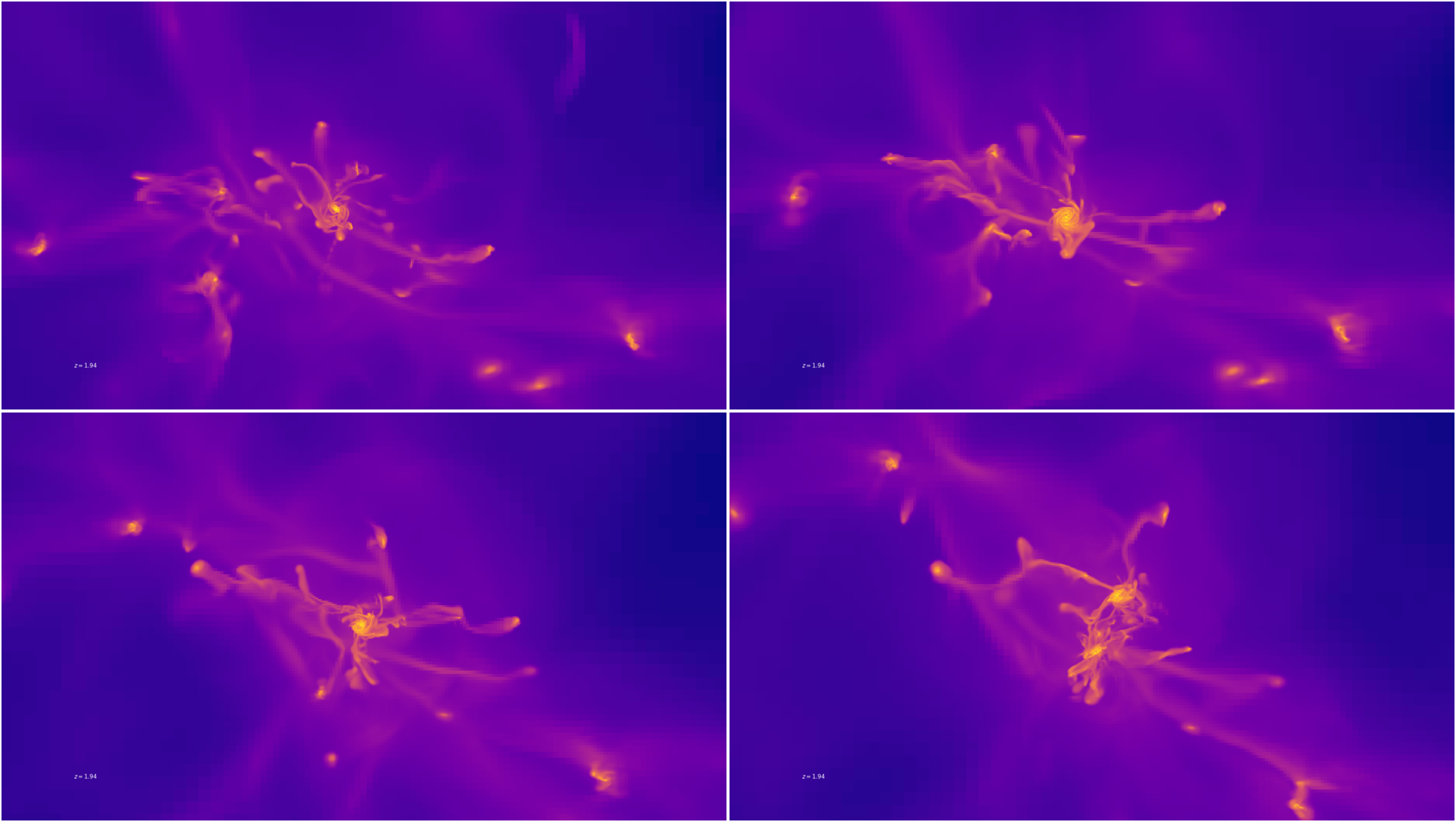
Sampling \(p(\mathbf{J}|M_\star, M_\mathrm{DM}, d_\mathrm{fil}, \dots)\)
Baryon angular momentum
Full hydro simulations
(10Mh @ DiRAC):
- Resolve disk height
\(\Delta x = 35\ \mathrm{kpc}\) - \(z \geq 2\), \(M_\mathrm{200c} = 10^{12}\ \mathrm{M}_\odot\)
- SF + AGN & SN feedback
-
Tracer particles
CC+19 - 3 galaxies, 5× scenario each
\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)

Sampling \(p(\mathbf{J}|M_\star, M_\mathrm{DM}, d_\mathrm{fil}, \dots)\)
… by delaying/hastening time of last major merger
Corentin Cadiou


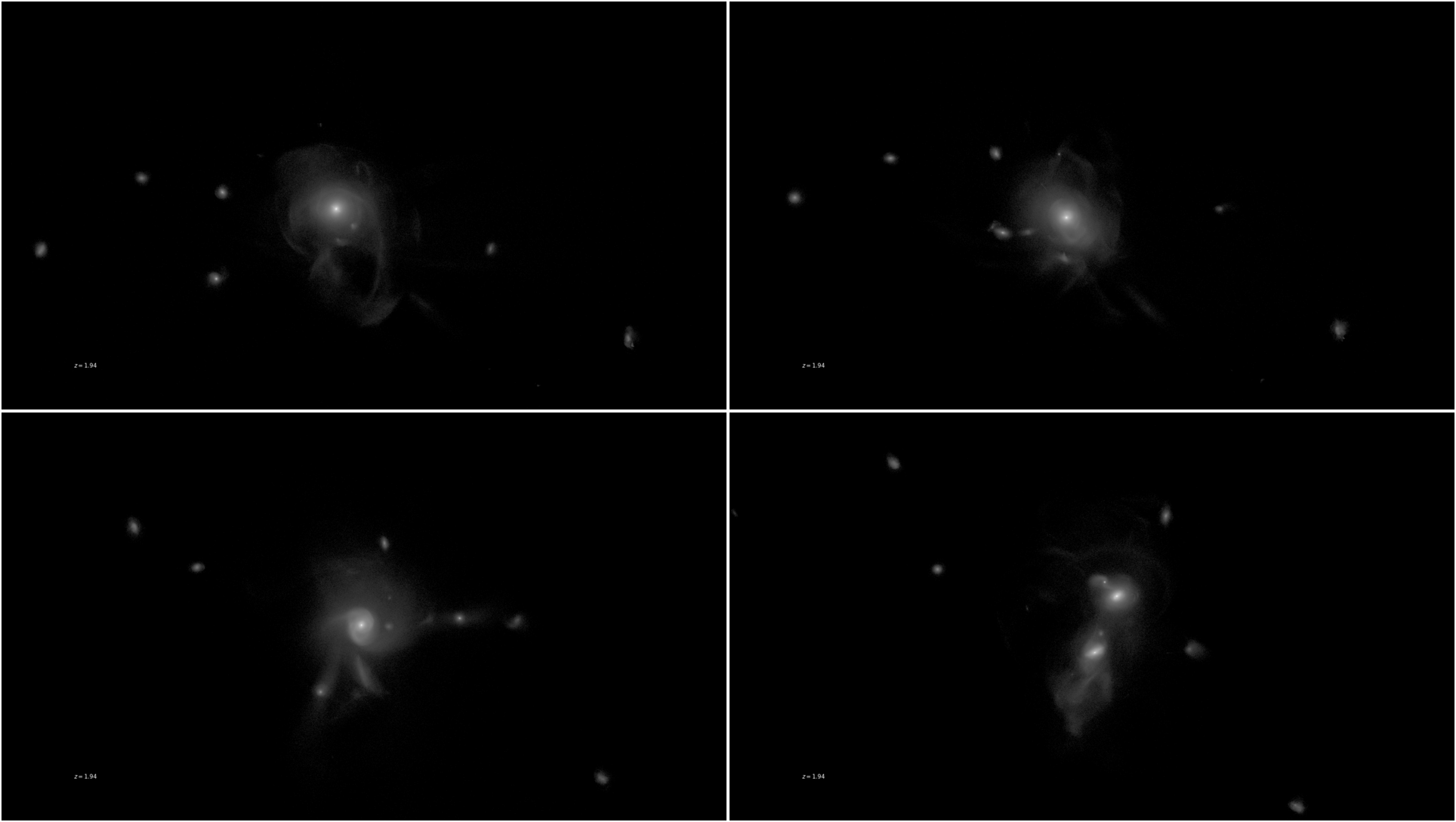
INPUT \(z=\infty\)
OUTPUT
\(z=2\)

\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)
\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)



✅ Stellar AM driven by (past) tides with the cosmic web (which can be predicted)
✅ Useful to make sense out of e.g. JWST data
Corentin Cadiou
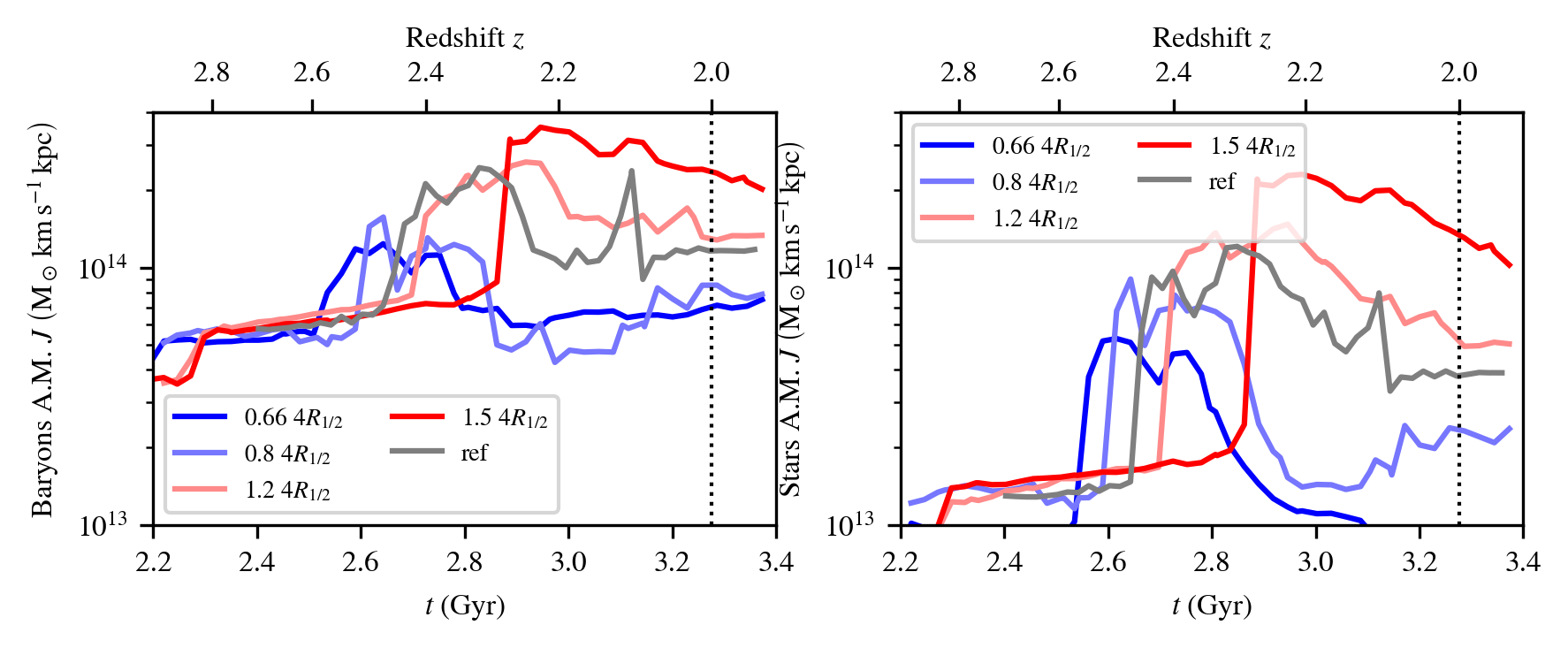


\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)
\( j_0 \times 0.66\)
\( j_0 \times 0.8\)
\( j_0 \times 1.2\)
\( j_0 \times 1.5\)



Corentin Cadiou

✅ Changes in baryon spAM \(\propto\) changes in Halo spAM
\({\color{#808080}{\lambda_\mathrm{DM}}} \underset{f_j}{\Longrightarrow} \lambda_\mathrm{\color{#0000ff}{bar}\color{#daa520}{yon}} \underset{\text{SF+fb}}{\Longrightarrow} \color{#daa520}\lambda_\star\)
❗Per-galaxy fluctuation of \(\lambda_\star/\lambda_\mathrm{DM} \)
\(\Rightarrow\) cannot be captured with HOD modelling
Gas + stars spAM
Stars spAM
Halo spAM
Halo spAM
Corentin Cadiou
Where is the
CW — galaxy boundary?
Spoiler: it's the CGM
CC+Pichon+Dubois, 21, arXiv: 2110.05384
Kocjan, CC in prep.
By no means complete review!
Corentin Cadiou
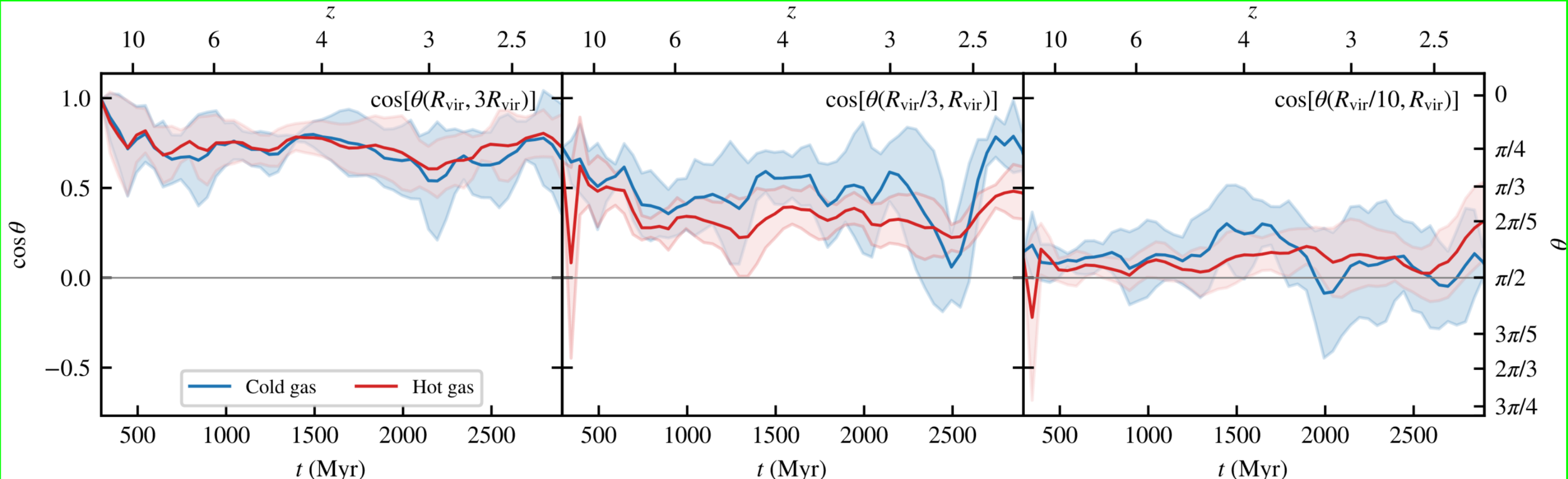
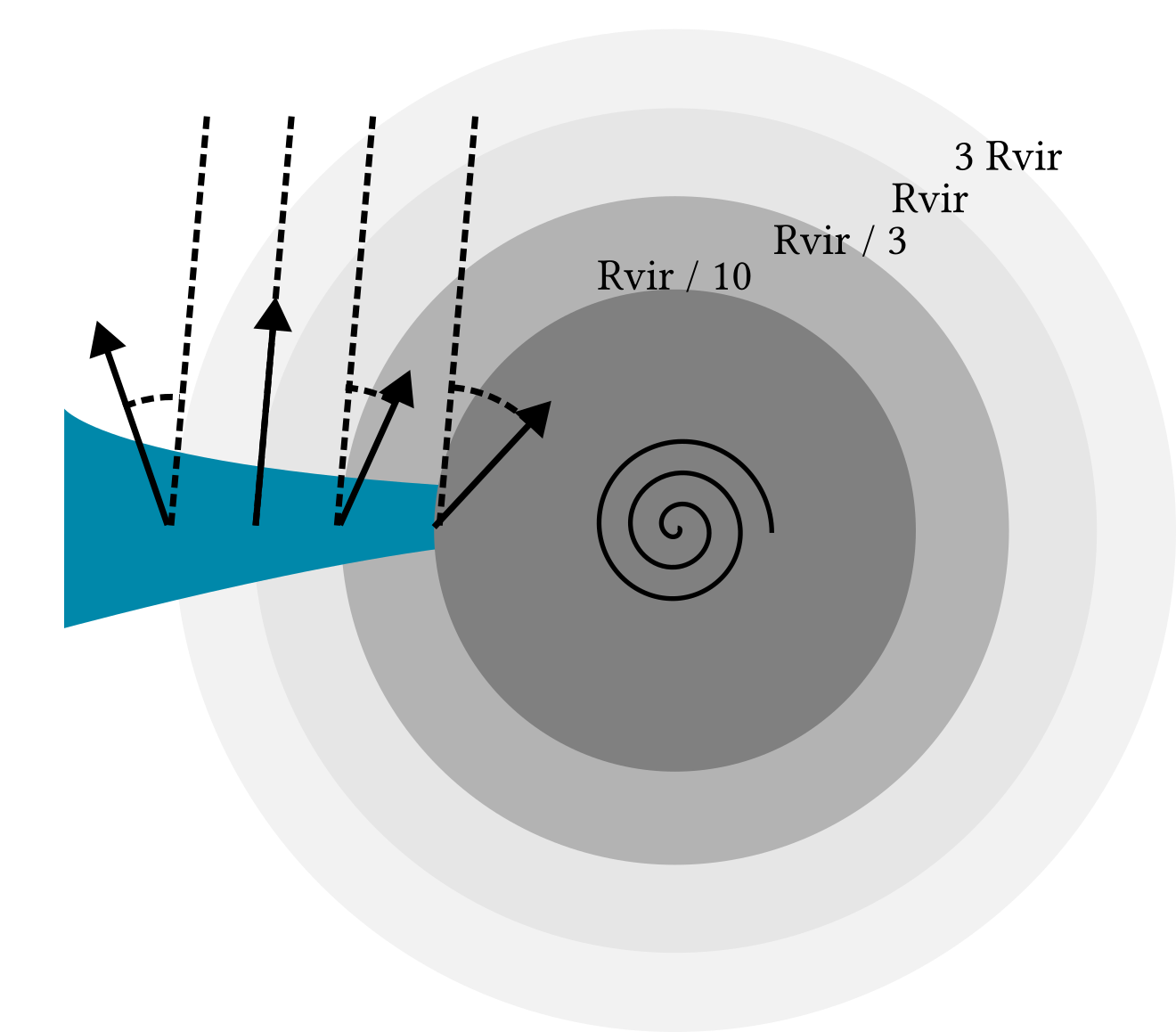
[See also Danovich+15, Prieto+17]


✅ Most of re-alignment happens in the CGM \(0.1\leq \displaystyle\frac{r}{R_\mathrm{vir}}\leq 0.3\)
The longer gas remains in CGM, the more it realigns with disk
[See also Danovich+15, Prieto+17]

Ongoing work by Z. Kocjan

[Kocjan, CC+ in prep]

Filamentary accretion ~ Cold flow = \(T \leq 10^5\mathrm{K}\) for \(0.3R_\mathrm{vir} < r < 2R_\mathrm{vir}\)


Filamentary accretion ~ Cold flow = \(T \leq 10^5\mathrm{K}\) for \(0.3R_\mathrm{vir} < r < 2R_\mathrm{vir}\)
Not necessarily fast-track to star formation ⇒ lose connection to CW?
[Kocjan, CC+ in prep]
\(M_\mathrm{DM}(z=2)\approx 10^{11}-10^{12} \mathrm{M_\odot}\)
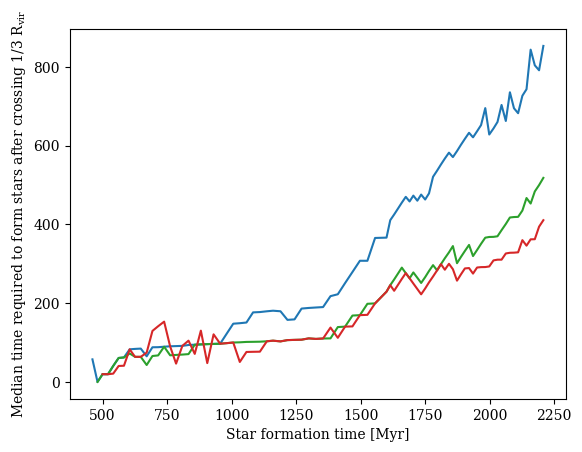

Ongoing work by Z. Kocjan
Conclusion & outlook
Corentin Cadiou
Conclusion & outlook

-
\(j_\mathrm{DM}\) responds linearly to perturbations
Good accuracy (few ~10%) achievable for individual halos in principle iff correct growth rate+boundary
-
\(j_\mathrm{gal}\) retain memory of the cosmic web
Galaxies may be less stochastic than expected
Intrinsic alignment to be expected
Galactic spin & DM spins are (partially) independent at level of individual galaxies -
Anisotropic environment matters for second props.
⇒ ~15% change in mass
⇒ 50% of population scatter for concentration
Very promising for intrinsic alignment studies!



Corentin Cadiou
What's the effect of anisotropic env DM/gal formation?
Study same object, different environment.
CC+21, arXiv: 2107.03407
Cosmic web drives AM acquisition... what scales? what's affected?
Corentin Cadiou



Corentin Cadiou


What if the galaxy had formed here instead?


Corentin Cadiou


What if the galaxy had formed here instead?


or here?
Corentin Cadiou
The “splicing” technique

- Generate ICs
- Integrate (\(N\)-nody)
- Select region of interest
- Trace back to ICs
- “Splice”
- Integrate again
\(t\)

Splicing: equivalent of constraining field at all points in spliced region
Corentin Cadiou
The causal origin of DM halo concentration


\(M^{(1)}_{200\mathrm{c}}, c^{(1)}_\mathrm{NFW}, \dots\)


\(M^{(2)}_{200\mathrm{c}}, c^{(2)}_\mathrm{NFW}, \dots\)


\(M^{(\dots)}_{200\mathrm{c}}, c^{(\dots)}_\mathrm{NFW}, \dots\)


\(M^{(10)}_{200\mathrm{c}}, c^{(10)}_\mathrm{NFW}, \dots\)
Same halo in 10× different environments
Repeat experiment for 7 halos (70 realisations in total)
Corentin Cadiou
Same halo in 10× different environments
Repeat experiment for 7 halos (70 realisations in total)


\(M^{(1)}_{200\mathrm{c}}, c^{(1)}_\mathrm{NFW}, \dots\)


\(M^{(2)}_{200\mathrm{c}}, c^{(2)}_\mathrm{NFW}, \dots\)


\(M^{(\dots)}_{200\mathrm{c}}, c^{(\dots)}_\mathrm{NFW}, \dots\)


\(M^{(10)}_{200\mathrm{c}}, c^{(10)}_\mathrm{NFW}, \dots\)

The causal origin of DM halo concentration
Corentin Cadiou
Same halo in 10× different environments
Repeat experiment for 7 halos (70 realisations in total)


\(M^{(1)}_{200\mathrm{c}}, c^{(1)}_\mathrm{NFW}, \dots\)


\(M^{(2)}_{200\mathrm{c}}, c^{(2)}_\mathrm{NFW}, \dots\)


\(M^{(\dots)}_{200\mathrm{c}}, c^{(\dots)}_\mathrm{NFW}, \dots\)


\(M^{(10)}_{200\mathrm{c}}, c^{(10)}_\mathrm{NFW}, \dots\)


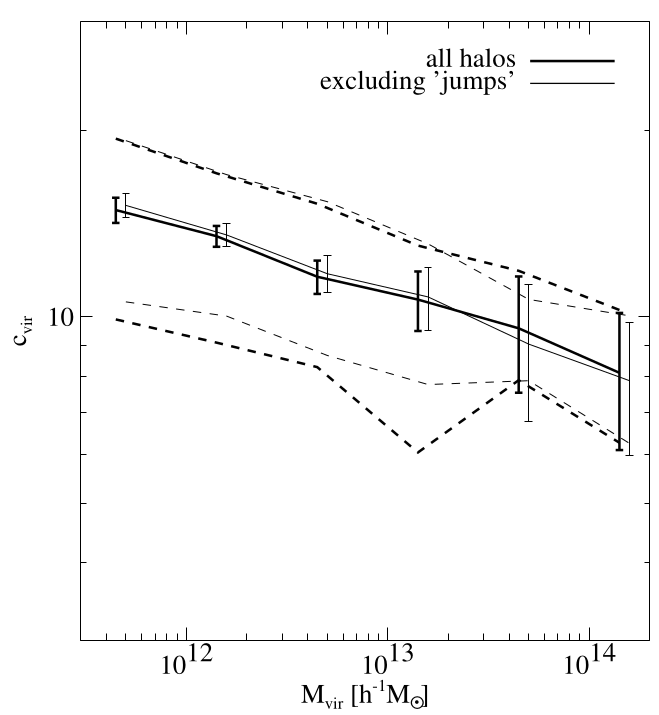
The causal origin of DM halo concentration
50% of population
Corentin Cadiou


Ex Uno Plures: direct measure of the impact of the cosmic web on individual objects to shed light on their population statistics



Corentin Cadiou
The Co-evolution of the CW and Galaxies across Cosmic Time

The causal origin of DM halo concentration


$$\rho_\mathrm{DM}(r) = \frac{\rho_0}{\frac{r}{R_\mathrm{vir}/c} \left(1 + \frac{r}{R_\mathrm{vir}/c}\right)^2}$$
Wechsler+02
Origin of scatter at fixed \(M_\mathrm{vir}\)?
Predicting angular momentum



- Angular momentum of individual regions can be predicted accurately.
- AM of halos ⇒ requires boundaries of patch


\[\mathbf{L}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
[On patch boundaries: see Lucie-Smith+18]
Splicing in 1D





Splicing in 1D


Most likely* field \(f\) with
- same value in spliced region (\(a\)),
- as close as possible outside (\(b\))



Mathematically \(f\) is solution of:
\( f= a\) in \(\Gamma\)
minimizes \(\mathcal{Q} = (b-f)^\dagger\mathbf{C}^{-1}(b-f) \) outside \(\Gamma\)
Can we control baryonic
angular momentum?
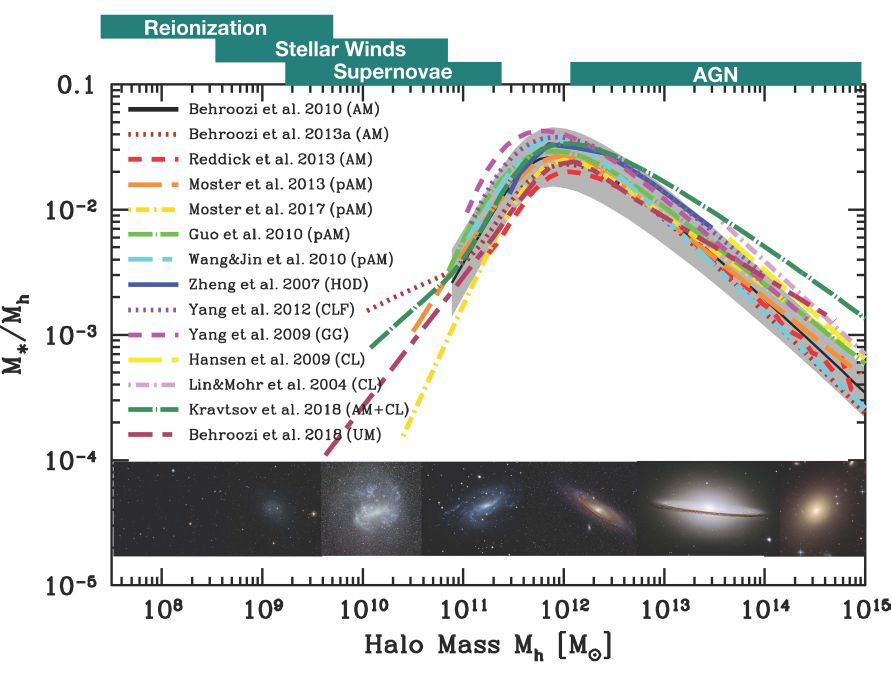
Wechsler & Tinker 18
\({\color{red}M_\star} / M_\mathrm{h} \ll \Omega_b / \Omega_m \)
⇒ baryons & DM stem from different regions


Baryons more strongly bound
⇒ less prone to being ejected



Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Temporary conclusions
-
angular momentum is predictable
-
boundary of halos in the ICs is a hard problem
⇒ limits practicality of predictions (for now)
-
baryons appear to be simpler!
⇒ good news for weak lensing predictions
⇒ key to understand morphology



Galaxy formation in cosmology: the role of the environment




Environmental effects:
- source of “pollution” in weak lensing surveys
⇒ intrinsic alignment
- extra parameters in semi-analytical models
⇒ galaxy-halo correlation



\( l_0 \times 1.2\)
\( l_0 \times 1.5\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)



\( l_0 \times 1.2\)
\( l_0 \times 1.5\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)



- AM of baryons originates from initial conditions…
- can be controlled…
- and regulate galaxy morphology
- Negligible AGN/SN global self-regulation
Galaxy formation
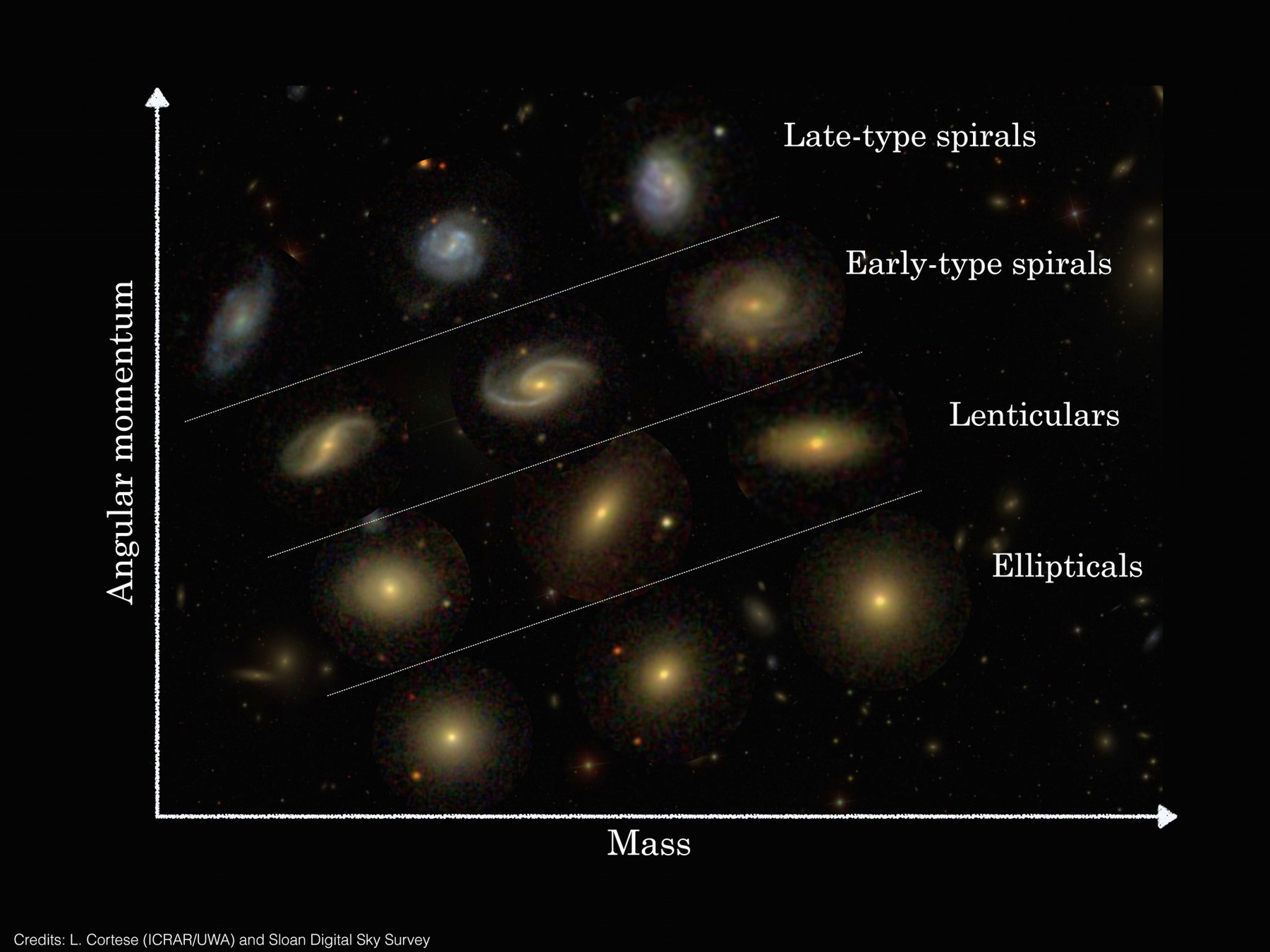
[L. Cortese; SDSS.]
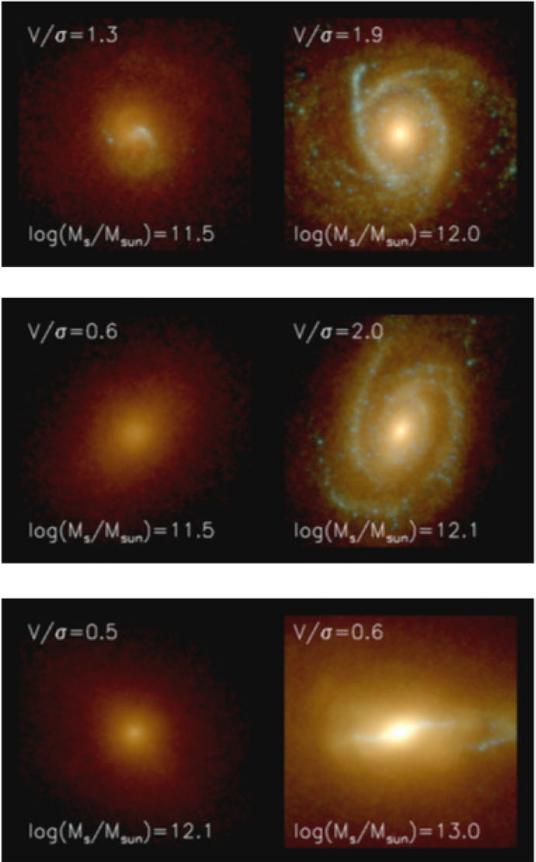


[Dubois+16]
AGN no AGN
Origin of morphological diversity at fixed mass?

[L. Cortese; SDSS.]



[Dubois+16]
AGN no AGN
Origin of morphological diversity at fixed mass?
How to explain environmental effects?
[Kraljic+ in prep]

Galaxy formation

[Danovich+15]
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
III. Torque down in inner halo
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
III. Torque down in inner halo
IV. Mixing in inner disk & bulge
The origin of high \(z\) angular momentum
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
See Cadiou+21c
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?