On the causal origin of angular momentum
of dark matter halos and galaxies



With A. Pontzen & H. Peiris: 2012.02201
Corentin Cadiou | Journées du PNCG, Strasbourg



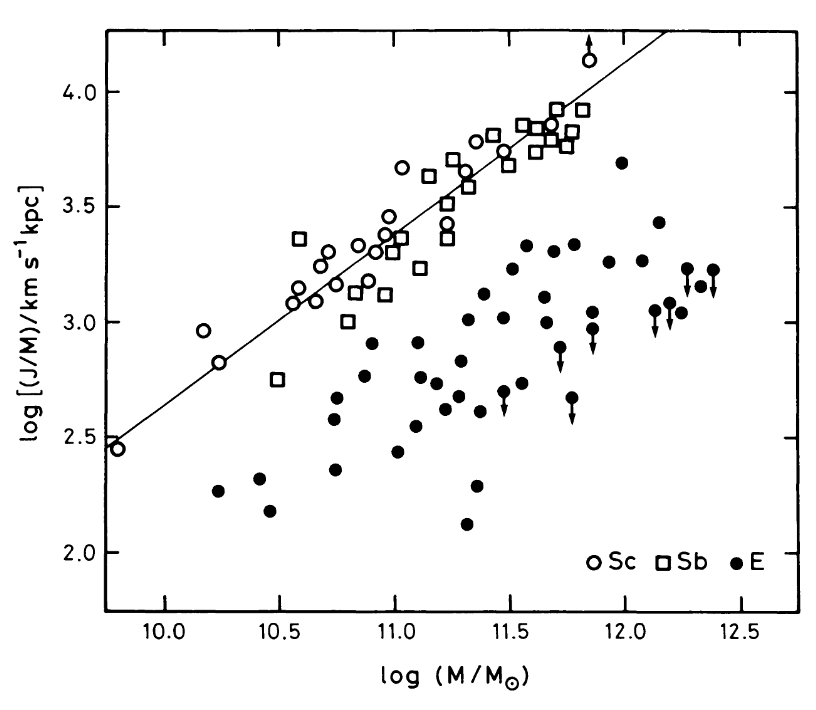
Fall 83 (\(z=0\))
Spiral galaxies \(\leftrightarrow\) high \(J_\star\)
3 variables: \(M_\star, J_\star\), morpho.
What causes what?
Some possible scenarios:
- Quench then spin down?
- Grow & spin down then quench?
- Spin down then quench
Galaxy formation & angular momentum
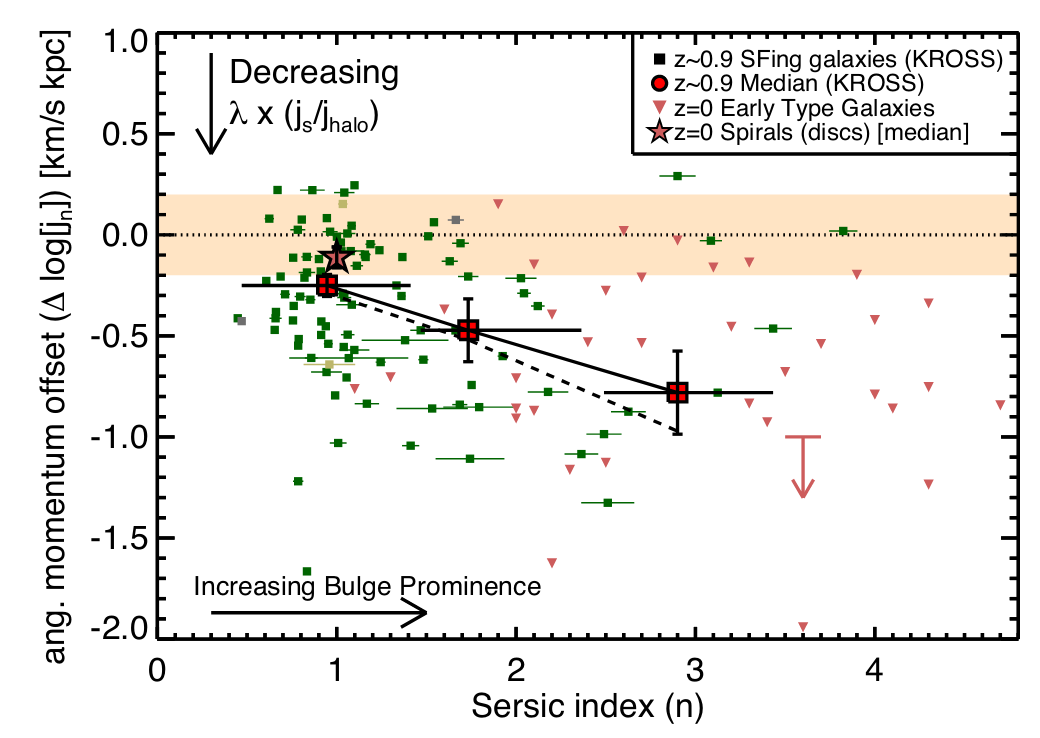
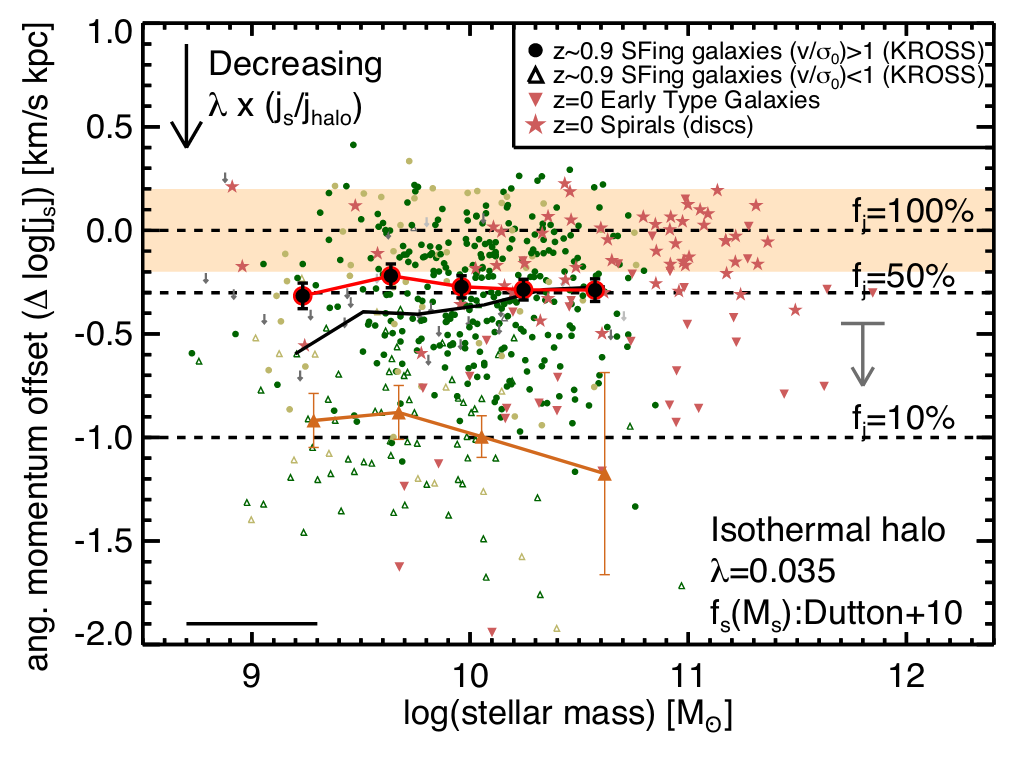
Harrison+17 (KMOS, \(z=1\))






Spiral galaxies \(\leftrightarrow\) high \(J_\star\): also at high-\(z\)
\(M_\star, J_\star,M_\mathrm{BH}\), morpho, bulge fraction, …
What causes what?
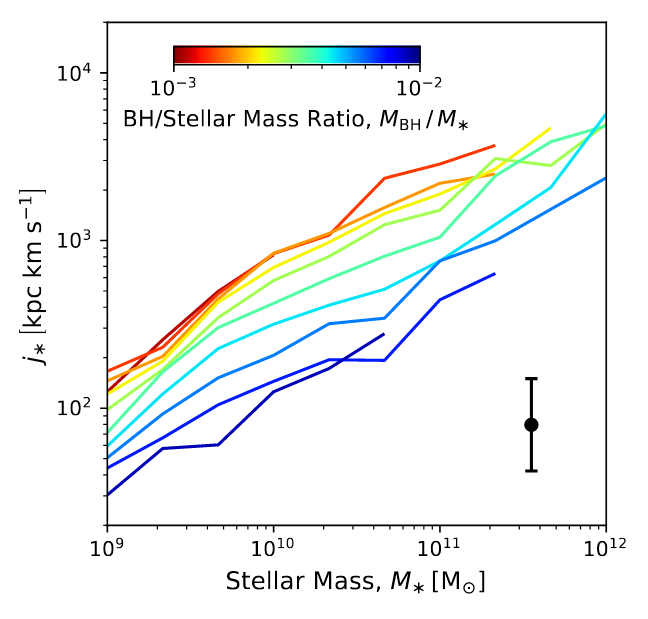
Rodriguez-Gomez+22 (TNG)


Cosmology & angular momentum

Correlation between environment & galaxy properties (cf previous session!)
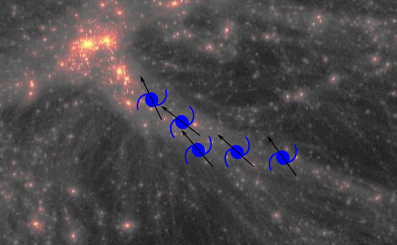

Weak lensing signal
Classical model of gal form


1
2
3
\(M_\mathrm{DM}, j_\mathrm{DM}, \dots\)
4
\(M_\star, j_\star, \dots\)

Hierarchical clustering
\(N\)-body physics
Accretion of gas
star form, feedback, cooling, ...
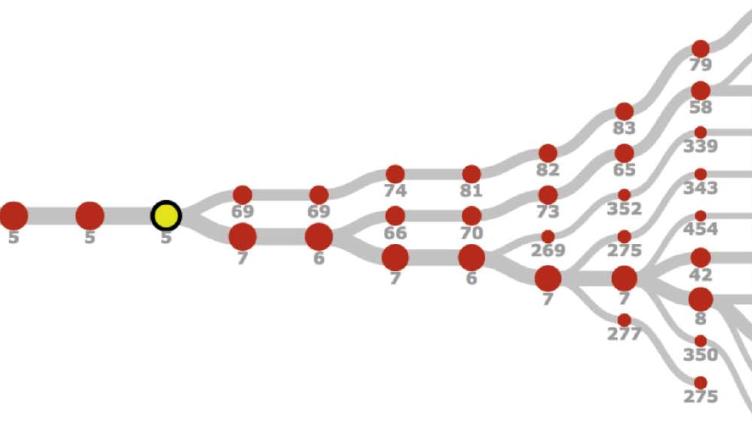
Classical model of gal form


1
2
\(M_\mathrm{DM}, j_\mathrm{DM}, \dots\)
4
\(M_\star, j_\star, \dots\)

Hierarchical clustering
\(N\)-body physics
Accretion of gas
star form, feedback, cooling, ...

Part I
3
Classical model of gal form


1
2
\(M_\mathrm{DM}\),
\(j_\mathrm{DM}, \dots\)
4
\(M_\star\),
\(j_\star, \dots\)

Hierarchical clustering
\(N\)-body physics
Accretion of gas
star form, feedback, cooling, ...

Part I
Part II
3
The origin of DM angular momentum

\(j_\mathrm{DM}\)

Predicting angular momentum



\(z=0\)
\( z = 100\)
Predicting angular momentum



\(z=0\)
\( z = 100\)
\[\mathbf{L}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
Position w.r.t. center
Velocity
[White 84]

\(\mathbf{r}\)\(\times\)\(\mathbf{v}\)
Predicting angular momentum



\(z=0\)
\( z = 100\)
[Porciani+02]

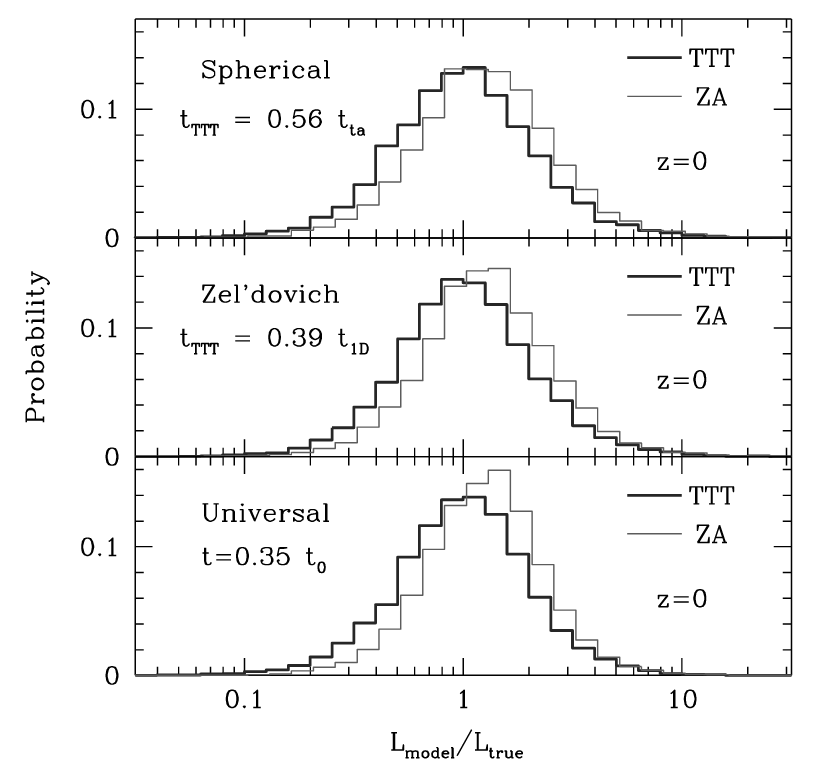
Scatter of 1 dex!
Predicting angular momentum



\(z=0\)
\( z = 100\)
[Genetic modifications: Roth+16, see also Rey&Pontzen 18, Stopyra+20]



Predicting angular momentum










Predicting angular momentum











Predicting angular momentum

- Angular momentum of individual regions can be predicted accurately.
- AM of halos ⇒ requires boundaries of patch


\[\mathbf{L}_\mathrm{lin.} \propto \int\mathrm{d}^3q(\mathbf{q}-\bar{\mathbf{q}})\times \nabla\phi\]
[On patch boundaries: see Lucie-Smith+18]
Can we control baryonic
angular momentum?

\(j_\star\)

Can we control baryonic
angular momentum?
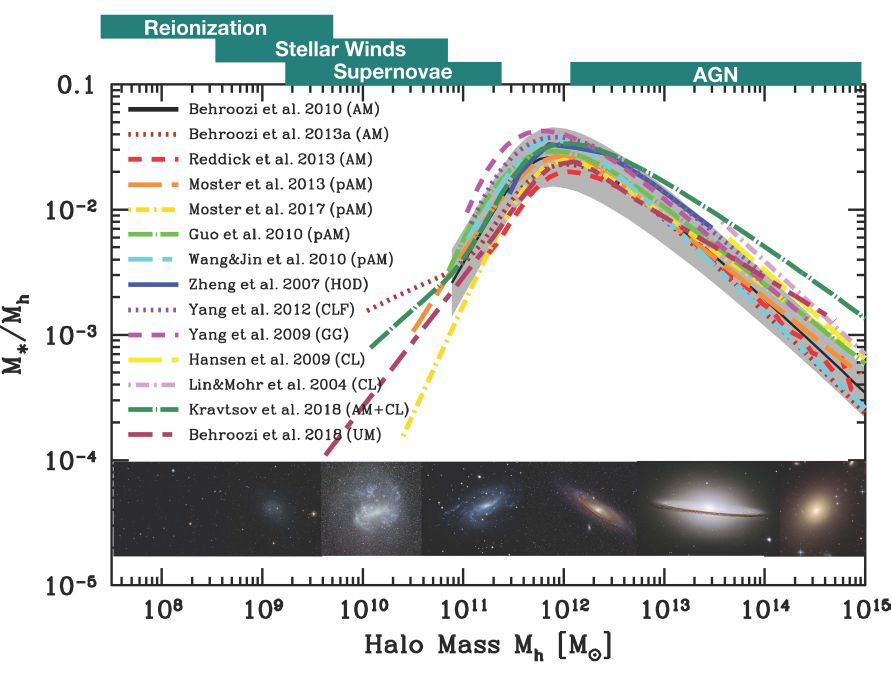
Wechsler & Tinker 18
\({\color{red}M_\star} / M_\mathrm{h} \ll \Omega_b / \Omega_m \)
⇒ baryons & DM stem from different regions


Baryons more strongly bound
⇒ less prone to being ejected


[On ≠ patch: see Liao+17]
Numerical setup
Simulations (9Mh @ DiRAC):
- Resolution \(\Delta x = 35\ \mathrm{kpc}\)
- \(M_\mathrm{200c} = 10^{12}\ \mathrm{M}_\odot\) @ \(z=2\)
- Modify \(l(z=2)\)
- SF + AGN & SN feedback (New-Horizon model, Dubois+21)
-
Tracer particles
Cadiou+19
\( l_0 \times 0.66\)
\( l_0 \times 0.8\)
\( l_0 \times 1.2\)
\( l_0 \times 1.5\)
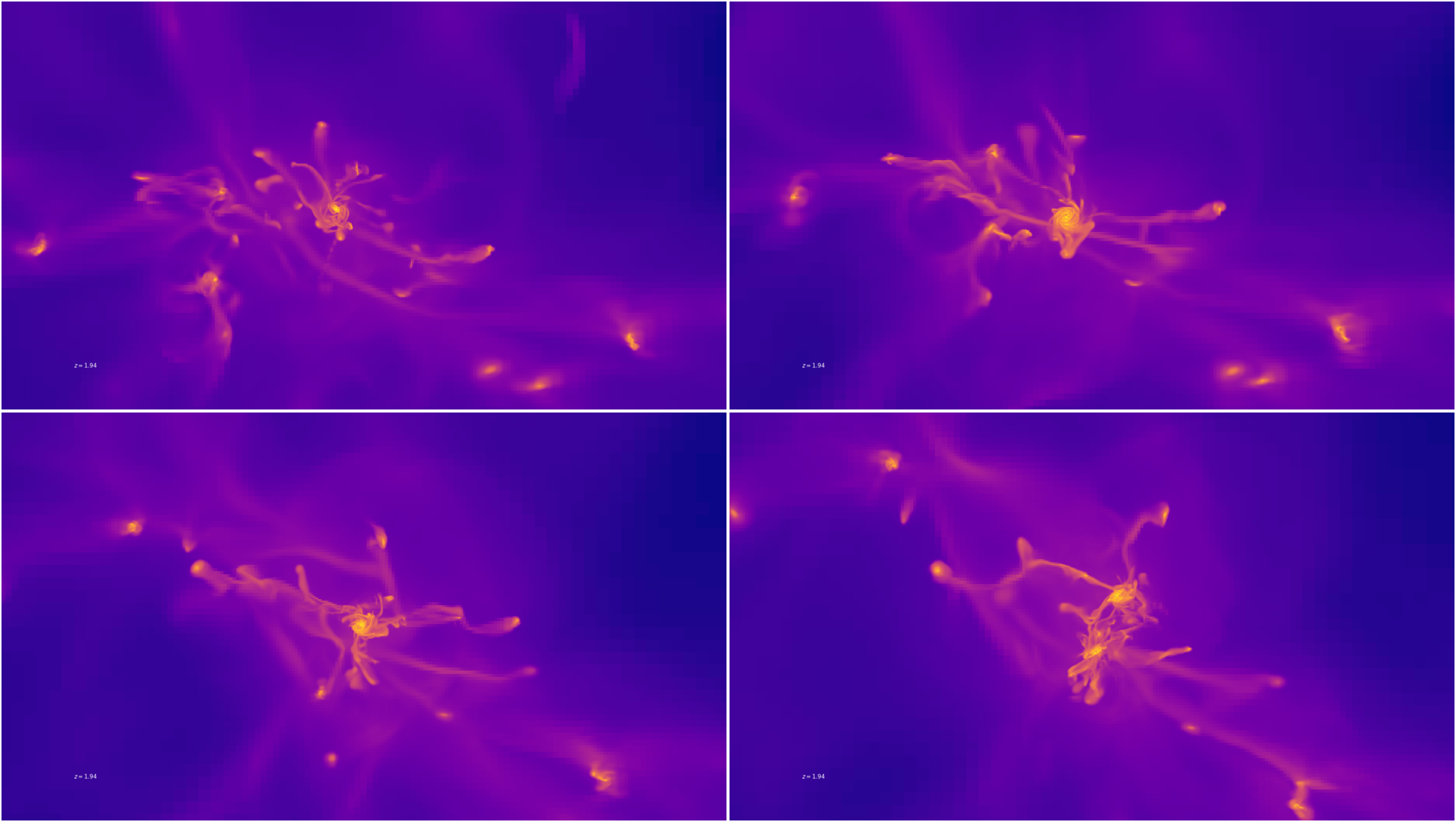


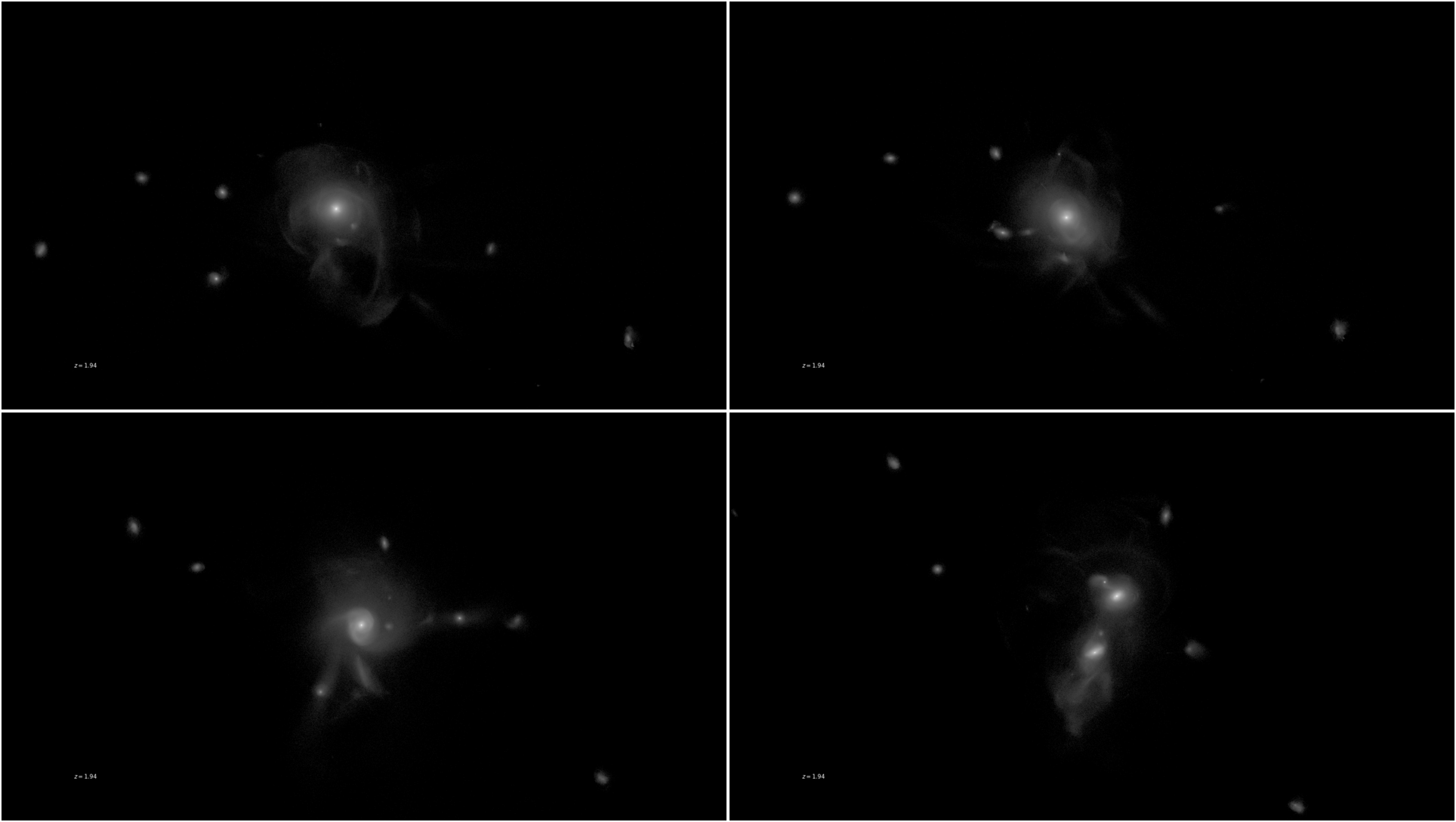
INPUT: Modified at \(z=200\)
OUTPUT:
Measured at \(z=200\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)
\( l_0 \times 1.2\)
\( l_0 \times 1.5\)
\( l_0 \times 0.66\)
\( l_0 \times 0.8\)
\( l_0 \times 1.2\)
\( l_0 \times 1.5\)






What drives AM up/down?
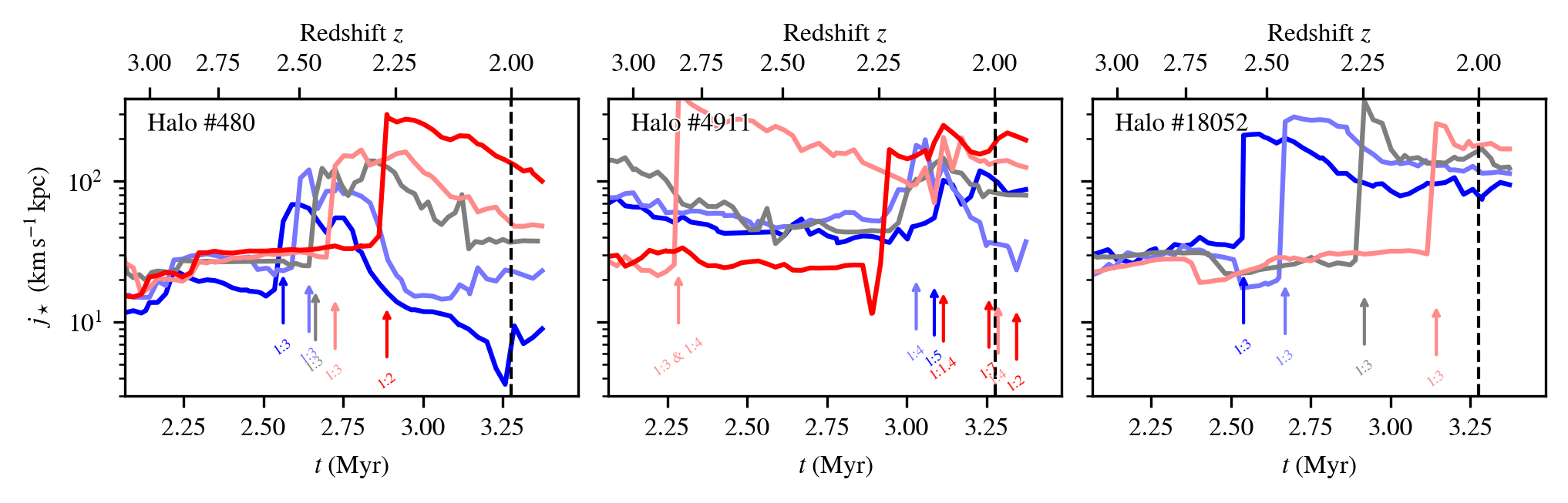
PRELIMINARY
PRELIMINARY
Conclusion & outlook
Conclusion & outlook
Questions?
More infos in Cadiou+21 (2012.02201)
Cadiou+22 (should appear this week!)
-
angular momentum of DM is predictable
-
boundary of halos in the ICs is a hard problem
⇒ limits practicality of predictions
-
stellar AM can be controlled!
⇒ imposed by mergers
⇒ controls B/T, \(v/\sigma\), morpho, …
⇒ weak lensing predictions easier than expected?


⚠️
\(j_\mathrm{DM}\)
⚠️
✅
\(j_\star\)

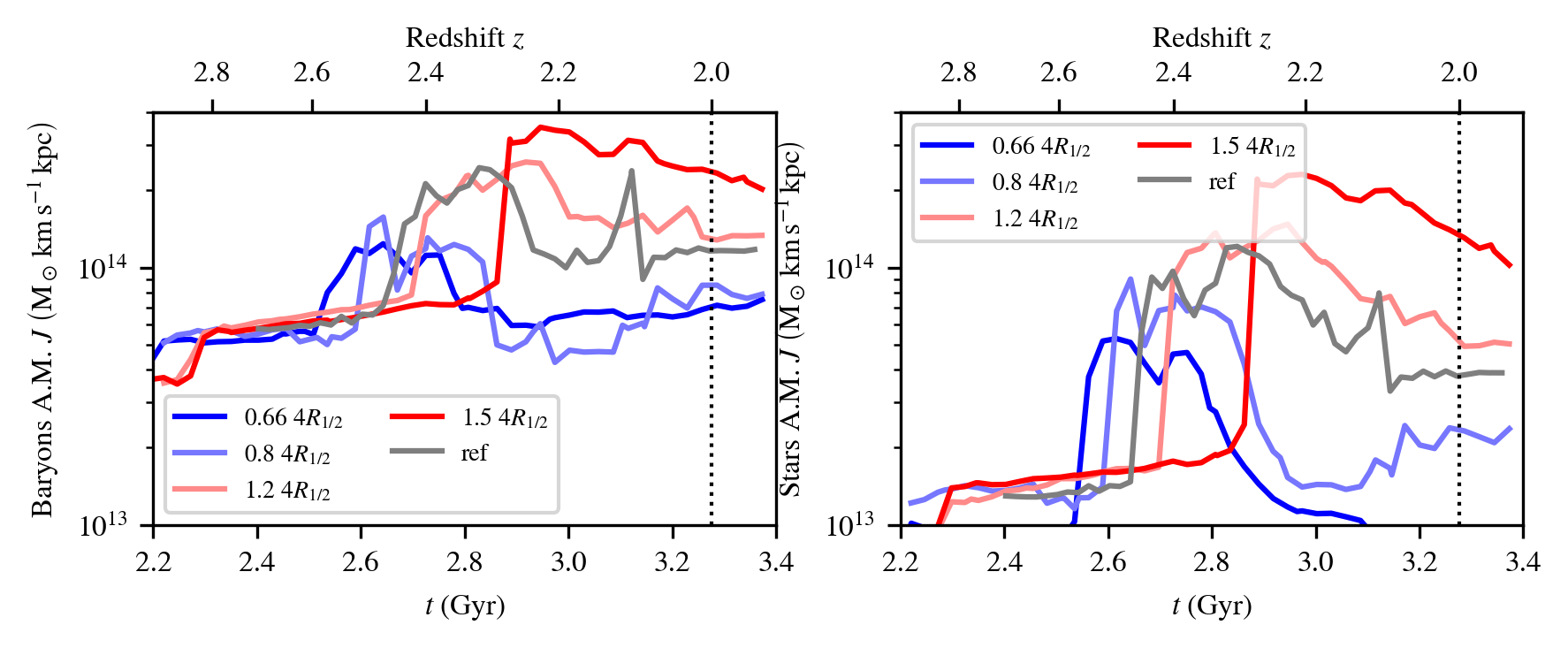

- AM of stars originates from initial conditions…
- can be controlled…
- \(j_\star\) regulate galaxy disk, bulge, \(v/\sigma\), \(R_{1/2}\)
- Negligible AGN/SN global self-regulation

\( l_0 \times 1.2\)
\( l_0 \times 1.5\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)
Temporary conclusions
-
angular momentum is predictable
-
boundary of halos in the ICs is a hard problem
⇒ limits practicality of predictions (for now)
-
baryons appear to be simpler!
⇒ good news for weak lensing predictions
⇒ key to understand morphology
-
but why do some objects grow their AM faster/slower?




Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Verify that
\[\xi_\mathrm{lin}(r) \sim \left\langle {\color{green}\underbrace{\delta(x=d)}_\mathrm{in}} {\color{purple} \underbrace{\delta(x=d+r)}_\mathrm{out}}\right\rangle \]
is the same in spliced / ref simulation.

Galaxy formation
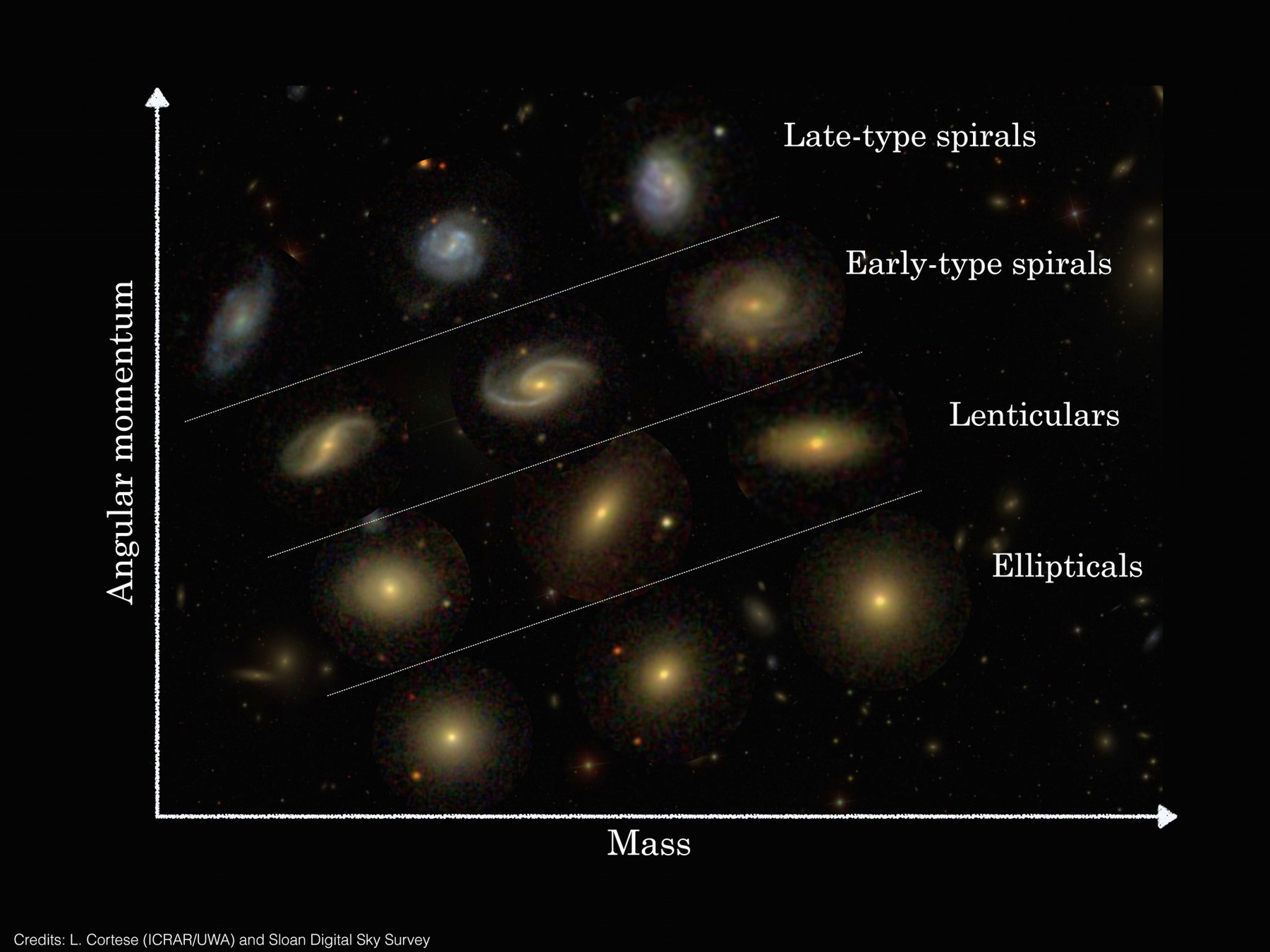
[L. Cortese; SDSS.]
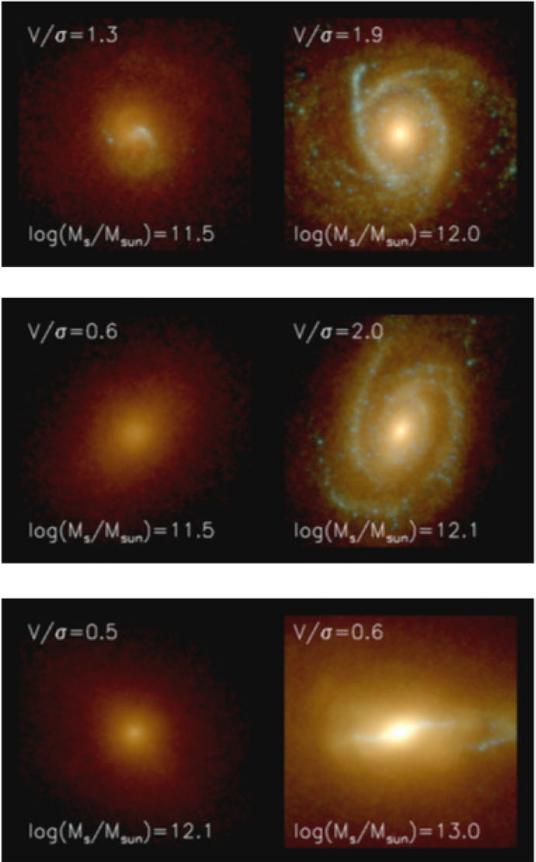


[Dubois+16]
AGN no AGN
Origin of morphological diversity at fixed mass?

[L. Cortese; SDSS.]



[Dubois+16]
AGN no AGN
Origin of morphological diversity at fixed mass?
How to explain environmental effects?

Galaxy formation


[Danovich+15]
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
III. Torque down in inner halo
The origin of high \(z\) angular momentum

[Danovich+15]
I. Torque with cosmic web
II. Transport at constant AM
III. Torque down in inner halo
IV. Mixing in inner disk & bulge
The origin of high \(z\) angular momentum
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
See Cadiou+21c
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?
The origin of high \(z\) angular momentum

[Danovich+15]
IV. Mixing in inner disk & bulge
Fraction that ends up in disk vs. IGM?
Influence of galactic physics?
III. Torque down in inner halo
Origin of torque down (pressure or gravity)?
Loss of link with cosmic AM?
II. Transport at constant AM
Same evolution in cold/hot accretion modes?
I. Torque with cosmic web
Predict pre-accretion AM?
Alignment with environment?



+


\( R_{1/2} \)
\( l_0 \times 1.2\)
\( l_0 \times 1.5\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)
\( l_0 \times 1.2\)
\( l_0 \times 1.5\)


\( l_0 \times 1.2\)
\( l_0 \times 1.5\)

\( l_0 \times 0.66\)
\( l_0 \times 0.8\)


