Christopher Makler

Stanford University Department of Economics

Econ 50: Lecture 14

Resource Constraints and Production Possibilities

Today's Agenda

Part 1: Resource Constraints and the PPF

Part 2: Optimization 

The "Desert Island" Model

Resource constraints and the PPF

Deriving the equation of the short-run PPF

Shifts in the PPF

The Marginal Rate of Transformation

Relationship between MPL and MRT

The "Gravitational Pull" argument

Tangency when calculus works

Corners and kinks

 

The “Desert Island" Model

Economics is the study of how
we use scarce resources 
to satisfy our unlimited wants

Resources

Goods

Happiness

🌎

⌚️

🤓

Production functions

Utility functions

Demand

Supply

Equilibrium

🤩

🏪

⚖

Wednesday: Little Green Pieces of Paper

Multiple Uses of Resources

Labor

Fish

🐟

Coconuts

🥥

[GOOD 1]

⏳

[GOOD 2]

L_1
L_2
\overline L

Resource Constraint

L_1
L_2
L_1 + L_2 = \overline L
\overline L
\overline L
\text{Total labor available }=\overline L

Production Possibilities

\text{Fish }(x_1)
\text{Coconuts }(x_2)

Resource Constraint

L_1
L_2
L_1 + L_2 = \overline L
\overline L
\overline L
\text{Total labor available }=\overline L
\text{(Good 1 - Good 2 space)}

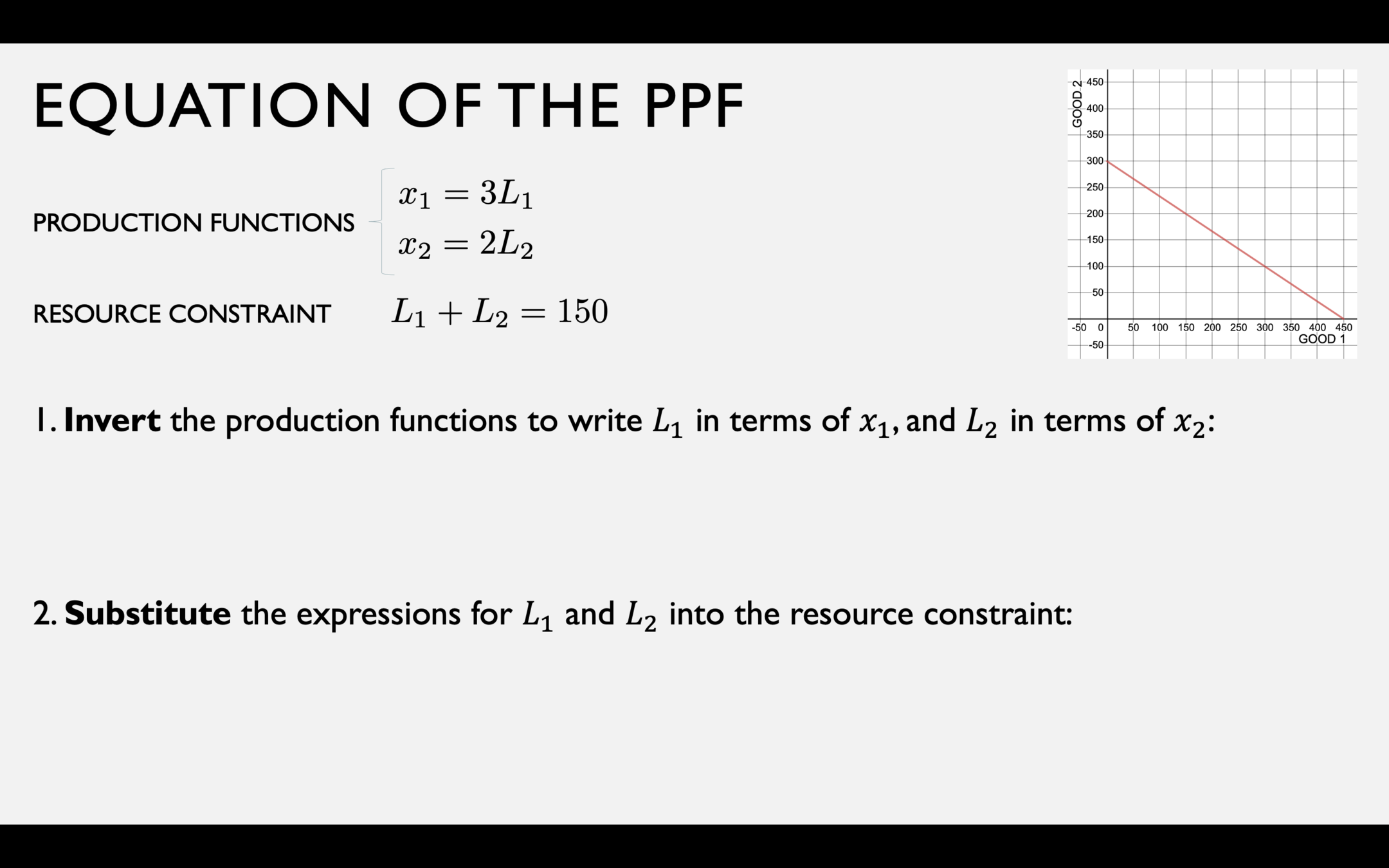
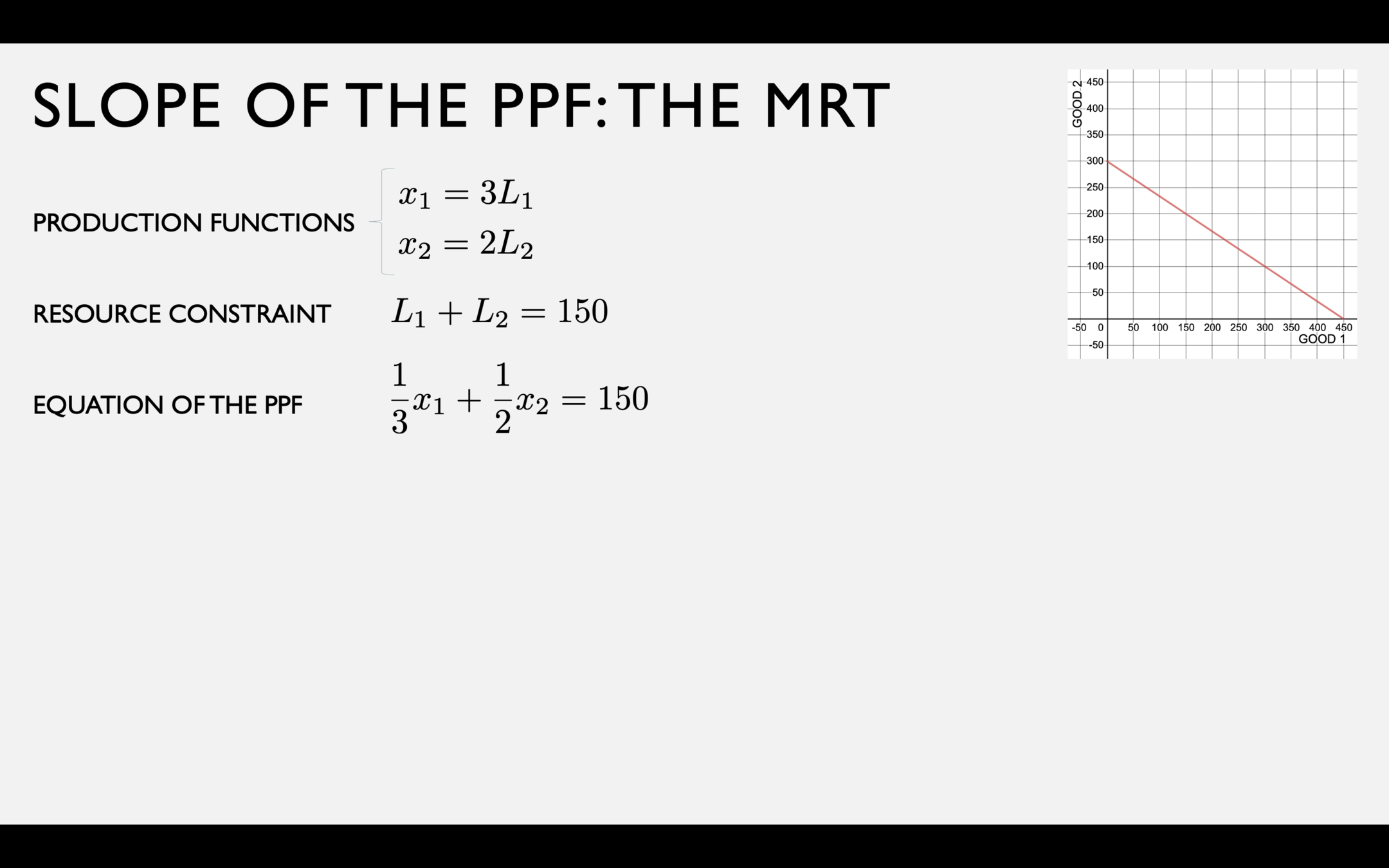
A PPF with Linear Technologies

x_1 = f_1(L_1) = 3L_1
x_2 = f_2(L_2) = 2L_2

Fish production function

Coconut production function

Resource Constraint

L_1 + L_2 = 150

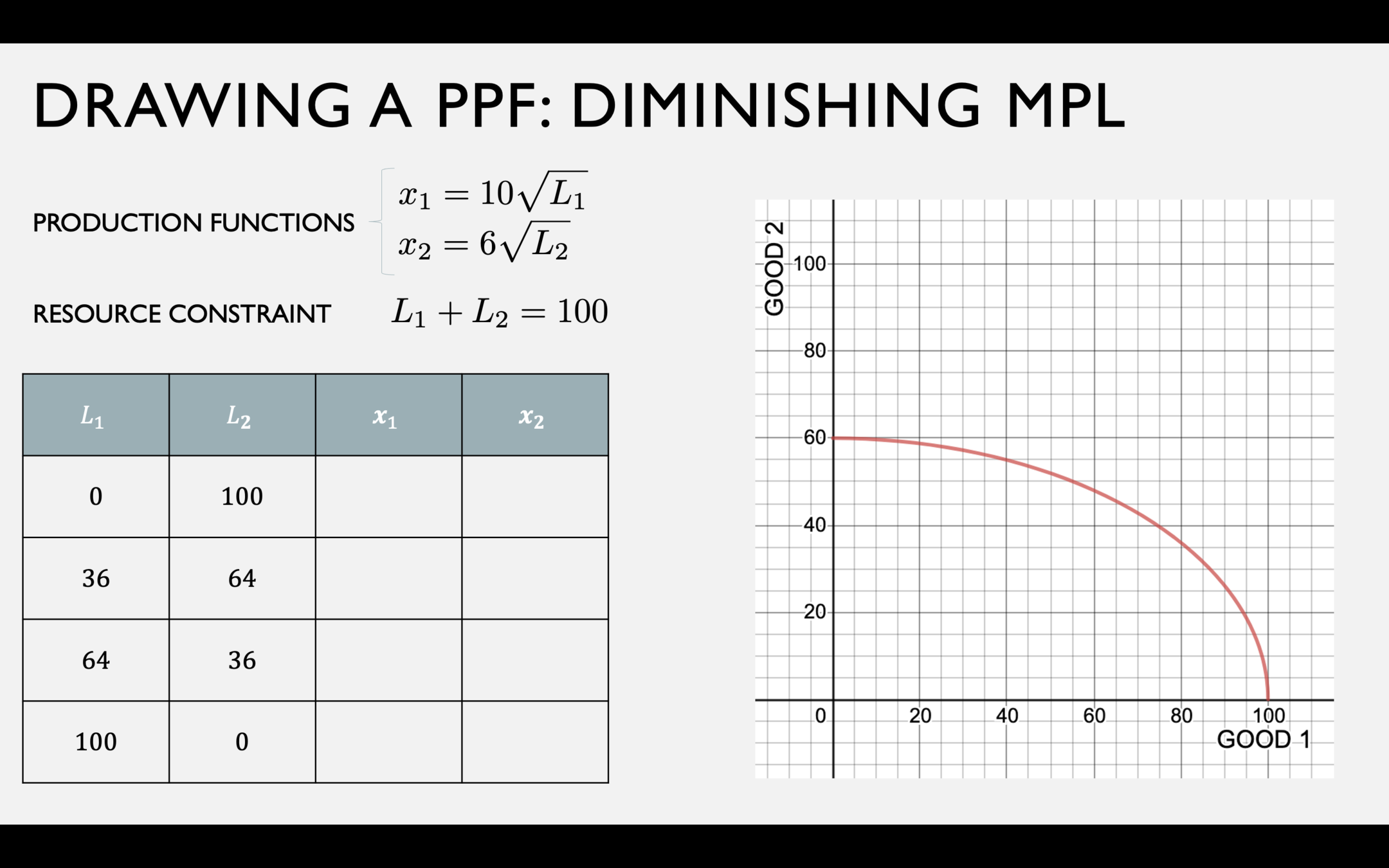
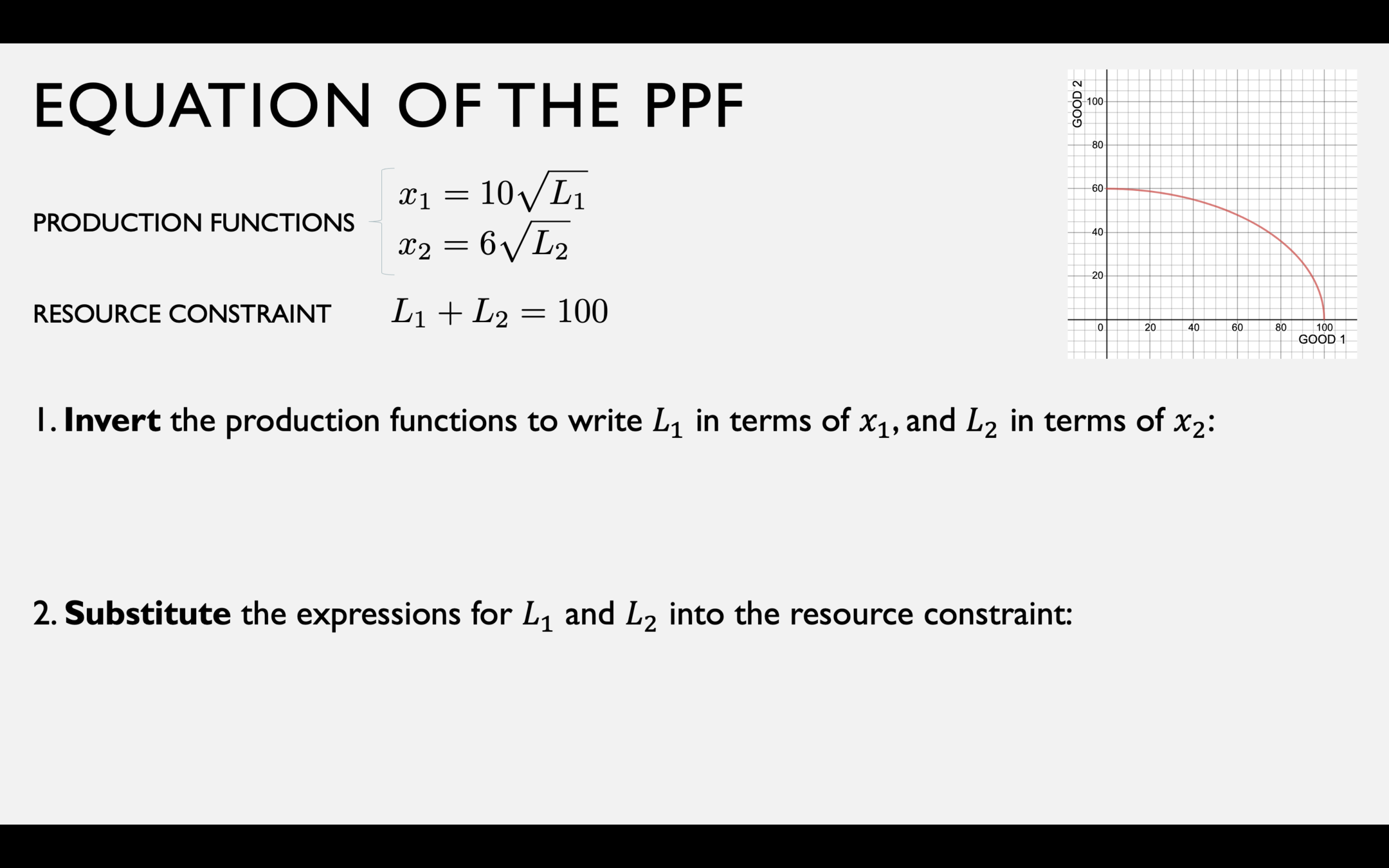
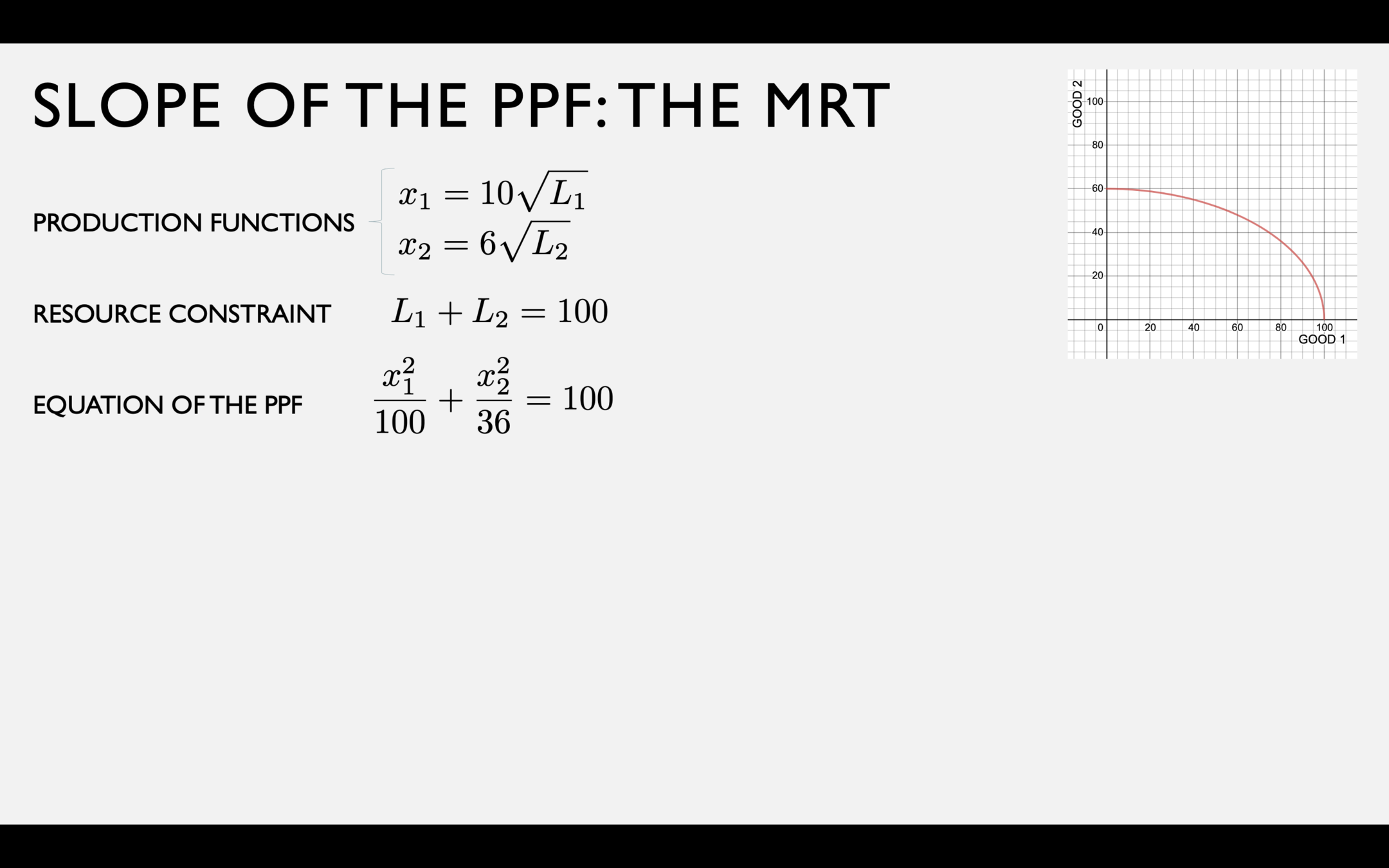
A PPF with Diminishing \(MP_L\)

x_1 = f_1(L_1) = 10\sqrt{L_1}
x_2 = f_2(L_2) = 6\sqrt{L_2}

Fish production function

Coconut production function

Resource Constraint

L_1 + L_2 = 100
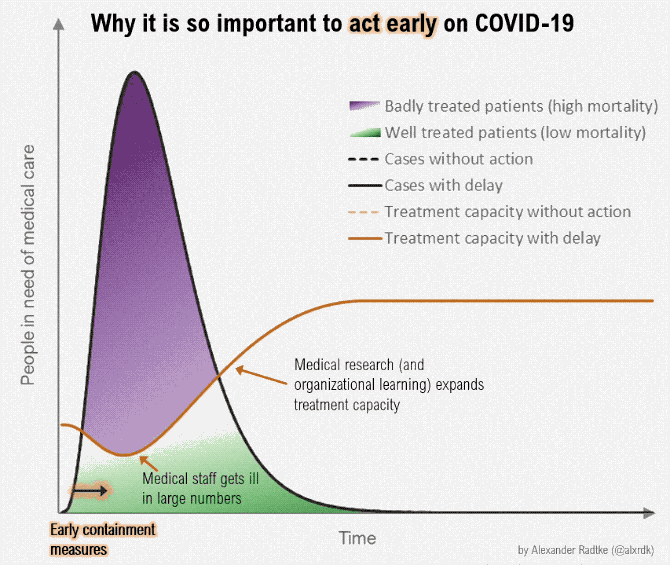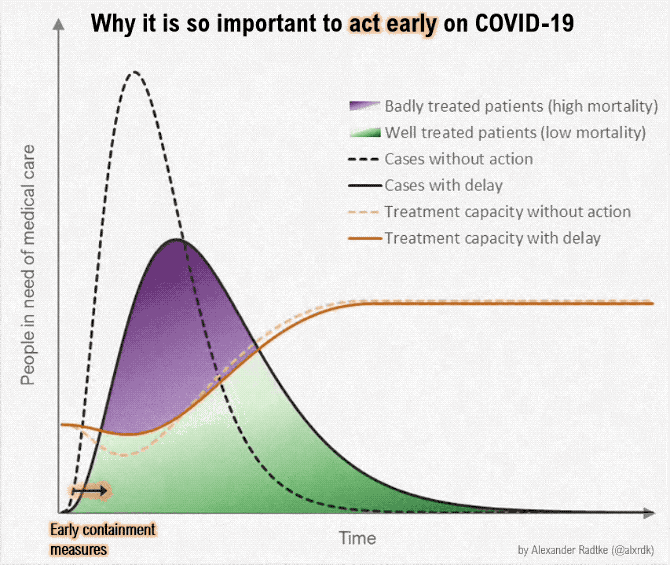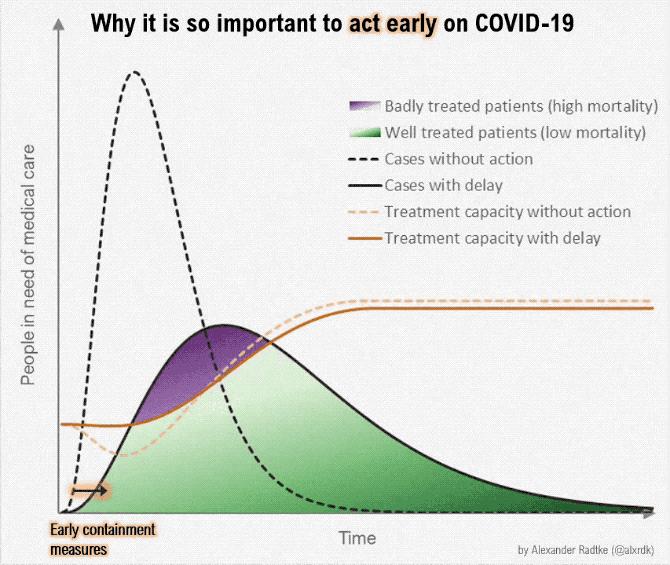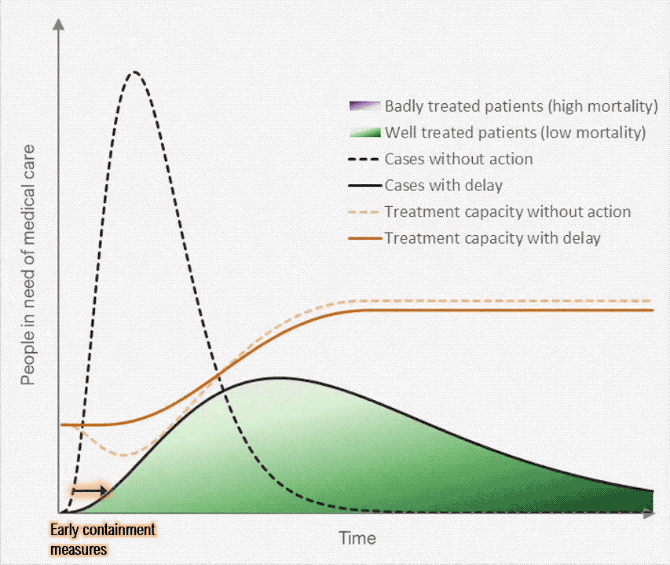
  • Suppose we want to produce a lot more of something -- ventilators, masks, toilet paper, hand sanitizer
  • Some resources can be reallocated quickly; others are more specialized and can't be quickly repurposed
  • How can we "scale up" in the short run and the long run?
  • How do short-run tradeoffs compare with long-run tradeoffs?

Shifts in the PPF

f_1(L_1) = {5 \over 4}\sqrt{L_1K_1}
f_2(L_2) = \sqrt{L_2K_2}

Consider an economy with \(\overline L = 100\) units of labor and \(\overline K = 100\) units of capital.

In the short run, \(K_1 = 64\) and \(K_2 = 36\).

In the long run, capital can be reallocated in any combination between goods 1 and 2.

Max in SR

Max in LR

  • Up to now: how a short-run PPF can shift due to changing the allocation of capital, holding production functions constant.
  • What happens when the technology itself (i.e. the production function) changes?

Improvements in Technology

The New York Times, Oct. 29, 2013

Insider, July 23, 2020

f_1(L_1) = A_1\sqrt{L_1K_1}
f_2(L_2) = A_2\sqrt{L_2K_2}

Consider an economy with \(\overline L = 100\) units of labor and \(\overline K = 100\) units of capital.

In the short run, \(K_1 = 64\) and \(K_2 = 36\).

In the long run, capital can be reallocated in any combination between goods 1 and 2.

Max in SR

Max in LR

The Slope of the PPF

Slope of the PPF:
Marginal Rate of Transformation (MRT)

Rate at which one good may be “transformed" into another

...by reallocating resources from one to the other.

Opportunity cost of producing an additional unit of good 1,
in terms of good 2

Note: we will generally treat this as a positive number
(the magnitude of the slope)

Suppose we're allocating 100 units of labor to fish (good 1),
and 50 of labor to coconuts (good 2).

Now suppose we shift
one unit of labor
from coconuts to fish.

How many fish do we gain?

\Delta_2
\Delta_1

100

98

300

303

How many coconuts do we lose?

\Delta_2 = MP_{L_2} = 2\text{ coconuts}
\Delta_1 = MP_{L_1} = 3\text{ fish}
\left|\text{slope}\right| = \frac{MP_{L_2}}{MP_{L_1}}

Relationship between MPL's and MRT

x_1 = f_1(L_1) = 3L_1
x_2 = f_2(L_2) = 2L_2

Fish production function

Coconut production function

Resource Constraint

L_1 + L_2 = 150

PPF

Diminishing \(MP_L\)'s
and Increasing \(MRT\)

Important Notes

The MRT is the slope of the PPF at some output combination \((x_1,x_2)\)

You should therefore write it in terms of \(x_1\) and \(x_2\), not \(L_1\) and \(L_2\).

You can use two methods to find the MRT:
the ratio of the MPL's, or the implicit function theorem.

CHECK YOUR UNDERSTANDING

Chuck has \(\overline L = 8\) total hours of labor,
and the production functions
\(x_1 = 2 \sqrt{L_1}\) and \(x_2 = 4\sqrt{L_2}\).

 

What is his MRT if he spends
half his time producing each good?

CHECK YOUR UNDERSTANDING

Charlene has the PPF given by
\(2x_1^3 + 3x_2^4 = 1072\)

What is her MRT if she produces the output combination \((8,2)\)?

Verbal Analysis: MRS, MRT, and the “Gravitational Pull" towards Optimality 

Fish vs. Coconuts

  • Can spend your time catching fish (good 1)
    or collecting coconuts (good 2)
  • What is your optimal division of labor
    between the two?
  • Intuitively: if you're optimizing, you
    couldn't reallocate your time in a way
    that would make you better off.
  • The last hour devoted to fish must
    bring you the same amount of utility
    as the last hour devoted to coconuts

Marginal Rate of Transformation (MRT)

  • The  number of coconuts you need to give up in order to get another fish
  • Opportunity cost of fish in terms of coconuts

Marginal Rate of Substitution (MRS)

  • The number of coconuts you are willing to give up in order to get another fish
  • Willingness to "pay" for fish in terms of coconuts

Both of these are measured in
coconuts per fish
(units of good 2/units of good 1)

Marginal Rate of Transformation (MRT)

  • The  number of coconuts you need to give up in order to get another fish
  • Opportunity cost of fish in terms of coconuts

Marginal Rate of Substitution (MRS)

  • The number of coconuts you are willing to give up in order to get another fish
  • Willingness to "pay" for fish in terms of coconuts

Opportunity cost of marginal fish produced is less than the number of coconuts
you'd be willing to "pay" for a fish.

Opportunity cost of marginal fish produced is more than the number of coconuts
you'd be willing to "pay" for a fish.

Better to spend less time fishing
and more time making coconuts.

Better to spend more time fishing
and less time collecting coconuts.

MRS
>
MRT
MRS
<
MRT

Better to produce
more good 1
and less good 2.

MRS
>
MRT
MRS
<
MRT

“Gravitational Pull" Towards Optimality

Better to produce
more good 2
and less good 1.

These forces are always true.

In certain circumstances, optimality occurs where MRS = MRT.

Graphical Analysis:
PPFs and Indifference Curves

The story so far, in two graphs

Production Possibilities Frontier
Resources, Production Functions → Stuff

Indifference Curves
Stuff → Happiness (utility)

Both of these graphs are in the same "Good 1 - Good 2" space

Better to produce
more good 1
and less good 2.

MRS
>
MRT

Better to produce
less good 1
and more good 2.

MRS
<
MRT

When Calculus Fails:
Kinks and Corners

MRS = {100 \over x_1}

What would Lagrange find...?

MRS = {100 \over x_1}

What would Lagrange find...?

MRT = {5 \over \sqrt{100 - x_1}}

What would Lagrange find...?

Kinks (Discontinuities)

Kinked Indifference Curve:

Kinked Constraint:

Discontinuities in the MRS
(e.g. Perfect Complements utility function)

Discontinuities in the MRT
(e.g. homework question with two factories)

PPF: x_1^2 + x_2^2 = 80
u(x_1,x_2) = \min\left\{{x_1 \over 2}, x_2\right\}

What are some bundles that give the same utility as (4,8)?

What is the MRS at (4,8)? What about in the other place where the indifference curve intersects the PPF?

Here are some more indifference curves.
Where is the optimal bundle? 

How can we solve for the optimal bundle?

PPF: x_1^2 + x_2^2 = 80
u(x_1,x_2) = \min\left\{{x_1 \over 2}, x_2\right\}