Trading from an Endowment

Christopher Makler

Stanford University Department of Economics

Econ 51: Lecture 2

Today's Agenda

Part 1: Exchange Optimization

Part 2: Comparative Statics

The endowment budget line

Optimal choice with a constant price

Different prices for buying and selling

Optimization at general prices

Gross demand and net demand

Net demand and net supply

Applications: Labor Supply, Intertemporal Choice, Risk and Uncertainty

 

Marginal Rate of Substitution

X = (10,24)

🍏🍏

🍏🍏

🍏🍏

🍏🍏

🍏🍏

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

Y=(12,20)

🍏🍏

🍏🍏

🍏🍏

🍏🍏

🍏🍏

🍏🍏

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

🍌🍌🍌🍌

Suppose you were indifferent between the following two bundles:

Starting at bundle X,
you would be willing
to give up 4 bananas
to get 2 apples

Let apples be good 1, and bananas be good 2.

Starting at bundle Y,
you would be willing
to give up 2 apples
to get 4 bananas

MRS = {4 \text{ bananas} \over 2 \text{ apples}}
= 2\ {\text{bananas} \over \text{apple}}

Visually: the MRS is the magnitude of the slope
of an indifference curve

Econ 50

Consumer starts with money,
can buy a bundle of goods.

Econ 51

Consumer starts with a bundle,
can trade one good for another

Straight trade/barter

Sell some of one good for money,
use the money to buy the other good

Trading from an Endowment

Good 1

Good 2

e_2
e_1
E

Note: lots of different notation for the endowment bundle!

 

Varian uses \(\omega\), some other people use \(x_1^E\)

x_2
x_1
X

Suppose you'd like to move from that endowment to some other bundle X

You start out with some endowment E

This involves trading some of your good 1 to get some more good 2

\Delta x_1
\Delta x_2
\Delta x_1 = e_1 - x_1
\Delta x_2 = x_2 - e_2

Buying and Selling

Good 1

Good 2

e_2
e_1
E
x_2
x_1
X

If you can't find someone to trade good 1 for good 2 directly, you could sell some of your good 1 and use the money to buy good 2.

Suppose you sell \(\Delta x_1\) of good 1 at price \(p_1\). How much money would you get?

Suppose you wanted to buy \(\Delta x_2\) of good 2 at price \(p_2\). How much would that cost?

p_1 \Delta x_1
p_2 \Delta x_2
\Delta x_1
\Delta x_2
\Delta x_1 = e_1 - x_1
\Delta x_2 = x_2 - e_2
=
p_1 (e_1 - x_1)
=
p_2 (x_2 - e_2)

Buying and Selling

Good 1

Good 2

e_2
e_1
E
x_2
x_1
X
\Delta x_1
\Delta x_2
\Delta x_1 = e_1 - x_1
\Delta x_2 = x_2 - e_2
p_1 (e_1 - x_1)
=
p_2 (x_2 - e_2)

If the amount you get from selling good 1 exactly equals the amount you spend on good 2, then

p_2x_2 - p_2e_2 = p_1e_1 - p_1x_1
p_1x_1 + p_2x_2 = p_1e_1 +p_2e_2

monetary value of \(E\)
at market prices

monetary value of \(X\)
at market prices

(Basically: you can afford any bundle with the same monetary value as your endowment.)

Endowment Budget Line

Good 1

Good 2

e_2
e_1
E
p_1x_1 + p_2x_2 = p_1e_1 +p_2e_2

If you sell all your good 1 for \(p_1\),
how much good 2 can you consume?

If you sell all your good 2 for \(p_2\),
how much good 1 can you consume?

If \(x_1 = 0\):

If \(x_2 = 0\):

x_2 = e_2 + {p_1e_1 \over p_2}
x_1 = e_1 + {p_2e_2 \over p_1}

Endowment Budget Line

Good 1

Good 2

e_2
e_1
E
p_1x_1 + p_2x_2 = p_1e_1 +p_2e_2
e_2 + {p_1e_1 \over p_2}
e_1 + {p_2e_2 \over p_1}

Liquidation value of your endowment

\hat m

Divide both sides by \(p_2\):

{p_1 \over p_2}x_1 + x_2 = {p_1 \over p_2} e_1 + e_2
{\hat m \over p_2} =

Divide both sides by \(p_1\):

x_1 + {p_2 \over p_1}x_2 = e_1 + {p_2 \over p_1}e_2
{\hat m \over p_1} =

In other words: the endowment budget line is just like a normal budget line,
but the amount of money you have is the liquidation value of your endowment.

Endowment Budget Line

p_1x_1+p_2x_2=p_1e_1+p_2e_2

Divide both sides by \(p_2\):

Divide both sides by \(p_1\):

The budget line only depends on the price ratio \({p_1 \over p_2}\),
not the individual prices.

{p_1 \over p_2}x_1 + x_2 = {p_1 \over p_2} e_1 + e_2
x_1 + {p_2 \over p_1}x_2 = e_1 + {p_2 \over p_1}e_2

Effect of a Change in Prices

What happens if the price of good 1 doubles?

What happens if both prices double?

pollev.com/chrismakler

Bob has an endowment of (8,8) and can buy and sell goods 1 and 2. What happens to his endowment budget line if the price of good 1 decreases? You may select more than one answer.

Optimization

Optimization problem with money

Optimization problem with an endowment

\displaystyle{\max_{x_1,x_2}\ u(x_1,x_2)}
\text{s.t. }p_1x_1 + p_2x_2 = m
\displaystyle{\max_{x_1,x_2}\ u(x_1,x_2)}
\text{s.t. }p_1x_1 + p_2x_2 = p_1e_1+p_2e_2

Procedure is exactly the same - we just have a different equation for the budget constraint.

pollev.com/chrismakler

Bob has the endowment (8,8) and the utility function $$u(x_1,x_2)=x_1x_2$$If he faces prices \(p_1 = 10\) and \(p_2 = 5\), what is his optimal choice?

Optimization: Income vs. Endowment

Recall: The “Gravitational Pull" Argument

MRS > {p_1 \over p_2}
MRS < {p_1 \over p_2}

Indifference curve is steeper
than the budget constraint

Can increase utility by
moving to the right
along the budget constraint

Indifference curve is flatter
than the budget constraint

Can increase utility by
moving to the left
along the budget constraint

Good 1

Good 2

pollev.com/chrismakler

Suppose Alison has the endowment (12,2) and the utility function $$u(x_1,x_2)=x_1x_2$$ If the price of good 2 is 6, for what price of good 1 will she be willing to sell some of her good 1? 

Different Prices for Buying and Selling

Tickets

Money

If you sell all your tickets,
how much money will you have?

If you spend all your money on additional tickets, how many tickets will you have?

Suppose you have 40 tickets and $1200.

40 \text{ tickets} \times \$25/\text{ticket} = \$1000
\$1000 + \$1200 = \$2200

1200

40

E

2200

Slope = \(p^{\text{sell}}\) = $25/ticket

Slope = \(p^{\text{buy}}\) = $60/ticket

\$1200 \div \$60/\text{ticket} = 20\text{ tickets}
40\text{ tickets} + 20\text{ tickets} = 60\text{ tickets}

You can sell tickets for $25 each,
or buy additional tickets for $60 each.

60

Part II: Comparative Statics

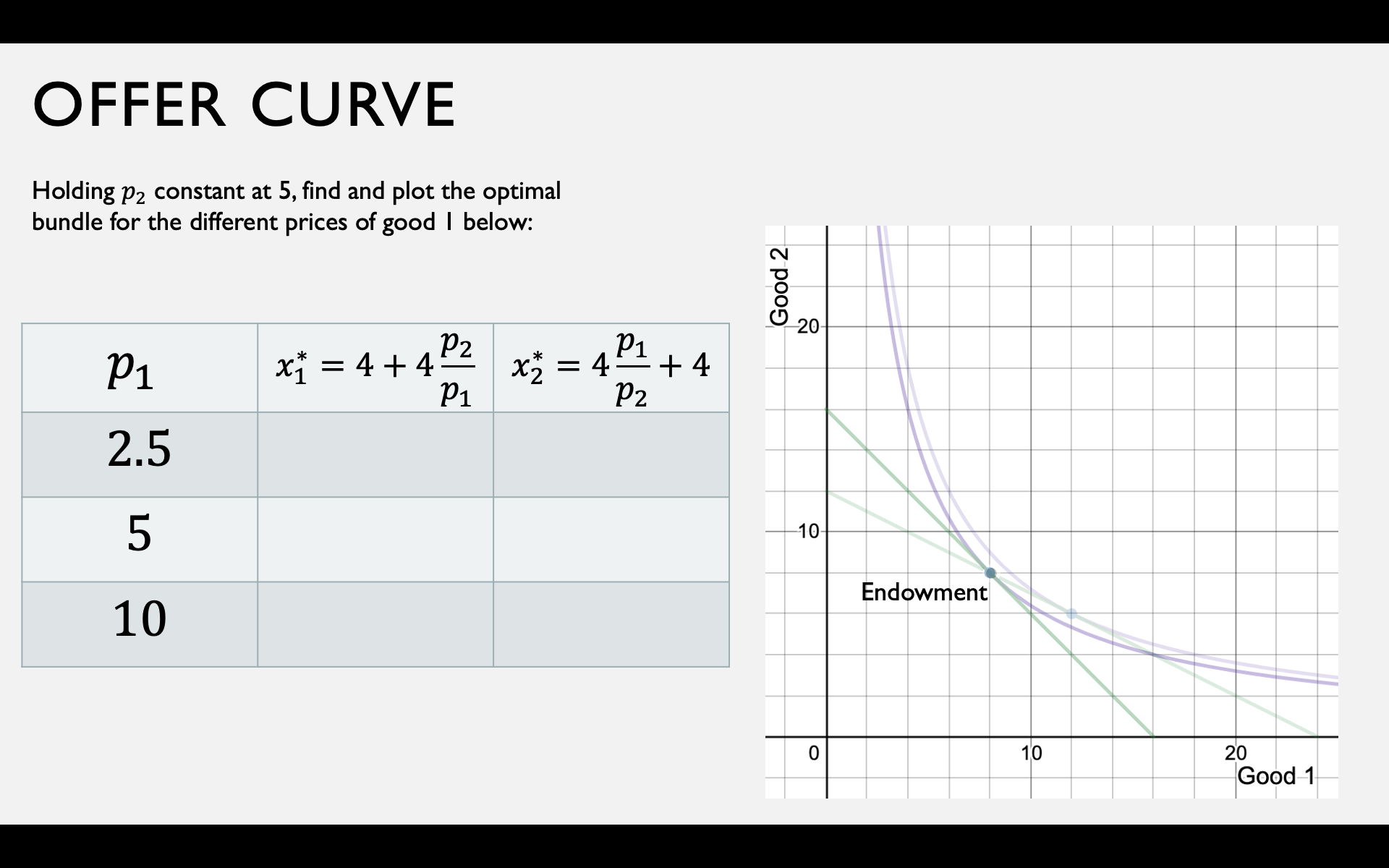
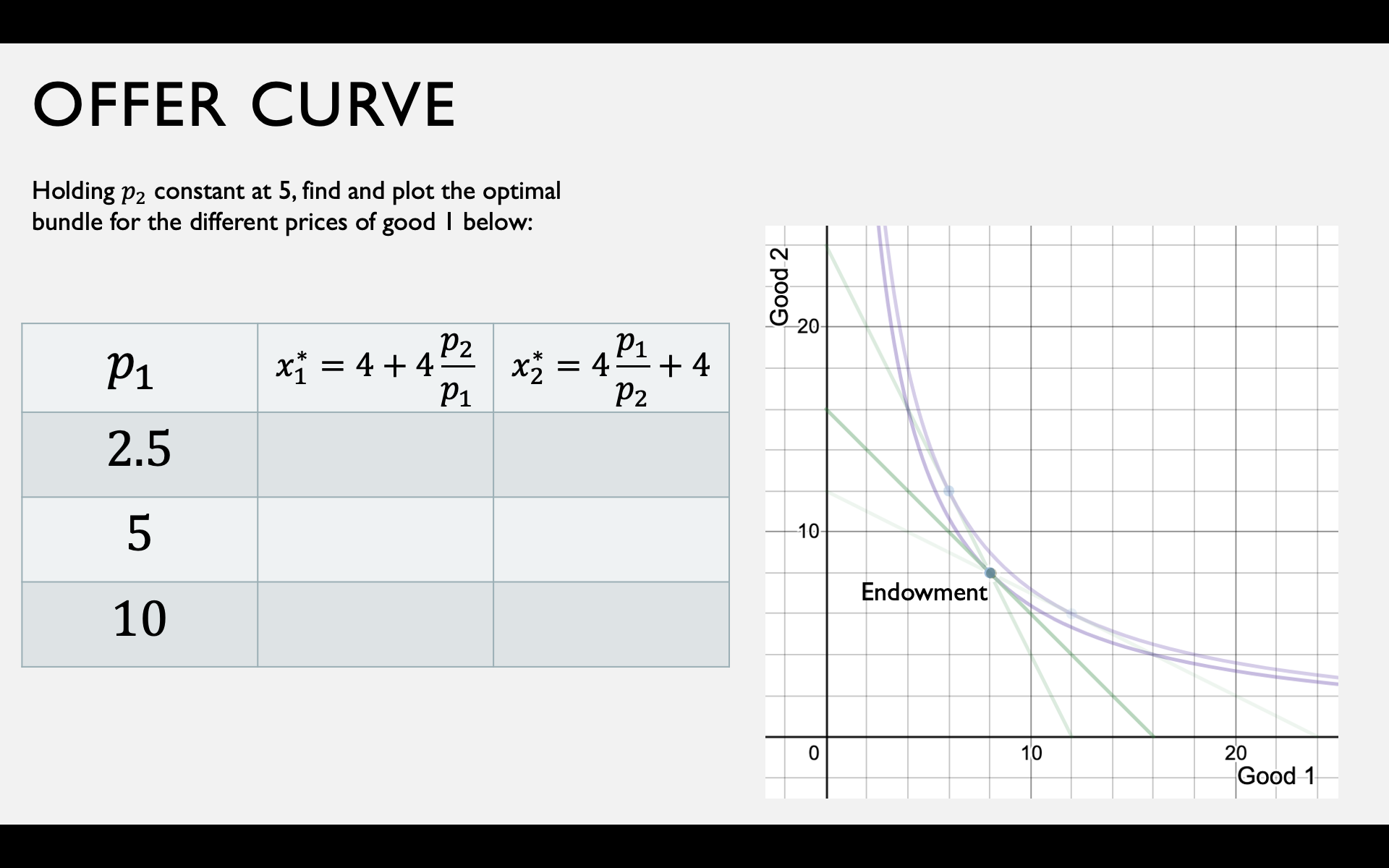
Gross Demands and Net Demands

The total quantity of a good
you want to consume (i.e. end up with)
at different prices.

Gross Demand

The transaction you want to engage in
(the amount you want to buy or sell)
at different prices.

Net Demand

x_1^*(p_1,p_2,e_1,e_2)
x_1^*(p_1,p_2,e_1,e_2) - e_1
\text{Net demand = }x_1^* - e_1

Is this positive or negative?

Positive: you are a net demander of good 1.

Negative: you are a net supplier of good 1.

d_1(p_1 | p_2) = \begin{cases} 0 & \text{ if } x_1^\star \le e_1\\x_1^\star - e_1 & \text{ if } x_1^\star \ge e_1\end{cases}
s_1(p_1 | p_2) = \begin{cases}e_1 - x_1^\star & \text{ if } x_1^\star \le e_1 \\ 0 & \text{ if } x_1^\star \ge e_1\end{cases}

Most Important Takeaways

The endowment budget line depends only on the price ratio, not on individual prices.

Whether you're a net demander or supplier depends on the relationship between the price ratio and the MRS at the endowment.

Applications

Labor supply

"Good 1" = time

"Good 2" = money

Working: selling time for money

Intertemporal Choice

"Good 1" = money in the present

"Good 2" = money in the future

Saving: selling current money to
get more money in the future

Borrowing: selling future money to get more money in the present

"Goods" =
money in different
"states of the world"

Trading: betting, investing, insurance

Uncertainty and Risk

Application 1:
Labor Supply

Leisure-Consumption Tradeoff

Leisure (R)

Consumption (C)

24
24 - L
M
M + \Delta C

You trade \(L\) hours of labor for some amount of consumption, \(\Delta C\). 

You start with 24 hours of leisure and \(M\) dollars.

L
\Delta C

You end up consuming \(R = 24 - L\) hours of leisure,
and \(C = M + \Delta C\) dollars worth of consumption.

R

Selling Labor at a Constant Wage

Leisure (R)

Consumption (C)

24
24 - L
M
M + wL

You sell \(L\) hours of labor at wage rate \(w\).

You start with 24 hours of leisure and \(M\) dollars.

You earn \(\Delta C = wL\) dollars in addition to the \(M\) you had.

L
wL
M + 24w
C = M + wL
C = M + w(24 - R)

...and you consume \(R = 24 - L\) hours of leisure.

wR + C = 24w + M

Budget Line Equation

Leisure (R)

Consumption (C)

24
M
M + 24w
wR + C = 24w + M
p_1x_1 + p_2x_2 = p_1e_1 + p_2e_2
x_1: R
x_2: C
p_1: w
p_2: 1
e_1: 24
e_2: M

This is just an endowment budget line

Optimal Supply of Labor

Preferences are over the two "good" things: leisure and consumption

u(R,C)
wR + C = 24w + M

We've just derived the budget constraint in terms of leisure and consumption as well:

Maximize utility as usual, with one caveat:
you can only sell your leisure time, not buy it.

When will labor supply be zero?

Remember: you only want to sell good 1 (in this case, your time) if

MRS(e_1,e_2)<{p_1 \over p_2}