PHYS 207.013

Chapter 8

potential energy

conservation of energy

Instructor: Dr. Bianco

TAs: Joey Betz; Lily Padlow

 

University of Delaware - Spring 2021

https://slides.com/federicabianco/phys20713_8

Energy

- kinetic energy

- work

-Potential energy -conservation of energy

H&R CH8 potential energy - conservation of energy

Instantaneous Work

H&R CH8 potential energy - conservation of energy

Instantaneous Work

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dx

[N     m]

F dx = ma ~dx = m \frac{dv}{dt} dx \\ v = \frac{dx}{dt} => dx = v ~dt\\
W_{AB} = \int_A^B F dx = \int_A^B m \frac{dv}{dt} v {dt} = \int_A^B m v ~dv
= m \int_A^B v ~dv = \frac{1}{2}m v^2 |_A^B

Instantaneous Work

H&R CH8 potential energy - conservation of energy

K = \frac{1}{2} m v^2

kinetic energy

= m \int_A^B v ~dv = \frac{1}{2} mv_B^2 - \frac{1}{2}m v_A^2
W_{AB} =
W_{AB} = \int_A^B F dx

[N     m]

Instantaneous Work

H&R CH8 potential energy - conservation of energy

K = \frac{1}{2} m v^2

kinetic energy

= m \int_A^B v ~dv = \frac{1}{2} mv_B^2 - \frac{1}{2}m v_A^2 = K_B - K_A
W_{AB} =
W_{AB} = \int_A^B F dx

[N     m]

Instantaneous Work

H&R CH8 potential energy - conservation of energy

= m \int_A^B v ~dv = \frac{1}{2} mv_B^2 - \frac{1}{2}m v_A^2 = K_B - K_A
K = \frac{1}{2} m v^2

kinetic energy

W_{AB} =
W_{AB} = \int_A^B F dx

[N     m]

W_{AB} = K_B - K_A

work-energy theorem

H&R CH8 potential energy - conservation of energy

W_{AB} = K_B - K_A

A

B

h

W_{AB} = K_B - K_A
W_{AB} = \vec{F}\cdot\vec{d}

W and K in 1D

v

H&R CH8 potential energy - conservation of energy

W_{AB} = K_B - K_A

A

B

h

W_{AB} = K_B - K_A
W_{AB} = \vec{F}\cdot\vec{d}
m\vec{g}
W_{AB} = mgh\cos{(180^o)}= -mgh

W and K in 1D

lift m from A to B

v

H&R CH8 potential energy - conservation of energy

W_{AB} = K_B - K_A

A

B

h

W_{AB} = K_B - K_A
W_{AB} = \vec{F}\cdot\vec{d}
m\vec{g}
W_{AB} = mgh\cos{(180^o)}= -mgh
W_{AB} = \vec{F}\cdot\vec{d}
\Delta K = mgh

W and K in 1D

W and K in 1D

H&R CH8 potential energy - conservation of energy

A

B

h

m\vec{g}
W_{up} = -mgh\\ W_{down} = mgh
W_{rowndtrip} = 0

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

F

F

\vec{F} = F_x \hat{i} +F_y \hat{j}+F_z \hat{k}
\vec{dr} = dx \hat{i} +dy \hat{j}+dz \hat{k}

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

F

F

\vec{F} = F_x \hat{i} +F_y \hat{j}+F_z \hat{k}
\vec{dr} = dx \hat{i} +dy \hat{j}+dz \hat{k}

At every step in the path:

 

dW = Fx \cdot dx+ Fy \cdot dy+ Fz \cdot dz

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

F

F

\vec{F} = F_x \hat{i} +F_y \hat{j}+F_z \hat{k}
\vec{dr} = dx \hat{i} +dy \hat{j}+dz \hat{k}

At every step in the path:

 

\int_A^BdW = \int_A^BF_x \cdot dx+ \int_A^BF_y \cdot dy+ \int_A^BF_z \cdot dz

each piece is the 1D prob we did before

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

F

F

\vec{F} = F_x \hat{i} +F_y \hat{j}+F_z \hat{k}
\vec{dr} = dx \hat{i} +dy \hat{j}+dz \hat{k}

At every step in the path:

 

\int_A^BdW = \frac{1}{2}(v_{Bx}^2 - v_{Ax}^2) + \frac{1}{2}(v_{By}^2 - v_{Ay}^2) + \frac{1}{2}(v_{Bz}^2 - v_{Az}^2)

W and K in 3D

H&R CH8 potential energy - conservation of energy

W_{AB} = \int_A^B F dr
d\vec{r}
d\vec{r}

F

F

\vec{F} = F_x \hat{i} +F_y \hat{j}+F_z \hat{k}
\vec{dr} = dx \hat{i} +dy \hat{j}+dz \hat{k}

At every step in the path:

 

\int_A^BdW = \frac{1}{2}(v_{Bx}^2 - v_{Ax}^2) + \frac{1}{2}(v_{By}^2 - v_{Ay}^2) + \frac{1}{2}(v_{Bz}^2 - v_{Az}^2) \\ = \frac{1}{2}mv^2_A - \frac{1}{2}mv^2_B = \Delta K

W and K in 3D

slightly more practical

H&R CH8 potential energy - conservation of energy

d\vec{r}
d\vec{r}

F

F

\vec{F} = m \vec{g} = F_y \hat{j}
\int_A^BdW = \int_A^BF \cdot dr = \int_A^BFy \cdot dy = \\ \int_A^B m g dy = -mgh

h

W and K in 3D

slightly more practical

H&R CH8 potential energy - conservation of energy

d\vec{r}
d\vec{r}

F

F

\vec{F} = m \vec{g} = F_y \hat{j}

h

only force is g: acts along y

W and K in 3D

slightly more practical

H&R CH8 potential energy - conservation of energy

d\vec{r}
d\vec{r}

F

F

\vec{F} = m \vec{g} = F_y \hat{j}
\int_A^BdW = \int_A^BF \cdot dr = \int_A^BFy \cdot dy = \\ \int_A^B m g dy = -mgh

h

But this does not depend on the crazy path I took!!

W and K in 3D

slightly more practical

H&R CH8 potential energy - conservation of energy

F

\vec{F} = m \vec{g} = F_y \hat{j}
\int_A^BdW = \int_A^BF \cdot dr = \int_A^BFy \cdot dy = \\ \int_A^B m g dy = -mgh

h

But this does not depend on the crazy path I took!!

W and K in 3D

slightly more practical

H&R CH8 potential energy - conservation of energy

F

\vec{F} = m \vec{g} = F_y \hat{j}
\int_A^BdW = \int_A^BF \cdot dr = \int_A^BFy \cdot dy = \\ \int_A^B m g dy = -mgh

h

But this does not depend on the crazy path I took!!

Instantaneous Work

H&R CH8 potential energy - conservation of energy

If the Work done by a Force does not depend on the path taken that force is said to be conservative

Conservative Forces

H&R CH8 potential energy - conservation of energy

If the Work done by a Force does not depend on the path taken that force is said to be  conservative

- e.g. Gravity is conservative

 

can you think of  a non conservative force and another non conservative force?

Conservative Forces

H&R CH8 potential energy - conservation of energy

If the Work done by a Force does not depend on the path taken that force is said to be  conservative

- e.g. Gravity is conservative

 

can you think of  a non conservative force and another non conservative force?

CONSERVATIVE: Gravity - Spring

NON-CONSERVATIVE: Friction

Conservation of Mechanical Energy

H&R CH8 potential energy - conservation of energy

A

A

B

h

m\vec{g}
W = -mgh = -mg (y_B-y_A)

lift m from A to B

W = K_B - K_A

v

Conservation of Mechanical Energy

H&R CH8 potential energy - conservation of energy

A

A

B

h

m\vec{g}
W = -mgh = -mg (y_B-y_A)

lift m from A to B

-mgy_B + mgy_A = K_B - K_A
W = K_B - K_A

Conservation of Mechanical Energy

H&R CH8 potential energy - conservation of energy

A

A

B

h

m\vec{g}
W = -mgh = -mg (y_B-y_A)

lift m from A to B

-mgy_B + mgy_A = K_B - K_A
W = K_B - K_A
K_A + mgy_A = K_B + mgy_B

Conservation of Mechanical Energy

H&R CH8 potential energy - conservation of energy

A

A

B

h

m\vec{g}

lift m from A to B

K_A + mgy_A = K_B + mgy_B

The sum of potential energy and kinetic energy remains the same

when the force is conservative

call mgy Potential Energy

use the letter U to refer to it

the 0 point of the potential gravitational energy

H&R CH8 potential energy - conservation of energy

A

A

this point is arbitrary: the only thing that matters is the distance h - just be consistent!

A

B

0

h

U = mgh

the 0 point of the potential gravitational energy

H&R CH8 potential energy - conservation of energy

A

A

this point is arbitrary: the only thing that matters is the distance h - just be consistent!

A

B

-h

0

U = mgh

the 0 point of the potential gravitational energy

H&R CH8 potential energy - conservation of energy

A

A

this point is arbitrary: the only thing that matters is the distance h - just be consistent!

A

B

h

2h

U = mgh

0

Conservation of Elastic Energy

U_{spring} = \frac{1}{2}kx^2

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

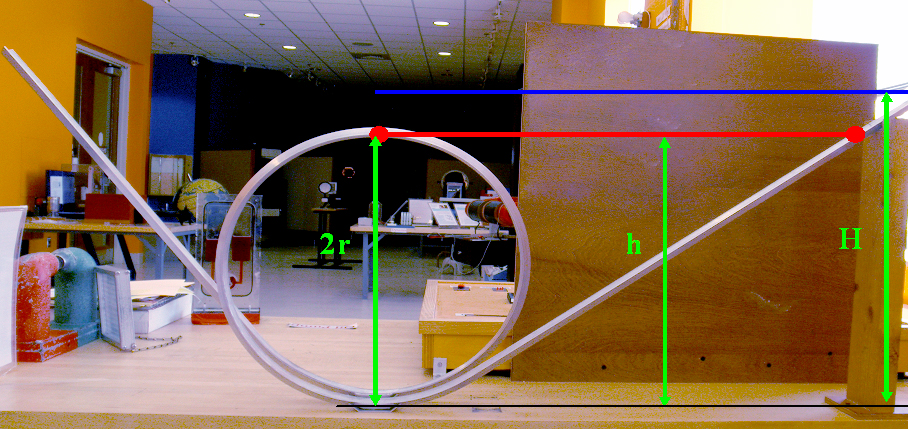
loop-the-loop problem

H&R CH8 potential energy - conservation of energy

will a ball released from the top left of this track make it around the loop?

H&R CH8 potential energy - conservation of energy

A

B

C

D

E

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

U_A + K_A = U_B+K_B=U_C+K_C =U_D+K_D =U_E+K_E

A

C

D

E

\mathrm{starting~at}~h_0:\\ mgh_0 = mgy + \frac{1}{2}mv^2

everywhere

B

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

U_A + K_A = U_B+K_B=U_C+K_C =U_D+K_D =U_E+K_E

A

B

C

D

E

mgh = mgy + \frac{1}{2}mv^2

everywhere

\frac{v^2_D}{r} = a >= g

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

U_A + K_A = U_B+K_B=U_C+K_C =U_D+K_D =U_E+K_E

A

B

C

D

E

mgh = mgy + \frac{1}{2}mv^2
\frac{v^2_D}{r} = a >= g
v^2 = 2g(h -y)

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

U_A + K_A = U_B+K_B=U_C+K_C =U_D+K_D =U_E+K_E

A

B

C

D

E

mgh = mgy + \frac{1}{2}mv^2
\frac{v^2_D}{r} = a >= g
v^2 = 2g(h -y)
v_D^2 = 2g(h -2r) >= gr

loop-the-loop problem

H&R CH8 potential energy - conservation of energy

U_A + K_A = U_B+K_B=U_C+K_C =U_D+K_D =U_E+K_E

A

B

C

D

E

mgh = mgy + \frac{1}{2}mv^2
\frac{v^2_D}{r} = a >= g
v^2 = 2g(h -y)
h >= \frac{1}{2}r + 2r = \frac{5}{2}r

pendulum problem

H&R CH8 potential energy - conservation of energy

what can you immediately say about the height a pendulum will reach on the opposite side of the initial release point?

pendulum problem

H&R CH8 potential energy - conservation of energy

what can you immediately say about the height a pendulum will reach on the opposite side of the initial release point?

how high can it go?

pendulum problem

H&R CH8 potential energy - conservation of energy

what can you immediately say about the height a pendulum will reach on the opposite side of the initial release point?

how high can it go?

why will it eventually stop?

Conservation of Energy

EM = K + U~ \mathrm{is~conserved}

Conservation of Energy

\Delta U =- \int_{x_i}^{x_f} F(x) dx
F(x) = -\frac{dU(x)}{dx}
EM = K + U~ \mathrm{is~conserved}

Conservation of Energy

slope U < 0

=> F > 0

Turning point: K = 0

U=E

particle changes direction

E_{mec} = 5J
EM = K + U~ \\ EM~\mathrm{is~conserved}

if the system is isolated and only contains conservative forces

Conservation of Energy

slope U < 0

=> F > 0

Turning point: K = 0

U=E

particle changes direction

E_{mec} = 3J
EM = K + U~ \\ EM~\mathrm{is~conserved}

if the system is isolated and only contains conservative forces

Conservation of Energy

Neutral Equilibrium: U + Emec

K = 0

dU/dx = F = 0

E_{mec} = 4J
EM = K + U~ \\ EM~\mathrm{is~conserved}

if the system is isolated and only contains conservative forces

Conservation of Energy

K(x)=0

K(d+dx)>0

K(d-dx)>0

Unstable equilibrium: U=E

K=0

 

particle would start moving if displaced

E_{mec} = 3J
K(x\pm dx) \neq 0
EM = K + U~ \\ EM~\mathrm{is~conserved}

if the system is isolated and only contains conservative forces

Conservation of Energy

U = Emec

U(x+/- dx) > Emec

Stable equilibrium:

U = E

 

particle cannot move without violating K>0

E_{mec} = 1J
U(x\pm dx) > E
EM = K + U~ \\ EM~\mathrm{is~conserved}

if the system is isolated and only contains conservative forces

Non conservative forces

\Delta E_{mec} =- f_k d\\ Fd = \Delta E_{mec} + \Delta E_{ther}

exercise: think about how the examples we saw earlier, pendulum, loop-the-loop would change if we included non-conservative forces in the problem

KEY POINTS:

  • Gravitational potential energy: 

 

  • Hooks potential energy

  • The potential energy relates to the force

  • in absence of non conservative forces, for an isolated system, the mechanical energy is conserved

U_s = kx
\Delta U =- \int_{x_i}^{x_f} F(x) dx

potential energy and

conservation of energy

E_{mec} = U + K = \mathrm{const}

H&R CH7 kinetic energy and work

U_g = mgh