We can see Temperature

• Black---Grey $\Rightarrow$ Cold to warm
• Red----Yellow $\Rightarrow$ hot
• Blue----White $\Rightarrow$ blazing hot
• "White Hot" $\Rightarrow$  ...illusion that could have altered history

Touch and say ouch!

Touch and loose finger

Two Theories Collide

• Light
  • Newton: light spectra
  • Hertz: spectra=frequency
• Heat
  • Boltzman: Temperature is smoothed out statistic of total energy, i.e. motion of particles.

Ultraviolet Catastrophe

• Boltzman + Hertz $\Rightarrow$ all colors  equally welcome....and leads to infinite energy.

• Max Planck: one color at each temperature, i.e.: energy $E$ proportional to frequency $\nu$ $$E=\hbar \nu$$ 
  • Boltzman $\exp{E/T}$ terms become Planck's $$\exp(\hbar \nu/T)$$
  • But only matches observation when $\nu$ is an integer...the first quantization.
• $\hbar\approx 6.62\times 10^{-34}$ is Planck Constant.

Could have been missed...

(Newton) White = blend of all colors

So "white hot" supports Boltzman theory of heat.

Eventually we learned human vision at extremes is grey scale (rods not cones).

...science is not inevitable

Photo-electric effect

Shine a light on metal at right frequency $\nu$, 

Electrons scatter instantly,

No "warm up", non-continuous effect.

Einstein. Argues light is discrete particles (later called "photons") having energy exactly Planck's formula $$E=\hbar \nu$$

Light is a Particle.

I can hear sound (wave) around trees

Can't see (particle) around trees.

...Newton and others

Light is a wave.

Prism spreads light by slowing its speed so frequencies split,

Just like waves in water. 

...Huygens and others

Young's 2-slit experiment. Eventually proved both are correct.

Maxwell extended to all electromagnetism

Jubobroff, CC BY-SA 3.0 , via Wikimedia Commons

Note that "observer" is an apparatuses, not a human

Heisenberg's Uncertainty .

$$\Delta (position)\cdot \Delta(momentum) \geq \frac{\hbar}{4\pi}$$

($\Delta$ standard dev. in measurement) 

the $4\pi$ is unit specific.

Particle-wave "duality" is intuitive justification

Interpretations

• Copenhagen: states are waves that collapse to a particle when measured.
• Pilot Wave: particles are surfing on waves, sometimes you watch the wave, sometimes the surfer
• Many Worlds:  all possibilities happen, but in parallel worlds
• Bayesianism: measurement merely updates our probabilities to better match new evidence.

Interpretations of Measurement

• Copenhagen: states are waves that collapse(undefined/Circular argument) to a particle when measured.
• Pilot Wave: particles are surfing on waves, sometimes you watch the wave, sometimes the surfer (incomplete/how does entanglement propogate to surfer instantly?).
• Many Worlds:  all possibilities happen, but in parallel worlds(untestable/Argumentum ad Mysteriam).
• Bayesianism: measurement merely updates our(anthropic) probabilities to better match new evidence.

Books say Dirac Notation

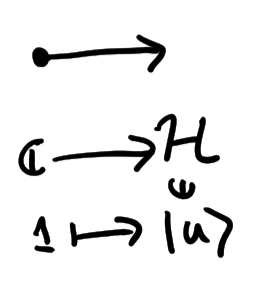
``Bra-t''$$\langle t|=\begin{bmatrix} \bar{t}_1 & \cdots & \bar{t}_n\end{bmatrix}$$

``ket-u'' $$|u\rangle = \begin{bmatrix} u_1\\ \vdots \\ u_n\end{bmatrix}$$

``bracket-t-u''

$$\langle t|u\rangle=t^{\dagger} u \in \mathbb{C}$$

Generalize to infinite dimensional Hilbert spaces $\mathcal{H}$ where $\langle t|$ becomes an integral (Riesz Representation Theorem).

$\langle t|$ vs. $|t\rangle$ all taste,

I like rows, they use typed text space better.

Reality

$$\langle +-+| = \frac{\sqrt{2}}{2} (\langle 010|+\langle 101|)$$

$$H^{\otimes 4}|0000\rangle = |x\rangle$$

$$QFT(|x\rangle) =\frac{1}{\sqrt{2^n}}\bigotimes_{i=1}^n\left(|0\rangle+e^{x_i 2^{-i+1}\pi i}|1\rangle\right)$$

Extend Dirac Notation

What is a tensor? 

$$U_R=R^X/\mathrm{Span}_R S=\mathrm{Pres}_R\langle X|S\rangle$$

$${_R V}=R^Y/\mathrm{Span}_R T=\mathrm{Pres}_R\langle Y|T\rangle$$

$$U\otimes_R V = R^{X\times Y}/(R^X\otimes T+S\otimes R^Y)$$

$$\begin{aligned} \otimes  :R^X\times & R^Y\to R^{X\times Y}\\ (u\otimes v)_{xy}& =u_x v_y\end{aligned}$$

I.e.$$u\otimes v=\begin{bmatrix}u_1\\ \vdots \\ u_{m}\end{bmatrix}\begin{bmatrix} v_1 & \cdots & v_n\end{bmatrix} = \begin{bmatrix} u_1 v_1 & \cdots & u_1 v_n\\ \vdots & & \vdots \\ u_m v_1 & \cdots & u_m v_n\end{bmatrix}$$

• Linear form $\langle t|:U_1\to \mathbb{C}$ so evaluate $|u_1\rangle$ as $$\langle t|u_1\rangle$$
• Bi-linear form $\langle t|:U_2\to (U_1\to \mathbb{C})$ so evaluate $|u_2\rangle$ as $$\langle t|u_2\rangle:U_1\to \mathbb{C}$$ then on $|u_1\rangle$ to get $$\langle t|u_2,u_1\rangle=\langle t|u_2\rangle|u_1\rangle\in \mathbb{C}$$
• Tri-linear...$$\langle t|:U_3\times U_2\times U_1\to \mathbb{C}$$
• $\ldots$

How to imagine tensors with high valence?

Look at data!

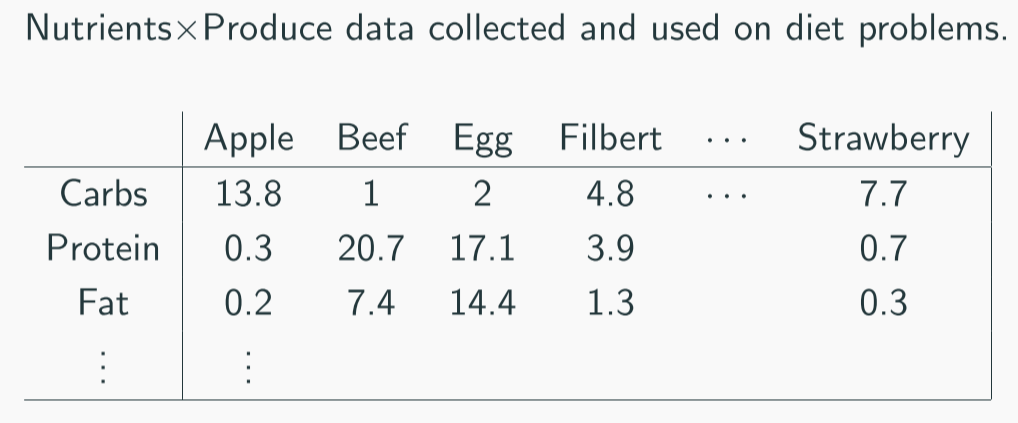
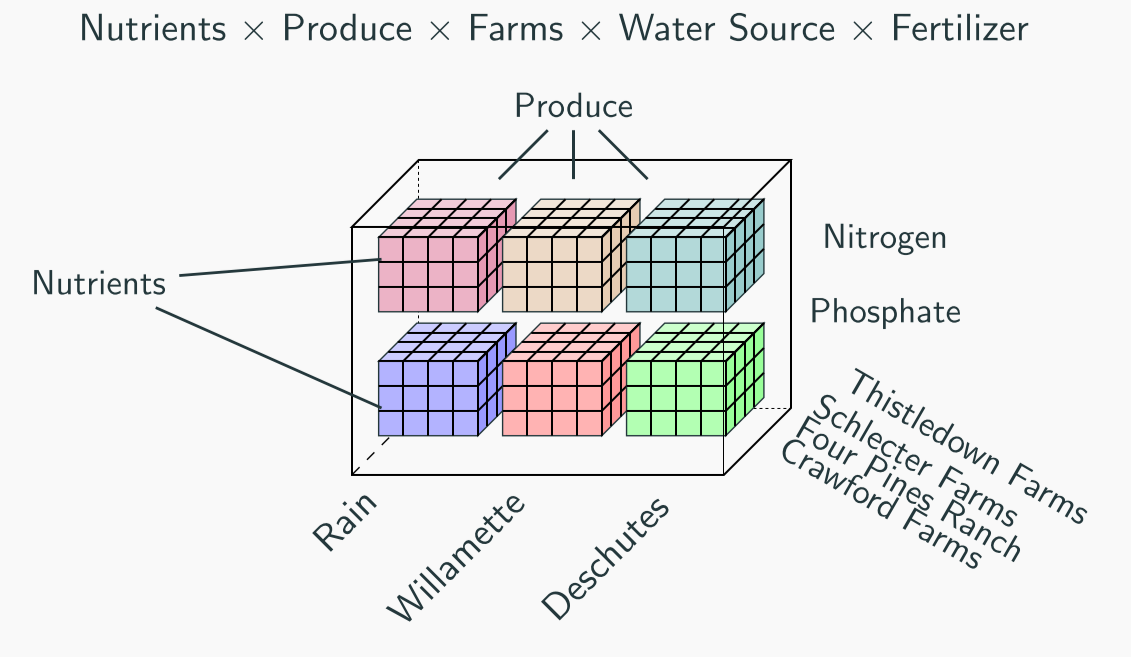
How to imagine tensors with high valence?

Look at data!

5-valent tensor

Tensor Networks

(Operads)

Make a vector...

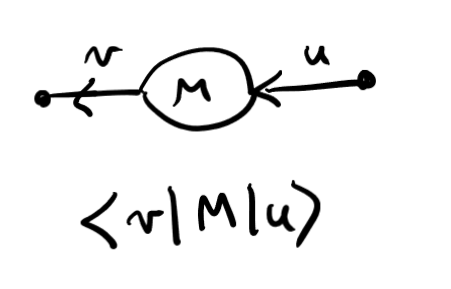
Make a 2-tensor...

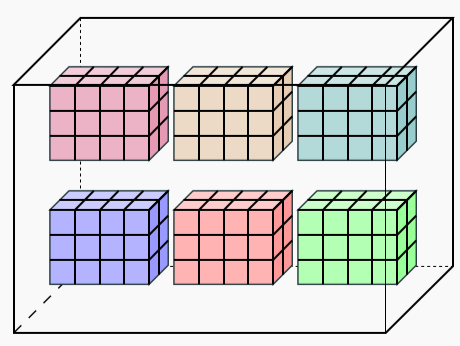
$$\Gamma_{abcde}\in \mathbb{C}$$

Extend Dirac Notation

Defn.  A tensor space $T$ is a vector space (module) with a linear map $$\langle \cdot |:T\to (V_1\otimes\cdots \otimes V_{\ell})^*$$ $t\in T$ is a tensor, $V_a$ axes (or modes, or legs), and $V_1,\ldots,V_{\ell}$ the (reference) frame.

$\langle \cdot|$ interprets $t\in T$ as multi-linear.

What is a tensor? 

....element of a tensor space.

$\langle t|$ interprets $t$ as multi-linear

Let $A=\mathbb{C}^{2}=\mathbb{C}\langle 0|\oplus \mathbb{C}\langle 1|$ and $B=\mathbb{C}^{3}=\mathbb{C}\langle 0|\oplus \mathbb{C}\langle 1|\oplus \mathbb{C}\langle 2|$.

Let $$\langle ab|:=\langle a|\langle b|=\langle a|\otimes \langle b|\in (A\otimes B)^*$$ 

$\langle t|$ interprets $t$ as multi-linear

$\langle 0|,\ldots\langle m|$ usually just a basis, but common to write $$x=x_n 2^n+\cdots+x_0 2^0\qquad x_i\in \{0,1\}$$ and so $$\langle x|=\langle x_n| \cdots \langle x_0|\in (\mathbb{C}^2)^{\otimes n}$$ hence integer data written in binary "bits", only quantum so "qu-bits"

$\langle t|$ interprets $t$ as multi-linear

QUIZ

Explain $$\langle \uparrow\uparrow|-i\langle \downarrow\downarrow|$$

• Need a vector space with basis $\{\uparrow,\downarrow\}$ so $\mathbb{C}^2$ will do.
• Need two copies, so $\mathbb{C}^2\otimes \mathbb{C}^2$, i.e. $(2\times 2)$ complex matrices.
• $$\langle \uparrow\uparrow|-i\langle\downarrow \downarrow|=\begin{bmatrix}1 & 0 \\ 0 & 0 \end{bmatrix}-i\begin{bmatrix}0 & 0 \\ 0 & 1\end{bmatrix}=\begin{bmatrix} 1 & 0 \\ 0 & -i\end{bmatrix}$$

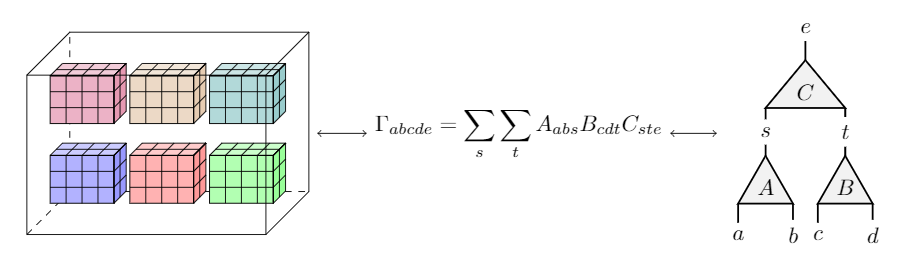
Tensor is a grid of numbers.

Numbers can be results of formulas ("tensor contraction")

Structure of formulas $\Leftrightarrow$ Structure of Entanglement of many bodies

Question I

• Find tensor network decompositions

• Explain relations between equivalent decompositions

• Classify, at least on small scales.

Math is graph theory & algebra & topology.

Quantum materials

• Classical Chemistry:
  • Given atoms,
  • find & bend the rules for bonding.
  • Combinatorics: matching problems: $H$ wants an electron, $OH$ has more than it wants$\Rightarrow$ $H_2 )$)
  • Topology: molecule shape prevents easy bonding
  • Algebra: crystal symmetry, degrees of freedom forced by representation theory.

Quantum materials

• Classical Chemistry:
  • Given atoms,
  • find & bend the rules for bonding.
  • combinatorics, topology, algebra
• Quantum Materials:
  • Given particles
  • find & bend the rules for entanglement.
  • Algebraic
    • Isomorphism invariants (ranks, SVD, eigen, derivations, adjoints...)
    • Gauge symmetries
  • ​Combinatorial: networks
  • Perturbative, Annealing, Neural Nets,...

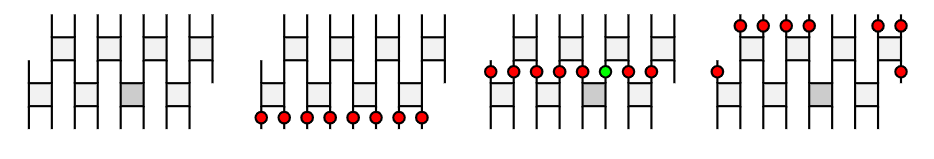
Tensor network of quantum entanglements in a quantum material.

Material interacts with environment on boundary (red dots).

The boundary (edge) physics is about the symmetries of individuals.

Question 2

• Explain the boundary physics of quantum materials ("edge theory")

Math is isomorphism testing of tensors and graphs.

Notice the Noise (Green).  It somehow only locally obstructed symmetry.

Graphical properties of the network explain how to adjust edge theories under noise.

Question 2'

• Explain the boundary physics of quantum materials ("edge theory") under noise

Very strange that something as precise as graph theory, not analysis, handles noise model....

Recent "breakthroughs"

• Factoring 
• Ramsey Numbers
• Quantum Supremacy Advantage
• Time Crystal

Factoring

• Biggest RSA type factorization: 830-bits $2^{830}$, RSA of today is 2040-bits so about $2^{1200}$ TIMES HARDER
• Biggest Quantum factorization
  • NONE!
  • But kinda 15, 21
  • Needs a lot of qubits more than we have in the world to run fault-tolerant error-corrected
  • DWAVE is adiabatic, not circuit based, better for modeling quantum behavior not quantum computing 

Ramsey Number

• Adiabatic solution reproduced signals "near" expected values of known Ramsey numbers, and signals for others unknown....but no way to test.

Quantum Advantage

• Context:
  • Google buys UC Santa Barbara quantum computing lab.
• Newly minted Google Q Team invents a problem: "Can Quantum Computer generate probabilities of random quantum operations faster than a classical computer?"​
• Asked and answered: yes.  Gets a lot of press.
• Reaction within CS...
  • is this a well-posed question?
  • assume a classical computer has to analyze a quantum computer, not a head-to-head fight

Time Crystals

• Context:
  • Einstein: time-space unified.
  • Wilczek: crystals are symmetries in space...maybe there are symmetries in time?
• Synthesized quantum states in Google's quantum computer seem to have achieved this.
• Synthesized quantum experiments are what Feynman wanted quantum computer to do...it seems they might do that!

Reality

• Eventually quantum computers threaten security, so change the locks now
• Quantum computing has changed how we model computation --- much more attention to low-level efficiency useful in everyday computing
• Making quantum materials will let us make quantum who knows what.

Quantum Hype is...

... a demonstration of pure scientific progress,

less so engineering.