
Tensor networks for frustrated Ising models:
partial lifting of a macroscopic ground-state degeneracy
JC, B. Vanhecke et al., arXiv:2206.11788 (2022)
B. Vanhecke, JC, et al., Phys. Rev. Research 3 (2021)

Jeanne Colbois | Laboratoire de Physique Théorique, Université de Toulouse CNRS/UPS | France
IISER Pune - Condensed Matter, Statistical, AMO and Nonlinear Physics Events - Online - 29.09.2022

Andrew Smerald
KIT | Germany
Frank Verstraete
Ghent University | Belgium
Laurens Vanderstraeten
Ghent University | Belgium
Bram Vanhecke
University of Vienna | Austria
Frédéric Mila
EPFL | Switzerland






Frustration and motivation: artificial spin systems
A model with several macroscopically degenerate phases
Tensor networks for frustrated Ising models
Proving the ground-state energy?
> Motivation
> Introduction to tensor networks for classical spin systems
> A problem of contraction
Scope
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
1
J. Colbois

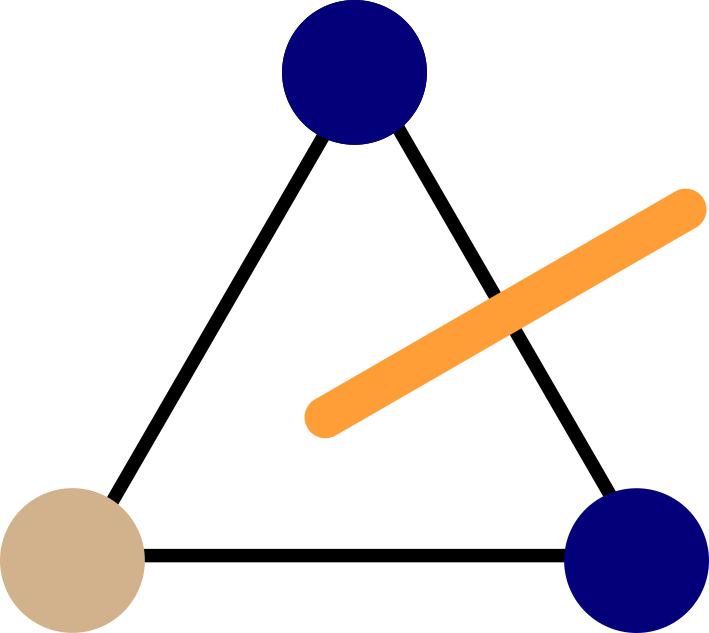
2-up 1-down (UUD),
2-down 1-up (DDU)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
2
J. Colbois
Frustration




2-up 1-down (UUD),
2-down 1-up (DDU)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
2
J. Colbois
Frustration


G.H. Wannier, PR 79, (1950, 1973)
K. Kano and S. Naya, Prog. Theor. Phys. 10, (1953)


2-up 1-down (UUD),
2-down 1-up (DDU)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
2
J. Colbois
Frustration
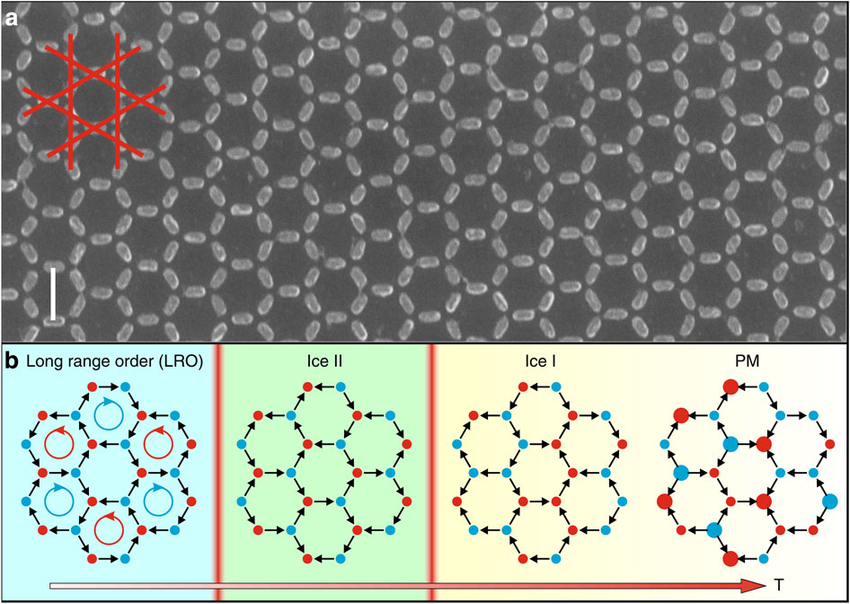
Anghinolfi et al.,
Nat. Commun. 6, (2015)
In-plane
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
3

Artificial spin systems
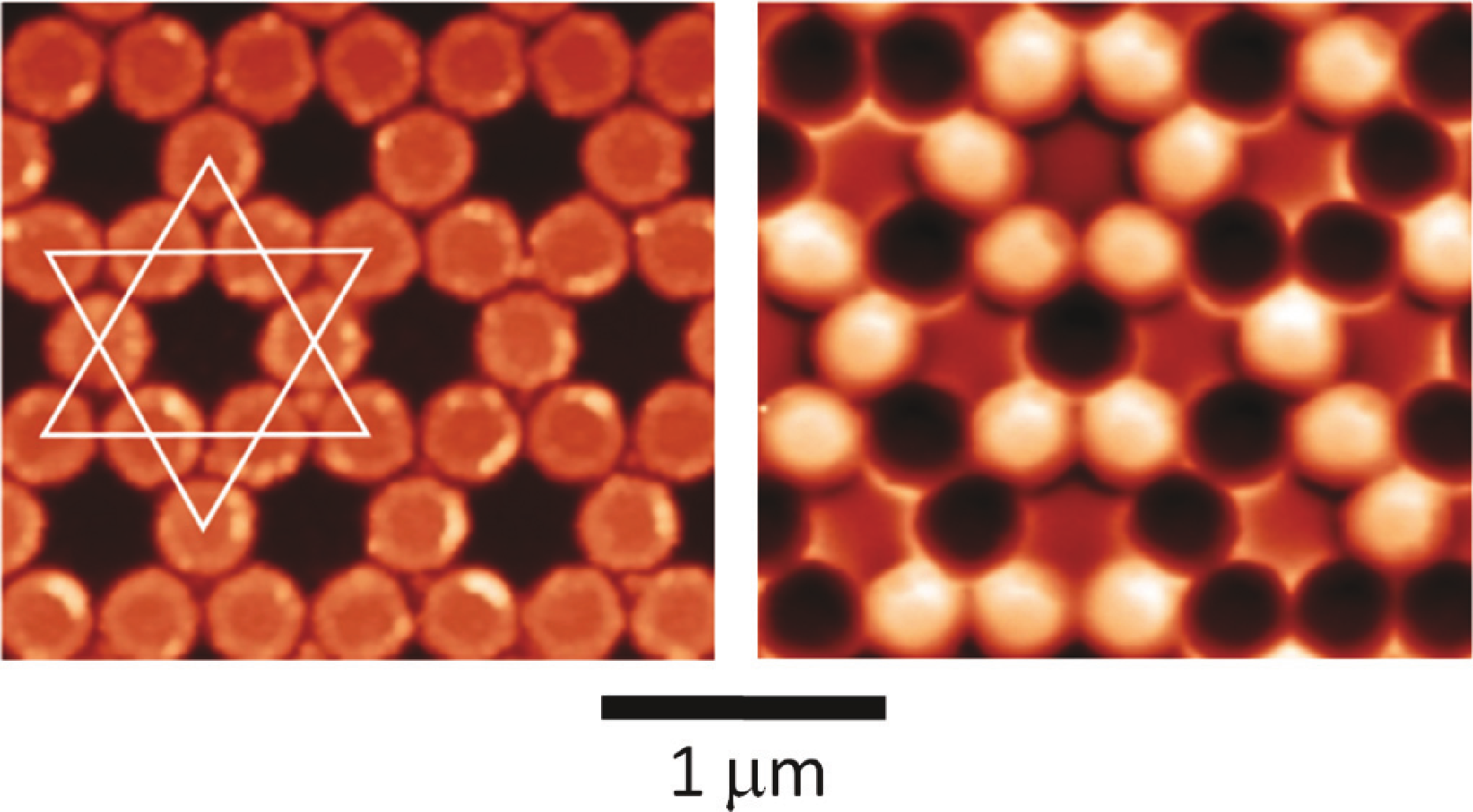
Chioar et al., PRB 90, (2014)
TbCo
Out-of-plane


Anghinolfi et al.,
Nat. Commun. 6, (2015)

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
3
In-plane
Artificial spin systems
I. A. Chioar, N. Rougemaille, B. Canals, PRB 93, (2016)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
L. Cugliandolo, L. Foini, M. Tarzia, PRB 101 (2020)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
4

Chioar et al., PRB 90, (2014)


Dipolar kagome Ising antiferromagnet (DKIAFM)
I. A. Chioar, N. Rougemaille, B. Canals, PRB 93, (2016)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
L. Cugliandolo, L. Foini, M. Tarzia, PRB 101 (2020)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
4

Chioar et al., PRB 90, (2014)



Dipolar kagome Ising antiferromagnet (DKIAFM)
I. A. Chioar, N. Rougemaille, B. Canals, PRB 93, (2016)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
L. Cugliandolo, L. Foini, M. Tarzia, PRB 101 (2020)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
4

Chioar et al., PRB 90, (2014)




Dipolar kagome Ising antiferromagnet (DKIAFM)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
4

Chioar et al., PRB 90, (2014)



Dipolar kagome Ising antiferromagnet (DKIAFM)

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
4

Chioar et al., PRB 90, (2014)



How does the degeneracy get lifted?
Dipolar kagome Ising antiferromagnet (DKIAFM)


Chioar et al., PRB 90, (2014)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
5
Short-range model and questions

Chioar et al., PRB 90, (2014)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
5
Short-range model and questions
(How) Does the degeneracy get lifted?

Chioar et al., PRB 90, (2014)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
5
Short-range model and questions
(How) Does the degeneracy get lifted?
Proving the ground-state energy?
Evaluating the residual entropy precisely?
(How) Does the degeneracy get lifted?
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
6
Expectations (1)
T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.

T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (1)
(How) Does the degeneracy get lifted?
6
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.


T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
JC, K. Hofhuis, et al., PRB 104, (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
7
Expectations (1) vs Reality

(How) Does the degeneracy get lifted?
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.


T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
JC, K. Hofhuis, et al., PRB 104, (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
7
Expectations (1) vs Reality

(How) Does the degeneracy get lifted?
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.


T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
JC, K. Hofhuis, et al., PRB 104, (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
7
Expectations (1) vs Reality

(How) Does the degeneracy get lifted?
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.


T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
JC, K. Hofhuis, et al., PRB 104, (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
7
Expectations (1) vs Reality

(How) Does the degeneracy get lifted?
The macroscopic g.s. degeneracy gets completely lifted by second-neighbor interactions.


T. Takagi and M. Mekata, JSPS 62, (1993)
A. Wills, R. Ballou, C. Lacroix, PRB 66, (2002)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
JC, K. Hofhuis, et al., PRB 104, (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
7
Expectations (1) vs Reality

(How) Does the degeneracy get lifted?
The macroscopic degeneracy can survive in the presence of third-neighbor interactions but only at very fine-tuned points.
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
8
Expectations (2)
(How) Does the degeneracy get lifted?
The macroscopic degeneracy can survive in the presence of third-neighbor interactions but only at very fine-tuned points.
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (2)
(How) Does the degeneracy get lifted?

8

The macroscopic degeneracy can survive in the presence of third-neighbor interactions but only at very fine-tuned points.

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (2)
(How) Does the degeneracy get lifted?
This talk: a series of macroscopically degenerate phases
8
Ising model = easy to solve?
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (3)
9
Ising model = easy to solve?
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (3)
9
- The long-range model is challenging (~300 spins) (glassy behavior)

I. A. Chioar, N. Rougemaille, B. Canals, PRB 93, (2016)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
L. Cugliandolo, L. Foini, M. Tarzia, PRB 101 (2020)
Ising model = easy to solve?
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Expectations (3)
9
- The long-range model is challenging (~300 spins) (glassy behavior)
I. A. Chioar, N. Rougemaille, B. Canals, PRB 93, (2016)
J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)
L. Cugliandolo, L. Foini, M. Tarzia, PRB 101 (2020)


J. Hamp, C. Castelnovo, R. Moessner, PRB 98, (2018)

- Short-range model :
- MC falls out-of-equilibrium
- Negative results from simple Pauling estimates
Proving the ground-state energy
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Frustration and motivation: artificial spin systems
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Kanamori's method
10
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Constrain the possible values of the correlations:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Kanamori's method
10
Constrain the possible values of the correlations:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Kanamori's method
10
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)

Translations + rotations
to cover the lattice
Constrain the possible values of the correlations:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Kanamori's method
10
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)


J. Colbois
Kanamori's method : applied to our problem
11
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)



J. Colbois
Kanamori's method : applied to our problem
11
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)



Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Kanamori's method : applied to our problem
11
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.



Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Kanamori's method : applied to our problem
11
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.



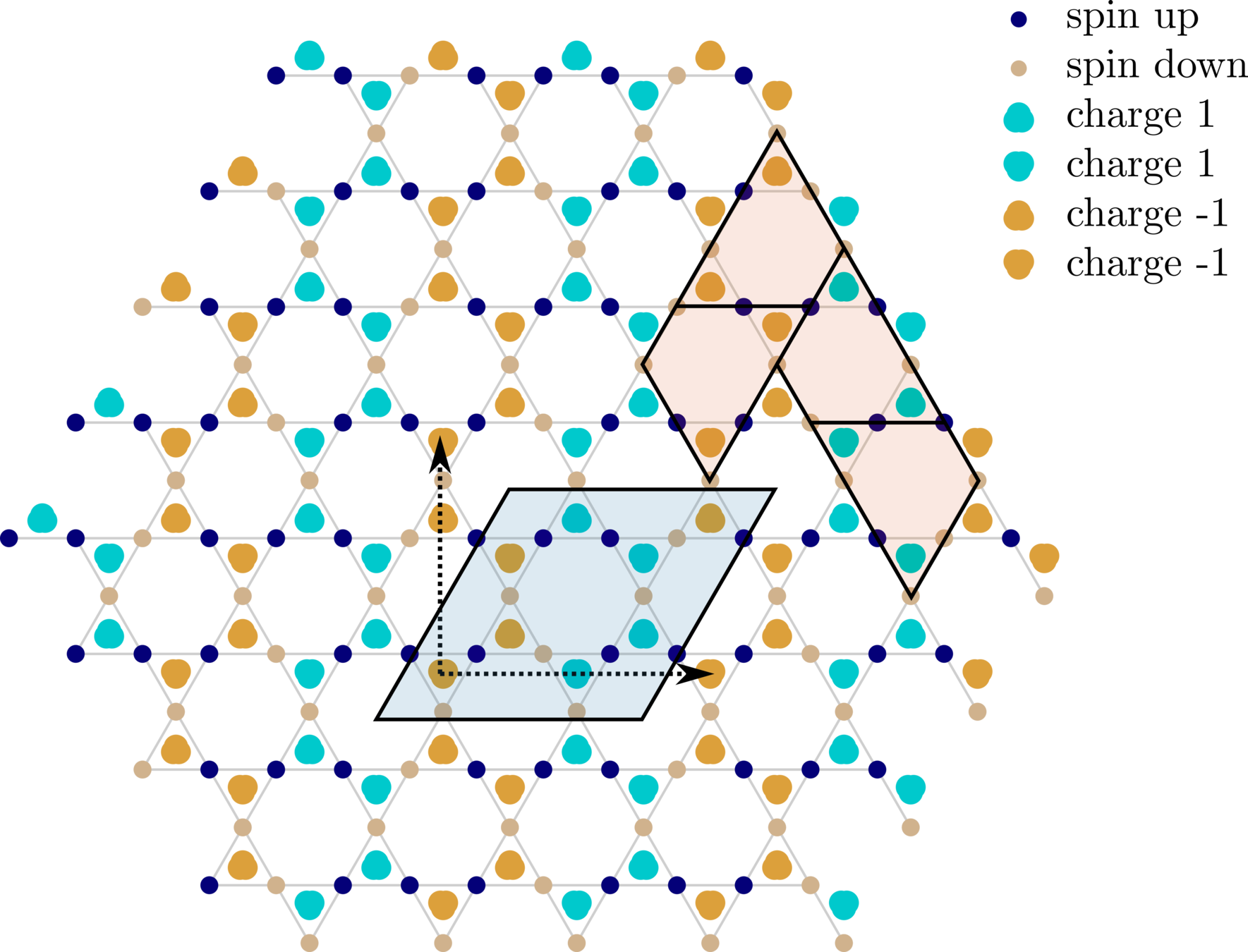
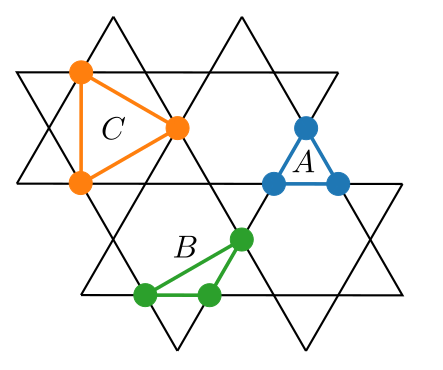
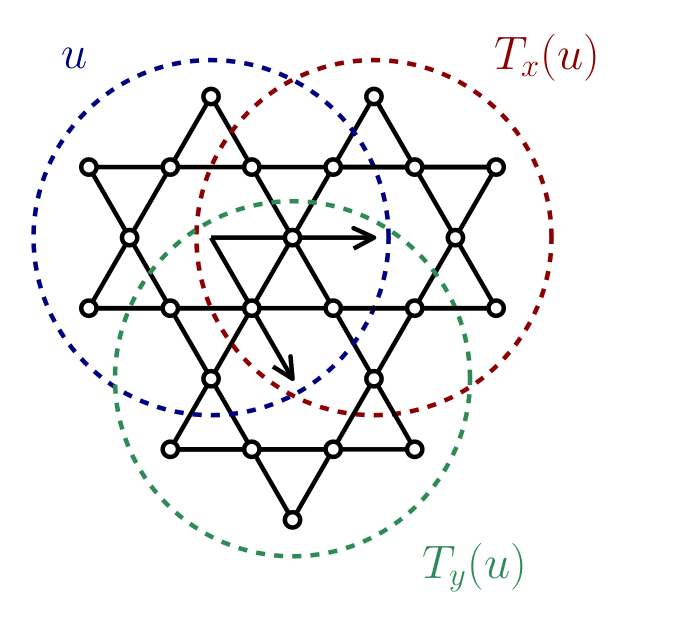
Not all triangles can respect the UUD/DDU rule at the same time
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Kanamori's method : applied to our problem
11
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Corners directly correspond to G.S. phases:

Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Obtaining ground-state energy lower bounds
12
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.

Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Corners directly correspond to G.S. phases:
J. Colbois
12
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Obtaining ground-state energy lower bounds

Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Corners directly correspond to G.S. phases:
J. Colbois
12
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Obtaining ground-state energy lower bounds
Corners directly correspond to G.S. phases:

Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Edges correspond to g.s. phase boundaries
J. Colbois
12
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Obtaining ground-state energy lower bounds
All the corners must be verified
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Proving the ground-state energy
13
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.



All the corners must be verified
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Proving the ground-state energy
13
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.



All the corners must be verified
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Proving the ground-state energy
13
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.



All the corners must be verified
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Proving the ground-state energy
13
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.




All the corners must be verified
Methods: J. Kanamori, Prog. Theor. Phys. 35, (1966)
F. Ducastelle, Cohesion and Structure No 3, (1990)
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
J. Colbois
Proving the ground-state energy
13
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Tensor networks for frustrated Ising models
Proving the ground-state energy
Frustration and motivation: artificial spin systems
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
> Motivation
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
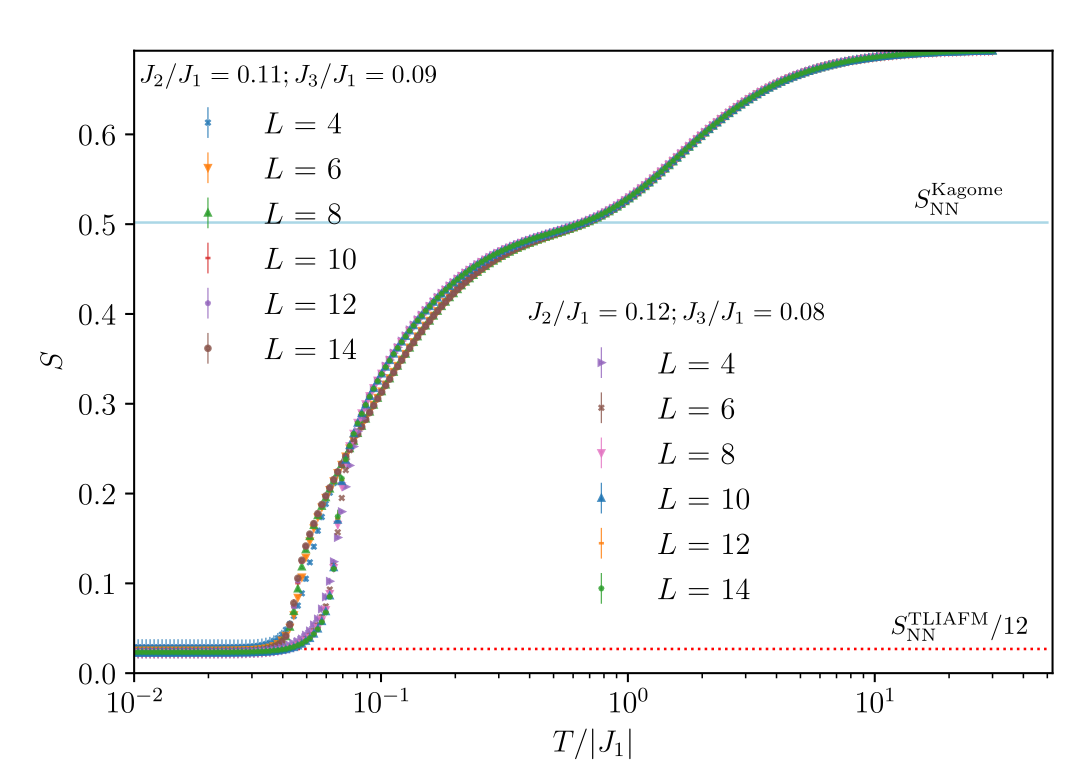
Motivation (1) : why not Monte Carlo?
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
Motivation (1) : why not Monte Carlo?
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
Motivation (1) : why not Monte Carlo?
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
Motivation (1) : why not Monte Carlo?
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
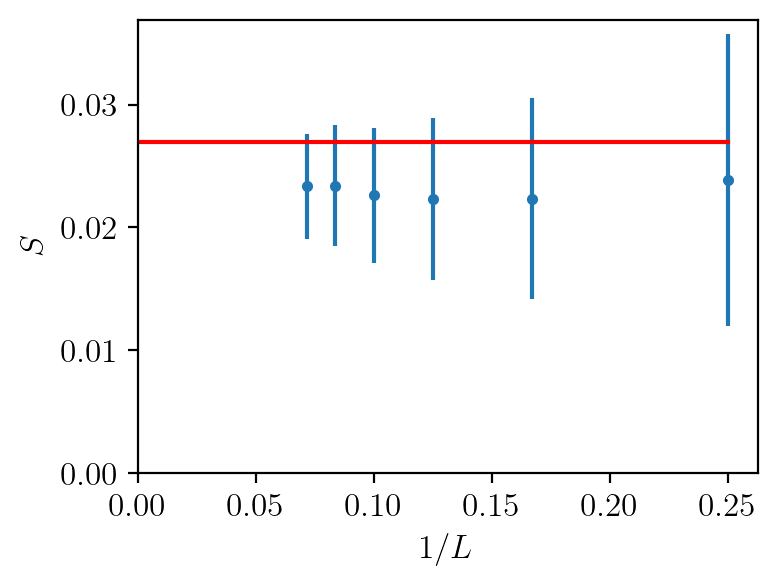
Motivation (1) : why not Monte Carlo?
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
Motivation (1) : why not Monte Carlo?
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Monte Carlo challenges:
- "Good" update
- Thermodynamic integration
- Good control over the finite-size scaling behavior

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
15
Motivation (1) : why not Monte Carlo?
G. Rakala, K. Damle, PRE 96, (2017)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
16
Motivation (2) : why tensor networks?



TNs are very powerful for 2D classical problems with short-range interactions
They give a direct access to the partition function "per site".
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
17
Motivation (3) : idea
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
17
Motivation (3) : idea
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
17
Motivation (3) : idea
We "just" have to compute the (normalized) partition function for one site...
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
17
Motivation (3) : idea
We "just" have to compute the (normalized) partition function for one site...
... which is the result that naturally comes out with a large precision from tensor networks.
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
17
Motivation (3) : idea
Tensor networks for frustrated Ising models
Proving the ground-state energy
Frustration and motivation: artificial spin systems
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
> Motivation
> Introduction to tensor networks for classical spin systems
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices

Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices

Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices


Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices


Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
Tensor network language:

Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices

"Tensor"
"Legs"
"Contraction"

Tensor network language:

"vector"

Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices

"Legs"
"Contraction"
Open legs = number of indices

Tensor network language:

"vector"
"Tensor"

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
18
Tensor networks : inspired by transfer matrices

"Tensor"
"Legs"
"Contraction"
Open legs = number of indices


"vector"

Tensor network language:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
19
Partition function of a 2D problem as a TN

R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
19
Partition function of a 2D problem as a TN


R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
19
Partition function of a 2D problem as a TN


R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
19
Partition function of a 2D problem as a TN
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996

Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
20
Contraction and leading eigenvector
"Exact contraction"
"Approximate contraction"
Tensor notation: R. Penrose, in Combinatorial Mathematics and its applications, Academic Press (1971)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
20
Contraction and leading eigenvector
"Exact contraction"
"Approximate contraction"

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
Contraction and leading eigenvector (2D)

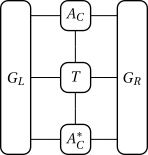
Row to row transfer matrix -> "Matrix product operator"
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
The Matrix Product State Ansatz
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
The Matrix Product State Ansatz
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996

High number of parameters
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
The Matrix Product State Ansatz
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996


High number of parameters
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
The Matrix Product State Ansatz
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996


High number of parameters
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
21
The Matrix Product State Ansatz
R. J. Baxter, J. Math. Phys. 9, 1968
R. Orús, G. Vidal, PRB 78, 2008
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996

High number of parameters

Much smaller number of parameters
(1 + 1)D
iTEBD / VUMPS
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D
2D
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D

2D

(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D

2D

(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D


R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
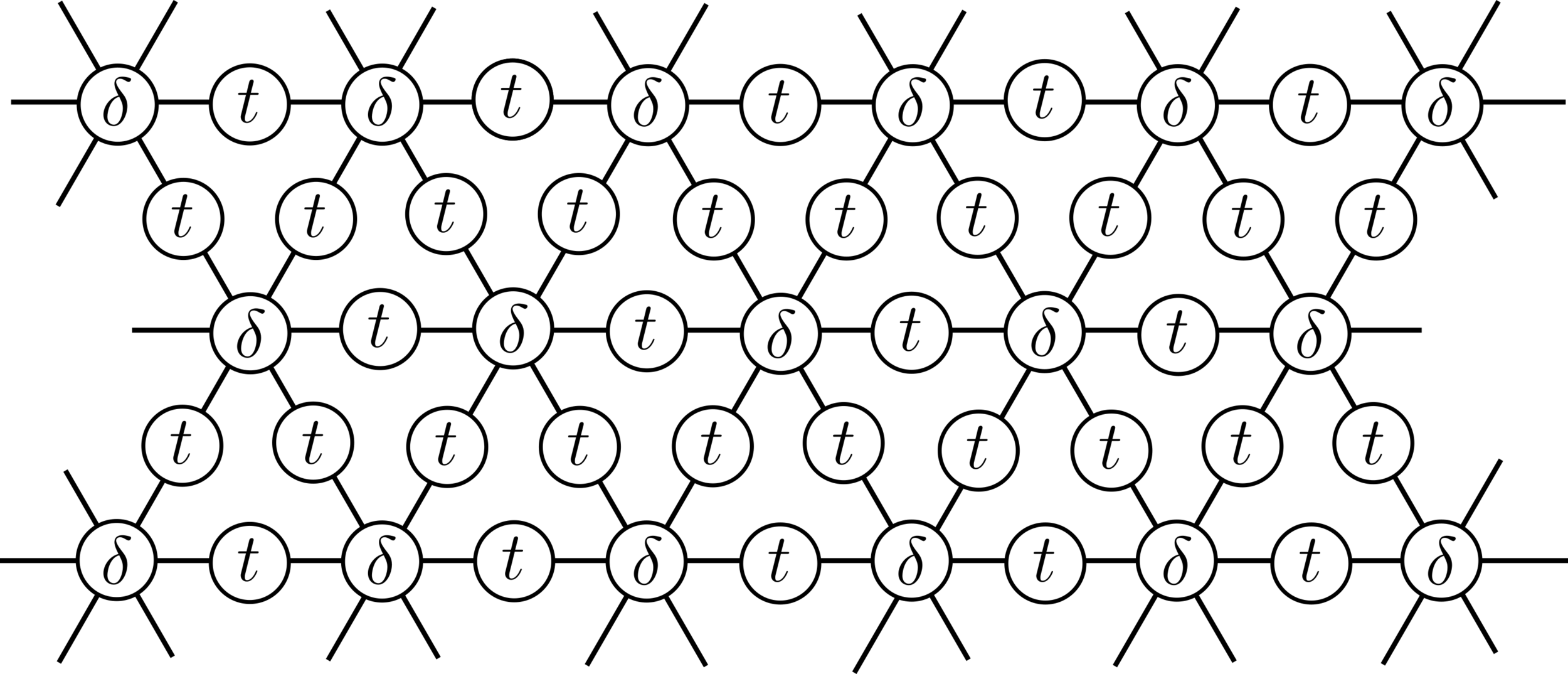
Environment
Iteratively solving fixed-point equations
T tensor can be replaced by observables
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
Environment
Iteratively solving fixed-point equations
T tensor can be replaced by observables
(1 + 1)D
iTEBD / VUMPS
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
22
(1+1)D versus 2D


2D



R. J. Baxter, J. Math. Phys. 9, 1968
T. Nishino, K. Okunishi, J. Phys. Soc. Jpn 65, 1996
Frustration and motivation: artificial spin systems
Tensor networks for frustrated Ising models
Proving the ground-state energy?
> Motivation
> Introduction to tensor networks for classical spin systems
> A problem of contraction
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
The issue
23

C. Wang, S.-M. Qin, H.-J. Zhou, PRB 90, (2014)
Z. Zhu, H. G. Katzgraber, arXiv:1903.07721 (2019)
B. Vanhecke, JC, et al. PRR 3, (2021)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
M. Friaz-Pérez, M, Mariën et al, arXiv:2104.13264, (2021)
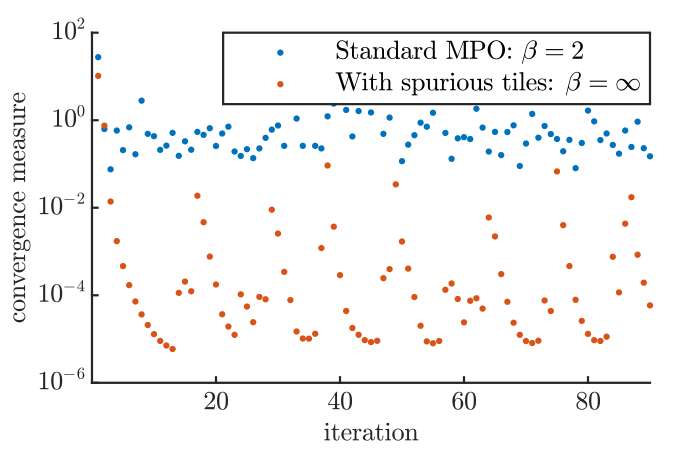
Fails in the presence of frustration and macroscopic g.s. degeneracy
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
The issue
23

C. Wang, S.-M. Qin, H.-J. Zhou, PRB 90, (2014)
Z. Zhu, H. G. Katzgraber, arXiv:1903.07721 (2019)
B. Vanhecke, JC, et al. PRR 3, (2021)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
M. Friaz-Pérez, M, Mariën et al, arXiv:2104.13264, (2021)
Fails in the presence of frustration and macroscopic g.s. degeneracy

C. Wang, S.-M. Qin, H.-J. Zhou, PRB 90, (2014)
Z. Zhu, H. G. Katzgraber, arXiv:1903.07721 (2019)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
Related to the inexact cancellation of very large
and very small factors.
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
The issue
23
Non-frustrated models:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
24
What is going on?
Non-frustrated models:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
What is going on?
24
Non-frustrated models:
Triangular/ kagome lattices:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
What is going on?
24
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Why is it intriguing?
J. Colbois
25



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Why is it intriguing?
J. Colbois
25



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Why is it intriguing?
J. Colbois


25



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Why is it intriguing?
J. Colbois


The convergence of the tensor network contraction depends on the formulation of the partition function
25
C. Wang, S.-M. Qin, H.-J. Zhou, PRB 90, (2014)
Z. Zhu, H. G. Katzgraber, arXiv:1903.07721 (2019)
B. Vanhecke, JC, et al. PRR 3, (2021)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
M. Friaz-Pérez, M, Mariën et al, arXiv:2104.13264, (2021)
B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
C. K. Majumdar and D. K. Ghosh, J. Math. Phys. 10, (1969);
M. Kaburagi, J. Kanamori, Prog. Theor. Phys. 54 , (1975);
B. Sriram Shastry and B. Sutherland, Physica 108 B+C, (1981);
W. Huang, D. A. Kitchaev, et. al. , Phys. Rev. B 94, (2016)
J. Colbois

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
26
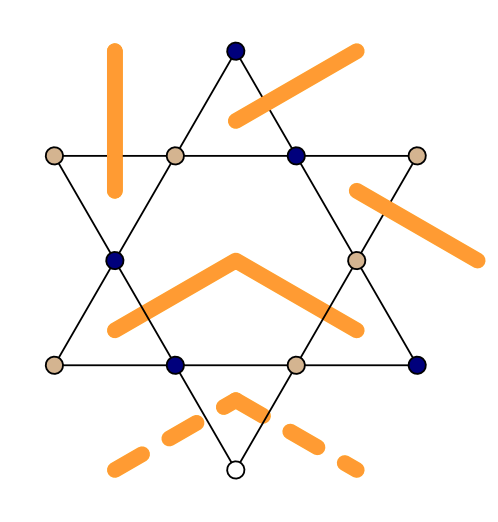
Systematically finding the local rule
1. Split the Hamiltonian in a different way
B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
C. K. Majumdar and D. K. Ghosh, J. Math. Phys. 10, (1969);
M. Kaburagi, J. Kanamori, Prog. Theor. Phys. 54 , (1975);
B. Sriram Shastry and B. Sutherland, Physica 108 B+C, (1981);
W. Huang, D. A. Kitchaev, et. al. , Phys. Rev. B 94, (2016)
J. Colbois


1. Split the Hamiltonian in a different way
2. Lower bound on the ground-state energy:
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Systematically finding the local rule
26
B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
C. K. Majumdar and D. K. Ghosh, J. Math. Phys. 10, (1969);
M. Kaburagi, J. Kanamori, Prog. Theor. Phys. 54 , (1975);
B. Sriram Shastry and B. Sutherland, Physica 108 B+C, (1981);
W. Huang, D. A. Kitchaev, et. al. , Phys. Rev. B 94, (2016)
J. Colbois



1. Split the Hamiltonian in a different way
2. Lower bound on the ground-state energy:
3. This lower bound can be maximized with respect to the weights (max-min approach):
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Systematically finding the local rule
26
B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
J. Colbois

1. Split the Hamiltonian in a different way
2. Lower bound on the ground-state energy:
3. This lower bound can be maximized with respect to the weights (max-min approach):
4. When the lower bound is saturated:


All the ground states are made of configurations that minimize the local Hamiltonian.

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Systematically finding the local rule
26

B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
27
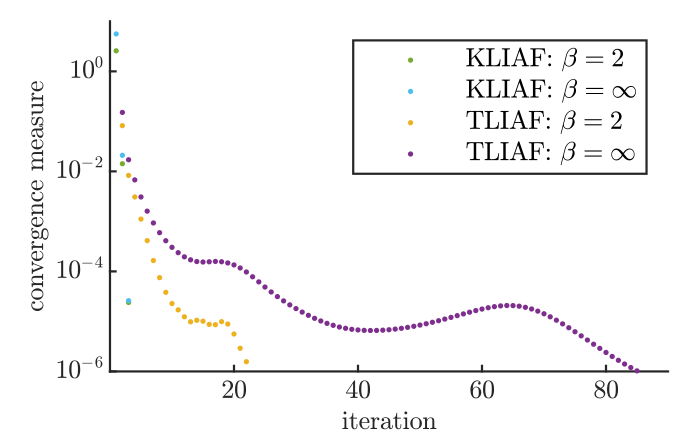
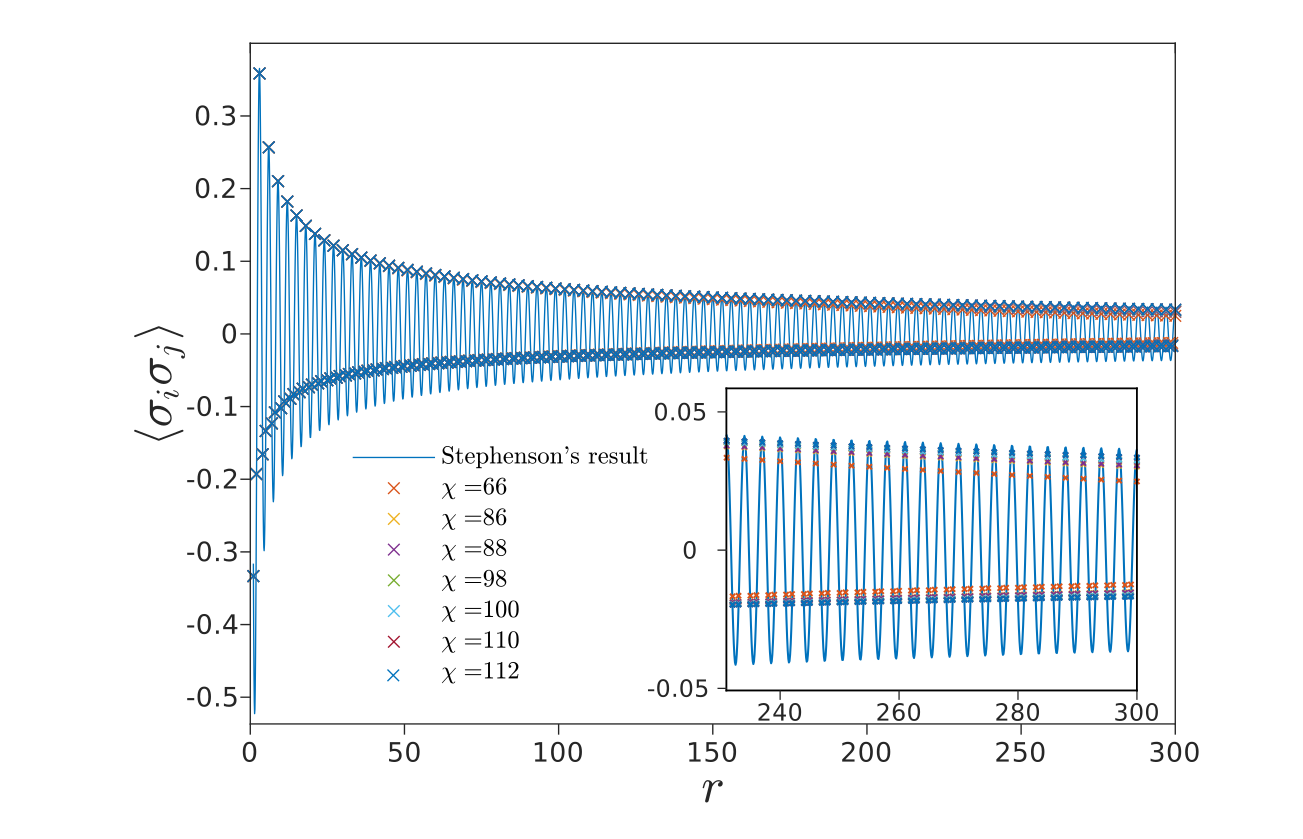
Does it work?

B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Does it work?
27


B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Does it work?
27


B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Does it work?
27

B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
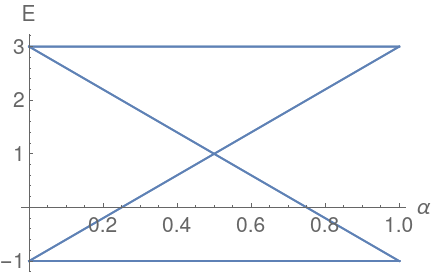
28
Spurious tiles

B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Spurious tiles
28



B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Spurious tiles
28




B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Spurious tiles
28





B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
Spurious tiles
28
B. Vanhecke, JC, L. Vanderstraeten, F. Verstraete, F. Mila, PRR 3, (2021)
F.F. Song, G. M. Zhang, PRB 105, (2022)
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
TNs for frustrated Ising models
J. Colbois
29
The convergence of the tensor network contraction depends on the formulation of the partition function
Tensor network approaches can be understood from the point of view of transfer matrices
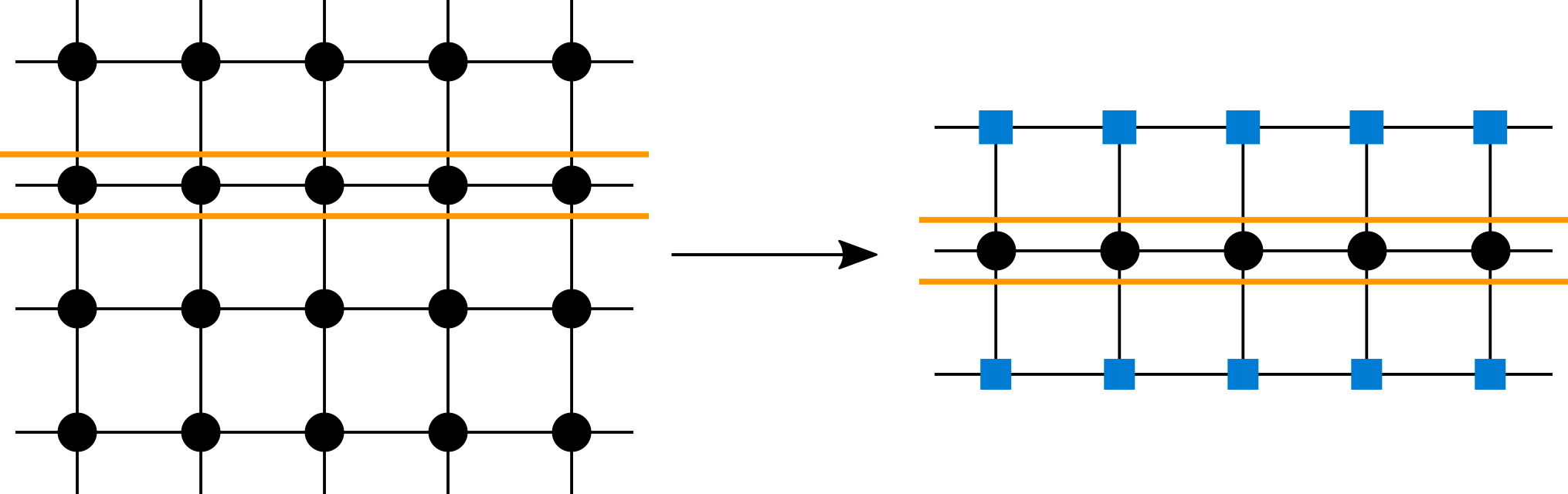
R. J. Baxter, J. Math. Phys. 9, 1968
Orús, Vidal, PRB 78, 2008;
V. Zauner-Stauber et. al. PRB 97,2018;
M. Fishman et. al PRB 98, 2018

C. Wang, S.-M. Qin, H.-J. Zhou, PRB 90, (2014)
Z. Zhu, H. G. Katzgraber, arXiv:1903.07721 (2019)
B. Vanhecke, JC, et al. PRR 3, (2021)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
M. Friaz-Pérez, M, Mariën et al, arXiv:2104.13264, (2021)
F.F. Song, G. M. Zhang, PRB 105, (2022)
If the ground-state rule is implemented at the level of the tensor, the algorithms converge

A model with several macroscopically degenerate phases
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.

JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
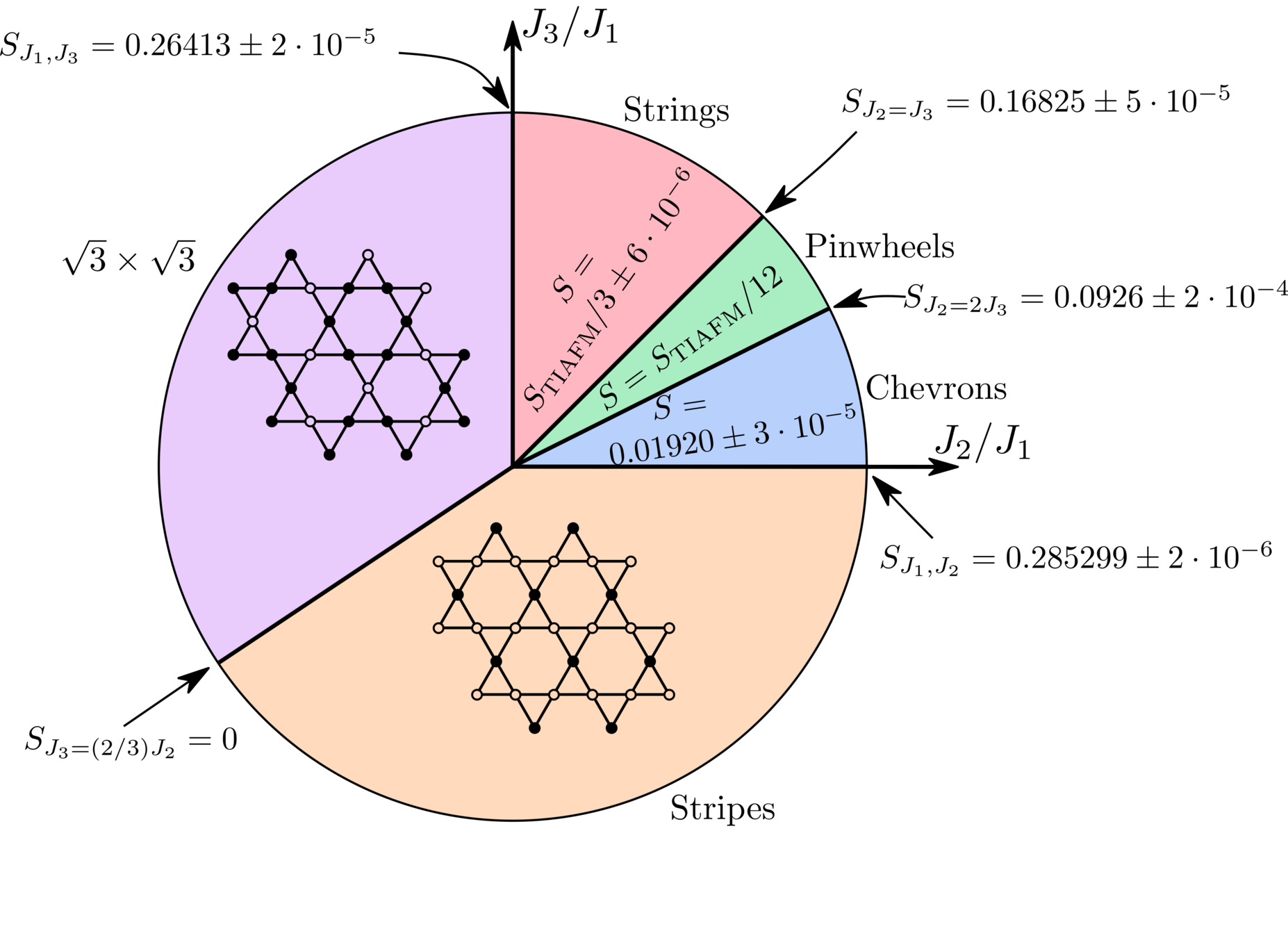
Phase diagram
J. Colbois
30
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Phase diagram
J. Colbois
30



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
Residual entropies
J. Colbois
31
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
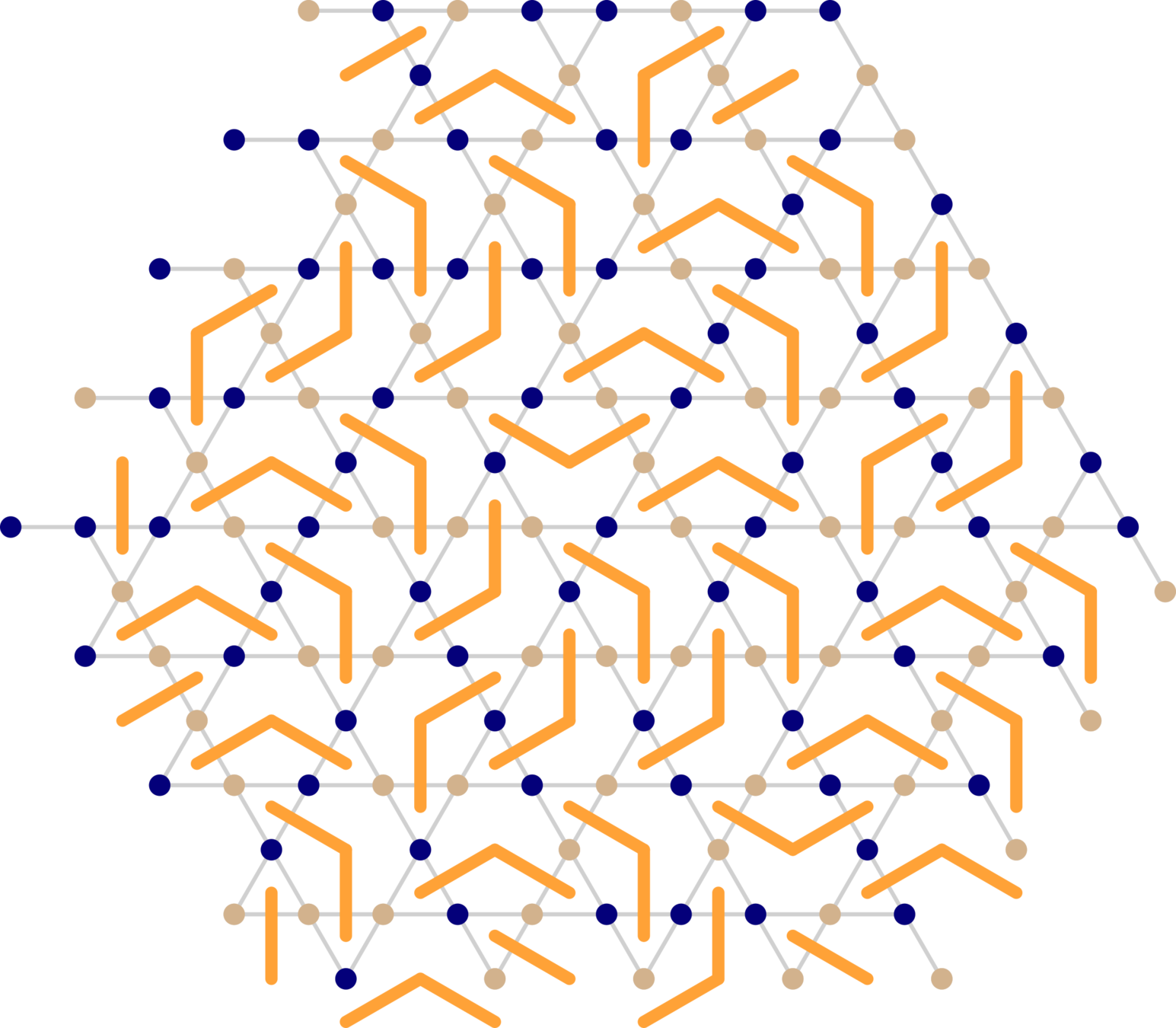
Residual entropies: chevrons phase
J. Colbois
31
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
31
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Residual entropies: chevrons phase




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
32
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Residual entropies: chevrons phase


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
33
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Residual entropies: pinwheels phase



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
33
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Residual entropies: pinwheels phase




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
33
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
Residual entropies: pinwheels phase




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
34
Residual entropies: pinwheels phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)






IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
34
Residual entropies: pinwheels phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)





IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
34
Residual entropies: pinwheels phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
35
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
35
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
35
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
36
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
36
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)




IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
36
Residual entropies: strings phase
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
37
Discussion
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)


IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
37
Discussion
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)



IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
37
Discussion
JC, B. Vanhecke, L. Vanderstraeten, et al., arXiv:2206.11788 (2022)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
38
Open questions...
Tensor network "problem": does this solution work for TNR?
Contraction of PEPS wavefunctions?
Use of the ground-state local rule: existence of the cluster?
Nature of the phases at higher temperatures?
A different approach for spin glasses: extend to approximate contraction?
Ronceray and Le Floch, PRE 100, (2019)
J. G. Liu, L. Wang, P. Zhan, PRL 126, (2021)
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
38
Open questions...
Ongoing work with Afonso Rufino , Samuel Nyckees and Frédéric Mila
The convergence of the tensor network contraction depends on the formulation of the partition function
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
39
Take home...
JC, B. Vanhecke et al., arXiv:2206.11788 (2022)
B. Vanhecke, JC, et al., Phys. Rev. Research 3 (2021)
If the ground-state rule is implemented at the level of the tensor, approximate contraction aglorithms converge
The convergence of the tensor network contraction depends on the formulation of the partition function
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
39
Take home...
JC, B. Vanhecke et al., arXiv:2206.11788 (2022)
B. Vanhecke, JC, et al., Phys. Rev. Research 3 (2021)
If the ground-state rule is implemented at the level of the tensor, approximate contraction aglorithms converge
The convergence of the tensor network contraction depends on the formulation of the partition function
IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
39
Take home...
Tensor networks are a powerful tool to study 2D classical spin systems!
JC, B. Vanhecke et al., arXiv:2206.11788 (2022)
B. Vanhecke, JC, et al., Phys. Rev. Research 3 (2021)
If the ground-state rule is implemented at the level of the tensor, approximate contraction aglorithms converge
The convergence of the tensor network contraction depends on the formulation of the partition function

IISER PUNE | 09.2022 | TNS FOR FRUSTRATED ISING MODELS : PARTIAL LIFTING OF G. S. DEG.
J. Colbois
39
Take home...
Tensor networks are a powerful tool to study 2D classical spin systems!
Thank you!
JC, B. Vanhecke et al., arXiv:2206.11788 (2022)
B. Vanhecke, JC, et al., Phys. Rev. Research 3 (2021)