Learning from DeFi: Would Automated
Market Makers Improve Equity Trading?

Katya Malinova and Andreas Park

Women in Microstructure

San Francisco, June 25 2023

Preliminaries & Some Motivation

Blockchain: borderless general purpose value and resource management tool

Basic Idea

DeFi: financial applications that run on blockchains

$\Rightarrow$ brought new ideas and tools

one new market institution: automated market makers

Decentralized trading with automated market makers (AMM)

AMMs price: mechanical, based on the amount of liquidity deposits.

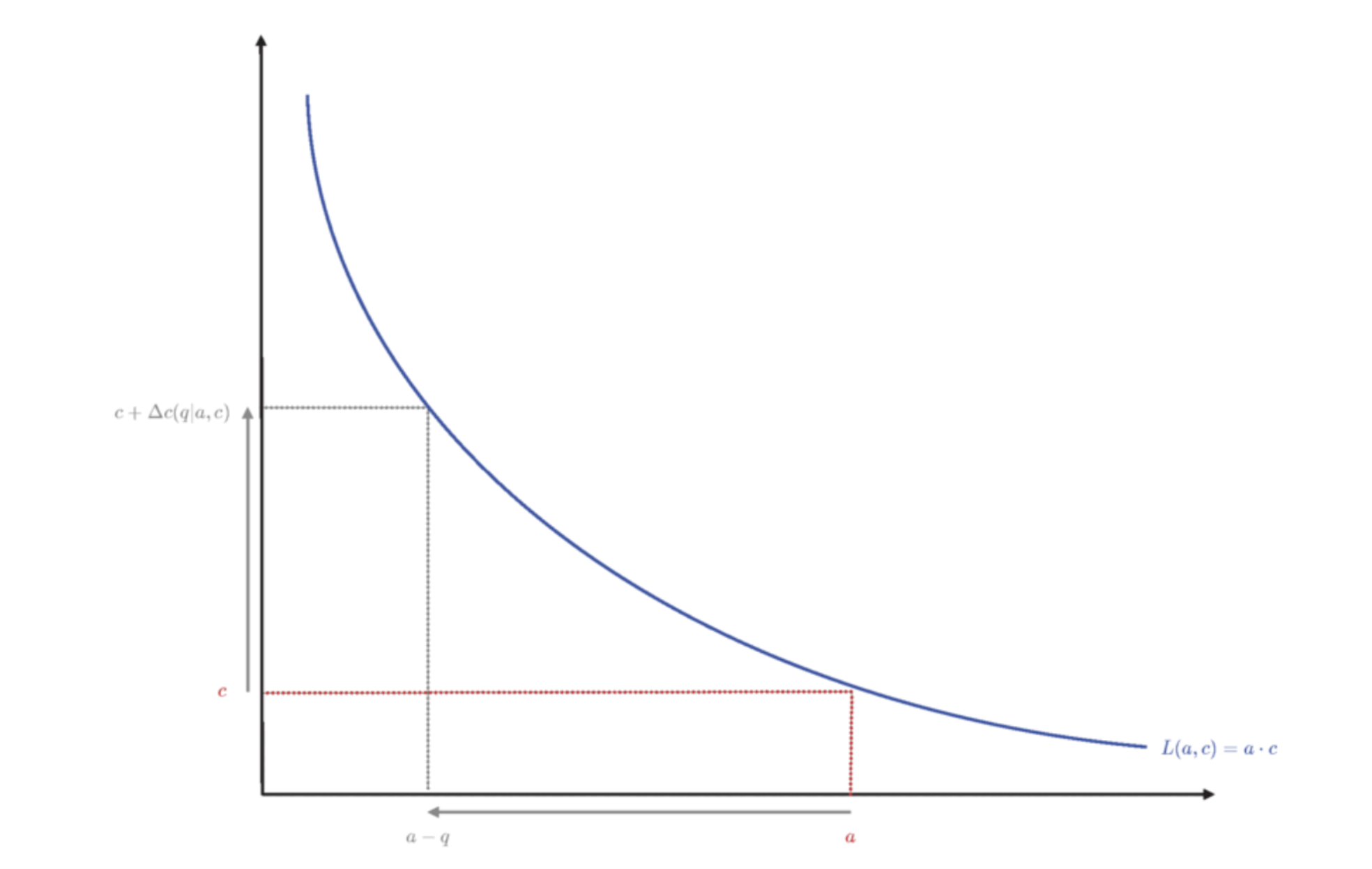
\[L(a-q,c+\Delta c)=L(a,c)\]

\[a\]

\[a-q\]

\[c+\Delta c\]

\[c\]

Given the initial deposits (a, c), the total cost $\Delta c$ for $q$ units is such that:

Remove (buy) $q$ units of the asset from the pool

Add (pay) $\Delta c$ cash to the pool

\[a\]

asset (stock)

cash

liquidity after trade $=$ liquidity before trade

Key Components

Our question:
1. Can an economically viable AMM be designed for current equity markets?
2. Would such an AMM improve current markets?

pooling of liquidity

pro-rated
- fee income
- risk

Liquidity providers:
- use existing assets to earn passive (fee) income

Liquidity demanders:
- predictable price
- continuous trading
- ample liquidity

Liquidity Supply and Demand in an Automated Market Maker

\[(a-q)\cdot(c+\Delta c)=a\cdot c\]

\[a\]

\[a-q\]

\[c+\Delta c\]

\[c\]

$\Rightarrow$ Cost of buying $q$ \[\Delta c=\frac{c}{a-q}\times q\]
Average price per unit \[p(q)=\frac{c}{a-q}\]

Initial marginal price = initial asset value: \[p(0) =\frac{c}{a}\]

Average "spread" paid: \[\frac{p(q)}{p(0)}-1=\frac{q}{a-q}.\]

larger liquidity deposits $a$ $\Rightarrow$
- lower costs for liquidity demanders

Most Common Pricing: Constant Product: $L(a,c)=a\cdot c$

Per unit $p(q)$ as liquidity deposits increase

\[(a-q)\cdot(c+\Delta c)=a\cdot c\]

\[a\]

\[a-q\]

\[c+\Delta c\]

\[c\]

$\Rightarrow$ Total cost of trading $q$ \[\Delta c=\frac{c}{a-q}\times q\]
Average price per unit \[p(q)=\frac{c}{a-q}\]

Initial marginal price = initial asset value: \[p(0) =\frac{c}{a}\]

Liquidity providers: positional losses

If trade is informed $\Rightarrow$ new asset value \[=\frac{c+\Delta c}{a-q}>p(q)\]

larger liquidity deposits $\Rightarrow$
- larger positional losses for liquidity providers

Liquidity providers "undercharge" the informed

Basics of Liquidity Provision in an AMM

\[\underbrace{F p_0 V}_{\text{fees earned on balanced flow}}+\int_0^\infty\underbrace{(\Delta c(q^*)-q^*p_t(R)}_{\text{adverse selection loss when the return is $R$}} +\underbrace{F \cdot \Delta c(q^*))}_{\text{fees earned from arbitrageurs}}~\phi(R)dR \ge 0.\]

$q^* $ is what arbitrageurs trade to move the price to reflect $R$

fundamental value $\nearrow$
- $\Rightarrow$ price $\nearrow$
  - $\Rightarrow$ sold the asset for less than its worth
fundamental value $\searrow$
- $\Rightarrow$ price $\searrow$
  - $\Rightarrow$ bought the asset for more than its worth

LP compensation in AMM: liquidity providers earn fees

must earn more fees on balanced flow than what they lose on price movement

\[\text{fee income} +\underbrace{\text{what LP sold it for}-\text{value of net position}}_{\text{adverse selection loss}} \ge 0 \]

in AMMs:
protocol fee

takes the role of the TradFi bid-ask spread

a liquidity trade
- arbitrageurs trade back to the original price
- $\to$ liquidity deposits return to the original values $(a, c)$

losses on informed
no gains (nor losses) on noise

Basics of Liquidity Provision

\[\int_0^\infty\underbrace{(\Delta c(q^*)-q^*p_t(R)}_{\text{adverse selection loss when the return is $R$}} +\underbrace{F \cdot \Delta c(q^*))}_{\text{fees earned from arbitrageurs}}~\phi(R)dR +\underbrace{F p_0 V}_{\text{fees earned on balanced flow}}\ge 0\]

\[\frac{1}{\text{initial deposit}}\int_0^\infty(\Delta c(q^*)-q^*p_t(R)+F \cdot \Delta c(q^*))~\phi(R)dR +\frac{F p_0 V}{\text{initial deposit}}\ge 0\]

\[\int_0^\infty\left(\frac{\Delta c(q^*)-q^*p_t(R)}{\text{initial deposit}} +F \cdot \frac{\Delta c(q^*)}{\text{initial deposit}}\right)~\phi(R)dR +\frac{F p_0 V}{\text{initial deposit}}\ge 0\]

closed form functions of $R$ only
(see Barbon & Ranaldo (2022))

Sidebar: we can quantify how much a PASSIVE LP loses when the price moves by $R$

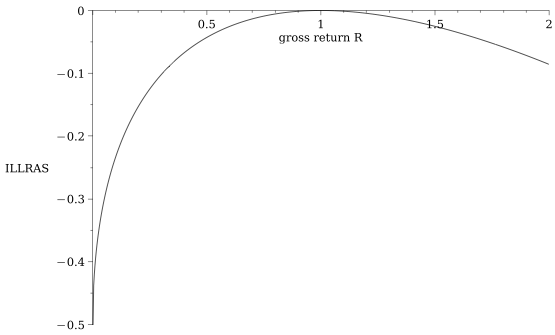
for orientation:

If the stock price drops by 10% the incremental loss for liquidity providers is 13 basis points on their deposit
- $\to$ total loss=-10.13%
If the stock price rises by 10%, the liquidity provider gains 12 basis points less on the deposit
- $\to$ total gain =9.88%

\[\frac{\text{adverse selection loss when the return is $R$}}{\text{initial deposit}}=\sqrt{R}-\frac{1}{2}(R+1)\]

same as in Barbon & Ranaldo (2022)

Liquidity Provision Decision

Express liquidity provision as a "collective" deposit $\alpha$ of firm's market cap
- initial deposit $\alpha \nearrow $ $\Rightarrow$ LP payoff $\searrow$

\[E[\text{IILRAS}(R)]+F\cdot E[\text{another function of }R]+F\cdot \frac{\text{dollar volume}}{\text{initial deposit}}\ge 0.\]

\[\text{what LP sold it for}-\text{value of net position}+\text{fee income} \ge 0 \]

Competitive liquidity provision
$\Rightarrow$ Find $\alpha$ such that LPs break-even, as a function of
- balanced volume
- asset volatility/return distribution
- fee

Liquidity Demander's Decision & (optimal) AMM Fees

Wants to trade some quantity $q$.
Better off with AMM relative to traditional market if

\[\text{AMM price impact} +\text{AMM fee} \le \text{bid-ask spread}.\]

Two opposing forces when $F\nearrow$ for liquidity demand
- more liquidity provision
  $\to$ lower price impact
- more fees to pay
Finding: there exists an optimal (for the liquidity demander) fee $>0$

\[F^\pi=\frac{1}{E[|\sqrt{R}-1|/2]+V}\left(-2q\ E[\text{ILLRAS}]+ \sqrt{-2qV\ E[\text{ILLRAS}]}\right).\]

What's next?

Have:
- equilibrium choices for competitive liquidity provision
- fee that minimizes liquidity demander AMM costs ($>0$)
Next:
- Calibrate to stock markets
- Optimal fees?
- AMM Feasible?
  - AMM costs at the optimal fee < bid-ask spread?

How we think of the Implementation of an AMM for our Empirical Analysis

Approach: daily AMM deposits

AMMs close overnight.
Market: opening auction $\to$ $p_0$
Determine: optimal fee; submit liquidity $a,c$
at ratio $p_0=c/a$ until break even $\alpha=\overline{\alpha}$
Liquidity locked for day
At EOD release deposits and fees
Back to 1.

Background on Data

Special Consideration 1: What volume?

some volume may be intermediated
with AMMs: no need for intermediation
$\to$ intermediated volume could disappear
$\to$ use volume/2
Some caveats, e.g.
- arbitrageur volumes
- larger volume if AMM has lower trading costs

Special Consideration 2: What's $q$ (the representative order size)?

use average per day
take long-run average + 2 std of daily averages
(also avg $\times 2$,$\times 4$, depth)

All displayed data CRSP $\cap$ WRDS

CRSP for shares outstanding
WRDS-computed statistics for
- quoted spreads (results similar for effective)
- volume
- open-to-close returns
- average trade sizes, VWAP
Time horizon: 2014 - March 2022
Exclude "tick pilot" period (Oct 2016-Oct 2018)
All common stocks (not ETFs) (~7550).
Explicitly not cutting by price or size
All "boundless" numbers are winsorized at 99%.

Special Consideration 3:

Where to get returns and volume?

Approach 1: "ad hoc"
- "one-day-back" look
- take yesterday's return and volume when deciding on liquidity provision in AMM
Approach 2: estimate historical return distribution

AMMs based on historical returns

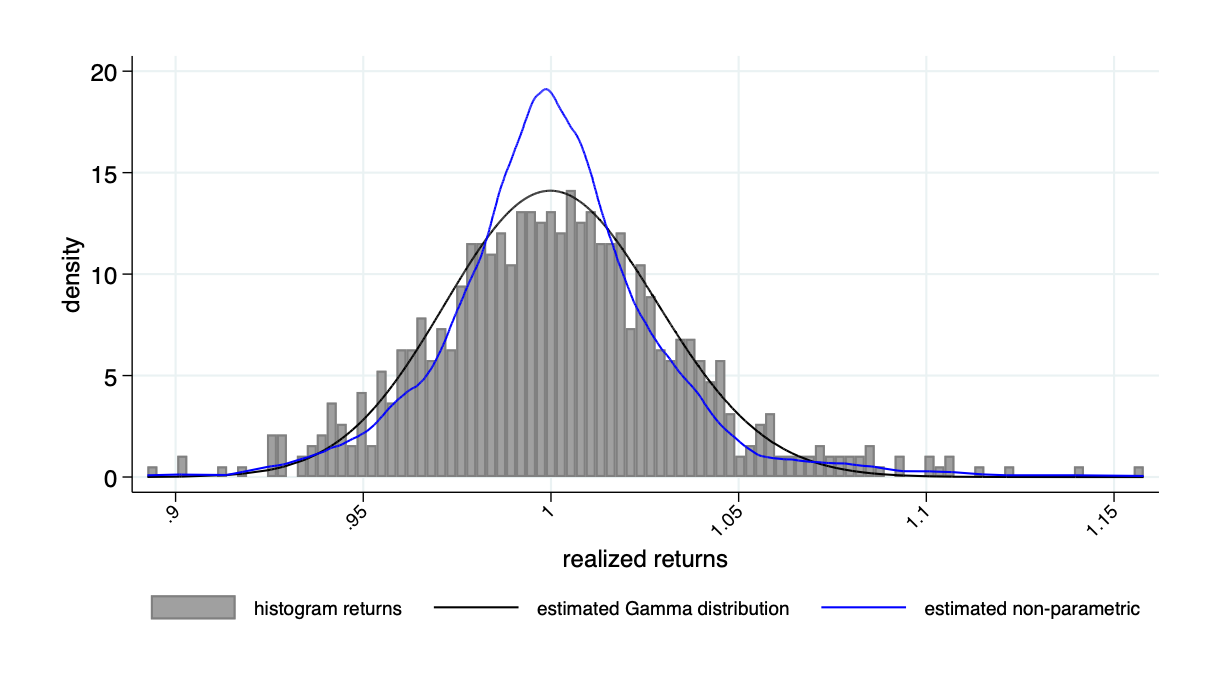
Return distribution example: Microsoft

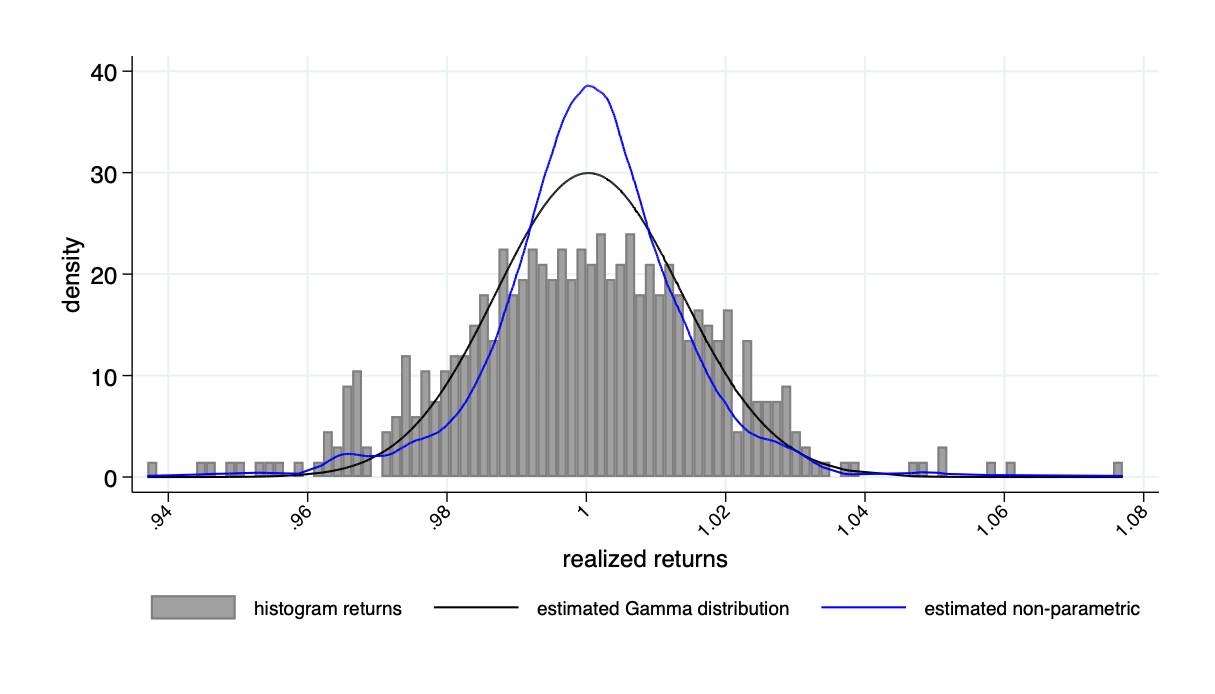
Return distribution example: Tesla

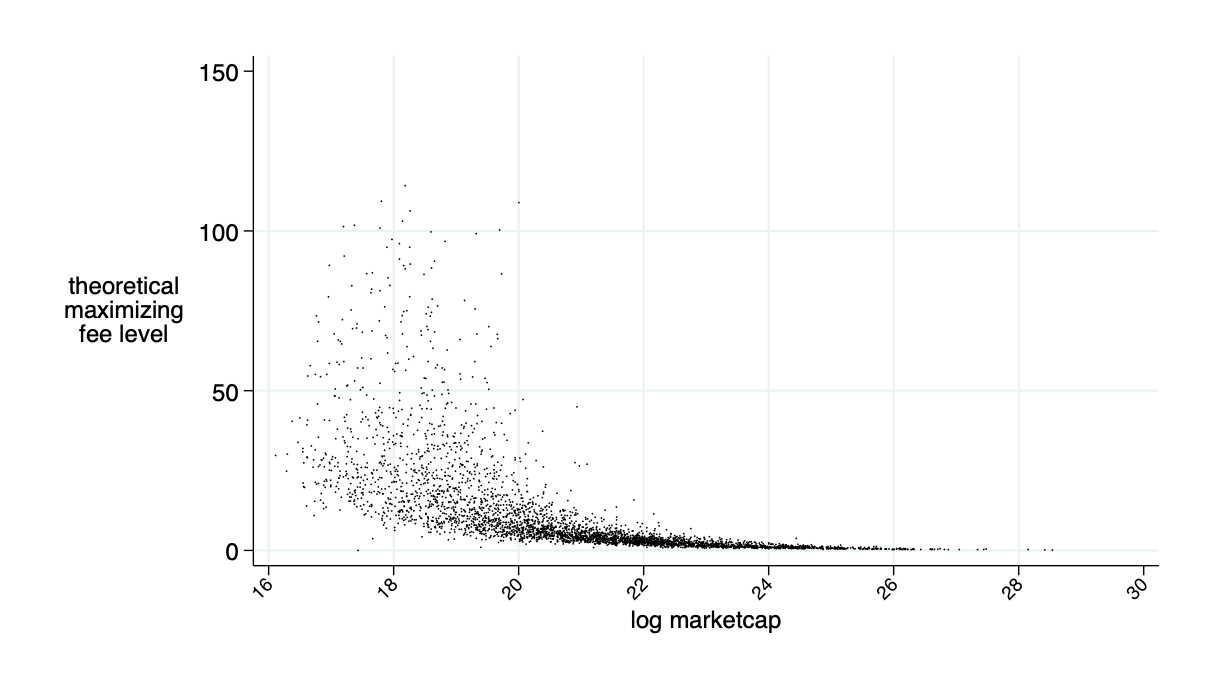
average $F^\pi=11$bps

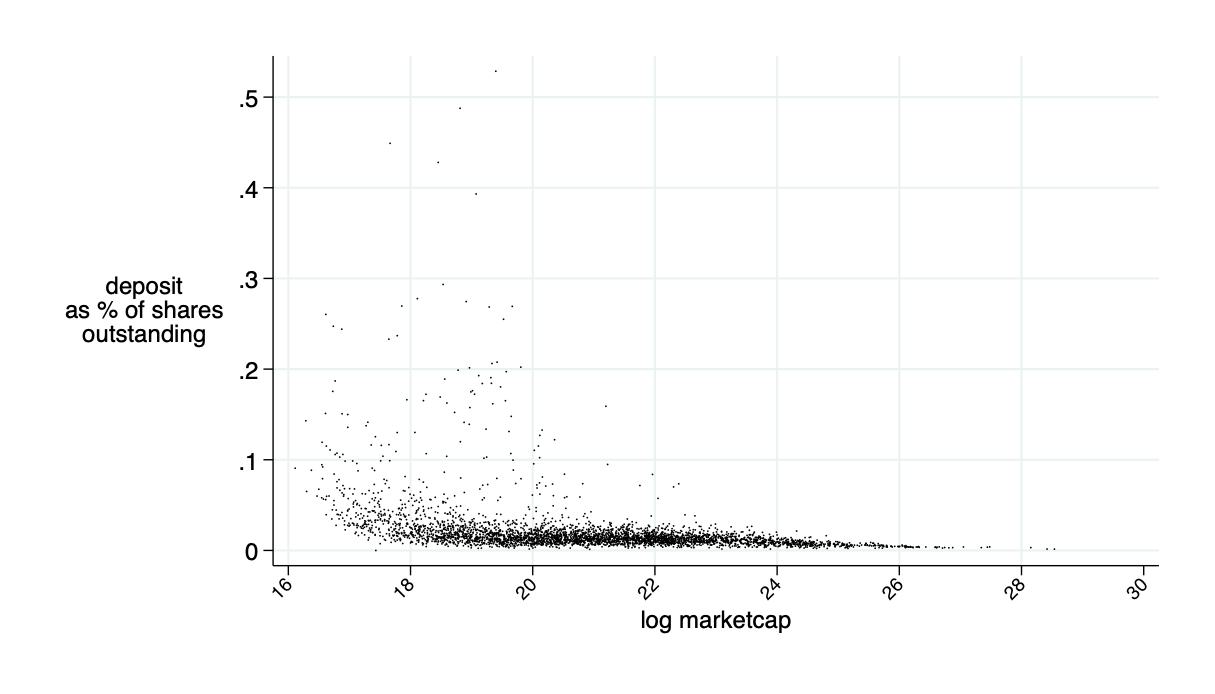
Average of the market cap to be deposited for competitive liquidity provision: $\bar{\alpha}\approx 2\%$

almost break even on average (average loss 0.2bps $\approx0$)

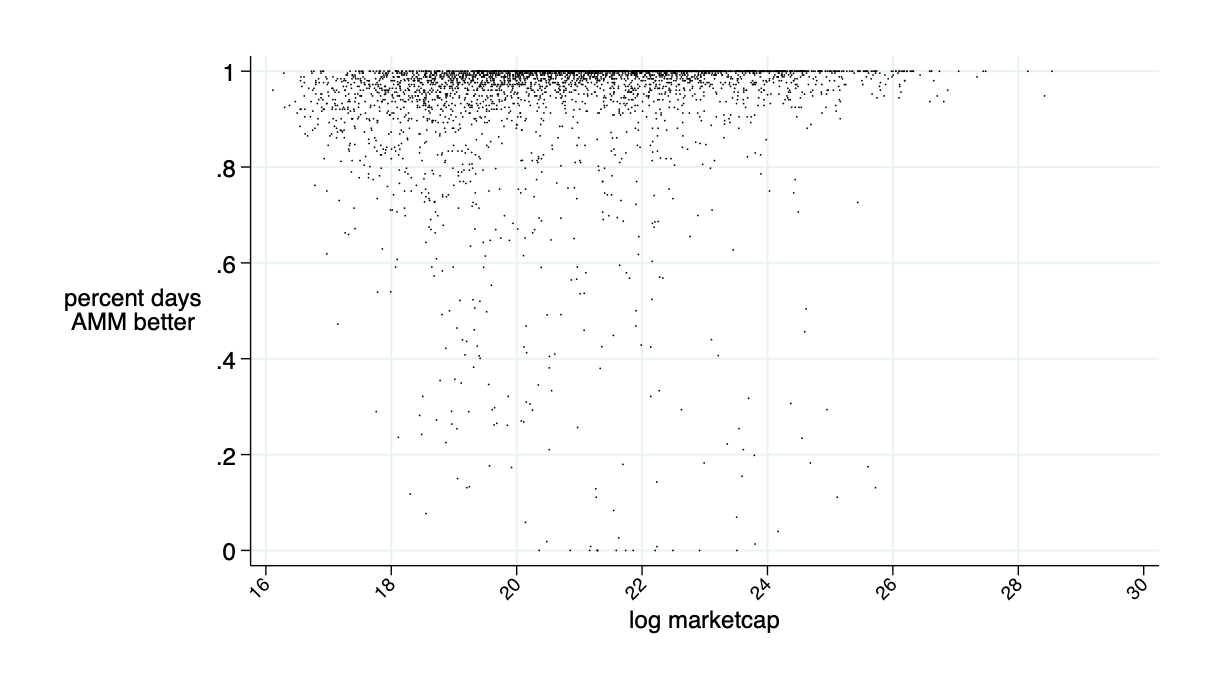
average: 94% of days AMM is cheaper than LOB for liq demanders

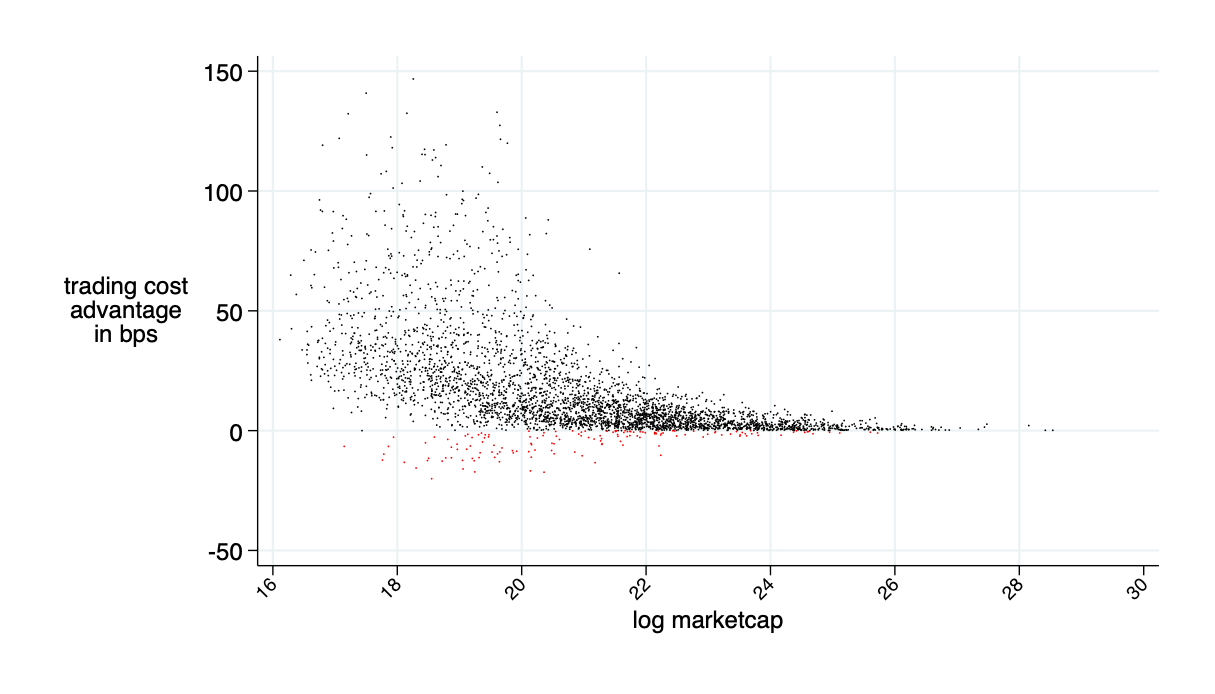
average savings: 16 bps

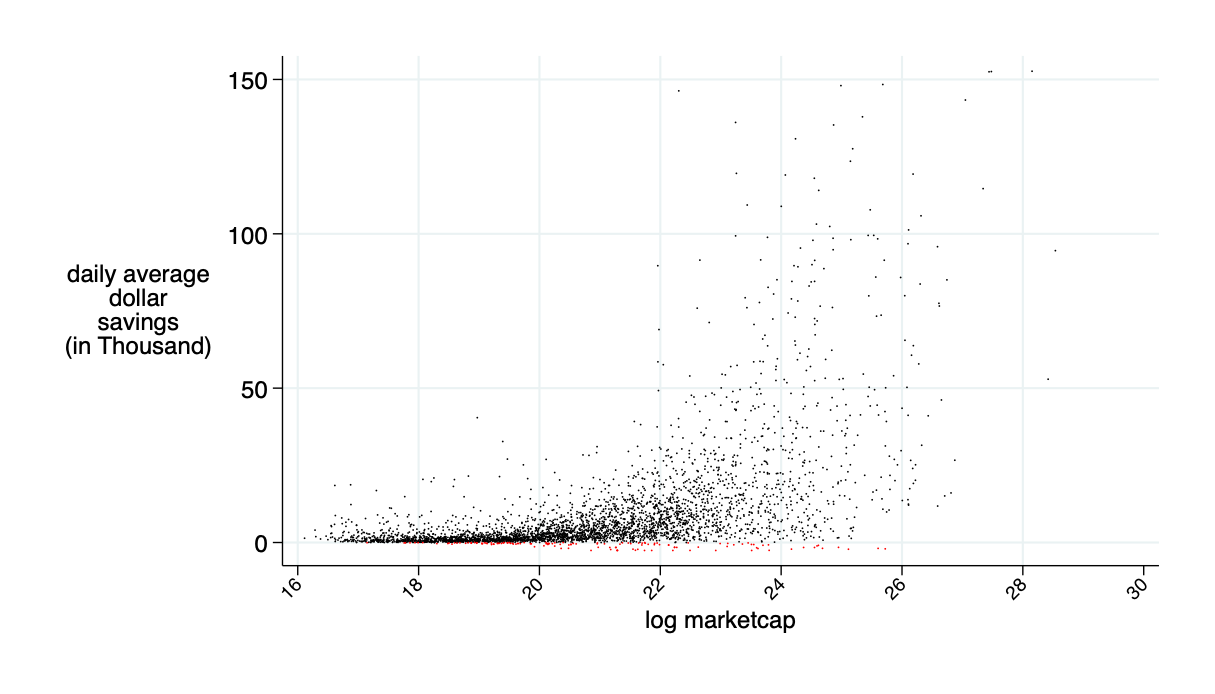
average daily: $9.5K

saves around 45% of transaction costs (measured as bid-ask spread)

average annual saving: $2.4 million

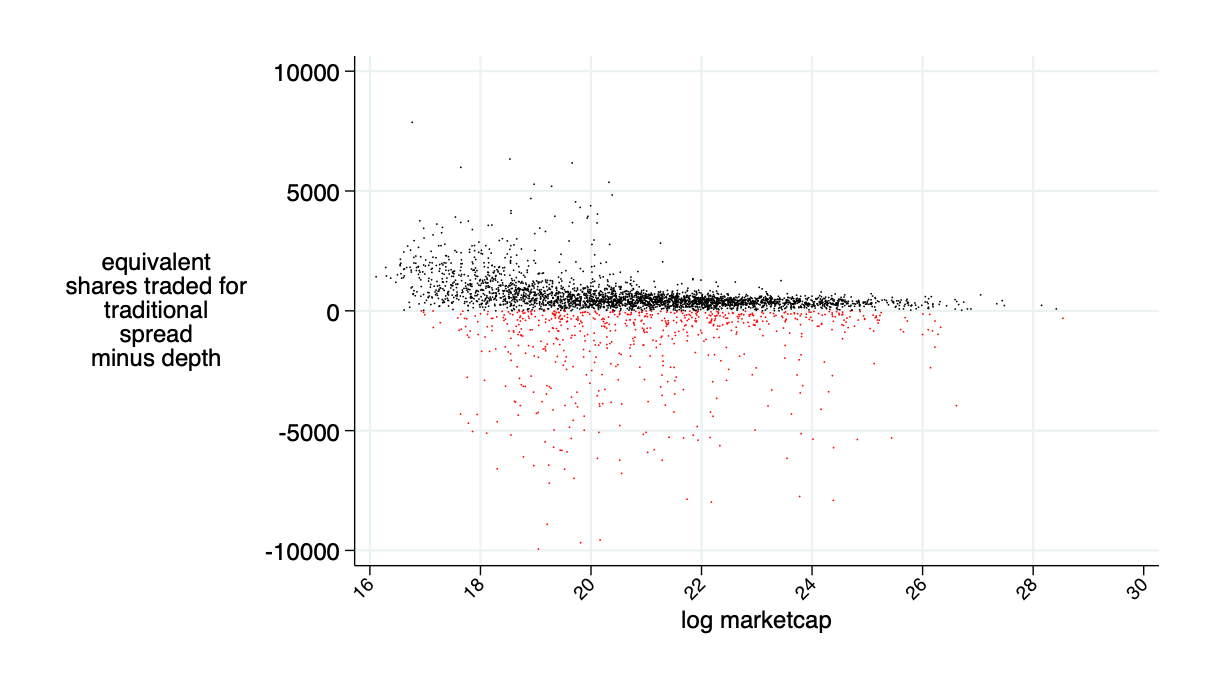
implied "excess depth" on AMM relative to the traditional market

Sidebar: Capital Requirement

Deposit Requirements

Our approach: measure liquidity provision in % of market cap
Share-based liquidity provision is trivial: the shares are just sitting at brokerages.
But: AMM requires an off-setting cash amount: $c =a\cdot p(0)$.
Cash is not free:
- at 6% annual rate, must pay 2bps per day.
- Would need to add to fees
But: do we need "all that cash"?
No.

(hand-waving argument)
2nd gen AMMs have liquidity provision "bands": specify price range for which one supplies liquidity
Here: specify range for $R\in(\underline{R},\overline{R})$
Outside range: don't trade.
Inside range: "full" liquidity with constant product formula.
Implication: only need cash and shares to satisfy in-range liquidity demand.

For return $R$, the following number of shares change hands: \[q=a\cdot(1-\sqrt{R^{-1}}).\]
Fraction of share deposit used \[\frac{q}{a}=1-\sqrt{R^{-1}}.\]
Fraction of cash used \[\frac{\Delta c ("R")}{c}=\frac{1-\sqrt{R^{-1}}}{\sqrt{R^{-1}}}.\]
Example for $R=.9$ (max allowed price drop $=10\%$) \[\frac{\Delta c ("R")}{c}=-5\%.\]
$\Rightarrow$ "real" cash requirements $\not=$ deposits

$\Rightarrow $ Need about 5% of the value of the shares deposited -- not 100% -- to cover up to a 10% return decline

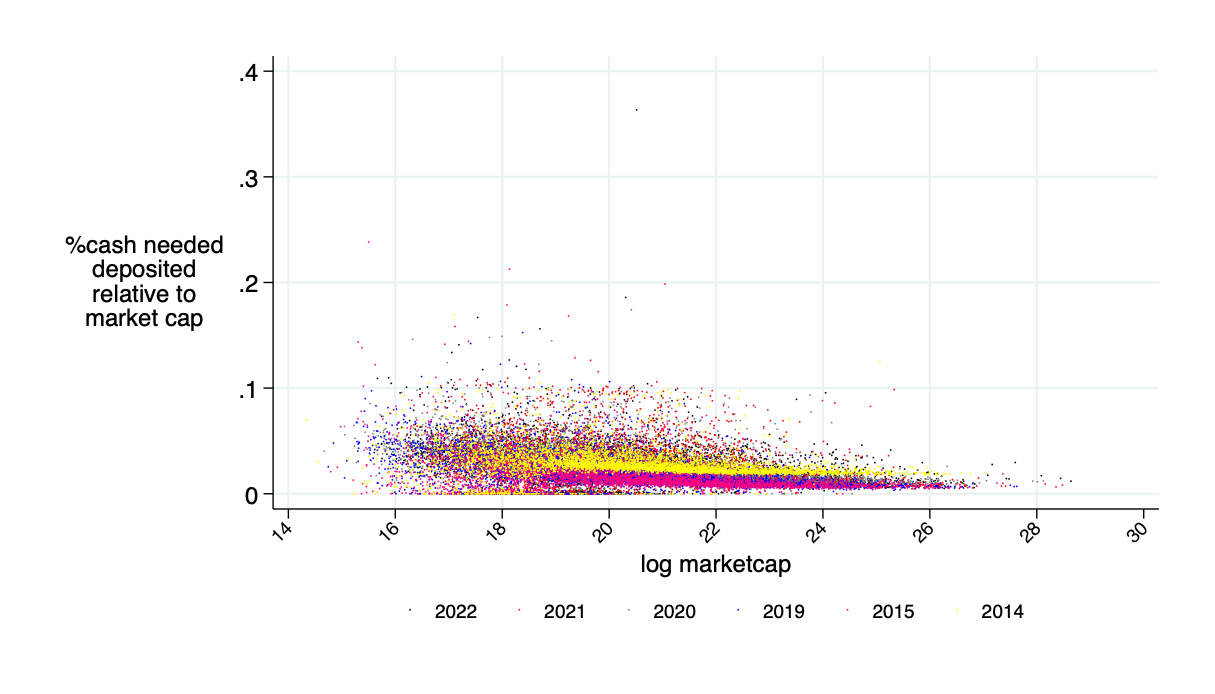
An alternative to -10% circuit breaker:

max cash needed based on long-run past average R $-$ 2 std

Summary

AMMs do not require a blockchain - just a concept
could be run in the existing world (though there are institutional and regulatory barriers)

Our question:
1. Can an economically viable AMM be designed for current equity markets?
2. Would such an AMM improve current markets?

Answers:
1. Yes.
2. Massively.

Source of Savings:
- Liquidity providers $\not=$ Citadel!
- $\to$ passive liquidity provision
- $\to$ use idle capital
- $\to$ + better risk sharing

pooling of liquidity

pro-rated
- fee income
- risk

Liquidity providers:
- use existing assets to earn passive income

Liquidity demanders:
- predictable price
- continuous trading
- ample liquidity

Optimally Designed AMMs with
"ad hoc" one-day backward look

Optimal fee $F^\pi$

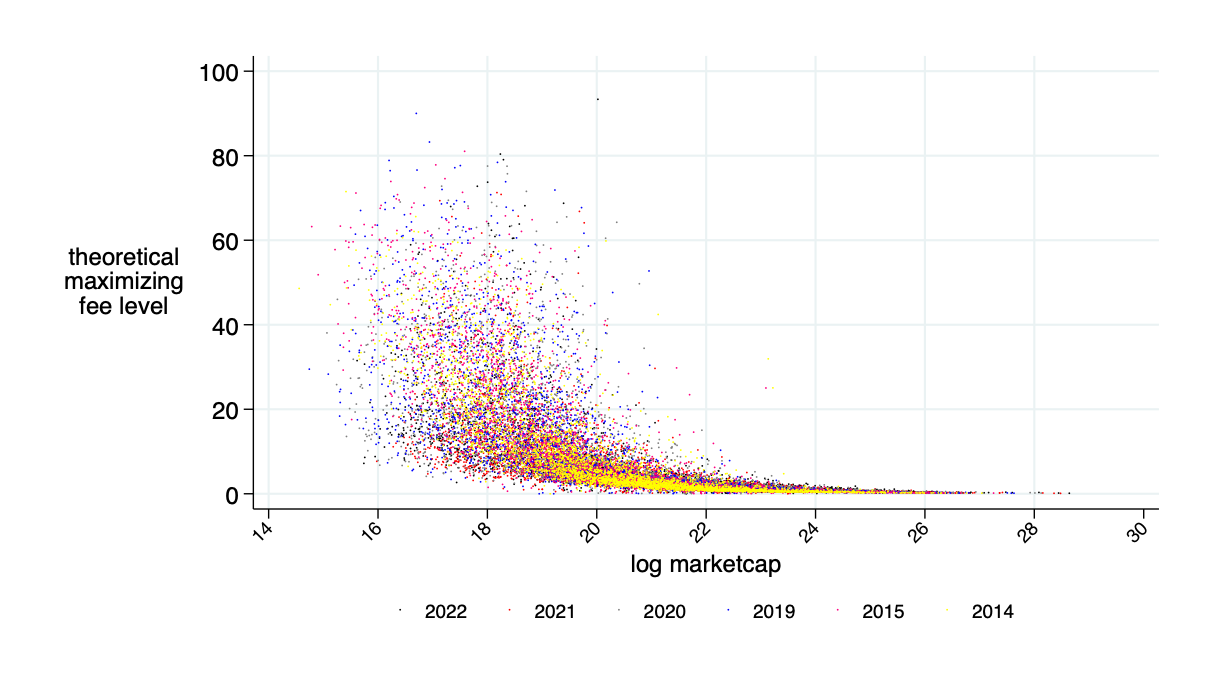
average benefits liquidity provider in bps (average=0)

Insight: Theory is OK - LP's about break even

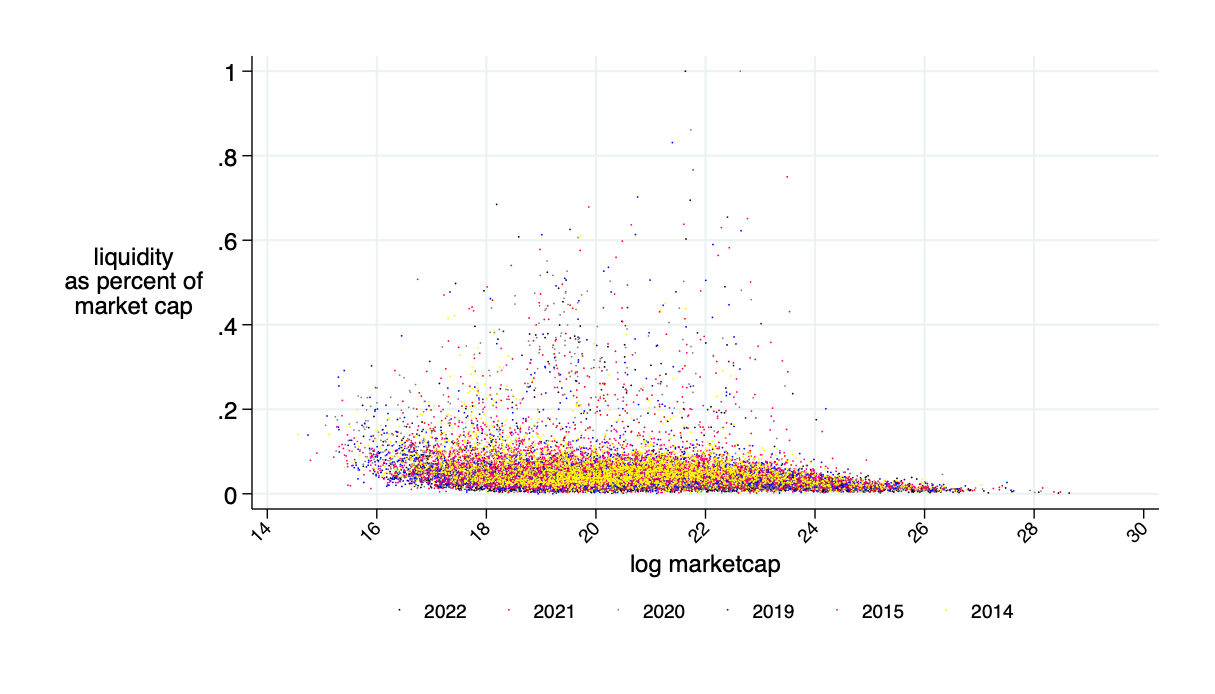
$\overline{\alpha}$ for $F=F^\pi$

Need about 10% of market cap in liquidity deposits to make this work

actually needed cash as fraction of "headline" amount

Only need about 5% of the 10% marketcap amount in cash

AMMs are better on about 85% of trading days

quoted spread minus AMM price impact minus AMM fee (all measured in bps)

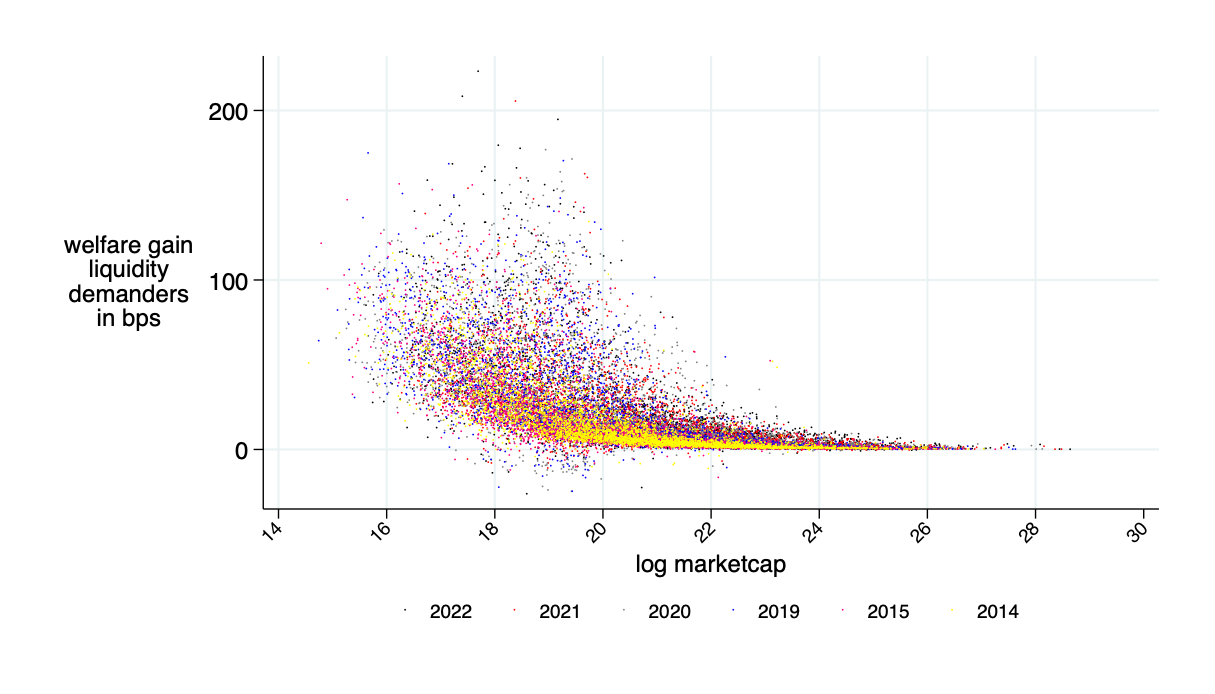
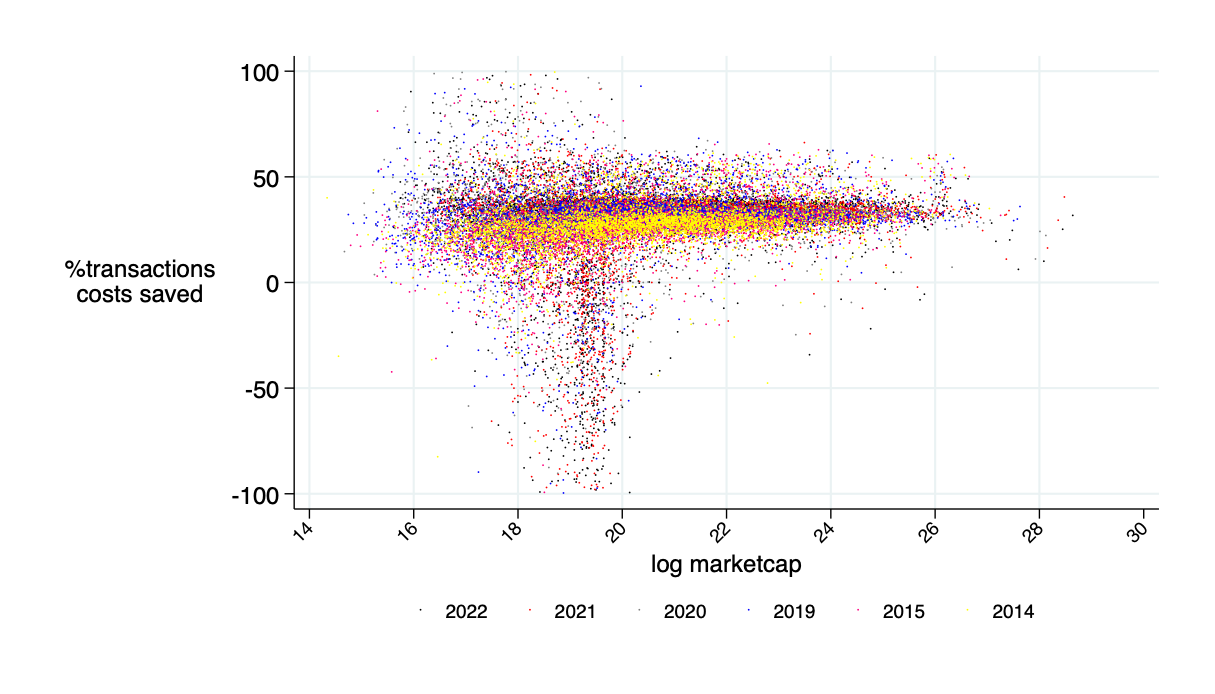
relative savings: what fraction of transactions costs would an AMM save? $\to$ about 30%

theoretical annual savings in transactions costs is about $15B

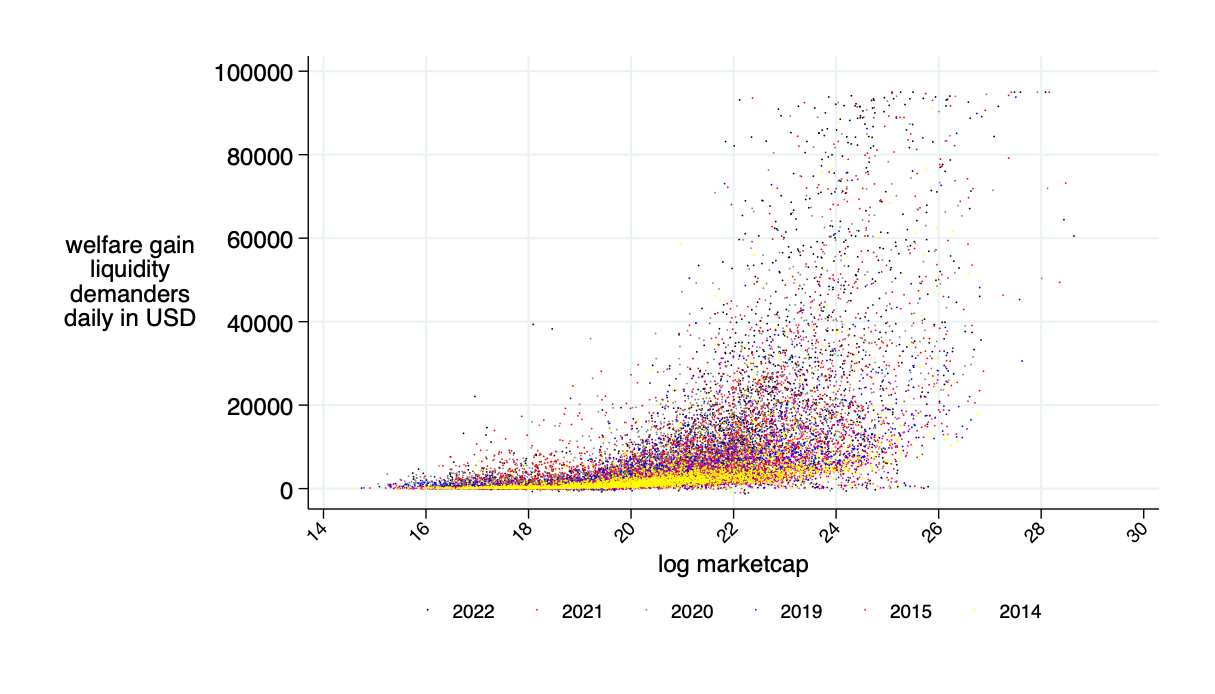
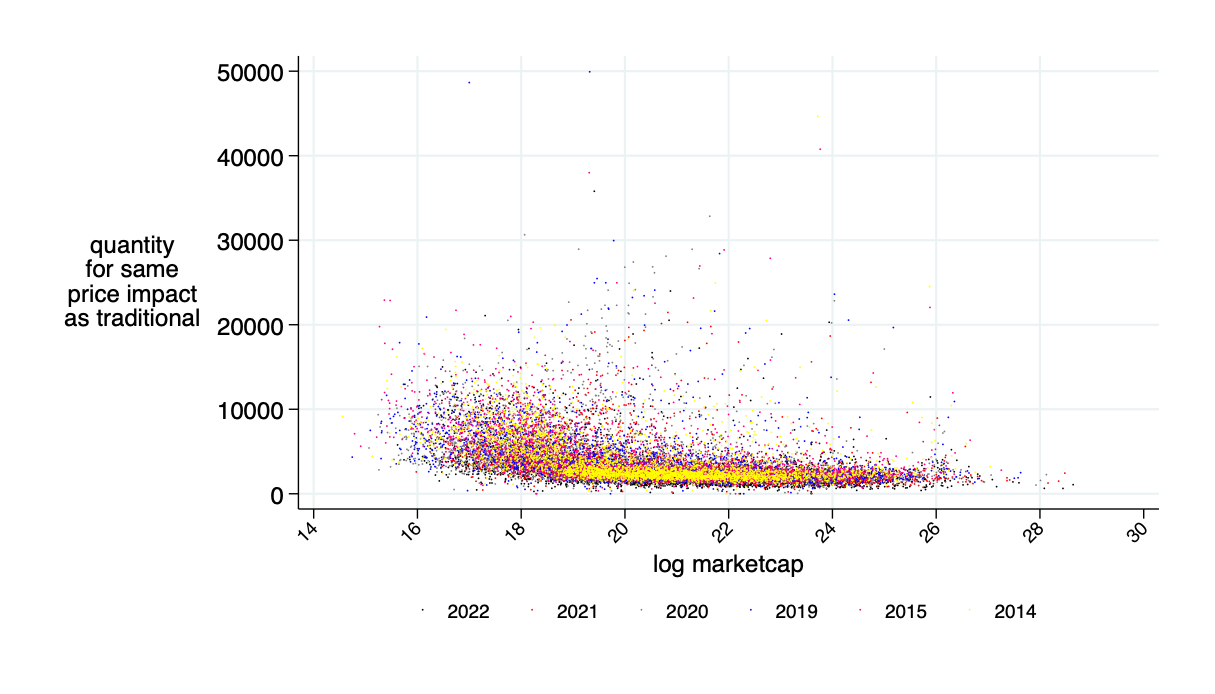
Literature

AMM Literature: a booming field

Theory
- Lehar and Parlour (2021): for many parametric configurations, investors prefer AMMs over the limit order market.
- Aoyagi and Ito (2021): co-existence of a centralized exchange and an automated market maker; informed traders react non-monotonically to changes in the risky asset’s volatility
- Capponi and Jia (2021): price volatility $\to$ welfare of AMM LPs; conditions for a breakdown of liquidity supply in the automated system; more convex pricing $\to$ lower arbitrage rents & less trading.
- Capponi, Jia, and Wang (2022): decision problems of validators, traders, and MEV bots under the Flashbots protocol.
- Park (2021): properties and conceptual challenges for AMM pricing functions
- Milionis, Moallemi, Roughgarden, and Zhang (2022): dynamic impermanent loss analysis for under constant product pricing.
- Hasbrouck, Rivera, and Saleh (2022): higher fee $\Rightarrow$ higher volume
Empirics:
- Lehar and Parlour (2021): price discovery better on AMMs
- Barbon and Ranaldo (2022): compare the liquidity CEX and DEX; argue that DEX prices are less efficient.