Tokenomics:
When Tokens Beat Equity

Technology

Legal/Regulation

Economic functions

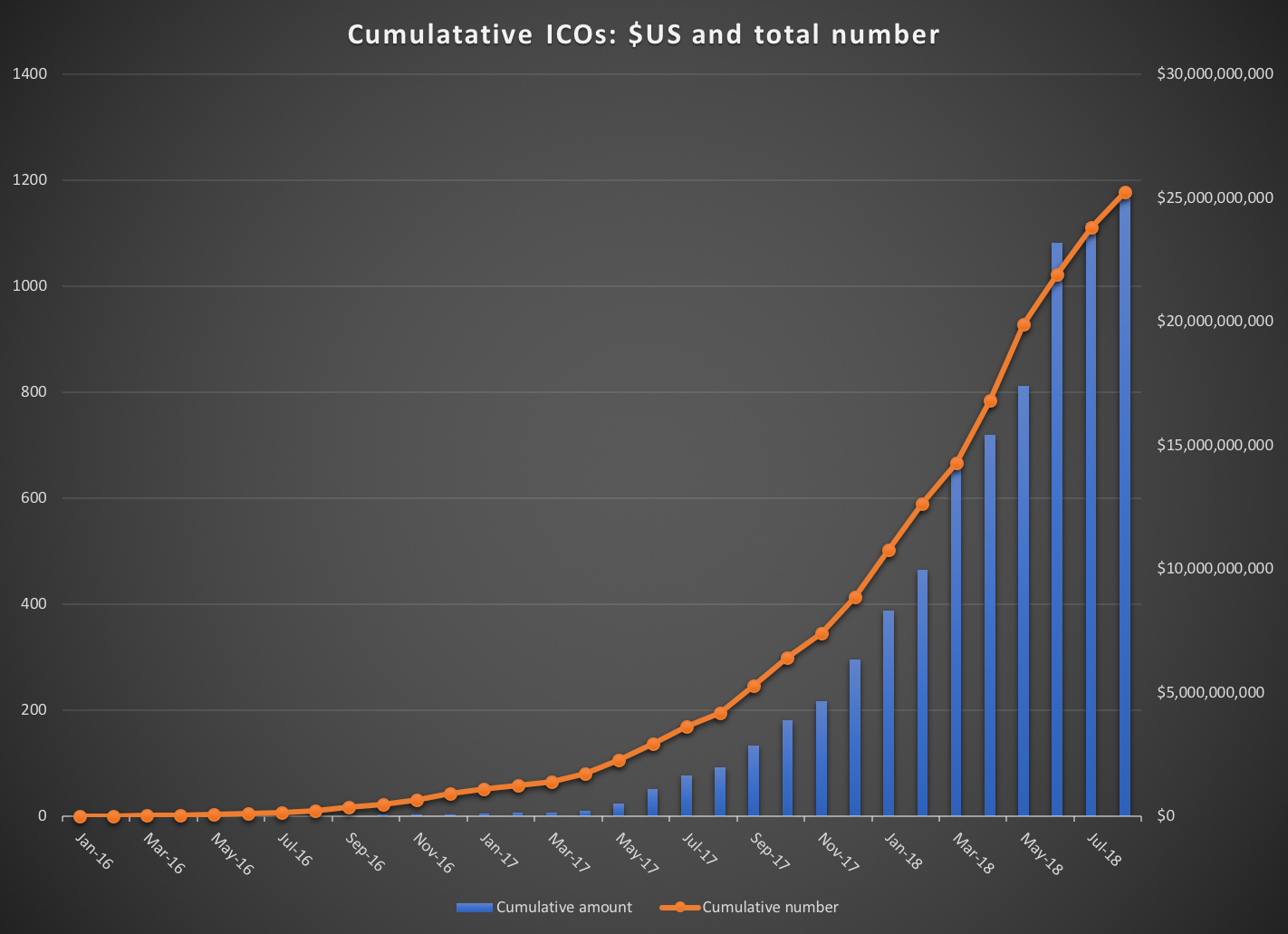
$25B total

$21B in 2018

Is there economic merit to tokens?

Do tokens solve an economic problem?

Infrastructure
 

reward and
internal currency

usage fee
or
incentive

usage fee
 

Service
 

Application
 

\(x_i\)

\(x_k\)

price

If financing with own funds  

\(\Rightarrow\) entrepreneur
     maximizes monopoly profits

\[\max_q (x-q)\cdot q -cq.\]

\(\Rightarrow\) produces
     monopoly quantity

marginal cost

marginal revenue

\(q^m=(x-c)/2\)

\(MR=x-2q\)

\(p(q)=x-q\)

general idea: sell future output

two approaches for token sales

sell a fraction of future revenue

sell units of future output

• we call it revenue sharing
• formally: sell \(\alpha_t\) of \(T\) tokens
• produce \(q\) units a require \(T/q\) tokens per unit

• we call this output presale
• formally: sell \(t\) tokens
• produce \(q\) units and keep revenue from \(q-t\) tokens

Entrepreneur does not internalize the effect of an extra output unit on the token value for the tokenholders!

Result: overproduction

entrepreneur issues \(t\) tokens

   for \(x\le t\): earns zero 

   for \(x>t\): solves \[\max_q  (q-t) (x-q)-cq.\]

    \(MR(q^{ps})+t=c\)

\[\Rightarrow q^{ps}=\frac{x-c+t}{2}>q^m\]

\(q^{ps}>q^m\)

\(\Rightarrow\) "tilts" marginal revenue for
     entrepreneuer left because
     get only fraction of revenue
​

\(\Rightarrow\) solves \((1-\alpha)\)MR(\(q^{rs}\)) = c

\[\Rightarrow q^{rs}=\frac{x-c/(1-\alpha_t)}{2}<q^m\]

Result: underproduction

NB: Similar to underinvestment in Chod and Lyandres (2020)

\(q^{rs}<q^m\)

revenue sharing: underproduction

output presale: overproduction

"does not internalize" = externality

address externality: TAX!

here: tax future token income

incremental token income gets shared

\(\Rightarrow\) combine the two to get the monopoly quantity!

Is token financing inferior? No!

• issue \(t\) tokens ex ante
• share \(\alpha_t\) of new tokens
• Entrepreneuer solves \[\max_q (1-\alpha_t) (q-t)(x-q) - cq\] solution \[q^t=(x+t-c/(1-\alpha_t))/2\]
• token share: \[\alpha_t=\frac{t}{c+t}\]

\(\alpha_tMR+t\)

\(q^t=q^m\)

Presell \(t\) tokens.

Collect enough funds to cover \(C_0\) + MC for the first \(t\) units.

As with equity, the entrepreneur receives the full NPV.

The entrepreneuer produces optimally at \(q^t=q^m\) (for any \(t\)).

If \(q^m<t\)  \(\Rightarrow\) redeem at rate \(t/q\) and tokenholders receive refund of \(c(t-q)\).

If monopoly quantity \(q^m>t\), then share \(\alpha_t\) of revenue from incremental \(q^m-t\) tokens with tokenholders

• costs her \(0\)
• \(\theta\sim U(0,\theta_l)\)
• \(\theta_l<\theta_h\)

• costs her \(C_e\)
• \(\theta\sim U(0,\theta_h)\)

common topic in corporate finance

very relevant in "decentralized" world where developers are scattered around the globe

also applicable to, e.g. established firms that do something new

assume \[\textit{NPV}(\text{effort})>0>\textit{NPV}(\text{no effort})\]

Investors (equity or token holders) only finance the project if the entrepreneur undertakes the effort

Solve for the optimal funding conditional on the entrepreneur taking the effort
 

Derive conditions such that the entrepreneur undertakes effort

1.

2.

possibly break even

cannot break even

entrepreneur

earns \((1-\alpha_s)\ \frac{(\theta_h-c)^3}{12\theta_h} -C_e \)

earns \((1-\alpha_s) \frac{(\theta_l-c)^3}{12\theta_l}\)

\(>\) ?

exert effort iff

\[\textit{NPV}_h-C_e\ge \textit{NPV}_h\times\frac{\theta_h}{\theta_l}\left(\frac{\theta_l-c}{\theta_h-c}\right)^3>0\]

demand \(\alpha_{s}\): \(\alpha_{s}\ \frac{(\theta_h-c)^3}{12\theta_h} =C_0 \)

token holders

possibly break even

cannot break even

entrepreneur

earns \(\frac{c}{c+t} \frac{2}{3\theta_h}\left(\frac{\theta_h-c}{2}-t \right)^3 -C_e \)

earns \(\frac{c}{c+t} \frac{2}{3\theta_l}\left(\frac{\theta_l-c}{2}-t \right)^3\)

\(>\) ?

exert effort iff

\[\textit{NPV}_h-C_e\ge \textit{NPV}_h\times\frac{\theta_h}{\theta_l}\left(\frac{\theta_l-c-2t}{\theta_h-c-2t}\right)^3\]

exert effort with equity financing iff

\[\textit{NPV}_h-C_e\ge \textit{NPV}_h\times\frac{\theta_h}{\theta_l}\left(\frac{\theta_l-c}{\theta_h-c}\right)^3>0\]

exert effort with token financing iff

\[\textit{NPV}_h-C_e\ge \textit{NPV}_h\times\frac{\theta_h}{\theta_l}\left(\frac{\theta_l-c-2t}{\theta_h-c-2t}\right)^3\]

Key insight: a token contract incentivizes effort better than equity (similarly to canonical debt vs. equity insights)

Optimal token contract has debt features:

    get nothing if demand is low (only original
    tokenholders get anything)
 

    benefit if demand is high

all projects that can be financed by equity can be financed by the optimal token contract but

some projects that can be financed by optimal tokens contracts cannot be financed by equity.