Link between hydrodynamics and quantum mechanics
Klas Modin
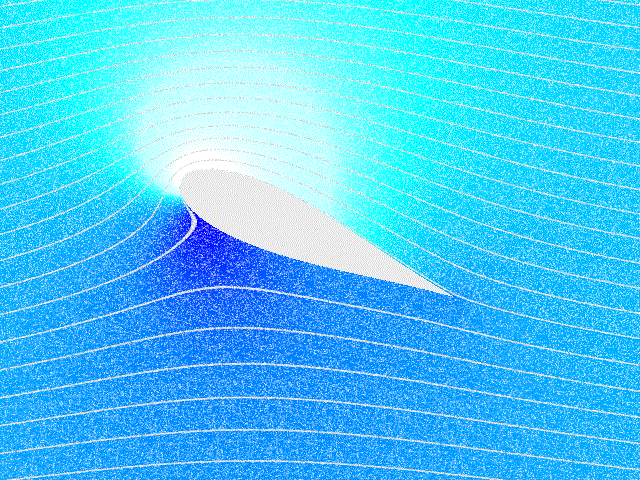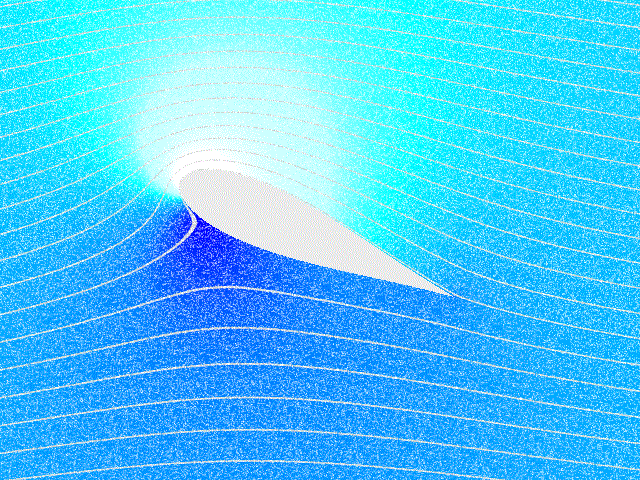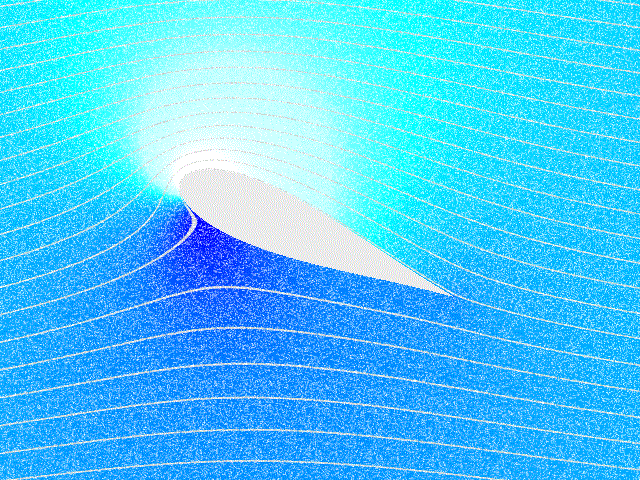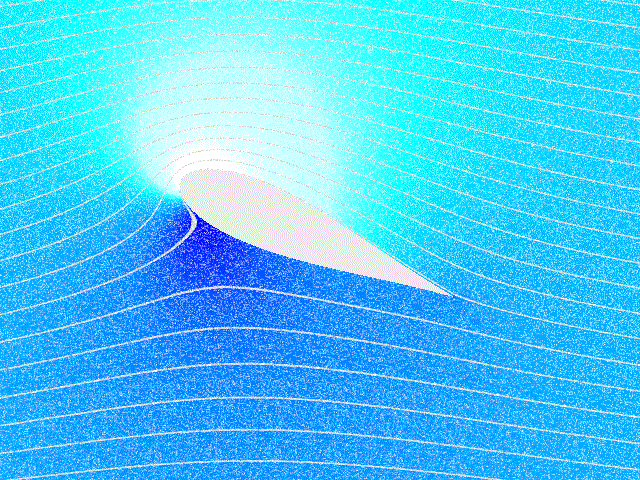
Objective: connection between Euler and Schrödinger equations
Leonhard Euler
1707 - 1783
Erwin Schrödinger
1887 - 1961
Why?
- Known since birth of QM, but often forgotten in modern textbooks
- Links to many active fields of mathematical research:
- Optimal transport
- Information theory
- PDE theory
- Stochastic differential equations
- etc.
- Illustrates "power" of geometry + physics
The Players
Player one: Euler fluid equations
\displaystyle\frac{\partial \mathbf v}{\partial t} + \mathbf v\cdot\nabla \mathbf v = -\nabla V-
incompressible
\displaystyle \frac{\partial \rho}{\partial t} + \mathrm{div}(\rho \mathbf v) = 0
P(\rho) = e'(\rho)\rho^2
\mathbf x
\mathbf v(\mathbf x)
\displaystyle \frac{1}{\rho}\nabla P(\rho)
\displaystyle \frac{1}{\rho}\nabla p
\displaystyle \mathrm{div}(\mathbf v) = 0
Pressure function:
\Omega
\displaystyle E(\mathbf v,\rho) = \frac{1}{2}\int_\Omega |\mathbf v|^2 \rho \, d\mathbf x + \int_\Omega e(\rho)\rho\,d \mathbf x
Total energy:
w(\rho) = e'(\rho)\rho+e(\rho)
Thermodynamic work:
\displaystyle \nabla w(\rho)
Potential function:
V = V(\mathbf x)
Player two: Schrödinger equation
\displaystyle\mathrm{i}\hbar\frac{\partial \psi}{\partial t} =\left[ \frac{-\hbar^2 }{2m}\Delta + V\right] \psi = 0
Wave function:
\psi: \Omega \to \mathbb C
Potential function:
V: \Omega \to \mathbb R
Conservation laws
Hamiltonian operator:
\displaystyle E(\psi) = \langle \psi,\hat H \psi \rangle_{L^2} = \int_\Omega (\frac{\hbar^2 \lvert\nabla\psi \rvert^2}{2m} + V\lvert\psi\rvert^2)d\mathbf x
\displaystyle \hat H = -\frac{\hbar^2}{2m}\Delta + V
Total energy:
Total probability:
\displaystyle 1=\langle \psi, \psi \rangle_{L^2} = \int_\Omega \lvert \psi\rvert^2 d\mathbf x
Connecting the dots
Euler equations
Schrödinger equation
Hamilton's equations on probabilities
Madelung transform
???
Special solution
Review of analytical mechanics
(Classical mechanics in the framework of differential geometry)
\displaystyle\dot{\mathbf{q}} =\frac{\partial H}{\partial \textbf p}
\displaystyle\dot{\mathbf{p}} =-\frac{\partial H}{\partial \mathbf q}
Properties
- Conservation of total energy \(H\)
- Conservation of phase space volume (symplecticity)
Evolves on phase space \(T^*M \simeq \mathbb{R}^{2n}\)
Examples:
Celestial mechanics
\(M=\mathbb{R}^{3N}\)
Rigid body
\(M = SO(3) \)
Hamiltonian dynamics on probability densities
Space of probability densities on \(\Omega\) :
\displaystyle P(\Omega) = \{ \rho \in C^\infty(\Omega)\mid \int_\Omega \rho\, d\mathbf x = 1, \; \rho > 0 \}
Now take as configuration manifold
\displaystyle M = P(\Omega) \;\Rightarrow\; T^* P(\Omega) = P(\Omega) \times C^\infty_0(\Omega)
Take as Hamiltonian
\displaystyle H(\rho,S) = \int_\Omega \left(\frac{\lvert\nabla S\rvert^2}{2m} + \frac{c}{4}\lvert\nabla\log(\rho)\rvert^2 + V\right)\rho\, d\mathbf x
Hamilton's equations on \(T^*P(\Omega)\)
\displaystyle\dot\rho = \frac{\delta H}{\delta S}
\displaystyle\dot S = -\frac{\delta H}{\delta \rho}
\(\Rightarrow\)
\displaystyle\dot\rho + \mathrm{div}(\rho\nabla S/m ) = 0
\displaystyle\dot S + \frac{\lvert\nabla S\rvert^2}{2m} - c \frac{\Delta\sqrt{\rho}}{\sqrt{\rho}} + V = 0
Apply gradient to second equation, yields:
\displaystyle\nabla\dot S + \frac{1}{m}\nabla S\cdot\nabla^2 S - c \nabla\frac{\Delta\sqrt{\rho}}{\sqrt{\rho}} + \nabla V = 0
Now set:
\displaystyle\mathbf v = \frac{1}{m}\nabla S
Which equation does \(\mathbf v\) fulfill?
We recover the Euler equations!
\displaystyle\dot\mathbf v + \mathbf v\cdot\nabla \mathbf v = -\nabla V -
\displaystyle\dot\rho + \mathrm{div}(\rho\mathbf v ) = 0
\displaystyle\mathbf v = \frac{1}{m}\nabla S
Thermodynamic work:
\displaystyle w(\rho) = -\frac{c\Delta\sqrt{\rho}}{m\sqrt{\rho}}
Internal energy:
\displaystyle e(\rho) = \frac{c}{4m}\lvert \nabla \log \rho \rvert^2
\surd
\nabla w(\rho)
Connecting the dots
Euler equations
Schrödinger equation
Hamilton's equations on probabilities
Madelung transform
Special solution
Madelung transform
Erwin Madelung
1881 - 1972
T^*P(\Omega) \to L^2(\Omega,\mathbb C)
(\rho,S) \mapsto \psi = \sqrt{\rho}\mathrm e^{\mathrm i S/\hbar}
\lvert\psi\rvert^2 = \rho
Notice that:
Key calculations for \( \psi = \sqrt{\rho}\mathrm e^{\mathrm i S/\hbar}\)
\displaystyle \dot\psi = \left(\frac{1}{2}\frac{\dot\rho}{\rho} + \frac{\mathrm i}{\hbar} \dot S\right)\psi
\displaystyle \nabla\psi = \left(\frac{\nabla\sqrt\rho}{\sqrt\rho} + \frac{\mathrm i}{\hbar} \nabla S\right)\psi
\displaystyle \Delta\psi = \left(\frac{\nabla\sqrt\rho}{\sqrt\rho} + \frac{\mathrm i}{\hbar} \nabla S\right)^2\psi + \left(\nabla\cdot\frac{\nabla\sqrt\rho}{\sqrt\rho} + \frac{\mathrm i}{\hbar} \Delta S\right)\psi
\displaystyle = \left(\frac{\Delta\sqrt\rho}{\sqrt\rho} - \frac{1}{\hbar^2}\lvert\nabla S\rvert^2 + \frac{\mathrm i }{\hbar} \frac{\mathrm{div}(\rho\nabla S)}{\rho} \right)\psi
\displaystyle \mathrm{i}\hbar\dot\psi = \left(- \dot S + \frac{\mathrm{i\hbar}}{2}\frac{\dot\rho}{\rho} \right)\psi
\displaystyle \left( - \frac{\hbar^2}{2m}\frac{\Delta\sqrt\rho}{\sqrt\rho} + \frac{\lvert\nabla S\rvert^2}{2m} + V - \frac{\mathrm i \hbar}{2m} \frac{\mathrm{div}(\rho\nabla S)}{\rho} \right)\psi
\displaystyle\mathrm{i}\hbar\dot\psi =\left[ \frac{-\hbar^2 }{2m}\Delta + V\right] \psi = 0
Left-hand side
Right-hand side
\displaystyle \frac{-\hbar^2 }{2m}\Delta\psi + V\psi =
Schrödinger equation
\displaystyle\dot\rho + \mathrm{div}(\rho\nabla S/m ) = 0
\displaystyle\dot S + \frac{\lvert\nabla S\rvert^2}{2m} - \frac{\hbar^2}{2m} \frac{\Delta\sqrt{\rho}}{\sqrt{\rho}} + V = 0
\displaystyle c= \frac{\hbar^2}{2m}
\surd
Connecting the dots
Euler equations
Schrödinger equation
Hamilton's equations on probabilities
Madelung transform
Special solution
Summary
- Link between compressible Euler equations (with "strange" internal energy) and Schrödinger equation
- Schrödinger equation is a classical Hamiltonian system on the infinite-dimensional space of probability densities
Further reading about the geometry of the Madelung transform: