Klas Modin

What is shape analysis?

Illustrating example

Source

Target

Q: What is a 'shape' ?

A: Anything diffeomorphisms act on

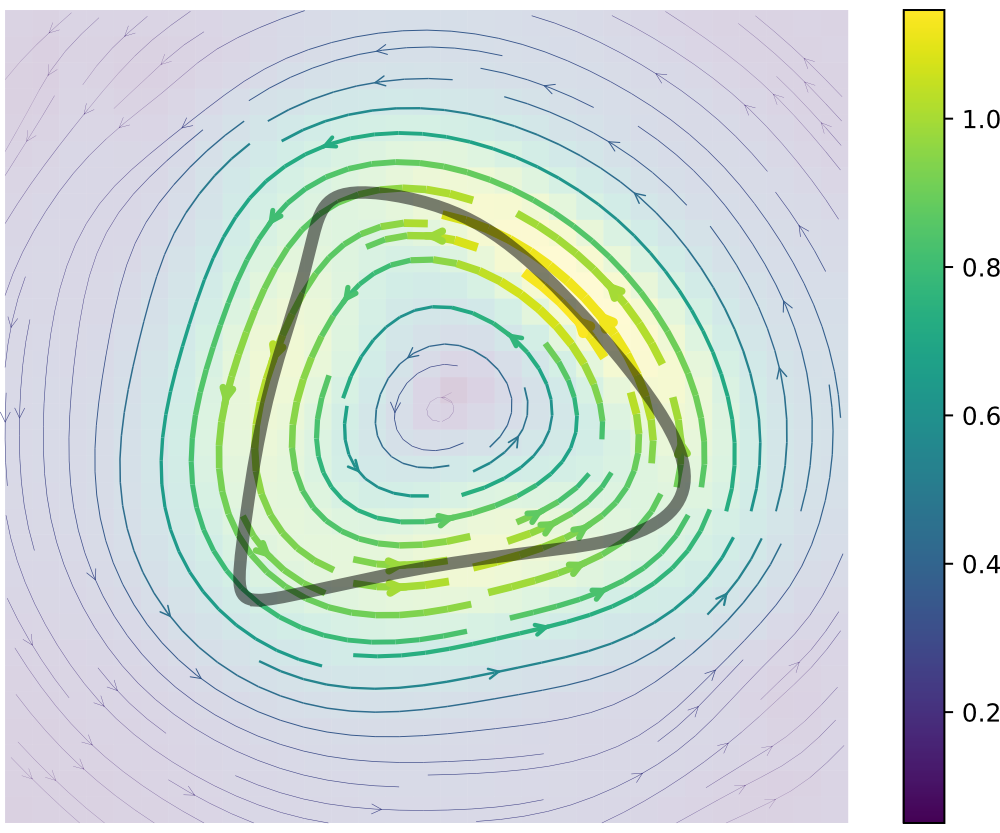
Basic idea

  • Riemannian geometry on
    manifold of diffeomorphisms
     
  • Variational problem \(\Rightarrow\) PDEs

Distance between shapes =

shortest diffeomorphic warp

from source to target

History of shape analysis

Shape

analysis

Optimal transport

Information theory

Hydrodynamics

Non-linear Schrödinger eq.

Probability theory

Shallow water equations

Numerical analysis of PDE

Fluids and optimal transport

\mathrm{Diff}(M)
\mathrm{P}(M)
\mathrm{Id}
\mu
\nu
\pi(\varphi)=\varphi_*\mu

Riemannian metric

\displaystyle\mathcal{G}_\varphi(\dot\varphi,\dot\varphi) = \int_{M}\left\vert \dot\varphi \right\vert^2 \mu

Induces metric

\overline{\mathcal{G}}_\mu(\dot\mu,\dot\mu) \Rightarrow d_W^2(\mu_0,\mu_1)
\mathrm{Hor}

[Arnold (1966), Benamou & Brenier (2000), Otto (2001)]

Invariance: \(\eta\in\mathrm{Diff}_{\mu}(M)\)

\displaystyle\mathcal{G}_\varphi(\dot\varphi,\dot\varphi) = \mathcal{G}_{\varphi\circ\eta}(\dot\varphi\circ\eta,\dot\varphi\circ\eta)

Exactly \(L^2\)-Wasserstein distance

\mathrm{Diff}_\mu(M)
\mathrm{Ver}

Geodesic equation on \(T\mathrm{Diff}_\mu/\mathrm{Diff}_\mu \simeq \mathcal{X}_{\mu}\)
\(\dot v + \nabla_v v = -\nabla p\)

Geodesic equation on \(T^*(\mathrm{Diff}/\mathrm{Diff}_\mu) \simeq T^*\mathrm{P}\)
\(\dot S + \frac{1}{2}|\nabla S| = 0 \quad \dot\rho + \mathrm{div}(\rho\nabla S) = 0\)

\(v=\nabla S\)