Aristotle (450 BCE):

Aristotle's cosmology attempted to explain why objects move

Natural Motion - Proceeds from the nature of the object, proportional to its weight.

Violent Motion - Imposed motion resulting from pushing or pulling.

All matter is continuous and composed of 4 basic elements (air, water, earth, fire).

Celestial bodies are made out of quintessence (the fifth essence) and follow different rules. Earth is at rest at the center of the solar system (geocentric model).

Galileo (1564-1642):

Galileo challenged Aristotle's cosmology after being accepted for over 2000 years

If there is no interference with a moving object it will keep moving in a straight line forever.

Objects fall at the same speed regardless of their weight.

Copernicus heliocentric model of the solar system is right. The Earth moves!

Galileo (1564-1642):

Galileo introduced the concept of inertia

If there is no interference with a moving object it will keep moving in a straight line forever.

Inertia is the tendency of an object to resists changes in motion

Inertia

The tendency of an object to resists changes in motion

Is a property of matter
Is proportional to the amount of matter (mass)

Newton (N) is a unit of Force

1 Kg has a weight of 9.8 N on Earth

1 N = 1 \ kg\frac{m}{s^2}

1 N = 1 \ kg\frac{m}{s^2}

1 Kg of mass is pulled down by the gravity of Earth with a force of 9.8 Newtons.

A more proper definition will come after we see Newton's 2nd law

Mass/Inertia vs. Weight

1. Pull slowly on the string -- what happens?

2. Pull quickly on the string -- what happens?

Newton's First Law of Motion

Newton refined Galileo's idea and made it his first law, appropriately called the law of inertia

Every object continues in a state of rest or of uniform speed in a straight line unless acted on by a nonzero net force.

Net Force & Vectors

Any quantity that requires both magnitude an direction for a complete description is a vector quantity

Force is a vector quantity. When more than a single force acts on an object , we consider the net force

Support Force, A.K.A Normal Force

Atoms on the push back up like a spring!

\sum{F} = 0

\sum{F} = 0

When you stand on two bathroom scales, with one foot on each scale and weight evenly distributed, each scale will read

A. Your weight

B. Half your weight

C. Zero

D. Actually more than your weight

Checkpoint

The Equilibrium Rule

When the net force is equal to zero there is not change in motion - a state of rest or constant velocity is maintained

\sum{F} = F_1 + F_2 + ... = 0

\sum{F} = F_1 + F_2 + ... = 0

What is the tension force on the right cable?

Checkpoint

T_left + T_right - 500 N - 400 N - 400 N = 0 N

T_right = 500 N + 400 N + 400 N - T_left = 1300 N - 800 N = 500 N

\sum{F} = F_1 + F_2 + ... = 0

\sum{F} = F_1 + F_2 + ... = 0

\sum{F} =

\sum{F} =

The Equilibrium Rule at constant velocity (dynamic equilibrium)

When the net force is equal to zero there is not change in motion - a state of rest or constant velocity is maintained

\sum{F} = 0

\sum{F} = 0

\sum{F} = F_{\mathrm{lift}}+F_{\mathrm{gravity}}+F_{\mathrm{propellers}}+F_{\mathrm{wind}}=0

\sum{F} = F_{\mathrm{lift}}+F_{\mathrm{gravity}}+F_{\mathrm{propellers}}+F_{\mathrm{wind}}=0

F_{\mathrm{propellers}}

F_{\mathrm{propellers}}

F_{\mathrm{gravity}}

F_{\mathrm{gravity}}

F_{\mathrm{wind}}

F_{\mathrm{wind}}

F_{\mathrm{lift}}

F_{\mathrm{lift}}

When Nellie pushes a crate across a factory floor at constant speed, the force of friction between the crate and the floor is?

A. Less than Nellie's push

B. Equal to Nellie's push

C. Equal and opposite to Nellie's push

D. More than Nellie's push

Checkpoint

Friction

The Equilibrium Rule

When the net force is equal to zero there is not change in motion - a state of rest or constant velocity is maintained

\sum{F} = 0

\sum{F} = 0

Newtown's 2nd law

Newtons 2nd Law

Forces cause acceleration

Inetertia resists acceleration

and

\mathrm{Acceleration} = \frac{\mathrm{Net \ Force}}{\mathrm{mass}}

\mathrm{Acceleration} = \frac{\mathrm{Net \ Force}}{\mathrm{mass}}

\mathrm{Acceleration} \propto \mathrm{Net \ Force}

\mathrm{Acceleration} \propto \mathrm{Net \ Force}

\mathrm{Acceleration} \propto \frac{1}{\mathrm{mass}}

\mathrm{Acceleration} \propto \frac{1}{\mathrm{mass}}

Newtons 2nd Law

Acceleration is inversely proportional to mass

\mathrm{Acceleration} \propto \frac{\mathrm{1}}{\mathrm{mass}}

\mathrm{Acceleration} \propto \frac{\mathrm{1}}{\mathrm{mass}}

\mathrm{Acceleration} = \frac{\mathrm{Net \ Force}}{\mathrm{mass}}

\mathrm{Acceleration} = \frac{\mathrm{Net \ Force}}{\mathrm{mass}}

Free falling objects experience the same acceleration regardless their mass

This is because:

\mathrm{Force \ of \ gravity} \propto \mathrm{mass}

\mathrm{Force \ of \ gravity} \propto \mathrm{mass}

\mathrm{Acceleration} \propto \frac{1}{\mathrm{mass}}

\mathrm{Acceleration} \propto \frac{1}{\mathrm{mass}}

but

So the mass cancels out:

\mathrm{Acceleration} = \frac{g m}{m}

\mathrm{Acceleration} = \frac{g m}{m}

Weight (force due to gravity)

Force = mass x acceleration

Weight = mass x grav. acceleration

Weight = mass x g

On the surface of Earth

where g = 9.8 m/s^2

g is the gravitational acceleration of all objects near the Earth's surface

F_g = mg

F_g = mg

Definition of a Newton

1 kg has a weight of 9.8 N on Earth

9.8 \ N = 1 \ kg \times 9.8 \ \frac{m}{s^2}

9.8 \ N = 1 \ kg \times 9.8 \ \frac{m}{s^2}

\mathrm{Force} = \mathrm{mass} \times \mathrm{acceleration}

\mathrm{Force} = \mathrm{mass} \times \mathrm{acceleration}

\Rightarrow 1 N = 1 \ kg \ \frac{m}{s^2}

\Rightarrow 1 N = 1 \ kg \ \frac{m}{s^2}

\mathrm{Force} = \mathrm{weight}= 9.8 N, \ \mathrm{mass} = 1 \ kg, \ a = g = 9.8 \ \frac{m}{s^2}

\mathrm{Force} = \mathrm{weight}= 9.8 N, \ \mathrm{mass} = 1 \ kg, \ a = g = 9.8 \ \frac{m}{s^2}

Newtown's 3rd Law

Forces and Interactions

If you push it pushes you back!

Newtons 3rd Law

To every action there is always an opposed equal reaction

Whenever an object exerts a force on a second object, the second object exerts an equal an opposite force to the first

Or in other words

Newtons 3rd Law: For every action there is a reaction

Force is the same, acceleration is different

acceleration is what causes the damage

Action and reaction of different masses

M

m

a = \frac{F}{m}

a = \frac{F}{m}

Summary of Newton's Laws

1st - Law of Inertia: Every object continues in a state of rest or uniform speed in a straight line unless acted on by a nonzero net force.

2nd - Acceleration is caused by a not zero net force: The acceleration of an object is directly proportional to the net force acting on a object, is in the direction of the net force, and inversely proportional to the mass of the object.

3rd - For every action there is a reaction: To every action there is always an opposed equal reaction.

a = \frac{F}{m}

a = \frac{F}{m}

My Short Summary of Newton's Laws

For every action there is a reaction: To every action there is always an opposed equal reaction.

a = \frac{F}{m}

a = \frac{F}{m}

Problem Solving with Newton's Laws

Problem Solving Procedure

Draw a free-body diagram showing all of the forces in the system of interest.
Determine the Net Force along each orthogonal dimension (e.g. x, y).
Use Newton's 2nd Law (a = F/m) to find the acceleration, the force or the mass.
If asked, use the equations of motion to find the velocity or position given the acceleration. Treat each dimension independently.

x = \frac{a}{2} \ t^2 + v_0 \ t + x_0

x = \frac{a}{2} \ t^2 + v_0 \ t + x_0

v = a \ t + v_0

v = a \ t + v_0

a = \frac{F}{m}

a = \frac{F}{m}

Equations you may need:

System of Interest

Type of Forces

Weight (W) & Normal Force (N)

W = mg

W = mg

Type of Forces

Friction (f)

f \propto N

f \propto N

f = \mu N

f = \mu N

Type of Forces

Tension (T)

Problem Solving Examples

Fill the magnitude of the forces

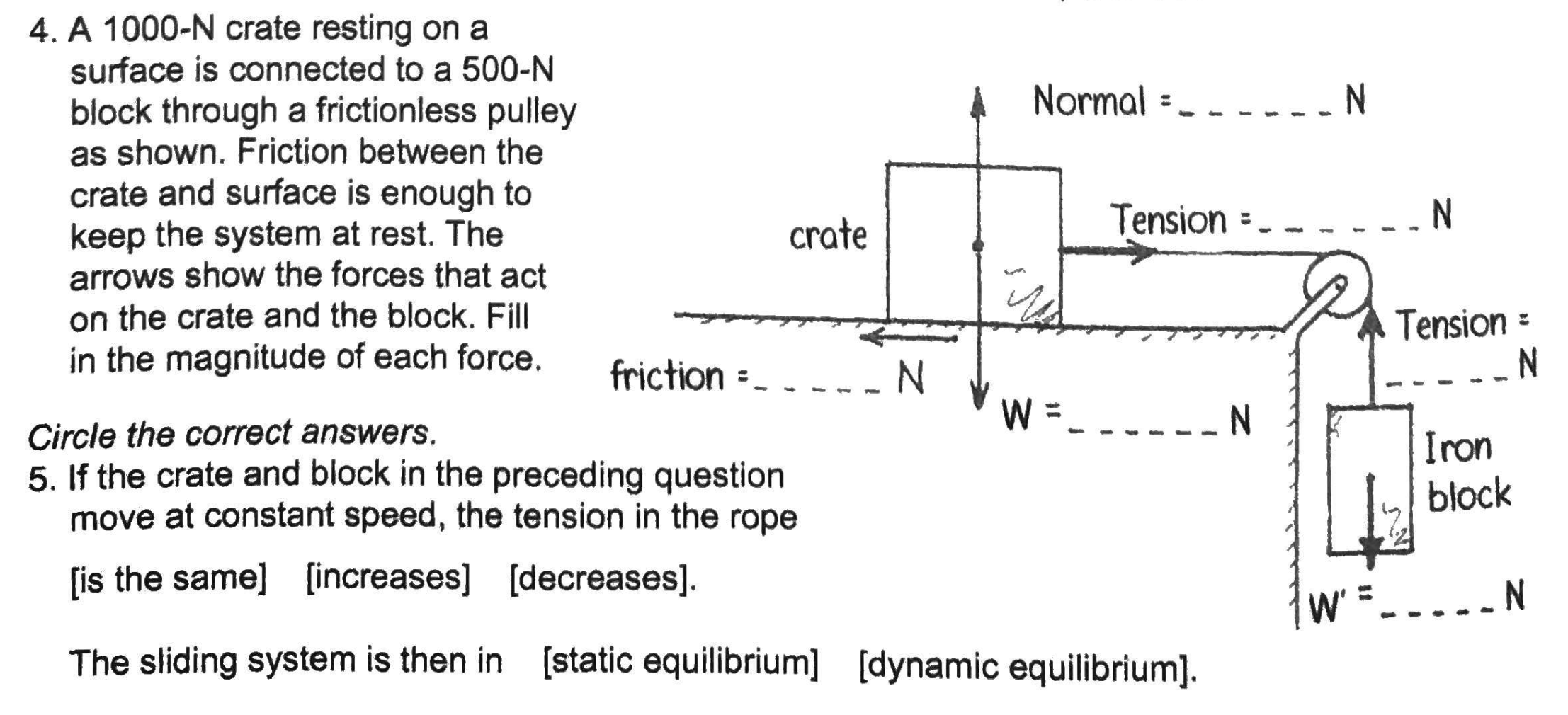
1000

500

1000

What is the friction force if the box is not moving?

What is the velocity at the bottom of the ramp (x = 0 m) if starts from rest (Vo = 0 m/s)?

A 76.0-kg person is being pulled away from a burning building. Calculate the tension in the two ropes if the person is momentarily motionless.

The End

Where does the mass of particles come from?

Isaac Newton

1642 - 1726

Isaac Newton was an English mathematician, astronomer, and physicist (described in his own day as a "natural philosopher") who is widely recognized as one of the most influential scientists of all time and a key figure in the scientific revolution. His book Philosophiæ Naturalis Principia Mathematica ("Mathematical Principles of Natural Philosophy"), first published in 1687, laid the foundations of classical mechanics. Newton also made pathbreaking contributions to optics, and he shares credit with Gottfried Wilhelm Leibniz for developing the infinitesimal calculus.

Inertia

If Galileo and Copernicus are right and the Earth moves, why if you jump next to a wall you don't get slammed by the wall moving at 30 km/s?

Motion is Relative

Are we moving right now?

Relative to what?

Relative to the center of the earth we are moving at 0.33 Km/s

Relative to the sun we are moving at 30 Km/s

Relative to the center of our Galaxy we are moving at 250 Km/s

Newton's Laws of Motion