Dual-symmetric Electromagnetism:
A Clifford-algebraic approach

Justin Dressel


Schmid College of Science and Technology

Institute for Quantum Studies

Chapman University

 

Advances in Operator Theory
with Applications to Mathematical Physics
November 12-16, 2018, Chapman U, Orange CA

 

Physical Assumption : Minkowski Metric

  • Events can be locally described by a Minkowski pseudo-metric space (+,-,-,-)
     
    • Local velocities (tangent vectors of curves that connect events) must be 4-vectors that obey this metric, according to observed Lorentz symmetries
       
    • Connecting locally flat tangent spaces constructs an event-history manifold
       
  • Idea : Construct associative algebra that encodes the metric as a vector contraction
     
    • Yields: (orthogonal) Clifford algebra  \(\text{Cl}_{1,3}(\mathbb{R})\)   ("Spacetime algebra")
      as full geometric tangent space of the event-history manifold
       
      • Nested Subalgebras (with natural physical meaning):

                   Clifford algebra       \(\text{Cl}_{3,0}(\mathbb{R})\)                  (3D space relative to reference frame)
                   Quaternions            \(\mathbb{H}\)                             (Rotation planes in 3D space)
                   Complex numbers  \(\mathbb{C}\)                             (Single rotation plane)
                   Real numbers          \(\mathbb{R}\)                             (Measurable quantity)

Constructing Spacetime Algebra

\text{Basis: } \gamma_0,\,\gamma_1,\,\gamma_2,\,\gamma_3

Minkowski Space \(\mathcal{M}_{1,3}(\mathbb{R})\) :

\displaystyle \begin{aligned}\eta(a+b,\,a+b) &= (a+b)^2 = a^2 + b^2 + ab + ba \\ &= \eta(a,a) + \eta(b,b) + 2\eta(a,b) \\ \implies& \eta(a,b) \equiv \frac{ab + ba}{2} \equiv a\cdot b \end{aligned}
\gamma_0 \cdot \gamma_0 = 1
\gamma_k \cdot \gamma_k = -1
\gamma_\mu \cdot \gamma_\nu = \eta_{\mu\nu} = \eta(\gamma_\mu,\,\gamma_\nu)

Minkowski metric
(symmetric bilinear form)

Algebraic Axioms:   \(a,b,c\in\mathcal{M}_{1,3}(\mathbb{R})\)

  1. Associativity
      \(a(bc) = (ab)c\)
     
  2. Left Distributivity
      \(a(b+c) = ab + ac\)
     
  3. Right Distributivity
      \((b+c)a = ba + ca\)
     
  4. Contraction
      \(a^2 = aa = \eta(a,\,a)\)

Axioms constructively define Clifford algebra \( \text{Cl}_{1,3}(\mathbb{R}) \)

\displaystyle ab = \frac{ab + ba}{2} + \frac{ab - ba}{2} \equiv a\cdot b + a\wedge b

Symmetric part of product encodes metric:

Anti-symmetric part is Grassman wedge product:

(others zero)

Graded Clifford Basis

  • Basis properties:
\gamma_0\gamma_1\gamma_2\gamma_3
\gamma_1\gamma_0 \quad \gamma_2\gamma_0 \quad \gamma_3\gamma_0 \quad | \quad \gamma_1\gamma_2 \quad \gamma_2\gamma_3 \quad \gamma_3\gamma_1
\gamma_0 \quad | \quad \gamma_1 \quad \gamma_2 \quad \gamma_3
1

Grade

0

1

2

3

\gamma_\mu\gamma_\nu = \begin{cases}-\gamma_\nu\gamma_\mu, & \mu\neq\nu \\ \eta_{\mu\nu}, & \mu = \nu \end{cases}

4

\gamma_1\gamma_2\gamma_3 \quad | \quad \gamma_0\gamma_2\gamma_3 \quad \gamma_0\gamma_1\gamma_3 \quad \gamma_0\gamma_1\gamma_2
2^4 = 16\;\text{elements}

Bivector Planes (of rotation):

3 hyperbolic | 3 elliptic

Vector Lines (of translation):

1 timelike | 3 spacelike

Scalar Points

Pseudovector Volumes:

1 spacelike | 3 timelike

Pseudoscalar 4-Volumes

Multivector:    \( M = \langle M \rangle_0 + \langle M \rangle_1 + \langle M \rangle_2 + \langle M \rangle_3 + \langle M \rangle_4 \)

  • Reciprocal basis:
\gamma^\mu \equiv (\gamma_\mu)^{-1} = \gamma_\mu / (\gamma_\mu)^2

Notational Simplifications

I
\vec{\sigma}_1 \quad \vec{\sigma}_2 \quad \vec{\sigma}_3 \quad | \quad I\vec{\sigma}_1 \quad I\vec{\sigma}_2 \quad I\vec{\sigma}_3
\gamma_0 \quad | \quad \gamma_1 \quad \gamma_2 \quad \gamma_3
1

0

1

2

3

I \equiv \gamma_0\gamma_1\gamma_2\gamma_3 \equiv \vec{\sigma}_1\vec{\sigma}_2\vec{\sigma}_3

(Spacetime-aware "imaginary unit" is the unit pseudoscalar: \(I^2 = -1\))

(commutes with even grade, anti-commutes with odd grade)

4

I\gamma_0 \quad | \quad I\gamma_1 \quad I\gamma_2 \quad I\gamma_3

Relative 3D space embedded as even-graded subspace:
\( \text{Cl}_{3,0}(\mathbb{R}) \)

 

Complexified 3-vector algebra is equivalent to this relative 3D Clifford algebra since \(I\) commutes with even grades

\vec{\sigma}_k \equiv \gamma_k\gamma_0 = \gamma_k \wedge \gamma_0

(Observed spatial directions in reference frame)
(Space axis dragged along temporal worldline)

Note duality transformation of left multiplication by \(-I\) : Hodge star operation

Multivector:    \( M = [\alpha + (v_0 + \vec{v})\gamma_0 + \vec{A}] + I[\vec{B} + (w_0 + \vec{w})\gamma_0 + \beta] \)

Complex Structure & Spacetime Splits

Proper Form of Multivector:   

\( M = \alpha + v + \mathbf{F} + Iw + I\beta = (\alpha + I\beta) + (v + Iw) + \mathbf{F} \)

 

 

Relative Frame Form of Multivector:

\( M = \alpha + (v_0 + \vec{v})\gamma_0 + (\vec{A} + I\vec{B}) + I(w_0 + \vec{w})\gamma_0 + I\beta \)

 

 

Components:

complex scalar

complex vector

bivector

scalar

polar paravector

polar vector

axial vector

axial paravector

pseudoscalar

4-vector

scalar

bivector

pseudovector

pseudoscalar

v = \sum_\mu v^\mu\gamma_\mu = \sum_\mu v_\mu \gamma^\mu = v_0 \gamma^0 + \sum_k v^k\vec{\sigma}_k\gamma_0
\mathbf{F} = \sum_{\mu,\nu} F^{\mu\nu}\gamma_\mu\gamma_\nu = \sum_{\mu,\nu} F_{\mu\nu} \gamma^\mu\gamma^\nu = \sum_k A^k\vec{\sigma}_k + I\sum_k B^k\vec{\sigma}_k

4-vector components, or a scalar and a relative 3-vector

rank-2 antisymmetric tensor components, or a pair of 3-vectors (one polar, one axial)

Fields and Derivatives

Multivector field:   (in tangent algebra at point \(x\) of manifold)

\( M(x) = \alpha(x) + v(x) + \mathbf{F}(x) + Iw(x) + I\beta(x) \)

 

Vector derivative (Dirac operator):

 

 

 

\( \displaystyle \vec{\nabla} \equiv \sum_k \vec{\sigma}^k \frac{\partial}{\partial x^k} \)  ;  \( \vec{\nabla}\vec{v} = \vec{\nabla}\cdot\vec{v} + \vec{\nabla} \wedge \vec{v} = \vec{\nabla}\cdot\vec{v} + (\vec{\nabla}\times \vec{v})I \)    (contains divergence and curl)

 

\(\nabla^2 = \nabla\gamma_0^2\nabla = (\partial_{ct} - \vec{\nabla})(\partial_{ct} + \vec{\nabla}) = \partial^2_{ct} - \vec{\nabla}\cdot\vec{\nabla} = \Box \)     (d'Alembertian)

\displaystyle \nabla \equiv \sum_\mu \gamma^\mu \frac{\partial}{\partial x^\mu} = \gamma_0 \left[\frac{\partial}{\partial (ct)} + \vec{\nabla} \right] = \left[ \frac{\partial}{\partial (ct)} - \vec{\nabla} \right] \gamma_0

Physical Grade Examples

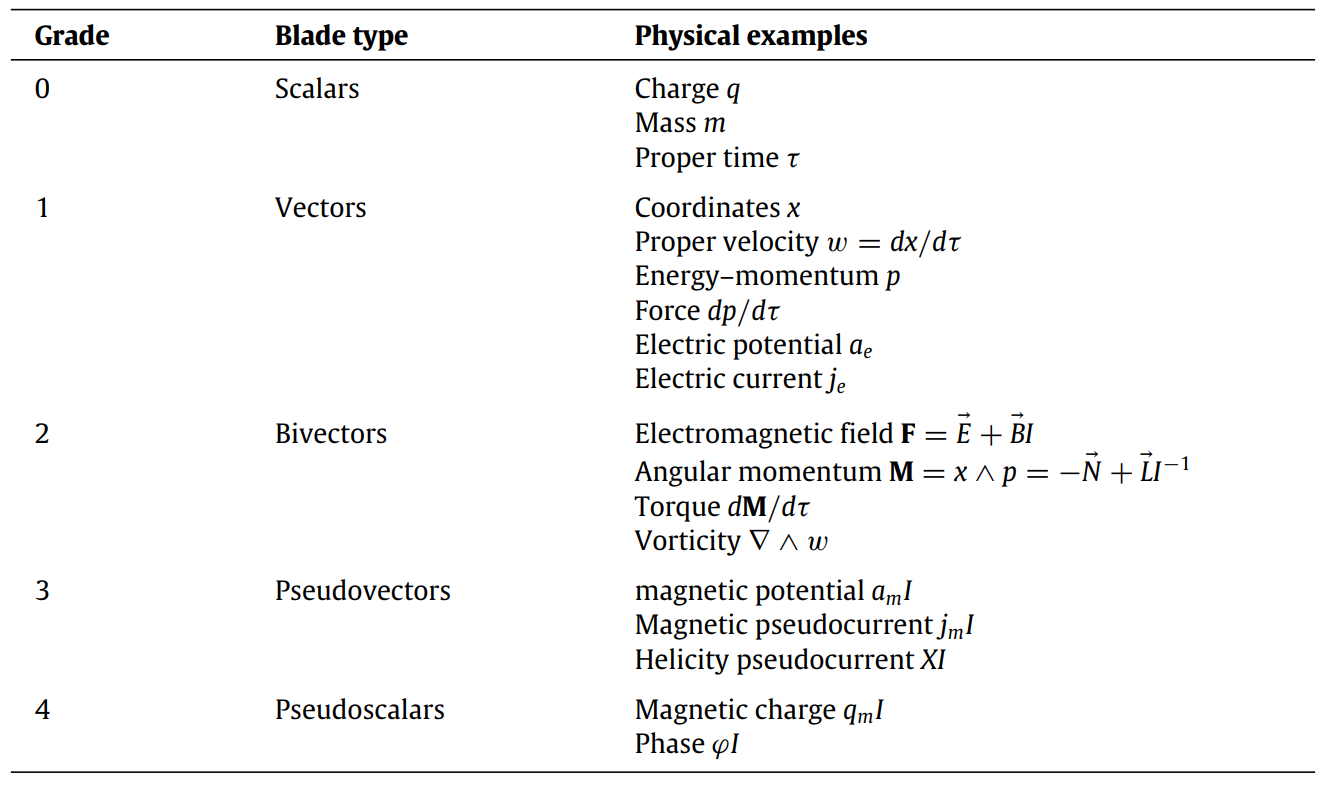
EM is naturally a bivector field

Maxwell's Equation

\mathbf{F}(x) = \sqrt{\epsilon_0}\left[\sum_{k=1}^3 E^k(x)\gamma_k\gamma_0 + Ic\sum_{k=1}^3 B^k(x) \gamma_k\gamma_0\right] = \sqrt{\epsilon_0}\vec{E}(x)+I\vec{B}(x)/\sqrt{\mu_0}
\nabla \mathbf{F} = j
j = \sum_\mu j^\mu_e \gamma_\mu + \sum_\mu j^{\mu}_{m} \gamma_\mu I = j_e + j_m I = \sqrt{\epsilon_0}(\rho_e + \vec{J}_e/c)\gamma_0 + \sqrt{\mu_0}(\rho_m + \vec{J}_m/c)\gamma_0 I

electric charge
density and current

This is Maxwell's Equation

\nabla F = \gamma_0(\vec{\nabla}\cdot\vec{E} + \partial_{ct}\vec{E}- \vec{\nabla}\times\vec{B}) + \gamma_0(\vec{\nabla}\cdot\vec{B} + \partial_{ct}\vec{B} + \vec{\nabla}\times\vec{E})I = \gamma_0(\rho_e - \vec{J}_e) + \gamma_0 (\rho_m - \vec{J}_m)I = j

Electromagnetic field bivector:

Same form as complex (Riemann-Silberstein) 3-vector

Electromagnetic source complex vector:

magnetic charge
density and current

Contains all of the usual 3D Maxwell's Equations, permitting both electric and magnetic charges

Canonical Field Decomposition and Phase

\mathbf{F}(x) = \sqrt{\epsilon_0}\vec{E}(x)+I\vec{B}(x)/\sqrt{\mu_0} = \mathbf{f}(x)\,e^{I\theta(x)/2}
\mathbf{f}^2 = |\mathbf{F}|^2 \geq 0
\mathbf{F}^2 = \left[\epsilon_0|\vec{E}|^2 - |\vec{B}|^2/\mu_0\right] + 2\sqrt{\epsilon_0/\mu_0}\,(\vec{E}\cdot\vec{B})I \equiv \ell_1 + \ell_2 I = |\mathbf{f}|^2 e^{I\theta}

Usual Lagrangian density for field

"axion" contribution to Lagrangian

Only possible Lorentz-invariant scalars

\mathbf{f}^2 = |\mathbf{F}|^2 = \sqrt{|\ell_1|^2 + |\ell_2|^2}
\displaystyle \theta = \tan^{-1}\frac{\ell_2}{\ell_1}

Note that a global phase degeneracy appears for a "null field" with zero magnitude

Null Solutions : Electromagnetic Waves

\mathbf{F}(x) = \sqrt{\epsilon_0}\vec{E}(x)+I\vec{B}(x)/\sqrt{\mu_0} = \mathbf{f}(x)\,e^{I\theta(x)/2}
\mathbf{f}^2 = |\mathbf{F}|^2 = 0

Even though null fields have global phase degeneracy, their local phases must be connected in order to satisfy Maxwell's equation, which breaks the phase symmetry

\mathbf{F}(x) = \mathbf{f}\,\exp[I\phi(x)]

Consider field with constant amplitude:

\nabla\mathbf{F} = 0 \implies [\nabla\phi]\mathbf{f} = 0

For phase and amplitude to both be nonzero, they must be noninvertible and therefore null

Since \(\mathbf{f}\) is constant, \(\nabla \phi\) must also be constant, which forces the linear solution :
\(\phi(x) = \phi_0 \pm k\cdot x \) with null wavevector \( k = \nabla \phi \),
as well as the decomposition \(\mathbf{f} = s\wedge k = -k\wedge s \) for some constant spacelike vector \(s\) s.t. \(s\cdot k = 0\)

Since the global phase is arbitrary, we choose \(\phi_0 = 0\) to find the plane-wave solutions:

    \( \mathbf{F}(x) = sk\,e^{\pm I k\cdot x} \)

 

Writing out the directional null factor \(k = (\omega/c)\gamma_0(1 - \vec{k}/|\vec{k}|)\) and \(s = \vec{E}\gamma_0 \), this solution expands to:

    \( \mathbf{F}(t,\vec{x}) = [\vec{E} \cos(\vec{k}\cdot\vec{x} - \omega t) \mp \hat{k}\times\vec{E} \sin(\vec{k}\cdot\vec{x} - \omega t)] + [\vec{E} \sin(\vec{k}\cdot\vec{x}-\omega t) \pm \hat{k}\times\vec{E} \cos(\vec{k}\cdot{x} - \omega t)]I \)

These are circularly polarized (constant helicity) electromagnetic plane wave modes.

Dual-symmetry : Electric-Magnetic Egalitarianism

\mathbf{F}(x) = \mathbf{f}(x)\,e^{I\theta(x)/2} = \sqrt{\epsilon_0}\vec{E}(x)+I\vec{B}(x)/\sqrt{\mu_0}
\nabla \mathbf{F} = j
j = j_e + j_m I = \sqrt{\epsilon_0}(\rho_e + \vec{J}_e/c)\gamma_0 + \sqrt{\mu_0}(\rho_m + \vec{J}_m/c)\gamma_0 I

\(\{\)

Complete EM dynamics:

A hidden gauge symmetry is now evident:

e^{-I\phi/2}\nabla \mathbf{F}e^{I\phi/2} = e^{-I\phi/2}je^{I\phi/2}

The pseudoscalar \(I\) generates phase rotations in the usual way:

this has the effect of swapping the electromagnetic fields and source charges

e^{-I\phi/2}\nabla \mathbf{F}e^{I\phi/2} = \nabla\left[e^{I\phi/2}\mathbf{F}e^{I\phi/2}\right] = \nabla \left[\mathbf{F}e^{I\phi}\right] = \nabla \left[ (\cos\phi\,\vec{E} - \sin\phi\,\vec{B}) + (\sin\phi\,\vec{E} + \cos\phi\,\vec{B})I\right] = \nabla(\vec{E}' + \vec{B}'I)
e^{-I\phi/2}je^{I\phi/2} = je^{I\phi} = (\cos\phi\,j_e - \sin\phi\,j_m) + (\sin\phi\,j_e + \cos\phi\,j_m)I = j_e' + j_m'I

Physics is the same provided that both source and field definitions are changed in tandem

Can always choose \(\phi\) to make all charges electric (\(j'_m = 0\))

Vacuum fields (\(j = 0\)) always have this field-exchange symmetry

Electromagnetic Vector Potentials

\mathbf{F}(x) = \nabla z(x)

Postulate vector potential:

z(x) = a_e(x) + a_m(x)I

Must be complex field to satisfy dual symmetry

\mathbf{F} = \mathbf{F}_e + \mathbf{F}_m I = \nabla \wedge a_e + (\nabla\wedge a_m)I
0 = \nabla \cdot a_e = \nabla\cdot a_m

Lorenz-Fitzgerald conditions assumed

\mathbf{F} = \vec{E} + \vec{B}I ; \qquad a_e = (\phi_e + \vec{A})\gamma_0 ; \qquad a_m = (\phi_m + \vec{C})\gamma_0
\vec{E} = -\vec{\nabla}\phi_e - \partial_{ct}\vec{A} - \vec{\nabla}\times\vec{C}
\vec{B} = -\vec{\nabla}\phi_m - \partial_{ct}\vec{C} + \vec{\nabla}\times\vec{A}

Relative EM fields regain apparent symmetry in definitions

\implies \nabla\mathbf{F}(x) = \nabla^2 z(x) = j(x)

Obvious wave equation

Lorentz Force

\displaystyle \frac{dp}{d\tau} = \langle\mathbf{F}j\rangle_0 = q_e\,\mathbf{F}\cdot w + q_m (\mathbf{F}\wedge w)I

Proper force law:

p \equiv m w \qquad j \equiv q w \qquad q \equiv q_0\,e^{I\theta} = q_e + q_m I

Charge must be complex to admit dual symmetry

\displaystyle w \equiv \frac{dx}{d\tau} = \gamma(c + \vec{v})\gamma_0 \qquad w^2 = c^2 \qquad \gamma \equiv (1 - |\vec{v}/c|^2)^{-1/2}
\displaystyle \gamma c \equiv \frac{dt}{d\tau} = w\cdot\gamma_0 \qquad \gamma\vec{v} \equiv \frac{d\vec{x}}{d\tau} = w\wedge\gamma_0
\displaystyle \frac{dp}{d\tau}\frac{d\tau}{dt}\gamma_0 = \frac{d\mathcal{E}}{d(ct)} + \frac{d\vec{p}}{dt} \qquad \mathcal{E} \equiv \gamma mc^2 \qquad \vec{p} = m\vec{v} \qquad \frac{\vec{v}}{c} = \frac{w\wedge \gamma_0}{w\cdot\gamma_0}

Relative force law:

\displaystyle \frac{d\mathcal{E}}{d(ct)} = q_e \vec{E}\cdot\vec{v} + q_m \vec{B}\cdot \vec{v}
\displaystyle \frac{d\vec{p}}{dt} = q_e \vec{E} + q_m \vec{B} + q_e \vec{v}\times\vec{B} - q_m \vec{v}\times\vec{E}

Rate of work performed (c=1)

Relative Lorentz force, including magnetic charge

Noether's Theorem and Dual Symmetry

e^{-I\phi/2}\nabla \mathbf{F}e^{I\phi/2} = e^{-I\phi/2}je^{I\phi/2}

A new gauge symmetry leads to an independently conserved quantity : certainly conserved in vacuum

\underline{X}(I) = [\chi I + \vec{S}I^{-1}]\gamma_0

Helicity Pseudocurrent (tensor) is newly conserved

\chi = \vec{B}_e \cdot \vec{A} - \vec{E}_m \cdot \vec{C}
\vec{S} = \vec{E}_e\times\vec{A} + \vec{B}_m\times \vec{C}

Spin-density vector is the helicity flux density

Scalar helicity density

These definitions only make sense when full dual-symmetric fields are used

They match postulated definitions of these quantities in the constrained case with \( \mathbf{F} = \mathbf{F}I \)

Conclusions 

  • Spacetime Clifford algebra is the natural language of electromagnetism
     
  • The dual-symmetric structure of the electromagnetic theory becomes manifest when expressed in geometrically invariant forms
     
  • The conserved quantity from Noether's theorem resulting from the continuous dual-symmetry in vacuum field propagation is the helicity
     
  • Dual-symmetry is spontaneously broken by the convention to make all charges electric. The symmetry is preserved if electric and magnetic charges are simultaneously swapped with the fields.

Thank you!

JD, et al. Physics Reports 589 1-71 (2015)