FESTIM: empowering tritium transport modelling for SPARC and ARC

Remi Delaporte-Mathurin

Outline

  • Why FESTIM?
  • What is FESTIM?
  • FESTIM for SPARC
  • FESTIM for ARC
  • FESTIM & CFS

Why FESTIM

The tritium issue

  • Radioactive substance
  • Health risks

 

→ Limit inventories

→ Detritiation

→ Accurate assessment

Safety hazards

Risk of contamination

  • Permeation to cooling systems
  • Challenges for maintenance and safety

 

→ Permeation barriers

→ Clean-up

Tritium self-sufficiency

  • Optimised fuel cycle is required for a fusion economy

 

 

→ Breeding blanket design

→ Sub-systems engineering

 

 

How to analyse this thermo-desorption spectrum?

What's the T inventory in the first wall of SPARC?

How much T will permeate to the coolant?

How long do we need to bake components for detritiation?

What is the extraction efficiency of the TES?

What is the T residence time of component X?

Hydrogen transport

Hydrogen transport

McNabb & Foster model

\frac{\partial c_\mathrm{m}}{\partial t} = \nabla \cdot (D \nabla c_\mathrm{m}) - \frac{\partial c_{\mathrm{t}, i}}{\partial t}
\frac{\partial c_{\mathrm{t}, i}}{\partial t} = k \ c_\mathrm{m} \ (n - c_{\mathrm{t}, i}) - p \ c_{\mathrm{t}, i}

Mobile H concentration

Trapped H concentration

\rho C_p \frac{\partial T}{\partial t} = \nabla \cdot (\lambda \nabla T)

Temperature dependence

McNabb & Foster model

\frac{\partial c_\mathrm{m}}{\partial t} = \nabla \cdot (D \nabla c_\mathrm{m}) - \frac{\partial c_{\mathrm{t}, i}}{\partial t}
\frac{\partial c_{\mathrm{t}, i}}{\partial t} = k \ c_\mathrm{m} \ (n - c_{\mathrm{t}, i}) - p \ c_{\mathrm{t}, i}

Time derivatives

McNabb & Foster model

\frac{\partial c_\mathrm{m}}{\partial t} = \nabla \cdot (D \nabla c_\mathrm{m}) - \frac{\partial c_{\mathrm{t}, i}}{\partial t}
\frac{\partial c_{\mathrm{t}, i}}{\partial t} = k \ c_\mathrm{m} \ (n - c_{\mathrm{t}, i}) - p \ c_{\mathrm{t}, i}

Diffusive term

McNabb & Foster model

\frac{\partial c_\mathrm{m}}{\partial t} = \nabla \cdot (D \nabla c_\mathrm{m}) - \frac{\partial c_{\mathrm{t}, i}}{\partial t}
\frac{\partial c_{\mathrm{t}, i}}{\partial t} = k \ c_\mathrm{m} \ (n - c_{\mathrm{t}, i}) - p \ c_{\mathrm{t}, i}

Trapping term

McNabb & Foster model

\frac{\partial c_\mathrm{m}}{\partial t} = \nabla \cdot (D \nabla c_\mathrm{m}) - \frac{\partial c_{\mathrm{t}, i}}{\partial t}
\frac{\partial c_{\mathrm{t}, i}}{\partial t} = k \ c_\mathrm{m} \ (n - c_{\mathrm{t}, i}) - p \ c_{\mathrm{t}, i}

Detrapping term

Surface physics

Molecular dissociation and recombination

\varphi = K_d \ P - K_r c_\mathrm{m}^2

Sievert's law of solubility

c_\mathrm{m} = K_S \ \sqrt{P}
c_\mathrm{m} = K_H \ P

Henry's law of solubility

c_\mathrm{m} = \frac{\varphi_{\mathrm{imp}} R_p}{D} + \sqrt{\frac{\varphi_{\mathrm{imp}}+K_d P}{K_r}}

Plasma + gas exposure

Application: ITER PFCs

We need a numerical tool

W

Cu

CuCrZr

Particle and heat fluxes

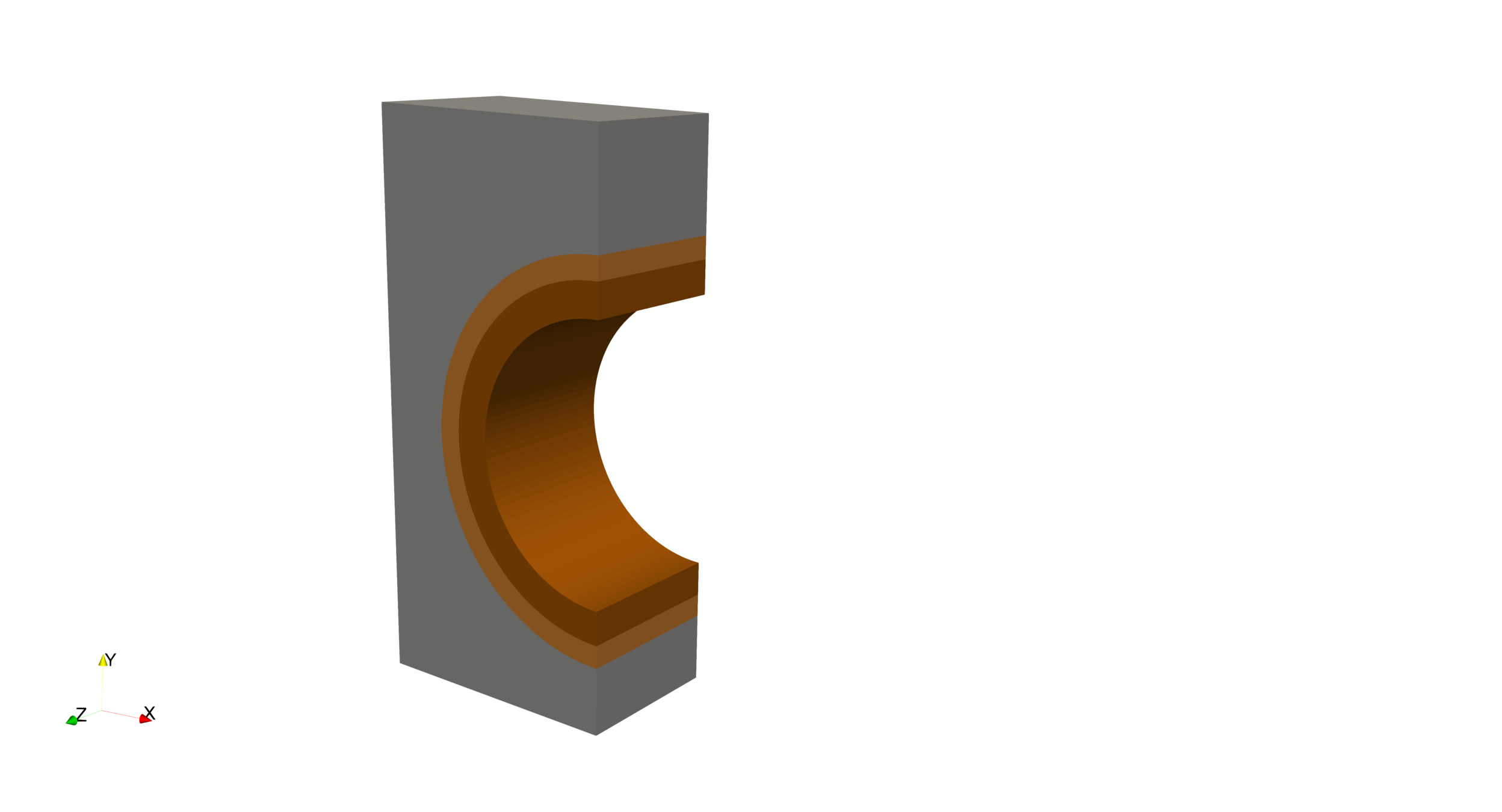
Convection

14 mm

Problem: predict T retention and permeation

 

  • Multi-dimensional
  • Multi-material
  • non homogeneous temperature

What is FESTIM 

User inputs

  • Material properties
  • Geometry
  • Boundary conditions
  • Initial conditions

Heat transfer model

T

Hydrogen transport model

FESTIM

Outputs

  • Tritium concentration fields \(c(x,t)\)
  • Temperature field \(T(x,t)\)
  • surface fluxes
  • inventories
  • average concentration
  • ...

2022

2019

Start of development

  • 1D/2D/3D
  • Finite elements
  • Python
  • multi-material
  • Heat transfer

History of FESTIM

open-source

  • development continuing at MIT

Oct 2023

v1.0 release

2024

FESTIM

An open-source python-based finite elements code for hydrogen transport ​

  • Based on the FEniCS library
  • Flexible
  • Complex geometries
  • Very well established library

FESTIM's philosophy

We want to make hydrogen transport simulations easy for everyone!

 

We also encourage contributions!

  • Feedback (suggestions, bug reports...)
  • Implement new features
  • Bug fixing
  • Documentation update (even small ones!)
  • ...

Look out for "Good first issues"

on GitHub

Meet the development team

Jonathan Dufour

CEA, France

+ all contributors

Remi Delaporte-Mathurin

MIT, US

@RemDelaporteMathurin

Samuele Meschini

MIT, US

@SamueleMeschini

Etienne Hodille

CEA, France

@ehodille

Gabriele Ferrero

PoliTo, Italy

@gabriele-ferrero

James Dark

CEA, France

@jhdark

Vladimir Kulagin

MEPhI, Russia

@KulaginVladimir

FESTIM is open-source

Check it out on Github

github.com/festim-dev/FESTIM

✅ More transparency

✅ More collaborations

✅ More flexibility

The code is missing a feature?

Just add it!

Found a bug?

Report it and we'll fix it!

FESTIM's development workflow

  • Anyone can create their own copy (fork) of the FESTIM repository and make changes
  • PRs are a place where FESTIM's maintainers review the proposed changes
  • ~500 tests are automatically run
  • The PR is only merged when all the tests pass ✅

fork

fork

pull request

FESTIM

Jane Doe

FESTIM

John Doe

FESTIM

festim-dev

pull request

How to use FESTIM

github.com/festim-dev/festim

User-support forum

festim.discourse.group

User-support forum

Join the Slack channel

Documentation

and installation instructions

festim.readthedocs.io

FESTIM is user-friendly

  • Very easy to learn
  • Plenty of libraries (numpy, scipy, matplotlib, HTM...)
  • Inuitive interface
conda install -c conda-forge fenics
pip install festim

Two lines to install!

import festim as F
import numpy as np

my_model = F.Simulation()

my_model.mesh = F.MeshFromVertices(
    vertices=np.linspace(0, 1e-6, num=1001)
)

my_model.materials = F.Material(id=1, D_0=1.9e-7, E_D=0.2)

my_model.T = 500  # K

my_model.boundary_conditions = [
    F.DirichletBC(
        surfaces=[1, 2],
        value=1e15,  # H/m3/s
        field=0
        )
]

my_model.settings = F.Settings(
    absolute_tolerance=1e10,
    relative_tolerance=1e-10,
    final_time=100  # s
    )


my_model.dt = F.Stepsize(0.1)  # s

my_model.initialise()

my_model.run()

FESTIM workshop

The FESTIM workshop is a series of tutorials

 

from basic problems to more advance cases

festim.discourse.group

FESTIM is fully documented

Check out the complete documentation at

festim.readthedocs.io

Installation instructions

User guide

  • Development guide
  • Tutorials
  • Theory background
  • API reference

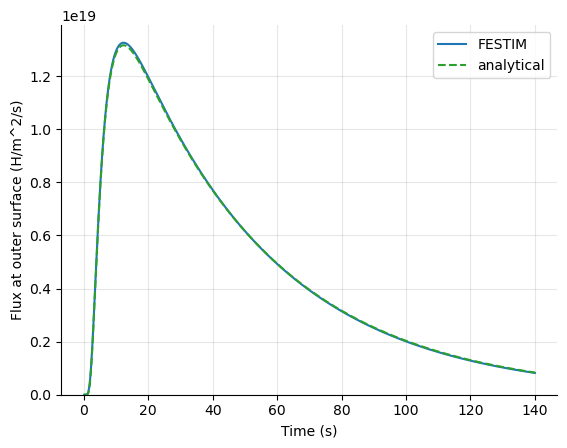
FESTIM is verified & validated

  • Validated against TDS, permeation experiments...
  • Verified against analytical solutions in many different problems
  • New V&V online book

festim-vv-report.readthedocs.io

 

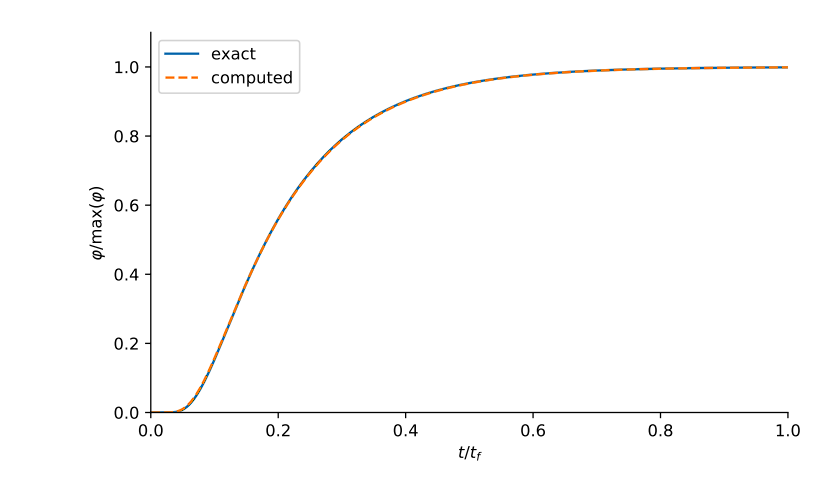
V&V

Effective-diffusion through a slab

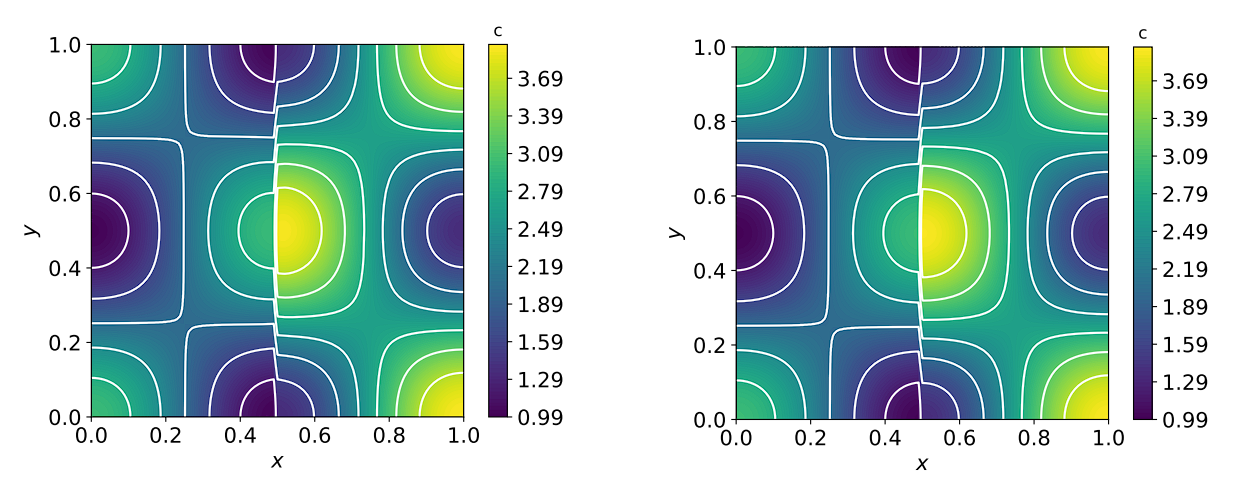
2D multi-material

Exact

Computed concentration

Method of Exact Solution

governing equations

exact solutions

parameters (sources, BCs, ICs)

FESTIM

computed solutions

solve

run

compare

⚠️sometimes very complex!

Method of Manufactured Solutions

governing equations

manufactured solutions

source terms, BCs and ICs

FESTIM

computed solutions

compare

FESTIM is used worldwide

8 private companies

10 universities

16 research organisations

📈5 years of development

📑13+ publications

🗣️110+ citations

🧑‍💻14+ contributors

🏛️24+ institutions using the code

🧑‍💻27+ Slack members

⭐75+ stars on GitHub

      3 workshops

FESTIM in numbers

Evolution of GitHub stars

Open source

SOFE workshop

New reference paper

FESTIM for SPARC

Retention studies

  • Delaporte-Mathurin et al 2024 International Journal of Hydrogen Energy 63 786–802
  • Delaporte-Mathurin et al 2024 Nucl. Fusion 64 026003
  • ITER plasma facing components
  • Transient estimation of tritium retention

T concentration

At

t = 10^7 \, \mathrm{s}
\mathrm{inventory} = \int c \, dV
T_\mathrm{surface} = 700 \, \mathrm{K}
c_\mathrm{m} = 10^{21} \, \mathrm{H\,m^{-3}}
c_\mathrm{m} = 10^{20} \, \mathrm{H\,m^{-3}}
T_\mathrm{surface} = 1000 \, \mathrm{K}

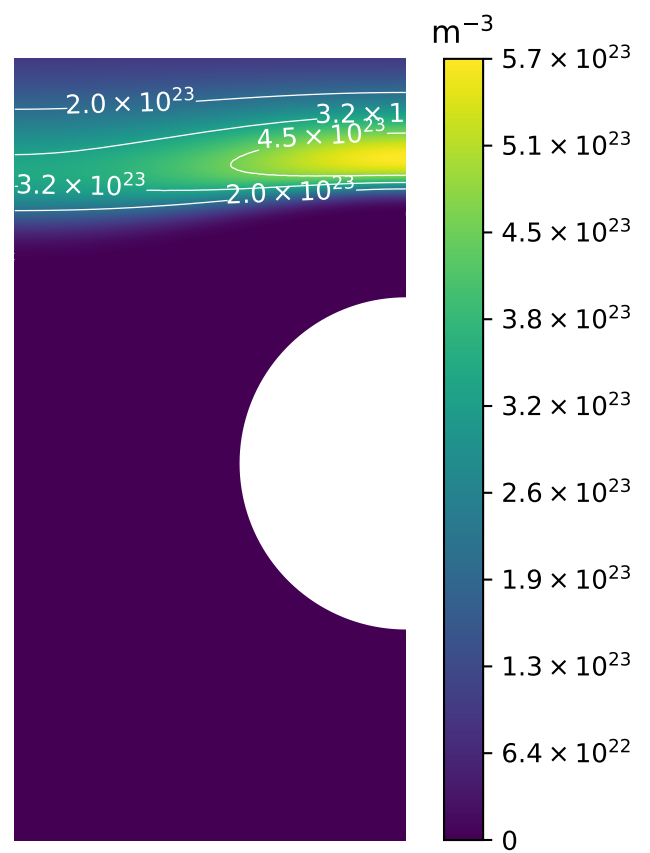
Parametric study

+

+

Parametric study

+

+

+

+

+

+

+

+

+

+

+

+

+

+

Parametric study

At

t = 10^7 \, \mathrm{s}

Gaussian Process Regression (GPR)

Chris Bowman. ‘C-Bowman/Inference-Tools: 0.5.3 Release’. Zenodo, 20 April 2020. 

Parametric study

Monoblock behaviour law

At

t = 10^7 \, \mathrm{s}

Rapid assessment of monoblock inventories

Inner Vertical Target

Inner Strike Point

Outer Strike Point

Outer Vertical Target

Dome

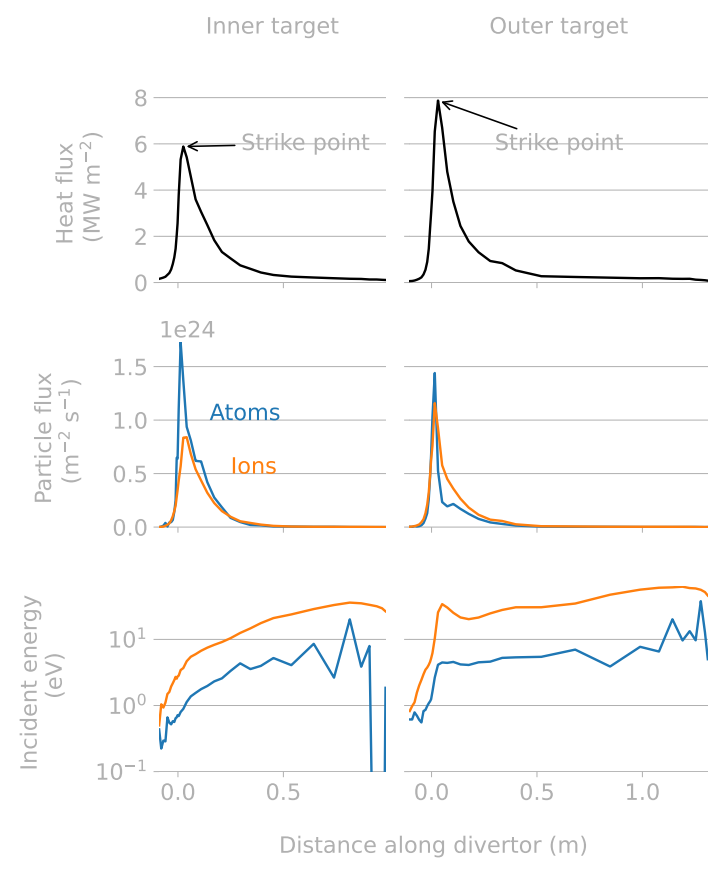
Converting SOLPS plasma inputs...

T_\mathrm{surface} = 1.1\times10^{-4} \, \varphi_\mathrm{heat} + T_\mathrm{coolant}
R_{p, \, i} = R_{p, \, i}(E_\mathrm{particle, \, i})

SOLPS runs: Pitts et al NME (2020)

c_\mathrm{surface} = \frac{\textcolor{#fd9b21}{\varphi_\mathrm{ions} \, R_{p, \, \mathrm{ions}}} + \textcolor{#3c78d8}{\varphi_\mathrm{atoms} \, R_{p \, \mathrm{atoms}} }}{D(T_\mathrm{surface})}

...to divertor inventory

\varphi_\mathrm{heat}
\varphi_\mathrm{particle}
T_\mathrm{surface}

Monoblock inventory

c_\mathrm{surface}
E_\mathrm{particle}
c_\mathrm{surface} = \frac{\varphi_\mathrm{ions} \, R_{p, \, \mathrm{ions}} + \varphi_\mathrm{atoms} \, R_{p \, \mathrm{atoms}}}{D(T_\mathrm{surface})}
T_\mathrm{surface} = 1.1\times10^{-4} \, \varphi_\mathrm{heat} + T_\mathrm{coolant}

+

Divertor inventory

SOLPS runs: Pitts et al NME (2020)

  • The strike point is not the maximum H inventory
  • The total divertor inventory can be computed

 

Delaporte-Mathurin et al Nucl. Fusion (2021)

The inventory is estimated from the surrogate model

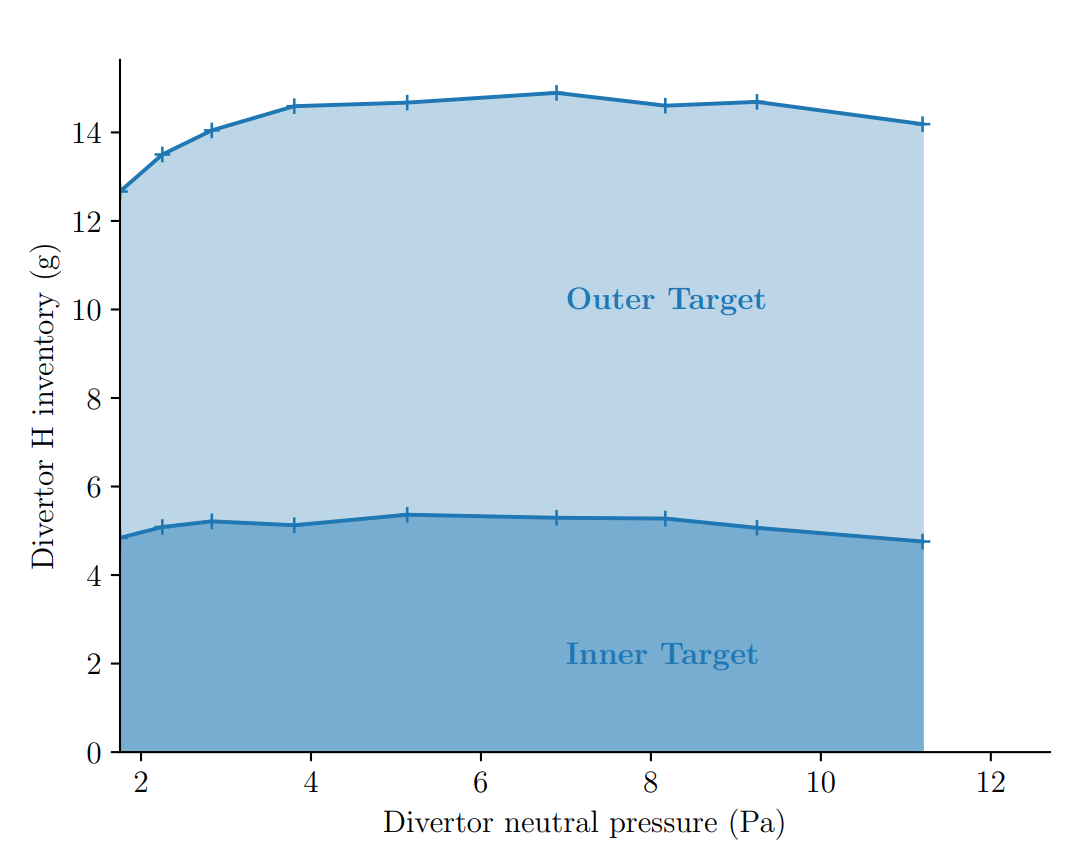
Divertor H inventory (g) at \( t = 10^7 \, \mathrm{s}\)

Safety limit \( = \) 700 g T

\( \max \approx \) 2 % limit ✅

ITER divertor inventory

Delaporte-Mathurin et al Nucl. Fusion (2021)

Detritiation studies

Delaporte-Mathurin et al 2024 Nucl. Fusion 64 026003

Influence of ELMs on retention

Kulagin, V., & Gasparyan, Y. Numerical Simulation of deuterium retention in tungsten under ELM-like conditions. J. Nucl. Mater. (under revision)

  • 1D model of a ITER monoblock
  • Transient heat transfer simulation
  • Varying surface heat flux

FESTIM for ARC

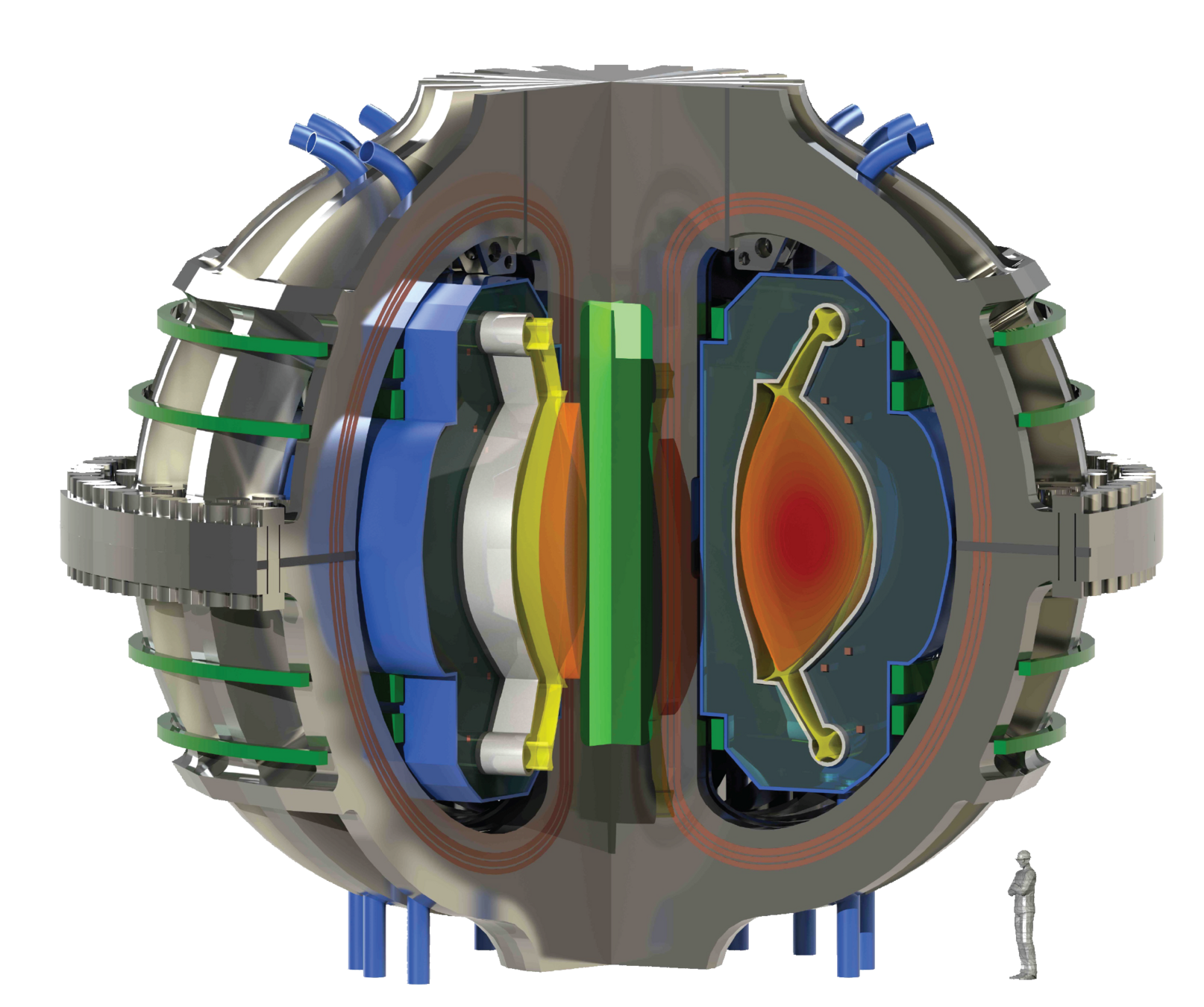
Breeding Blanket modelling

  • DEMO WCLL
  • Complex 3D geometry
  • Coupled to fluid dynamics
  • Tritium generation in the LiPb volume (computed from OpenMC)

James Dark et al 2021 Nucl. Fusion 61 116076

Tritium extraction system

courtesy of K. Dunnell (MIT)

  • Permeation Against Vacuum
  • Complex 3D geometry
  • Coupled with fluid dynamics
  • Tritium extraction from permeable membranes

Tritium extraction system

courtesy of K. Dunnell (MIT)

① neutrons are generated

 

② tritium is created from nuclear reactions

 

③ tritium is transported in the salt

 

④ tritium is released into the gas phase

 

⑤ tritium is collected and counted

BABY breeding experiment

BABY breeding experiment

Velocity

Temperature

Tritium concentration

BABY breeding experiment

courtesy of C. Weaver (MIT)

0D model

FESTIM model

Metal Foil Pumps for DIR

  • H is implanted in the first \( 10 \ \mathrm{nm} \)
  • Super-permeation regime is attained at high recombination energy (upstream surface)
  • Source code

Benedikt & Day, (2017) Fusion Engineering and Design

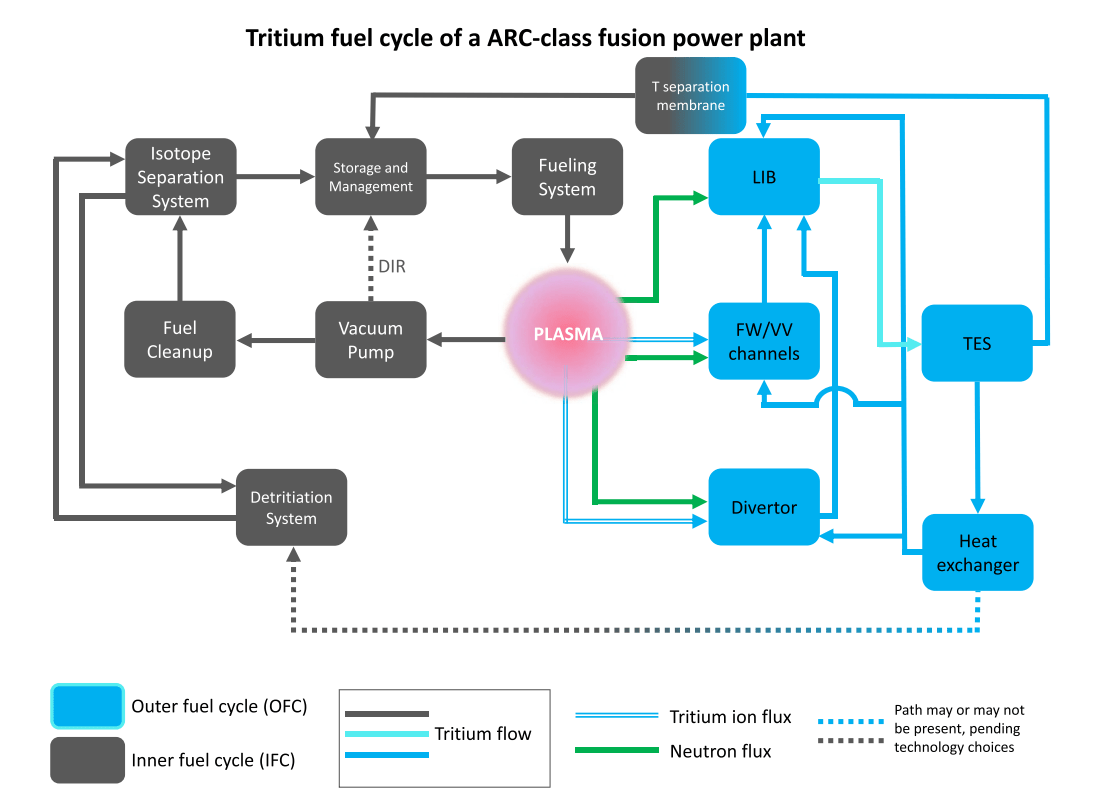
FESTIM can inform fuel cycle models

Samuele Meschini et al 2023 Nucl. Fusion 63 126005

Text

Permeation barriers

 

Towards the future: FESTIM 2

Chemical reactions

  • More suitable for molten salts
  • Even more flexibility

Multi-species transport

  • Isotopic exchange
  • Multi-level trapping
  • Transport of impurities, He, interstitials...
  • Validation on WEST PFCs

Finite element engine upgrade

  • Better performances (including in parallel)
  • Bigger models
  • Mixed topology meshes

Graphical user interface

Follow the development roadmap

github.com/orgs/festim-dev/projects/2

New examples

Isotope swapping

\mathrm{H} + [\ \ \ ]_\mathrm{trap} \ \substack{p \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k} \ [\mathrm{H}]_\mathrm{trap}
\mathrm{D} + [\ \ \ ]_\mathrm{trap} \ \substack{p \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k} \ [\mathrm{D}]_\mathrm{trap}
\mathrm{D} + [\mathrm{H}]_\mathrm{trap} \ \substack{k_\mathrm{swap} \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1.2em] k_\mathrm{swap}} \ [\mathrm{D}]_\mathrm{trap} + \mathrm{H}

same underlying equations!

Can be represented by festim.Reaction

Isotope swapping

my_model.species = [
    mobile_H,
    mobile_D,
    trapped_H,
    trapped_D,
]

my_model.reactions = [
    F.Reaction(
        k_0=k_0,
        E_k=0.39,
        p_0=1e13,
        E_p=1.2,
        reactant1=mobile_H,
        reactant2=empty_trap,
        product=trapped_H,
        volume=my_subdomain,
    ),
    F.Reaction(
        k_0=k_0,
        E_k=0.39,
        p_0=1e13,
        E_p=1.2,
        reactant1=mobile_D,
        reactant2=empty_trap,
        product=trapped_D,
        volume=my_subdomain,
    ),
    F.Reaction(
        k_0=k_0,
        E_k=0.1,
        p_0=k_0,
        E_p=0.1,
        reactant1=mobile_H,
        reactant2=trapped_D,
        product=[mobile_D, trapped_H],
        volume=my_subdomain,
    ),
]

Usual trapping reactions

Swapping reaction

4 species are defined

Multi-isotope, multi-level trapping

  • 7 different species
  • 6 reactions
  • \( c_H = 10^{20} \ \mathrm{m^{-3}} \) on the left
  • \( c_D = 10^{19} \ \mathrm{m^{-3}} \) on the right
  • Source code available here

1 trap, 2 levels, 2 isotopes

\mathrm{H} + [\ \ \ ] \ \substack{p_1 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_1} \ [\mathrm{H}_1\mathrm{D}_0]
\mathrm{H} + [\mathrm{H}_1\mathrm{D}_0] \ \substack{p_2 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_2} \ [\mathrm{H}_2\mathrm{D}_0]
\mathrm{D} + [\ \ \ ] \ \substack{p_3 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_3} \ [\mathrm{H}_0\mathrm{D}_1]
\mathrm{D} + [\mathrm{H}_0\mathrm{D}_1] \ \substack{p_4 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_4} \ [\mathrm{H}_0\mathrm{D}_2]
\mathrm{H} + [\mathrm{H}_0\mathrm{D}_1] \ \substack{p_5 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_5} \ [\mathrm{H}_1\mathrm{D}_1]
\mathrm{D} + [\mathrm{H}_1\mathrm{D}_0] \ \substack{p_6 \\[-1em] \longleftarrow\\[-1em] \longrightarrow \\[-1em] k_6} \ [\mathrm{H}_1\mathrm{D}_1]

\( \mathrm{H} \) = mobile H

\( \mathrm{D} \) = mobile D

\( [\mathrm{H}_x\mathrm{D}_y] \) = \(x\) H and \(y\) D in trap

Spherical cavity trapping

see Zibrov and Schmid, NME, 2024

for complete description

  • Implementation in FESTIM of the spherical cavity trapping model developed by Zibrov and Schmid
  • Custom trapping equations
  • Smooth implementation in FESTIM

Anisotropy

  • Anisotropic materials can be simulated with very few modifications
D = \begin{bmatrix} D_{xx} & 0\\ 0 & D_{yy} \end{bmatrix}

FESTIM & CFS

Your next tritium systems are likely to rely on tritium transport modelling.

 

Why not get involved in FESTIM to make sure it suits your needs?

Other application cases

Thermo-desorption

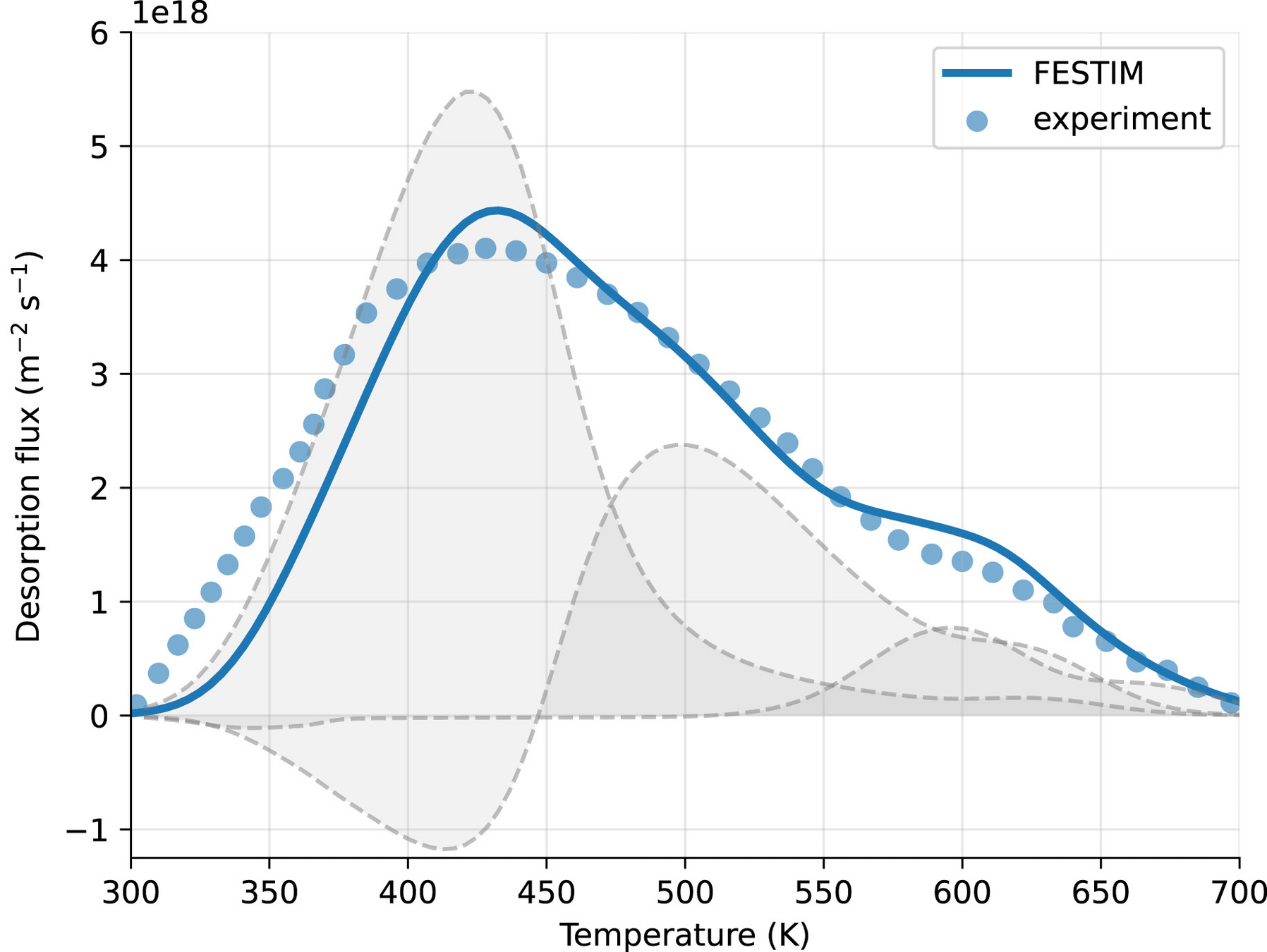
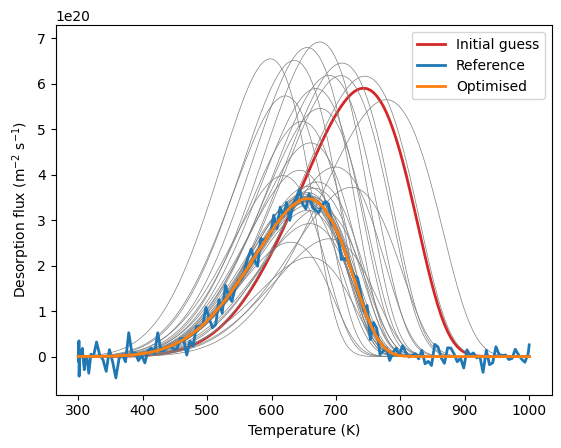
  • TDS analysis
  • Automated fitting routines

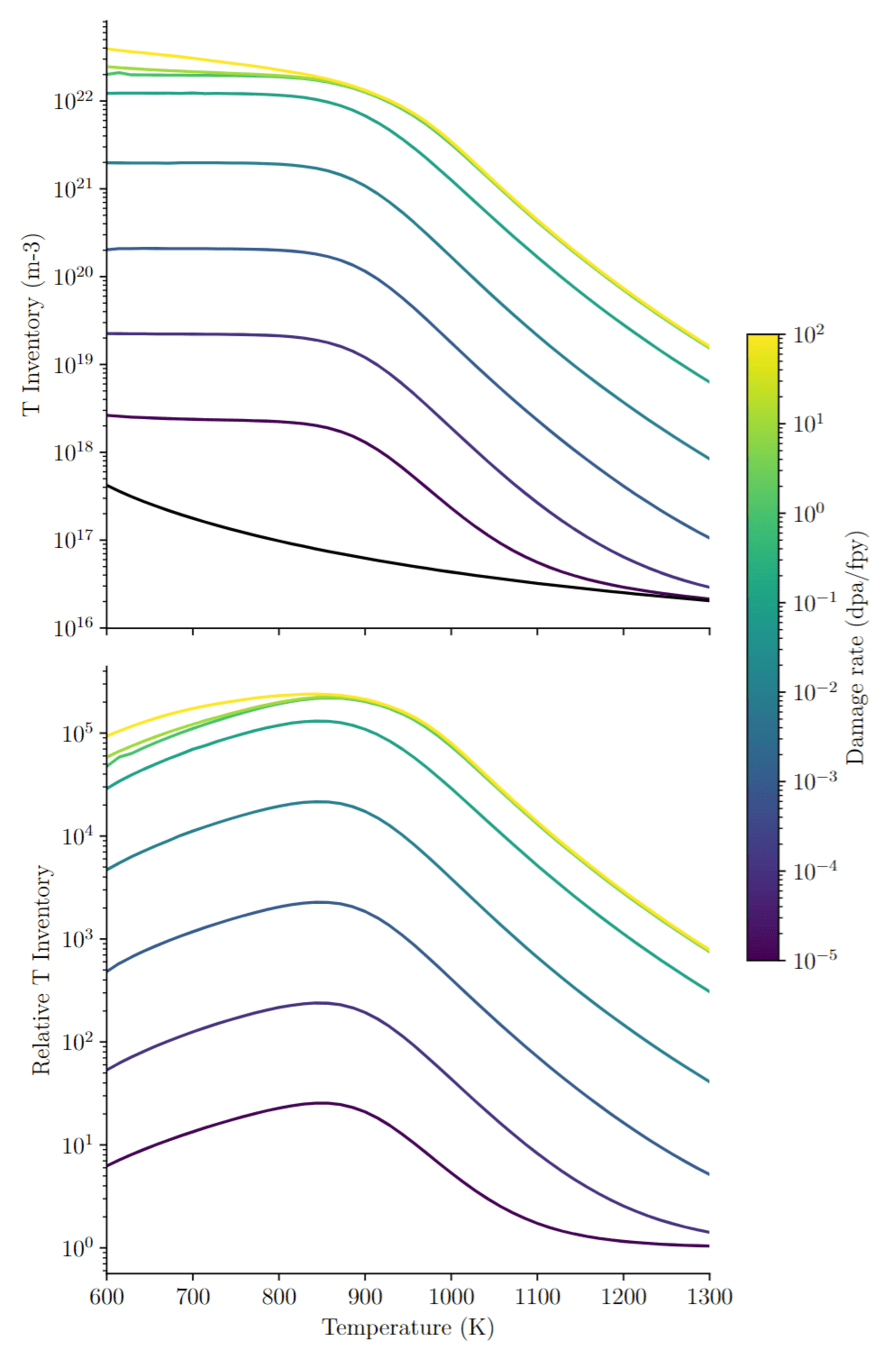
Neutron-induced trap model

  • Custom trapping model
  • Dynamic evolution of trap densities including annealing
  • Parametrised on TDS data from T Schwartz Selinger

 James Dark et al 2024 Nucl. Fusion 64 086026

Permeation experiment

No barrier

with barrier

Permeation barrier

Substrate

High H pressure

Low H pressure

Conservation of chemical potential

Permeation flux

\frac{c^-}{K_S^-} = \frac{c^+}{K_S^+}

Kinetic surface model

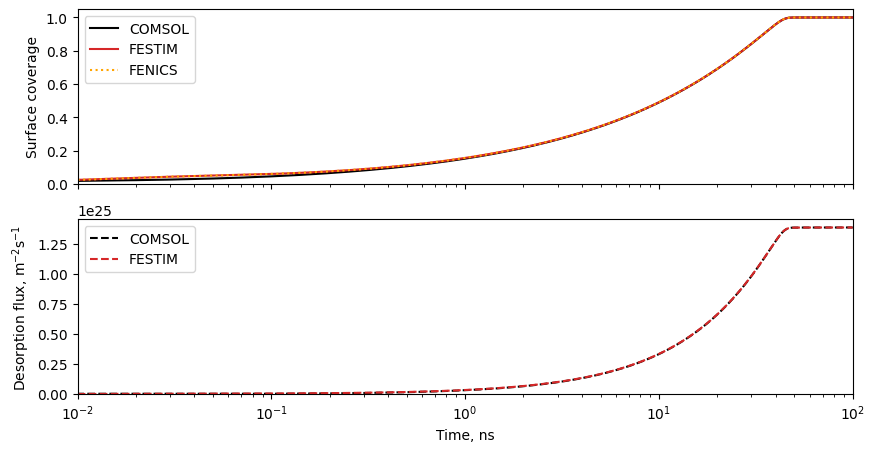
courtesy of V. Kulagin (MePhi)

Depleting enclosure

\(P(t)\)

permeation flux

Enclosure

\frac{dP(t)}{dt} \propto \varphi_\mathrm{permeation}\\ \varphi_\mathrm{permeation} \propto P(t)