A Lie Algebraic Perspective on Frame Theory
/codex21
this talk!
Collaborator

Florida State University
Funding
National Science Foundation (DMS–2107700)
Simons Foundation (#709150)
Finite Frames
\(\mathfrak{H} = \mathbb{R}^d\) or \(\mathbb{C}^d\). A (finite) frame in \(\mathfrak{H}\) is a spanning set \(f_1, \dots, f_N \in \mathfrak{H}\).
\(F = [f_1 | f_2 | \dots | f_N]\). The frame operator is \(FF^\ast \in \mathscr{H}(d)\).
Frequently specify \(\operatorname{spec}(FF^\ast) = (\lambda_1, \dots , \lambda_d)\) and/or \((\|f_1\|^2,\dots , \|f_N\|^2) = (r_1, \dots, r_N)\).
Gram Matrices
Both pieces of information are present in the Gram matrix
\(F^\ast F = \left[\langle f_i, f_j\rangle \right]_{i,j} \in \mathscr{H}(N)\)
since \(F^\ast F\) and \(F F^\ast\) have the same rank and nonzero eigenvalues.
Unitary equivalence classes of frames have the same Gram matrix: if \(U \in O(d)\) or \(U(d)\), then
\((UF)^\ast(UF) = F^\ast U^\ast U F = F^\ast F\).
For \(\boldsymbol{\lambda} = (\lambda_1, \dots , \lambda_d, 0, \dots 0)\),
\(\mathcal{O}_{\boldsymbol{\lambda}} := \{U \operatorname{diag}(\boldsymbol{\lambda}) U^\ast | U \in U(N)\}\subset \mathscr{H}(N)\)
is a flag manifold.
Lie Groups
Informal Definition. A Lie group is a group that is also a manifold (a “continuous group of symmetries”).
Examples.
- \(\mathbb{R}^n\), \(\mathbb{C}^n\)
- \(GL_n(\mathbb{R})\), \(GL_n(\mathbb{C})\)
- \(SL_n(\mathbb{R})\), \(SL_n(\mathbb{C})\)
- \(O(n)\), \(SO(n)\), \(U(n)\), \(SU(n)\) (e.g., \(SO(3)\simeq \mathbb{RP}^3\), \(SU(2) \simeq S^3\))
- Products (e.g. the compact torus \(U(1)^n\))
Lie Algebras
Informal Definition. A Lie algebra \(\mathfrak{g}\) (or \(\operatorname{Lie}(G)\)) associated to a Lie group \(G\) is the tangent space at the identity (the “infinitesimal symmetries”).
Examples.
- \(\operatorname{Lie}(\mathbb{R}^n) \simeq \mathbb{R}^n\)
- \(\mathfrak{gl}_n(\mathbb{R}) \simeq \mathbb{R}^{n \times n}\), \(\mathfrak{gl}_n(\mathbb{C}) \simeq \mathbb{C}^{n \times n}\)
- \(\mathfrak{sl}_n(\mathbb{R}) \simeq \{\text{traceless matrices}\}\), \(\mathfrak{sl}_n(\mathbb{C}) \simeq \{\text{traceless matrices}\}\)
- \(\mathfrak{o}(n) = \mathfrak{so}(n) \simeq \{\text{skew-symmetric matrices}\}\), \(\mathfrak{u}(n) \simeq \{\text{skew-Hermitian matrices}\}\), \(\mathfrak{su}(n) \simeq \{\text{traceless skew-Hermitian}\}\)
Suppose \(\gamma(t) \in U(n)\) with \(\gamma(0)=I\). Since \(I = \gamma(t)\gamma(t)^\ast\),
\(0 = \left. \frac{d}{dt}\right|_{t=0} \gamma(t)\gamma(t)^\ast = \gamma'(0)\gamma(0)^\ast + \gamma(0)\gamma'(t)^\ast = \gamma'(0) + \gamma'(0)^\ast\)
The Lie bracket \([\cdot , \cdot ]\) is the matrix commutator on matrix groups: \([A,B] = AB-BA\).
Adjoint Actions
A Lie group \(G\) acts on itself by conjugation: \(g \cdot h := g h g^{-1}\).
This fixes the identity, so linearizing gives an action of \(G\) on \(\mathfrak{g}\), called the adjoint action.
For matrix groups, the adjoint action is the conjugation action.
Example. \(\mathfrak{so}(3) \simeq \left\{\begin{pmatrix} 0 & -z & y \\ z & 0 & -x \\ -y & x & 0\end{pmatrix}:x,y,z \in \mathbb{R}\right\}\simeq (\mathbb{R}^3, \times)\)
Adjoint Orbits
If \(G\) is a Lie group, each orbit of the adjoint action is a symplectic manifold.\(^1\)
1. Strictly speaking, coadjoint orbits in \(\mathfrak{g}^\ast\) are symplectic.

\(i\mathscr{H}(N) = \mathfrak{u}(N)\), so conjugation orbits of Hermitian matrices can be interpreted as adjoint orbits.
Symplectic Geometry and Frame Theory
\(\mathcal{O}_{\boldsymbol{\lambda}} = \{U \operatorname{diag}(\boldsymbol{\lambda})U^\ast | U \in U(N)\} \simeq \{F \in \mathbb{C}^{d \times N} | \operatorname{spec}(FF^\ast) = (\lambda_1, \dots , \lambda_d)\}/U(d)\) is symplectic.
Proposition. The map recording diagonal entries is the momentum map of a Hamiltonian torus action.
Theorem [Atiyah and Guillemin–Sternberg]
Momentum maps of Hamiltonian torus actions have convex image and connected level sets.
Theorem [with Needham, 2021]
The space \(\mathscr{F}_{\boldsymbol{\lambda}}^{\mathbb{C}^d,N}(\boldsymbol{r})\subset \mathbb{C}^{d \times N}\) of frames with \(\operatorname{spec}(FF^\ast) = (\lambda_1, \dots , \lambda_d)\) and \(\|f_i\|^2 = r_i\) for \(i=1, \dots , N\) is path-connected.

A generalized frame homotopy theorem for complex frames.
How Could This Generalize?
\(\dim(\mathfrak{o}(N)) = \frac{N(N-1)}{2}\)
\(\dim(\{\text{symmetric } N \times N \text{ matrices}\})=\frac{N(N+1)}{2}\)
The analog of the unitary group for symplectic matrices is the (compact) symplectic group \(\operatorname{Sp}(N)\); its Lie algebra \(\mathfrak{sp}(N)\) consists of quaternionic skew-Hermitian matrices.
\(\dim(\mathfrak{sp}(N)) = 2N^2+N\)
\(\dim(\{\text{Hermitian quaternionic } N \times N \text{ matrices}\}) = 2N^2-N\)
Can’t identify these!
Cartan Decomposition
An involution \(\theta: \mathfrak{g} \to \mathfrak{g}\) is a Cartan involution if \(-B(\cdot , \theta \cdot)\) is positive-definite, where \(B(\cdot , \cdot)\) is the Killing form.
Example. \(\theta: \mathfrak{sl}_n(\mathbb{R}) \to \mathfrak{sl}_n(\mathbb{R})\) defined by \(\theta(X) = -X^T\). This is the derivative of the involution on \(SL_n(\mathbb{R})\) given by \(U \mapsto (U^{-1})^T\).
\(E_{+1} =: \mathfrak{k}\) consists of skew-symmetric matrices
\(E_{-1} =: \mathfrak{p}\) consists of (traceless) symmetric matrices
\(\mathfrak{sl}_n(\mathbb{R}) = \mathfrak{k} \oplus \mathfrak{p}\) is an orthogonal decomposition — the Cartan decomposition
\([\mathfrak{k},\mathfrak{p}]\subseteq \mathfrak{p}\), so the adjoint action of \(SO(n) = \exp(\mathfrak{k})\) restricts to an action on \(\mathfrak{p}\)
\([\mathfrak{p},\mathfrak{p}]\subseteq\mathfrak{k}\), so any Lie subalgebra of \(\mathfrak{p}\) is abelian.
Theorem. \(G\) non-compact, semisimple, and \(\mathfrak{g} = \mathfrak{k} \oplus \mathfrak{p}\) a Cartan decomposition with \(K = \exp(\mathfrak{k})\) and \(P = \exp(\mathfrak{p})\), then \(G = KP\) and \(T_{[I]} G/K \simeq \mathfrak{p}\).
The adjoint action of \(K\) on \(\mathfrak{p}\) is often called the isotropy representation.
Kostant’s Convexity Theorem
Theorem [Kostant]
Let \(G\) be a Lie group with Cartan decomposition of the Lie algebra \(\mathfrak{g} = \mathfrak{k} \oplus \mathfrak{p}\).
If \(\mathfrak{a} \subset \mathfrak{p}\) is a maximal abelian subspace, \(P: \mathfrak{p} \to \mathfrak{a}\) orthogonal projection, and \(\mathcal{O}_a\) the isotropy orbit of \(a \in \mathfrak{a}\), then
\(P(\mathcal{O}_a) = \operatorname{conv}(W \cdot a)\).
\(W = N_K(\mathfrak{a})/Z_K(\mathfrak{a})\) is the Weyl group associated to \((\mathfrak{a},\mathfrak{g})\).
Example.
\(G = SL_n(\mathbb{C})\), \(K=SU(N)\), \(\mathfrak{k} = \mathfrak{su}(N)\), \(\mathfrak{p} = \mathscr{H}_0(N)\), \(a = \operatorname{diag}(a_1, \dots , a_N)\),
\(\mathcal{O}_a = \{UaU^\ast | U \in SU(N)\}\)
\(P(\mathcal{O}_a) = \operatorname{conv}(S_N \cdot a)\) is essentially Schur–Horn.
Can play the same game with real or quaternionic matrices!
Connected Level Sets
Theorem [Mare]
Under additional technical assumptions,
\(H_{Z_K(\mathfrak{a})}^\ast (\mathcal{O}_a; \mathbb{Q}) \to H_{Z_K(\mathfrak{a})}^\ast(P^{-1}(a); \mathbb{Q})\)
is a surjection for all \(a \in \mathfrak{a}\).
In particular, \(P^{-1}(a)\) is connected if \(\mathcal{O}_a\) is.
Theorem [Kostant]
Let \(G\) be a Lie group with Cartan decomposition of the Lie algebra \(\mathfrak{g} = \mathfrak{k} \oplus \mathfrak{p}\).
If \(\mathfrak{a} \subset \mathfrak{p}\) is a maximal abelian subspace, \(P: \mathfrak{p} \to \mathfrak{a}\) orthogonal projection, and \(\mathcal{O}_a\) the isotropy orbit of \(a \in \mathfrak{a}\), then
\(P(\mathcal{O}_a) = \operatorname{conv}(W \cdot a)\).
\(W = N_K(\mathfrak{a})/Z_K(\mathfrak{a})\) is the Weyl group associated to \((\mathfrak{a},\mathfrak{g})\).
This implies the generalized frame homotopy theorem for complex frames.
Quaternions
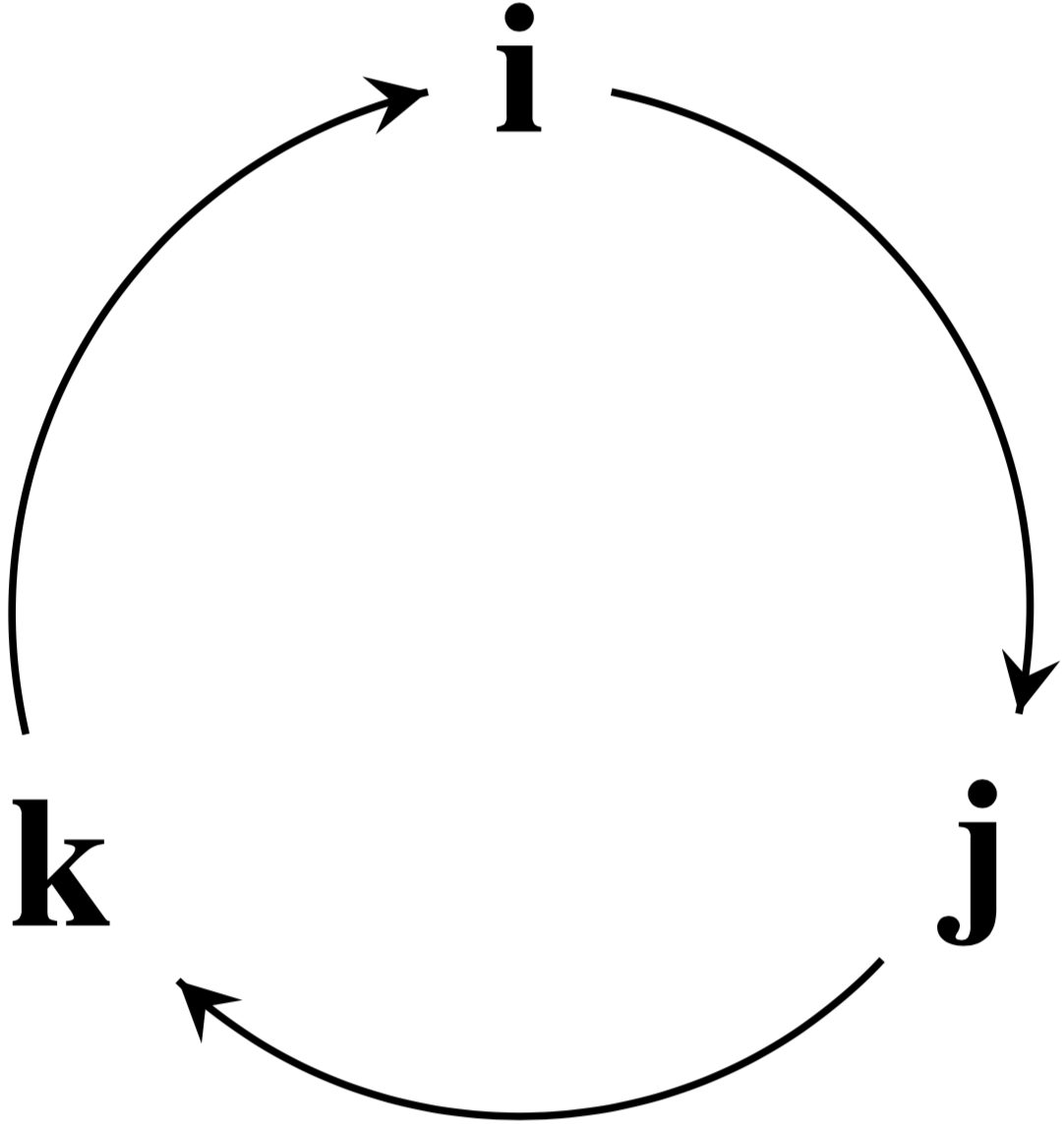
\(\mathbb{H} = \{a + b \boldsymbol{i} + c \boldsymbol{j} + d \boldsymbol{k}\}\)
\(\boldsymbol{i}^2 = \boldsymbol{j}^2 = \boldsymbol{k}^2 = -1\)
\(\boldsymbol{i}\boldsymbol{j}\boldsymbol{k} = -1\)
\(z+w \boldsymbol{j} \mapsto \begin{pmatrix} z & w \\ -\overline{w} & \overline{z}\end{pmatrix}\)
\(\mathbb{H}^d\) is a right vector space over the skew-field \(\mathbb{H}\).
Quaternionic Frames
A spanning set \(\{f_1, \dots , f_N\} \subset \mathbb{H}^d\) is a (finite) frame for \(\mathbb{H}^d\).
Much of standard frame theory goes through for quaternionic frames (see, e.g., Waldron).
In particular, the frame operator \(FF^\ast\) and the Gram matrix \(F^\ast F\) are well-defined, and \(\operatorname{Sp}(d)\) equivalence classes of frames in \(\mathbb{H}^{d \times N}\) are uniquely determined by their Gram matrices.
Realizing Quaternion Matrices as Complex Matrices
\(\Psi:\mathbb{H}^{N \times N} \to \mathbb{C}^{2N \times 2N}\) given by
\(\Psi(Z + W\boldsymbol{j}) = \begin{pmatrix} Z & W \\ -\overline{W} & \overline{Z} \end{pmatrix}\).
\(\Psi(\operatorname{Sp}(N)) \subset SU(2N)\) is the fixed point set of the involution
\(U \mapsto \Omega^\ast \overline{U} \Omega\),
where \(\Omega = \begin{pmatrix} 0 & I \\ -I & 0 \end{pmatrix}\).
Corresponding Cartan decomposition of the Lie algebra:
\(\mathfrak{su}(2N) = \mathfrak{k} \oplus \mathfrak{p} \simeq \Psi(\mathfrak{sp}(N)) \oplus \Psi(\mathscr{H}_0(N))\).
Results
Admissibility for Quaternionic Frames [with Needham]
\(\mathscr{F}_{\boldsymbol{\lambda}}^{\mathbb{H}^d,N}(\boldsymbol{r})\neq \emptyset\) if and only if \(\boldsymbol{r} \in \operatorname{conv}(S_N \cdot (\lambda_1, \dots , \lambda_d, 0, \dots , 0))\) (cf. Casazza–Leon).
\(\mathscr{F}_{\boldsymbol{\lambda}}^{\mathbb{H}^d,N}(\boldsymbol{r}):= \{F \in \mathbb{H}^{d \times N} | \operatorname{spec}(FF^\ast)=\boldsymbol{\lambda}, \|f_i\|^2 = r_i \text{ for all } i=1,\dots, N\}\)
\(\mathcal{O}_{\boldsymbol{\lambda}} = \{U \operatorname{diag}(\lambda_1, \dots , \lambda_d, 0, \dots , 0)U^\ast | U \in \operatorname{Sp}(N)\}\)
Key Idea. Kostant’s convexity theorem.
Generalized Frame Homotopy for Quaternionic Frames [with Needham]
\(\mathscr{F}_{\boldsymbol{\lambda}}^{\mathbb{H}^d,N}(\boldsymbol{r})\) is always path-connected.
Key Idea. Mare’s connectedness theorem.
Questions
Isotropy orbits of real symmetric matrices do not satisfy the technical hypotheses in Mare’s theorem; indeed, some \(\mathscr{F}_{\boldsymbol{\lambda}}^{\mathbb{R}^d,N}(\boldsymbol{r})\) spaces are disconnected. Can we characterize the \((\boldsymbol{\lambda},\boldsymbol{r})\) which lead to connectedness?
Principal isotropy orbits are examples of isoparametric submanifolds; does this give any insight into the uniform measure on real or quaternionic FUNTFs?
Is there an analog of the eigenstep method for quaternionic frames?
Is there any sensible notion of frames in Clifford algebras?
Thank you!
References
Symplectic geometry and connectivity of spaces of frames
Tom Needham and Clayton Shonkwiler
Advances in Computational Mathematics 47 (2021), no. 1, 5
Admissibility and frame homotopy for quaternionic frames
Tom Needham and Clayton Shonkwiler
Preprint, 2021