Black widow evolution:magnetic braking by an ablated wind

Almog

Yalinewich

Pulsar

Coffee

7.2.20

Mass Loss from a Black Widow

Energy budget

\frac{G M_{\odot}^2}{R_{\odot}} \approx 10^{48} \rm \, erg

L_{\odot} t_H \approx 10^{50} \, \rm erg

Efficiency?

Albedo?

(regressive energy distribution)

Extremely Low Mass Companion

PSR J1719-1438 and PSR J2322-2650

Parker Wind

\frac{\partial}{\partial r} \left(\rho v r^2\right) =0

p = \frac{\rho k T}{\mu}

\rho v \frac{\partial v}{\partial r} + \frac{\partial p}{\partial r} = - \frac{G M \rho}{r^2}

\frac{1}{2} \left(1 - \frac{k T}{\mu v^2} \right ) \frac{\partial v^2}{\partial r} = \frac{2 k T}{\mu r} - \frac{G M}{r^2}

Sonic Point

c = v

R_b \approx \frac{G M}{c^2}

\left(1 - \frac{c^2}{v^2} \right ) \frac{\partial}{\partial r} \frac{v^2}{2} = \frac{2 c^2}{r} - \frac{G M}{r^2}

r \ll R_g

r \gg R_g

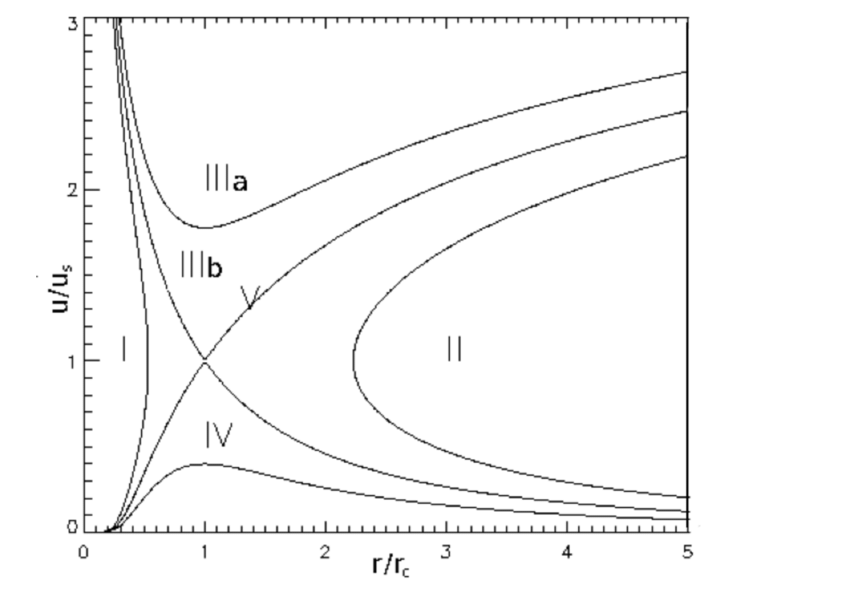
Hydrostatic

Coasting

Analytic Solution

\rho v \frac{\partial v}{\partial r} + \frac{\partial p}{\partial r} = - \frac{G M \rho}{r^2}

\rho v \frac{\partial v}{\partial r} + c^2\frac{\partial \rho}{\partial r} = - \frac{G M \rho}{r^2}

\frac{1}{2} v^2 + c^2 \ln \frac{\rho}{\rho_0} + \frac{G M}{R} - \frac{G M}{r} = 0

\rho_b \approx \rho_0 \exp \left(-R_b/R\right)

Complication: Heating & Cooling

\left(1 - \frac{c^2}{v^2} \right ) \frac{\partial}{\partial r} \frac{v^2}{2} = \frac{2 c^2}{r} - \frac{G M}{r^2} + \frac{\partial c^2}{\partial r}

R_b \approx \frac{G M}{c^2} / \left(1+\frac{d \ln c}{d \ln r}\right)

Mass flux

\dot{M} \approx \rho_b R_b^2 c

Radiative Processes

Heating

Inelastic Compton Scattering

Ionisation

H \approx \sigma F n x

\sigma \approx r_e^2

\sigma \approx \lambda r_e \left(\frac{E}{\varepsilon}\right)^3

lines

Inelastic Compton Scattering

x \approx \varepsilon /m_e c^2

Cooling

free free

free bound

C \approx \Lambda n^2

C_{ff} \approx \alpha \frac{m_e c^3}{r_e^4} \left(n r_e^3 \right)^2 \sqrt{\frac{k T}{m_e c^2}}

C_{fb} \approx \alpha^3 \frac{m_e c^3}{r_e^4} \left(n r_e^3 \right)^2 \sqrt{\frac{m_e c^2}{k T}}

lines

Cooling Function

Difficulty in cooling past 10,000 K

Minimum Pressure

p \propto \rho \cdot 10^4 K\propto \rho

High densities

constant temperature

Low densities

ff cooling

T \propto \frac{1}{\rho^2}

p \propto \rho T \propto \frac{1}{\rho}

minimum pressure

p_0

Temperature Scales

k T_g \approx \frac{G m}{R}

Escape temperature

Inverse Compton temperature

k T_{IC} \approx \varepsilon

Characteristic temperature

k T_{ch} \approx H \frac{R}{c_{ch}}

k T_{ch} \approx \left(\sigma^2 F^2 R^2 \mu\right)^{1/3}

Temperature Scales

k T_g \approx \frac{G m}{R}

Escape temperature

Inverse Compton temperature

k T_{IC} \approx \varepsilon

Characteristic temperature

k T_{ch} \approx H \frac{R}{c_{ch}}

k T_{ch} \approx \left(\sigma^2 F^2 R^2 \mu\right)^{1/3}

Hot Wind

T_g < T_{IC} < T_{ch}

Sonic point is extremely close to the surface

\frac{R_b}{R} - 1 \ll 1

Mass loss rate

\rho_b \approx \frac{p_0}{c_{IC}^2} \approx \frac{\mu p_0}{k T_{IC}}

\dot{M} \approx R^2 \rho_b c_{IC}

Intermediate Wind

T_g < T_{ch} < T_{IC}

Sonic point is withing a few stellar radii

R_b \approx R

Mass loss rate

\rho_b \approx \frac{p_0}{c_{ch}^2} \approx \frac{\mu p_0}{k T_{ch}}

\dot{M} \approx R^2 \rho_b c_{ch}

Cold Wind

T_{ch} < T_g < T_{IC}

Sonic point is much larger than stellar radius

R_b \gg R

speed of sound

c_s \approx v_g

\dot{M} \approx \frac{p_0}{c_{ch}} \frac{T_{ch}^2}{T_g^2}

velocity

k T_g \approx H \frac{R}{v}

mass loss

Roche Lobe Overflow

Gas escapes through narrow angle

\theta_g \approx \sqrt{\frac{T_{ch}}{T_g}}

Net mass loss

\dot{m} \approx R^2 \theta_g^2 \frac{p_0}{c_{ch}}

Mass Loss

Application

Characteristic temperature from Compton heating

k T_{ch} \approx \mu^{1/3} \left(\sigma_T F R\right)^{1/3}

Efficiency

\frac{G m \dot{m}}{R} \approx - \eta L_{\gamma} \left(\frac{R}{a}\right)^2

\eta \approx 2.2 \cdot 10^{-4} \left(\frac{L_{\gamma}}{L_{\odot}}\right)^{1/3} \left(\frac{m}{10^{-2} M_{\odot}}\right)^{1/9} \left(\frac{P_{\rm orb}}{1 \rm \, h}\right)^{-2/9}

Evaporation time

t_{\rm evap} \approx m/\dot{m} \approx

\approx 27 \, {\rm Gyr} \left(\frac{L_{\gamma}}{L_{\odot}}\right)^{-4/3} \left(\frac{m}{10^{-2} M_{\odot}}\right)^{8/9} \left(\frac{P_{\rm orb}}{1 \rm \, h}\right)^{-4/9}

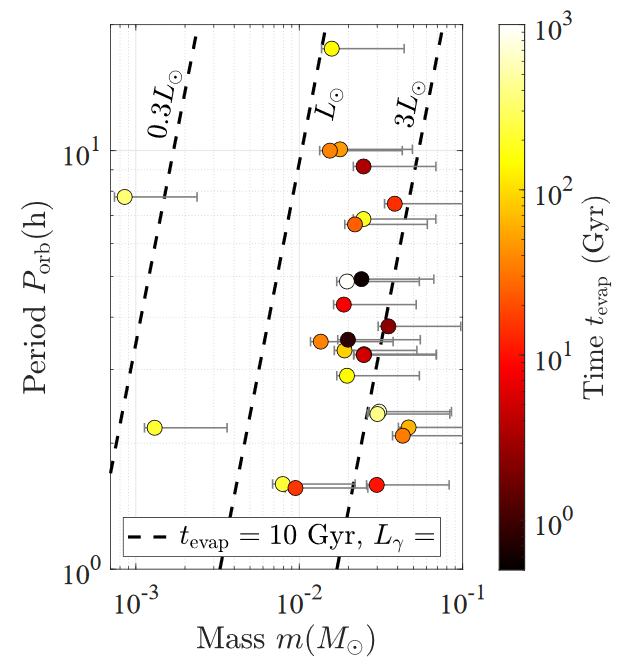
Comparison to Observations

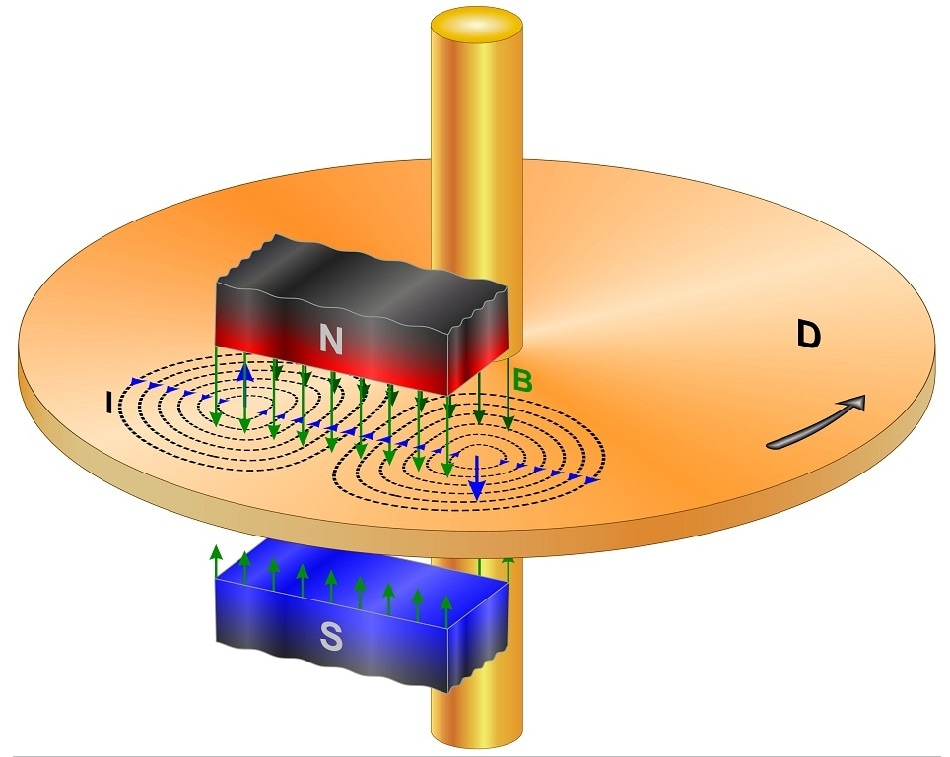
Magnetic Braking

Overview

Synchronised

Lagging

Magnetic breaking

compensation from orbital angular momentum

Net result: Depletion of orbital angular momentum

Alfven Radius

Split monopole magnetic field

B \approx B_0 \left(\frac{R_{NS}}{r}\right)^2

equilibrium between magnetic and ram pressure

B_0^2 R_A^2 \left(\frac{R}{R_A}\right)^4 \approx \dot{m} v \left(R_A\right)

Sun Spin Down

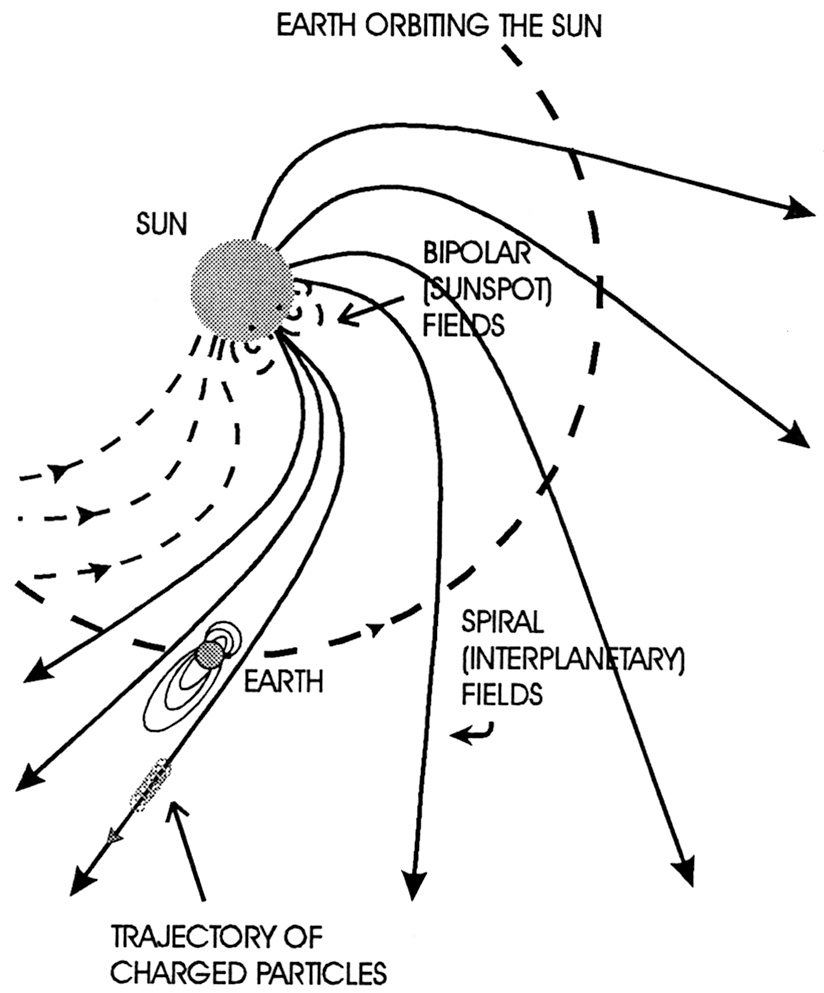
R_A \approx R \sqrt{\frac{B_{\odot}^2 R_{\odot}^2}{\dot{M}_{\odot} v_{\odot}}}

Matter is co - rotating up to the Alfven radius, so mass loss required for factor of two spin - down is

\frac{\Delta M}{M} \approx \frac{R^2}{R_A^2}

The sun could have lost enough mass in its lifetime

Spin Down Time

t_{\rm mag} \approx \frac{m}{\dot{m}} \frac{a^2}{R_A^2} \approx

\approx 31 \left(\frac{L_{\gamma}}{L_{\odot}}\right)^{-\frac{4}{9}} \left(\frac{P_{\rm orb}}{1 \, \rm h}\right)^{-\frac{34}{27}} \left(\frac{m}{10^2 M_{\odot}}\right)^{\frac{2}{27}} \left(\frac{B_0}{10^2 \, \rm G}\right)^{-\frac{4}{3}}

Evaporation time determined by

\min \left(t_{\rm evap}, t_{\rm mag}\right)

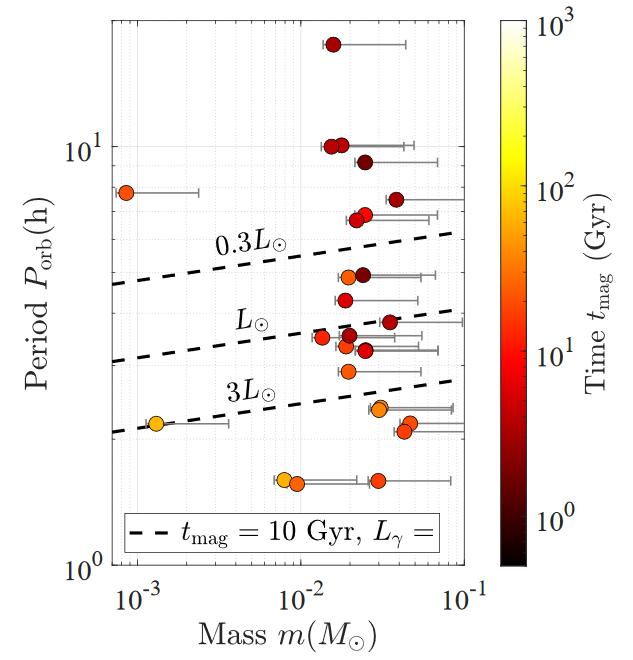
Comparison to Observations

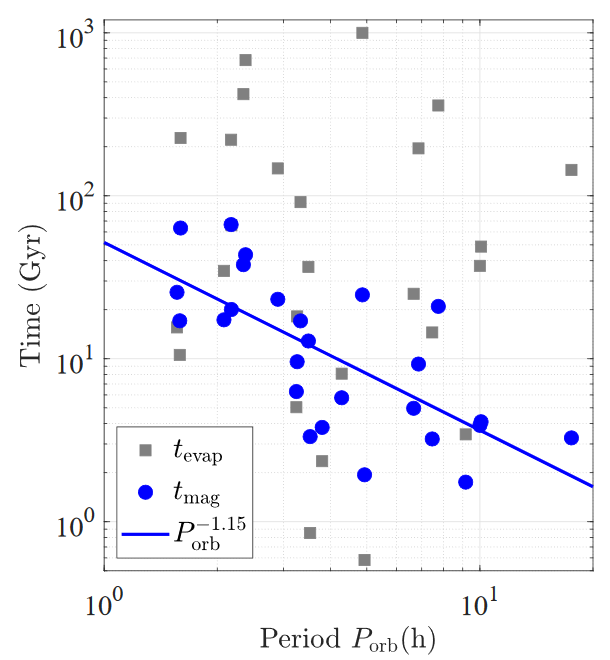
Conclusion

Evaporation with fixed orbital parameters is too slow to explain observations

mass loss is regulated by magnetic braking

questions?

Backup slides

Ionisation

Free Free emission

Free Bound emission