An Elusive Limit

f(x) = \frac{\sin(\tan x) - \tan(\sin x)}{\arcsin(\arctan x) - \arctan(\arcsin x)}
f(x)=sin(tanx)−tan(sinx)arcsin(arctanx)−arctan(arcsinx)f(x) = \frac{\sin(\tan x) - \tan(\sin x)}{\arcsin(\arctan x) - \arctan(\arcsin x)}
\displaystyle\lim_{x\to 0}f(x)=?
limx→0f(x)=?\displaystyle\lim_{x\to 0}f(x)=?

1.Use your computer algebra system to

  evaluate \(f(x)\) for \(x = 1, 0.1, 0.01, 0.001, 0.0001\)

  Does it appear that \(f\) has a limit as \(x \to 0\) ?

f (1) = 1.183832...

f (0.1) = 1.016736...

f (0.01) = 0.989637...

Wolfram

f(0.001)=0?

 *Infinite expression \(\frac{1}{0}\) encountered

f(0.0001) = Indeterminate

Excel

f(0.001) = 0

f(0.0001) = 0

2. Use the CAS to graph \(f\) near \(x=0\).

   Does it appear that

   \(f\) has a limit as \(x \to 0 \) ?

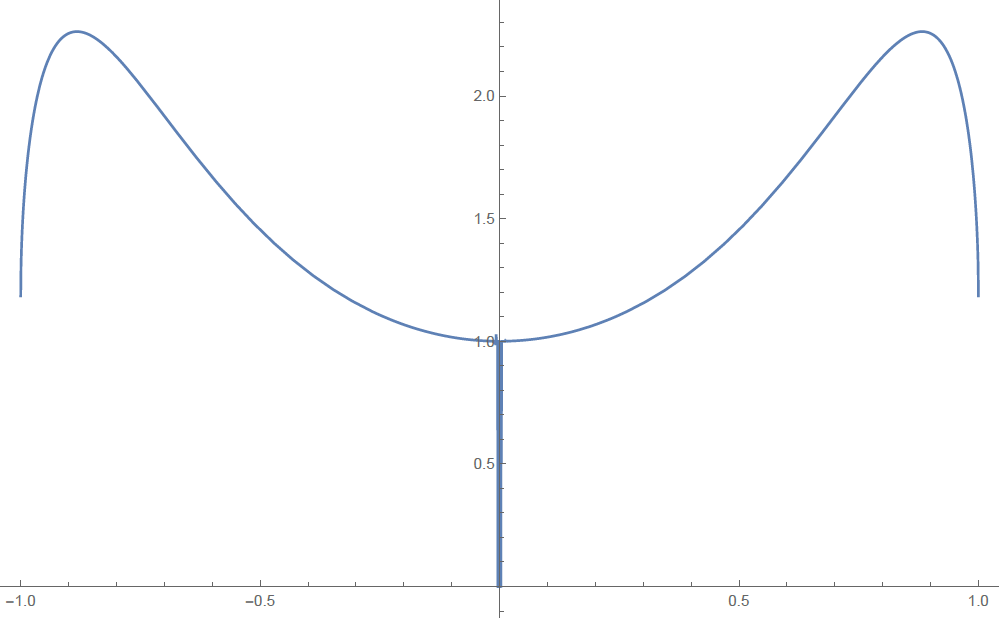
x \in[-1, 1]
x∈[−1,1]x \in[-1, 1]
x \in[-0.1, 0.1]
x∈[−0.1,0.1]x \in[-0.1, 0.1]
x \in[-0.01, 0.01]
x∈[−0.01,0.01]x \in[-0.01, 0.01]

3.Try to evaluate \(\displaystyle\lim_{x \to 0} f(x) \) with l'Hospital's

   Rule, using CAS to find derivatives of

   the numerator and denominator.

   What do you discover?

   How many applications of l'Hospital's

   Rule are required?

g(x)=\sin(\tan x) - \tan(\sin x)
g(x)=sin(tanx)−tan(sinx)g(x)=\sin(\tan x) - \tan(\sin x)
h(x)=\sin^{-1}(\tan^{-1}x) - \tan^{-1}(\sin^{-1}x)
h(x)=sin−1(tan−1x)−tan−1(sin−1x)h(x)=\sin^{-1}(\tan^{-1}x) - \tan^{-1}(\sin^{-1}x)
\text{let }f(x) = \frac{g(x)}{h(x)}
let f(x)=g(x)h(x)\text{let }f(x) = \frac{g(x)}{h(x)}
g'(0)=g''(0)=g'''(0)=g^{(4)}(0)=g^{(5)}(0)=g^{(6)}(0)=0
g′(0)=g′′(0)=g′′′(0)=g(4)(0)=g(5)(0)=g(6)(0)=0g'(0)=g''(0)=g'''(0)=g^{(4)}(0)=g^{(5)}(0)=g^{(6)}(0)=0
h'(0)=h''(0)=h'''(0)=h^{(4)}(0)=h^{(5)}(0)=h^{(6)}(0)=0
h′(0)=h′′(0)=h′′′(0)=h(4)(0)=h(5)(0)=h(6)(0)=0h'(0)=h''(0)=h'''(0)=h^{(4)}(0)=h^{(5)}(0)=h^{(6)}(0)=0
g^{(7)}(0)=h^{(7)}(0)=-\frac{7!}{30}
g(7)(0)=h(7)(0)=−7!30g^{(7)}(0)=h^{(7)}(0)=-\frac{7!}{30}

4.Evaluate \( \displaystyle\lim_{x \to 0} f(x) \) by using the CAS to find

   sufficiently many terms in the Taylor series of

   the numerator and denominator.

   (Use the command taylor in Maple or Series in Mathematica.)

\sin(\tan x) - \tan(\sin x)
sin(tanx)−tan(sinx)\sin(\tan x) - \tan(\sin x)
=-\frac{x^7}{30}-\frac{29x^9}{756}-\frac{1913x^{11}}{75600}+O(x^{13})
=−x730−29x9756−1913x1175600+O(x13)=-\frac{x^7}{30}-\frac{29x^9}{756}-\frac{1913x^{11}}{75600}+O(x^{13})
\sin^{-1}(\tan^{-1}x) - \tan^{-1}(\sin^{-1}x)
sin−1(tan−1x)−tan−1(sin−1x)\sin^{-1}(\tan^{-1}x) - \tan^{-1}(\sin^{-1}x)
=-\frac{x^7}{30}-\frac{13x^9}{756}-\frac{2329x^{11}}{75600}+O(x^{13})
=−x730−13x9756−2329x1175600+O(x13)=-\frac{x^7}{30}-\frac{13x^9}{756}-\frac{2329x^{11}}{75600}+O(x^{13})

5. Use the limit command on your

   CAS to find \(\displaystyle \lim_{x \to 0}f(x)\) directly.

   (Most computer algebra systems use the method

     of Problem 4 to compute limits.)

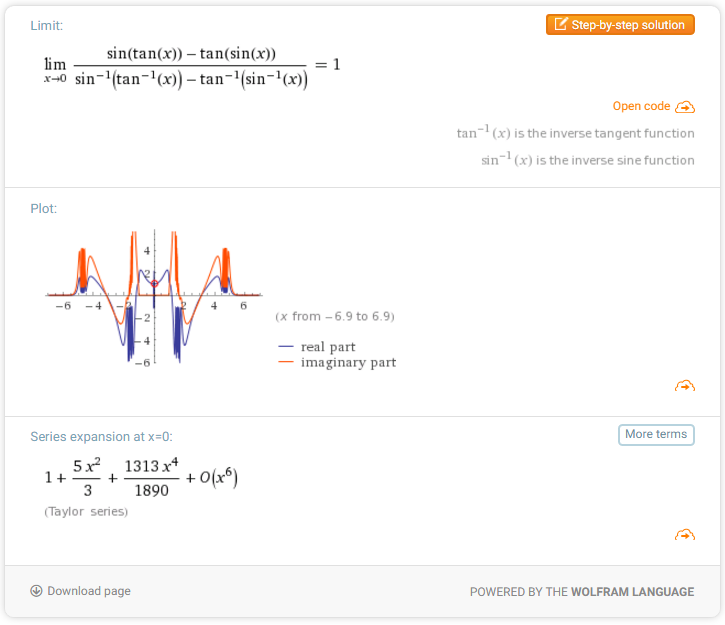
6. In view of the answers to Problems 4

   and 5, how do you explain the results of

   Problems 1 and 2?

Precision, Oscillation

>>> (sin(tan(t))-tan(sin(t))).series(t, 0.001)
                                  2                                   3
- 6.99960922556642e-16⋅(t - 0.001)  - 1.16678888772981e-12⋅(t - 0.001) 
                                  4     ⎛           5           ⎞
 - 1.16667149988666e-9⋅(t - 0.001)   + O⎝(t - 0.001) ; t → 0.001⎠


>>> (asin(atan(t))-atan(asin(t))).series(t, 0.001)
                                                       2
2.16840434497101e-19 - 7.00286183208387e-16⋅(t - 0.001) 
                                   3   ⎛           4           ⎞
 - 1.16670562100296e-12⋅(t - 0.001) + O⎝(t - 0.001) ; t → 0.001⎠

Tayler series at 0.0001 ?

desmos

graph 1 & 2

$ isympy

>>> (sin(tan(x))-tan(sin(x))).series(x, 0, 13)
   7       9         11
  x    29⋅x    1913⋅x      ⎛ 13⎞
- ── - ───── - ──────── + O⎝x  ⎠
  30    756     75600
>>> (asin(atan(x)) - atan(asin(x))).series(x, 0, 13)
   7       9         11
  x    13⋅x    2329⋅x      ⎛ 13⎞
- ── + ───── - ──────── + O⎝x  ⎠
  30    756     75600

Sympy

Made with Slides.com