PHYS 207.013

Chapter 11

rolling motion

Instructor: Dr. Bianco

TAs: Joey Betz; Lily Padlow

 

University of Delaware - Spring 2021

https://slides.com/federicabianco/phys20k713_11

Decolonizing science

work and rotational K

Remember:

\Delta K = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2 = W
W = \int_{x_i}^{x_f} F dx
P = \frac{dW}{dt} = F\frac{dx}{dt} = Fv
K = \frac{1}{2}mv^2
K = \frac{1}{2}I\omega^2

For rotation

work and rotational K

Remember:

\Delta K = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2 = W
W = \int_{x_i}^{x_f} F dx
P = \frac{dW}{dt} = F\frac{dx}{dt} = Fv
K = \frac{1}{2}mv^2
K = \frac{1}{2}I\omega^2
\Delta K = \frac{1}{2}I\omega_f^2 - \frac{1}{2}I\omega_i^2 = W

For rotation

work and rotational K

Remember:

\Delta K = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2 = W
W = \int_{x_i}^{x_f} F dx
P = \frac{dW}{dt} = F\frac{dx}{dt} = Fv
W = \int_{\theta_i}^{\theta_f} \tau d\theta
K = \frac{1}{2}mv^2
K = \frac{1}{2}I\omega^2
\Delta K = \frac{1}{2}I\omega_f^2 - \frac{1}{2}I\omega_i^2 = W

For rotation

work and rotational K

Remember:

\Delta K = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2 = W
W = \int_{x_i}^{x_f} F dx
P = \frac{dW}{dt} = F\frac{dx}{dt} = Fv
W = \int_{\theta_i}^{\theta_f} \tau d\theta
P = \frac{dW}{dt} = \tau\frac{d\theta}{dt} = \tau\omega
K = \frac{1}{2}mv^2
K = \frac{1}{2}I\omega^2
\Delta K = \frac{1}{2}I\omega_f^2 - \frac{1}{2}I\omega_i^2 = W

For rotation

work and rotational K

Remember:

\Delta K = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2 = W
W = \int_{x_i}^{x_f} F dx
P = \frac{dW}{dt} = F\frac{dx}{dt} = Fv
W = \int_{\theta_i}^{\theta_f} \tau d\theta
P = \frac{dW}{dt} = \tau\frac{d\theta}{dt} = \tau\omega
K = \frac{1}{2}mv^2
K = \frac{1}{2}I\omega^2
\Delta K = \frac{1}{2}I\omega_f^2 - \frac{1}{2}I\omega_i^2 = W

For rotation

rolling kinematics

rolling kinematics

s = \theta R\\ v = \omega R\\ v_{CoM} = \omega R

rolling kinematics

rotational inertia abour axis of rotation P

K = \frac{1}{2}I_p\omega^2

P

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

K = \frac{1}{2}(I_{CoM} + MR^2)\omega^2 = \\ \frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}MR^2\omega^2

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

K = \frac{1}{2}(I_{CoM} + MR^2)\omega^2 = \\ \frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}MR^2\omega^2
v_{CoM} = \omega R

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

K = \frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}Mv_{CoM}^2

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

K = \frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}Mv_{CoM}^2

K associated to rotation

rolling kinematics

rotational inertia abour axis of rotation P

P

K = \frac{1}{2}I_p\omega^2

Parallel axis theorem

CoM

I_P = I_{CoM} + MR^2

https://slides.com/federicabianco/phys207013_10#/7

K = \frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}Mv_{CoM}^2

K associated to rotation

K associated to translation

rolling kinematics

rolling kinematics: down a ramp

N

mg

fs

maCOM

a_{CoM} = - \frac{g \sin{\theta}}{1+\frac{I_{CoM}}{MR^2}}

rolling kinematics: down a ramp

N

mg

fs

maCOM

\theta = 90^o
a_{CoM} = - \frac{g}{1+\frac{I_{CoM}}{MR_0^2}}
a_{CoM} = - \frac{g \sin{\theta}}{1+\frac{I_{CoM}}{MR^2}}

=>

rolling kinematics: a yoyo

N

mg

fs

maCOM

\theta = 90^o
a_{CoM} = - \frac{g}{1+\frac{I_{CoM}}{MR_0^2}}
a_{CoM} = - \frac{g \sin{\theta}}{1+\frac{I_{CoM}}{MR^2}}

=>

rolling kinematics: a yoyo

rolling kinematics: energy 

h

U =
mgh
K =
\frac{1}{2}I_{CoM}\omega^2 + \frac{1}{2}m v_{CoM}^2
v_{h=0, CoM} =
U = \frac{1}{2}\frac{I_{CoM}}{R^2}v_{0,CoM}^2 + \frac{1}{2}m v_{0,CoM}^2
\sqrt{\frac{2mgh}{\frac{I_{CoM}}{R ^2} + m} }
U = \frac{1}{2}v_{0,CoM}^2(\frac{I_{CoM}}{R^2} + m)

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\vec{v}

0

\vec{r}

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\vec{v}

0

\vec{r}=0

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})

Angular momentum conservation

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})

Angular momentum conservation

\vec{L} = \sum{m_i\vec{r_i}\times\vec{v_i}} = \sum{\vec{l_i}}

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\vec{L} = \sum{\vec{l_i}}

Angular momentum conservation

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})

Angular momentum conservation

L_{CoM}

CoM

= const

M

\vec{L} = \sum{\vec{l_i}} = \sum m_i r_i v_i = \sum m_i r_i^2 \omega_i

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\vec{L} = \sum{\vec{l_i}}

Angular momentum is conserved!

L_{CoM}

if the object rotates along an axis that goes through its CoM the Angular Momentum is Conserved (sometimes we call it the "Spin")

= const

Angular momentum

Angular momentum  of a rigid body

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\frac{d\vec{v}}{dt} + \frac{d\vec{r}}{dt}\times\vec{v}\right)
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\vec{a} + \vec{v}\times\vec{v}\right)
\frac{d\vec{l}}{dt} =\vec{r}\times m\vec{a} = \tau
\frac{d\vec{L}}{dt} =\sum\vec{r}\times \vec{F} = \tau_{net}

Angular momentum

Angular momentum  of a rigid body

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\frac{d\vec{v}}{dt} + \frac{d\vec{r}}{dt}\times\vec{v}\right)
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\vec{a} + \vec{v}\times\vec{v}\right)
\frac{d\vec{l}}{dt} =\vec{r}\times m\vec{a} = \tau
\frac{d\vec{L}}{dt} =\sum\vec{r}\times \vec{F} = \tau_{net}
\theta
\vec{F}
\vec{r}

line of action

moment arm

Angular momentum

Angular momentum  of a rigid body

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\frac{d\vec{v}}{dt} + \frac{d\vec{r}}{dt}\times\vec{v}\right)
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\vec{a} + \vec{v}\times\vec{v}\right)
\frac{d\vec{l}}{dt} =\vec{r}\times m\vec{a} = \tau
\frac{d\vec{L}}{dt} =\sum\vec{r}\times \vec{F} = \tau_{net}
\theta
\vec{F}
\vec{r}

moment arm

\vec{F}

Fnet = 0 because of symmetry

line of action

CoM

Angular momentum

Angular momentum  of a rigid body

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\frac{d\vec{v}}{dt} + \frac{d\vec{r}}{dt}\times\vec{v}\right)
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\vec{a} + \vec{v}\times\vec{v}\right)
\frac{d\vec{l}}{dt} =\vec{r}\times m\vec{a} = \tau
\frac{d\vec{L}}{dt} =\sum\vec{r}\times \vec{F} = \tau_{net}
Li =\sum \Delta m_i {r_i}\sin{\theta} {v_i} =\\
\sum \Delta m_i ~{r_{i,p} v_i} =
\sum \Delta m_i (\omega r_{i,p} )r_{i,p} =
\omega \Delta m_ir_{i,p}^2 = I\omega

Angular momentum

\vec{l} = \vec{r}\times \vec{p} =
m(\vec{r} \times \vec{v})
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\frac{d\vec{v}}{dt} + \frac{d\vec{r}}{dt}\times\vec{v}\right)
\frac{d\vec{l}}{dt} = m\left(\vec{r}\times\vec{a} + \vec{v}\times\vec{v}\right)
\frac{d\vec{l}}{dt} =\vec{r}\times m\vec{a} = \tau
\frac{d\vec{l}}{dt} =\sum\vec{r}\times \vec{F} = \tau_{net}
\vec{L} = \sum{\vec{l_i}}
\vec{L} = \vec{I}\times\vec{\omega}

The Angular momentum of a system is conserved IF THERE ARE NO EXTERNAL FORCES

Angular momentum

is the reason why we exist!

Star formation

Angular momentum

is the reason why we exist!

Star formation

Gyroscope

is it magic??? no: its torque + angular momentum.

Gyroscope

is it magic??? no: its torque + angular momentum.

\vec{\tau} = \frac {d\vec{L}}{dt} \\ \tau = Mgr
\vec{L} = I \vec{\omega} \\
dL = \tau dt \\ d\phi = \frac{dL}{L} = \frac{Mgr dt}{I\omega}

this change in the direction of orientation of the axis of rotation of a body is called

PRECESSION

\\ \Omega = \frac{Mgr}{I\omega}

Gyroscope

is it magic??? no: its torque + angular momentum.

Gyroscope

is it magic??? no: its torque + angular momentum.

Gyroscope

is it magic??? no: its torque + angular momentum.

so the blades can change pitch to provide more lift.

on which blade would you provide the lift to tilt the helicopter forward???

we will talk about it in class on Tuesday!

A

B

C

D

Gyroscope

A

D

https://mars.nasa.gov/resources/25828/first-video-of-nasas-ingenuity-mars-helicopter-in-flight/

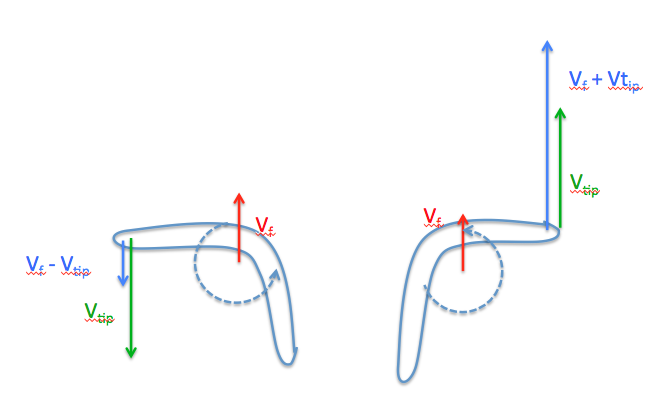
Gyroscope

Gyroscope

summary