Revisiting gravitational wave background from primordial black holes
Heling Deng
Arizona State University
2110.02460
Outline
- Primordial black holes
- PBH binary merger rate
- Gravitational wave background from PBH mergers
- Revisiting PBH binary merger rate
Outline
- Primordial black holes
- PBH binary merger rate
- Gravitational wave background from PBH mergers
- Revisiting PBH binary merger rate
LIGO BH
Supermassive black holes
LIGO black holes
Dark matter
SMBH
Primordial black holes (PBHs)
Astrophysical BHs
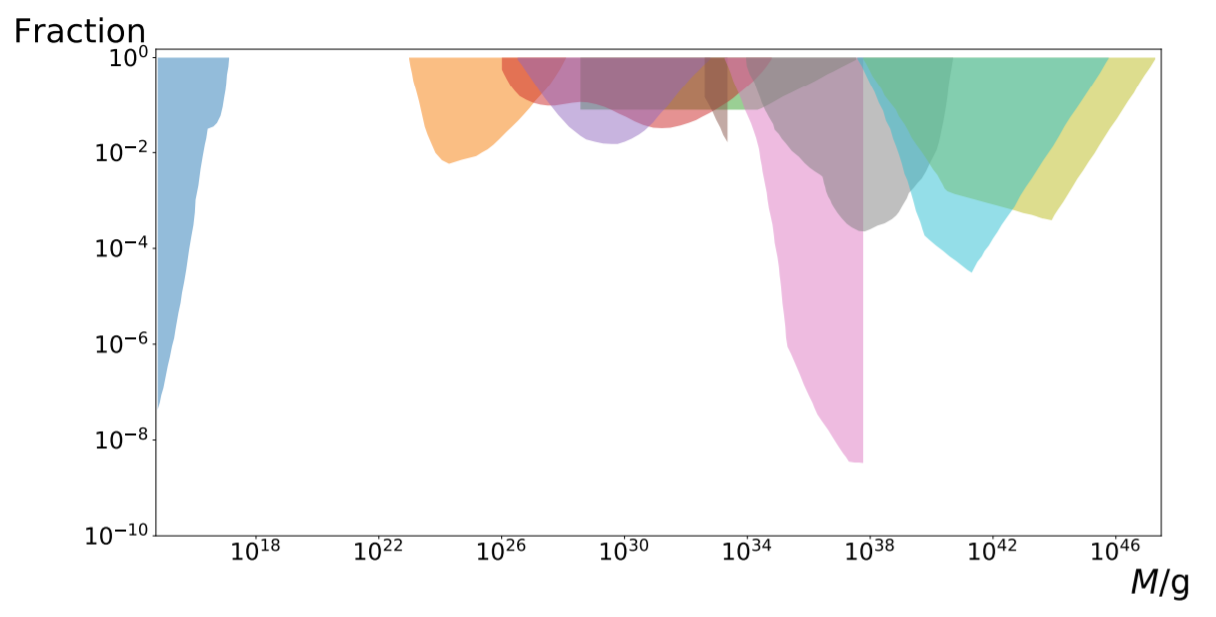
Observational constraints of PBHs as DM
\(M_\odot\sim 10^{33}\ \text{g}\)
Outline
- Primordial black holes
- PBH binary merger rate
- Gravitational wave background from PBH mergers
- Revisiting PBH binary merger rate
PBH binary
PBH binary
Semi-major axis: \(a\)
Coalescence time given by Peters formula
P. C. Peters, Phys. Rev. 136, B1224 (1964)
\(t_P \propto \frac{a^4}{M^3}(1-e^2)^{7/2}\)
Eccentricity: \(e\)
(~ Physical separation when binary forms)
PBH merger rate: \(P(a,e)\to P(t)\)
\(a\)
1603.08338, Sasaki, Suyama, Tanaka and Yokoyama
Examples:
\(M=10M_\odot, f=0.1\% \to t_c\sim 10^{19}\ \text{s}\)
\(M=100M_\odot, f=0.1\% \to t_c\sim 10^{17}\ \text{s}\)
\(t_c=10^{42}f^7\left(\frac{M}{M_\odot}\right)^{-5/3}\ \text{s}\), \(f\) is fraction of DM in PBHs
\(\log(\text{Merger event \# within Hubble time})\)
\(\log(\text{cosmic time})\)
\(t_c\)
\(t^{3/37}\)
\(t^{-1/7}\)
Outline
- Primordial black holes
- PBH binary merger rate
- Gravitational wave background from PBH mergers
- Revisiting PBH binary merger rate
PBH binary
\(a\)
Peters formula
\(t_P \propto \frac{a^4}{M^3}(1-e^2)^{7/2}\)
\(e \to 1, t_P \to 0?\)
Free-fall time: \(t_{ff}\sim \sqrt{\frac{a^3}{M}}\)
Coalescence time can be estimated by Peters formula only if \(t_P > t_{ff}\)
\(t\sim t_P+t_{ff}\)
Coalescence time should be
**Some PBH binaries were formed much later than previously expected**
**Effect significant for huge PBHs**
Example:
\(M=10^5M_\odot, f=0.1\% \to t_c\sim 10^{13}\ \text{s}\)
\(\log(t)\)
\(t_c\)
\(\log(\text{Merger event \# within Hubble time})\)
\(t^{1/2}\)
\(t^{-1/7}\)
Outline
- Primordial black holes
- PBH binary merger rate
- Gravitational wave background from PBH mergers
- Revisiting PBH binary merger rate
1903.05924, Wang, Terada and Kohri
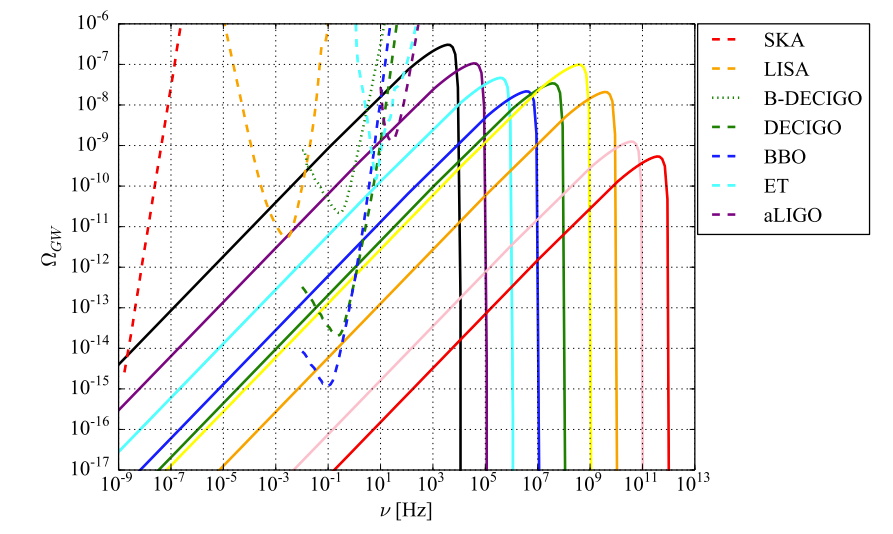
Examples of \(\Omega_{GW}\) from PBH mergers (small PBHs)
\(\Omega_{GW}\) has a peak near \(\nu \sim 10^4(M/M_\odot)^{-1}\ \text{Hz}\)
GWB from PBH mergers
Examples of \(\Omega_{GW}\) from PBH mergers taking into account the "free-fall time" effect

More stringent constraints on PBH abundance if we don’t see GWB in the future
GWB from PBH mergers

Observational constraints of PBHs as DM
\(M_\odot\sim 10^{33}\ \text{g}\)

Observational constraints of large PBHs as DM
Conclusions
- Peters formula underestimates merger rate of large PBHs
- GWB spectrum for mergers of PBHs with \(M>10^5M_\odot\) develops an extra peak
- If future missions do not see such a background, the fraction of dark matter in PBHs is constrained to \(f < 10^{−6} \text{-}10^{−4}\) for \(M\sim10\text{-}10^9M_\odot\)
PBH binary
Peters formula: \(t\propto\frac{x^{37}}{y^{21}} \to x\propto\left(\frac{y^{21}}{t}\right)^{1/37}\)
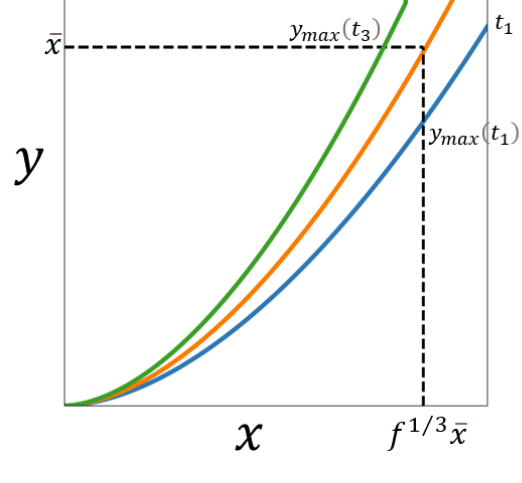
bounds:
- \(x<f^{1/3}\bar{x}\) -- ensure the binary is formed during the radiation era
- \(y<\bar{x}\) -- probability that two PBHs with separation > \(\bar{x}\) is exponentially suppressed
curves with constant \(t\)
Integration largely determined by \(y_{max}\)
\(t_2\)
\(t_3\)
\(t_1>t_2>t_3\)
\(P(t)\propto\int x^2y^2|\frac{\text{d}x}{\text{d}t}|\text{d}y\)
PBH binary
\(P(t)\propto\int x^2y^2|\frac{\text{d}x}{\text{d}t}|\text{d}y\)
results:
\(P(t)\approx\frac{0.005f}{t}\)
{
\(\left(\frac{t}{t_c}\right)^{-1/7},\ t > t_c\)
\(\left(\frac{t}{t_c}\right)^{3/37},\ t < t_c\)
where \(t_c\sim 10^{42}f^7\left(\frac{M}{M_\odot}\right)^{-5/3}\ \text{s}\)

curves with constant \(t\)
\(t_2\)
\(t_3\)
\(t_1>t_2>t_3\)
\(t_2=t_c\)
1603.08338, Sasaki, Suyama, Tanaka and Yokoyama
Example:
\(M=10M_\odot, f=0.1\% \to t_c\sim 10^{19}\ \text{s}\)
\(M=100M_\odot, f=0.1\% \to t_c\sim 10^{17}\ \text{s}\)
Peters formula: \(t\propto\frac{x^{37}}{y^{21}} \to x\propto\left(\frac{y^{21}}{t}\right)^{1/37}\)
PBH binary
Peters formula: \(t= t_P(x,y) \to x=F(y)\)

curves with constant \(t\)
Integration largely determined by \(y_{max}\)
\(t_2\)
\(t_3\)
\(t_1>t_2>t_3\)
\(P(t)\propto\int x^2y^2|\frac{\text{d}x}{\text{d}t}|\text{d}y\)
PBH binary
\(t= t_P+t_{ff} = \frac{3\rho^4}{170M^7}\frac{x^{37}}{y^{21}} + \frac{\rho^{3/2}}{M^2}x^6\)
\(\to y\propto\left(\frac{x^{37}}{M^2\rho^{-3/2}t-x^6}\right)^{1/21}\)

Integration largely determined by \(y_{max}\)
\(t_1>t_2>t_3\)
**PBH binaries could have a significantly larger merger rate "at some point" than previously expected**
\(P(t)\propto\int x^2y^2|\frac{\text{d}x}{\text{d}t}|\text{d}y\)
Peters formula: \(t\propto\frac{x^{37}}{y^{21}} \to x\propto\left(\frac{y^{21}}{t}\right)^{1/37}\)
PBH binary
free-fall time
Hubble time
When do two neighboring PBHs decouple from Hubble flow?
<
free fall time should grow slower than Hubble time
\(\to\)
binary should form during the radiation era
PBH binary
\(y\)
\({x}\)
At dust-radiation equality (\(z_{eq}\sim3000\))
\(\rho\) -- dark matter density
\(M\) -- PBH mass
\(f\) -- fraction of dark matter in PBHs
\(\to\) \(n\sim \bar{x}^{-3} \sim\frac{f\rho}{M}\)
- \(\bar{x}\) -- average physical separation between two PBHs
- \({x}\) -- physical distance between two neighboring PBHs without Newton
- \(y\) -- physical distance from the third nearby PBH to the binary
For a random PBH, the probability that a second BH is at \((x, x+\text{d}x)\) and a third BH is at \((y, y+\text{d}y)\) is
\(P(x,y)\text{d}x\text{d}y\propto x^2y^2\text{d}x\text{d}y\)
\(y\)
\({x}\)
PBH binary
\(\to\) For a random PBH, the probability that a merger occurs at \((t, t+\text{d}t)\) is
\(P(t)\text{d}t\propto\int x^2y^2|\frac{\text{d}x}{\text{d}t}|\text{d}y\text{d}t\)
Peters formula: \(t\propto\frac{x^{37}}{y^{21}} \to x\propto\left(\frac{y^{21}}{t}\right)^{1/37}\)
GWB from PBH mergers
\(\Omega_{GW}(\nu_d)=\frac{\nu_d}{\rho_c}\int N(z)\frac{\text{d}E_{GW}(\nu_s)}{\text{d}\nu_s}\text{d}z\)
- \(N(z)\text{d}z\) -- comoving number density of events at \((z,z+\text{d}z)\)
- \(\nu_d \ (\nu_s)\) -- GW frequency in detector (source)
- \(\frac{\text{d}E_{GW}(\nu_s)}{\text{d}\nu_s}\) -- GW energy spectrum from a single event
For PBH merger:
\(\frac{\text{d}E_{GW}(\nu_s)}{\text{d}\nu_s}\) from numerical work
\(N(z)\) from \(P(t)\)