Primordial black holes from vacuum bubbles
邓鹤凌
Arizona State University
Outline
- Primordial black holes
- Fitting LIGO black holes
- LIGO BHs from bubbles?
- PBHs from bubbles
Outline
- Primordial black holes
- Fitting LIGO black holes
- LIGO BHs from bubbles?
- PBHs from bubbles
LIGO BH
Supermassive black holes
SMBH
...
...
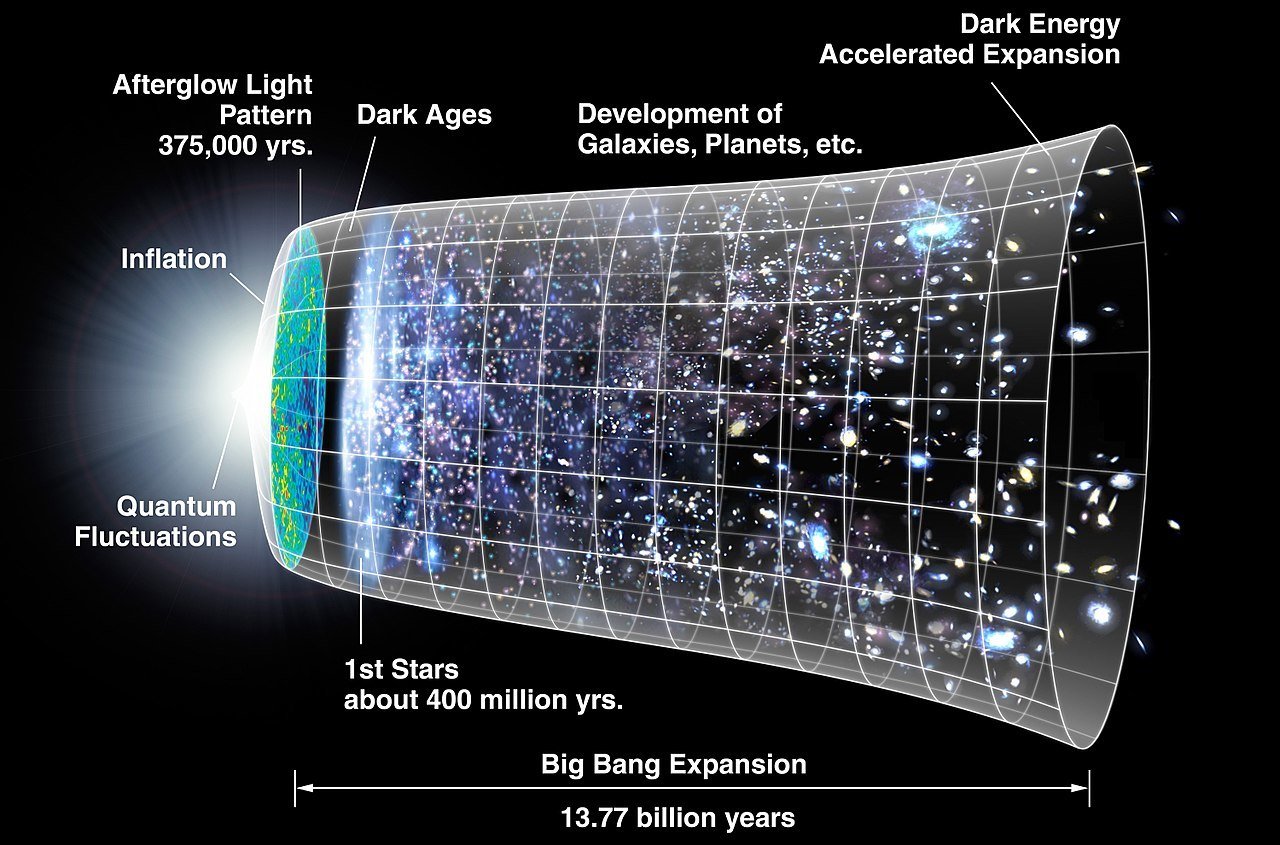
Primordial black holes (PBHs)
Astrophysical BHs
LIGO BH
Supermassive black holes
LIGO black holes
SMBH
...
...
Primordial black holes (PBHs)
Astrophysical BHs
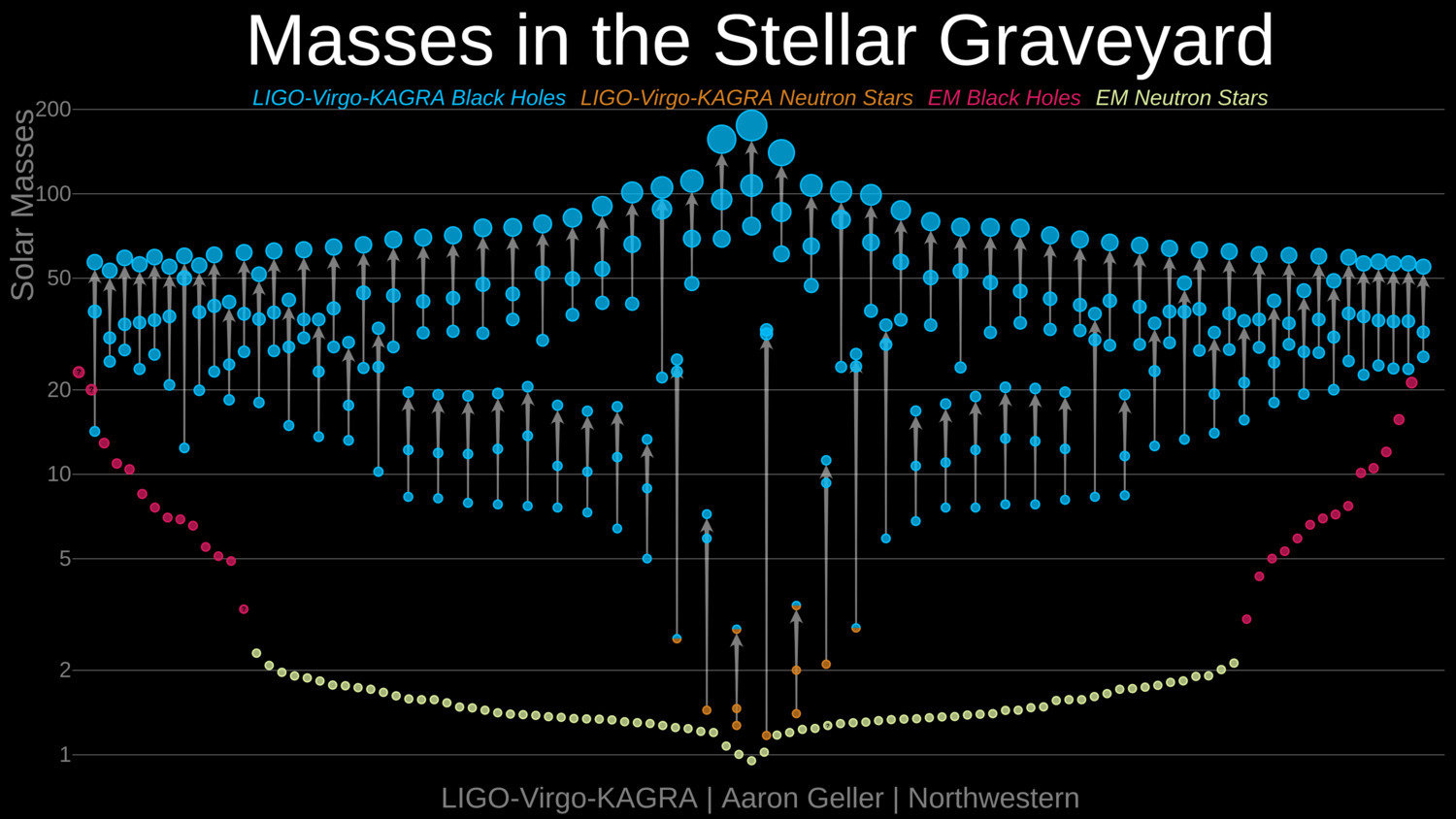
LIGO BHs
LIGO BH
Supermassive black holes
LIGO black holes
Dark matter
SMBH
...
...
Primordial black holes (PBHs)
Astrophysical BHs
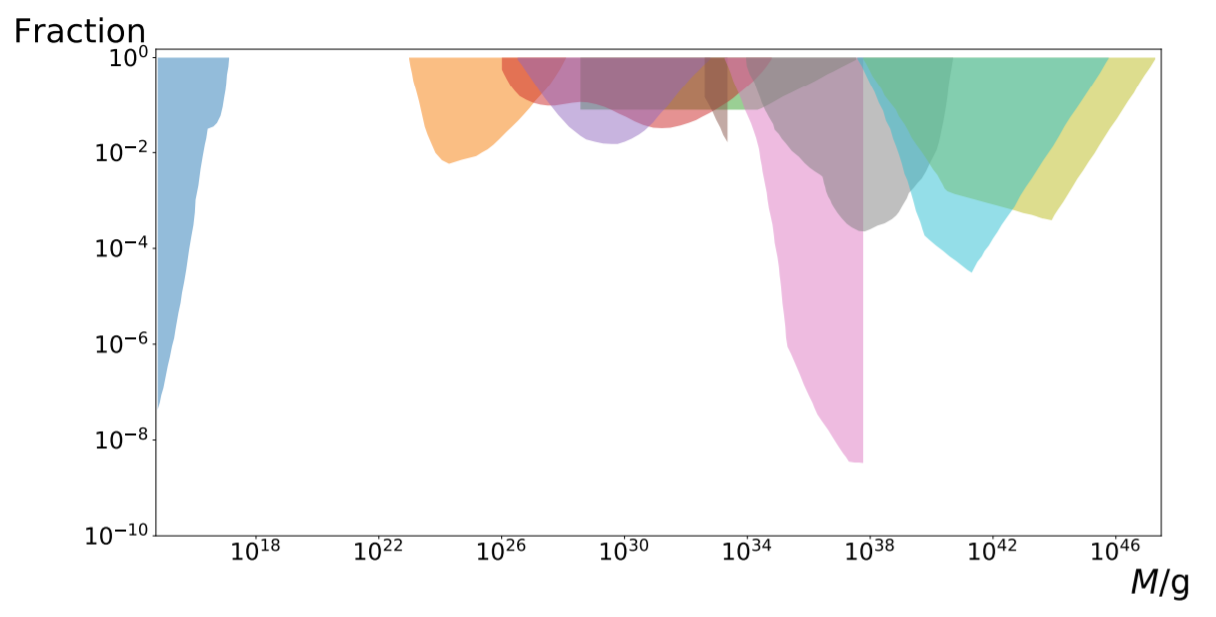
Observational constraints of PBHs as DM
Outline
- Primordial black holes
- Fitting LIGO black holes
- LIGO BHs from bubbles?
- PBHs from bubbles
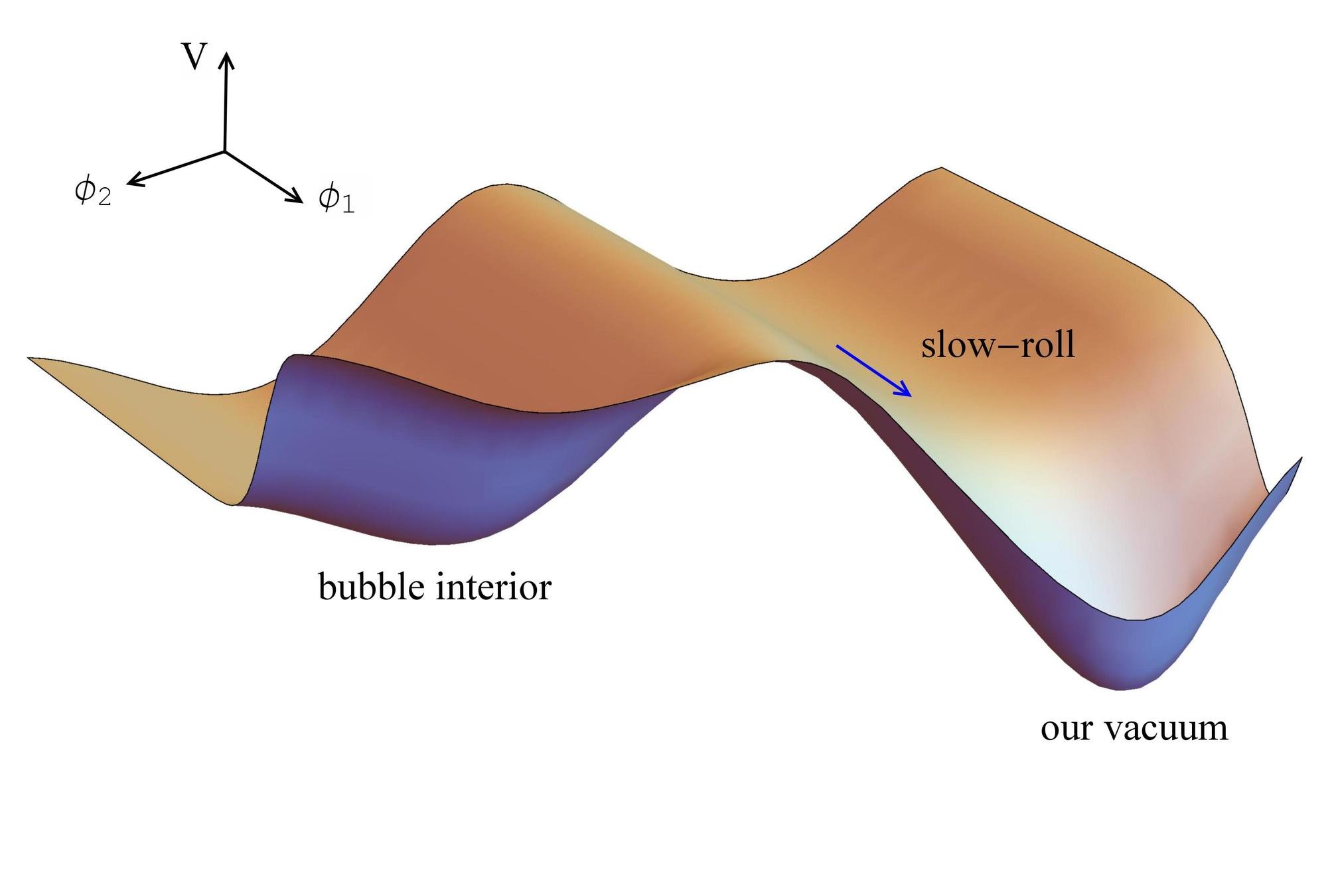


Inflation \(\rho_i \to\) radiation fluid \(\rho_i\)
Bubble interior \(\rho_b<\rho_i\)
Subcritical
Supercritical
Wormhole \(\to\) BH
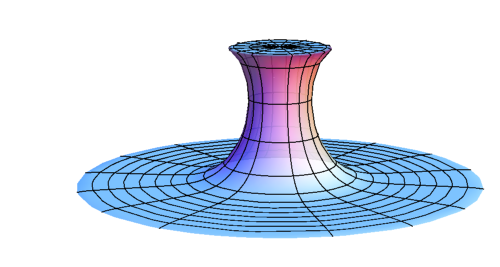

Used numerical simulations of full GR to find relation between BH mass and bubble size \(m(R)\)
subcritical
supercritical
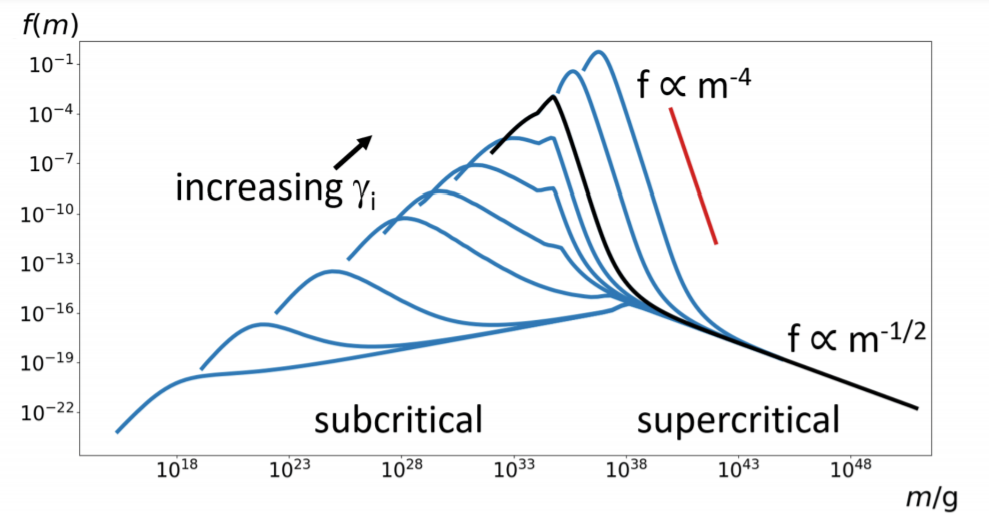
PBH mass function (fraction of DM in PBHs with mass \(\sim m\))
PBH mass density
Number density of bubbles (BHs)
const over time
Broken power law
BH mass distribution from bubbles
subcritical
supercritical
Outline
- Primordial black holes
- Fitting LIGO black holes
- LIGO BHs from bubbles?
- PBHs from bubbles


Mass distribution of LIGO BHs
A simple mass function
PBH mass function
Merger rate
Detection probability
Probability of each event \(p_i(m_1,m_2,z)\)
+
Likelihood of all LIGO events
Mergers reaching earth today
\(f(m)\) characterized by \(f_{PBH}, \alpha_1, \alpha_2, m_*\)
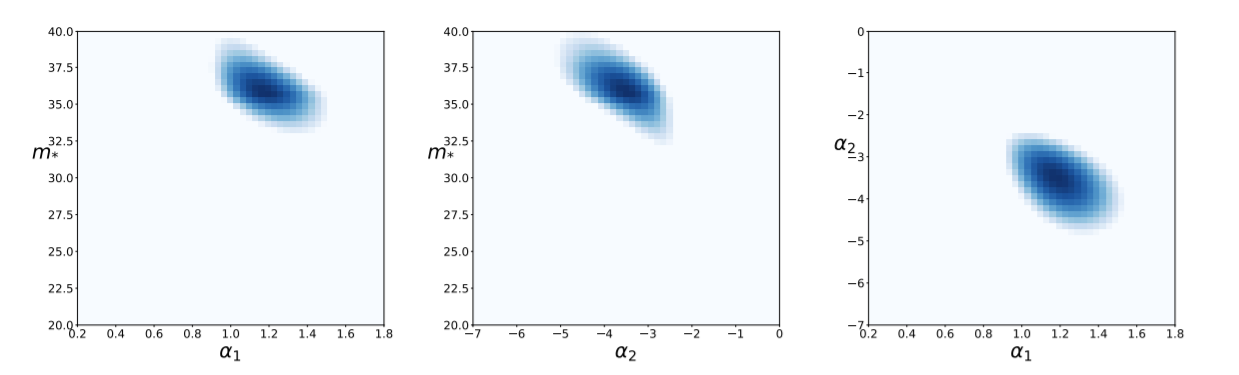
Maximizing \(\mathcal{L}\) in a 4-parameter space

Is there a mechanism that can generate such a mass function?
Outline
- Primordial black holes
- Fitting LIGO black holes
- LIGO BHs from bubbles?
- PBHs from bubbles
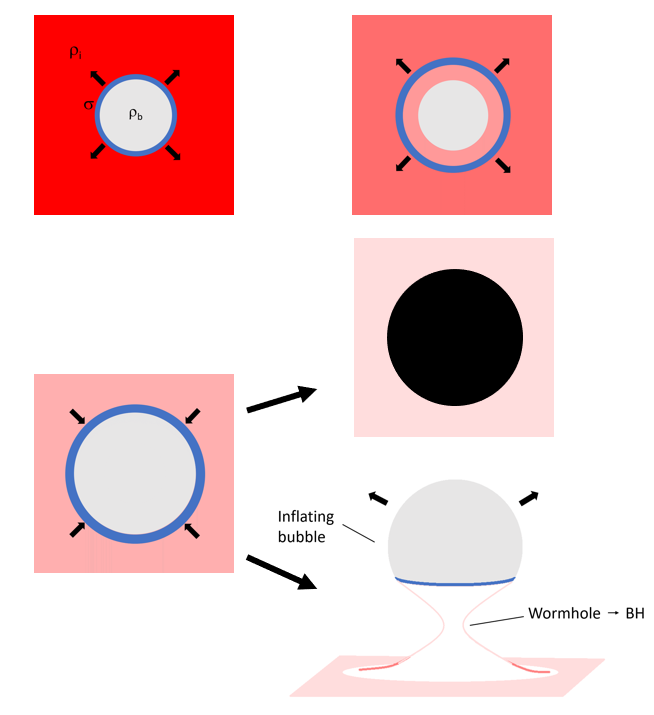
Fluid not get into bubble
Fluid gets into bubble

Observational constraints of PBHs as DM


Conclusions

PBH mass function
Merger rate
Detection probability
Expected number of observable events
Probability of each detected event
+
+
Likelihood of all LIGO events
Empty layer
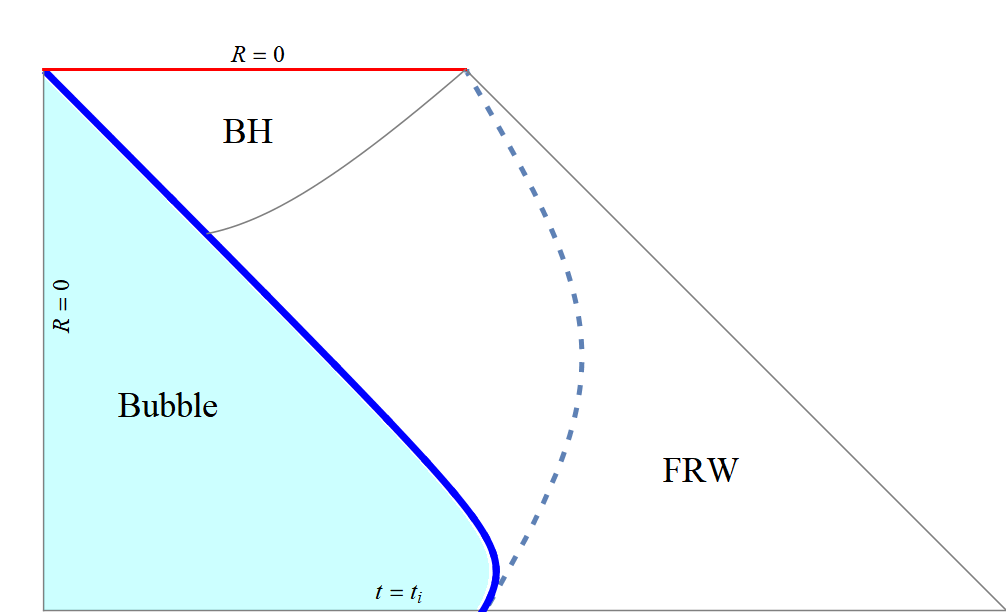
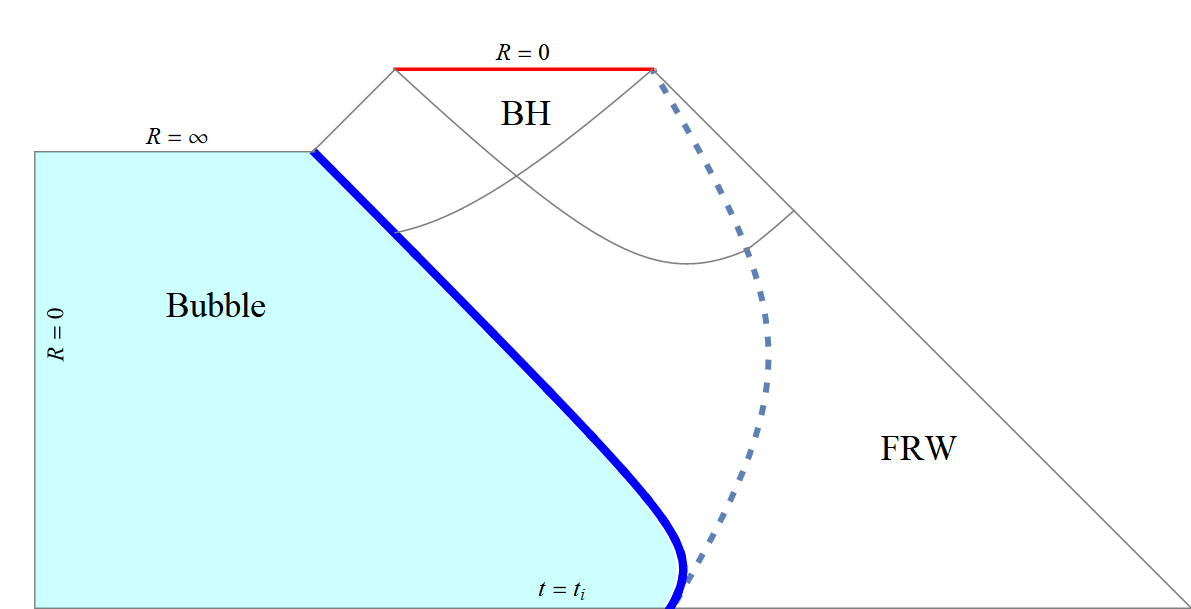
Intrinsic PBH merger rate
(Number of event \((m_1,m_2,z)\) per unit volume per unit time)
Signals reaching earth
(Number of event \((m_1,m_2,z)\) reaching the earth per unit time)
Detection probability \(p_{det}(m_1,m_2,z)\)