Cluster-size decay (Julia)

Cluster-size decay in kernel-based spatial random graphs

Júlia Komjáthy

joint with Joost Jorritsma, Dieter Mitsche

Random Discrete Structures

March 2023

Papers: tiny.cc/cluster-size-ksrg

Cluster-size decay in supercrit. nearest-neighbor percolation

\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)=

\exp\big(-\Theta(k^{\mathbf{\zeta}})\big),\qquad\zeta=\frac{d-1}d

[Grimmett & Marstrand '90, Kesten & Zhang '90]

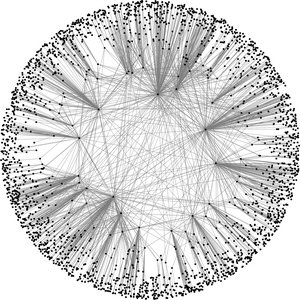
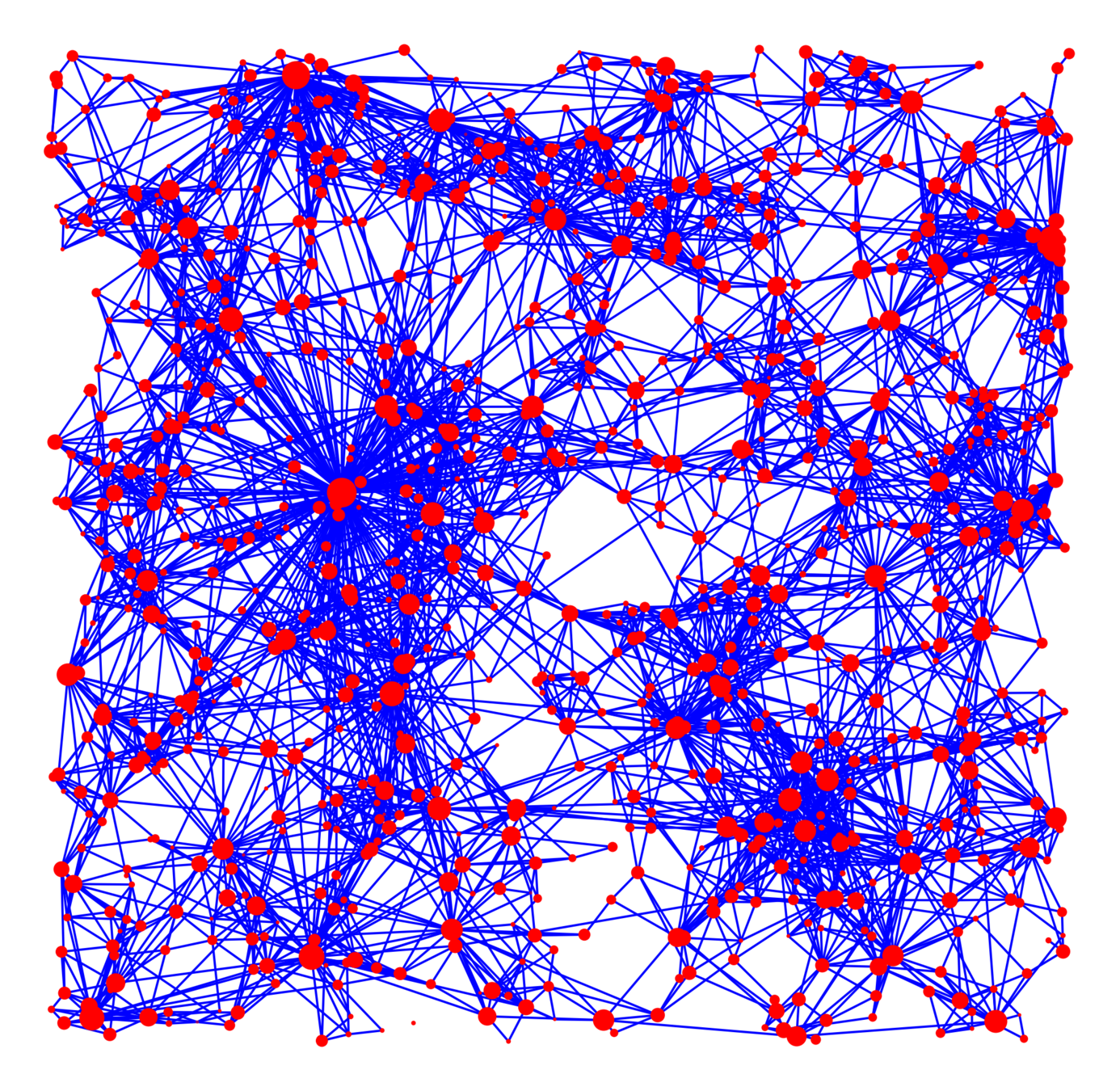
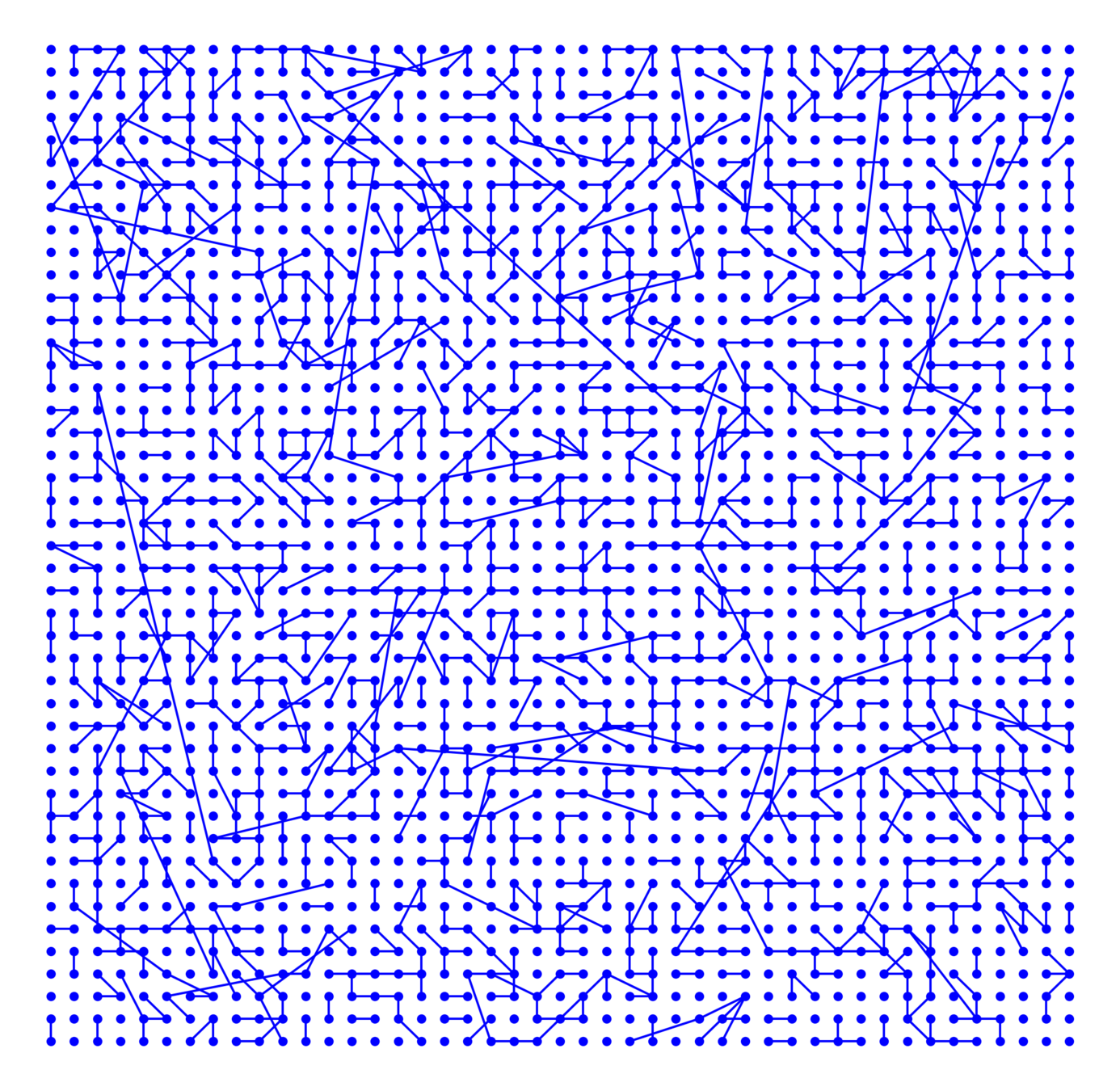
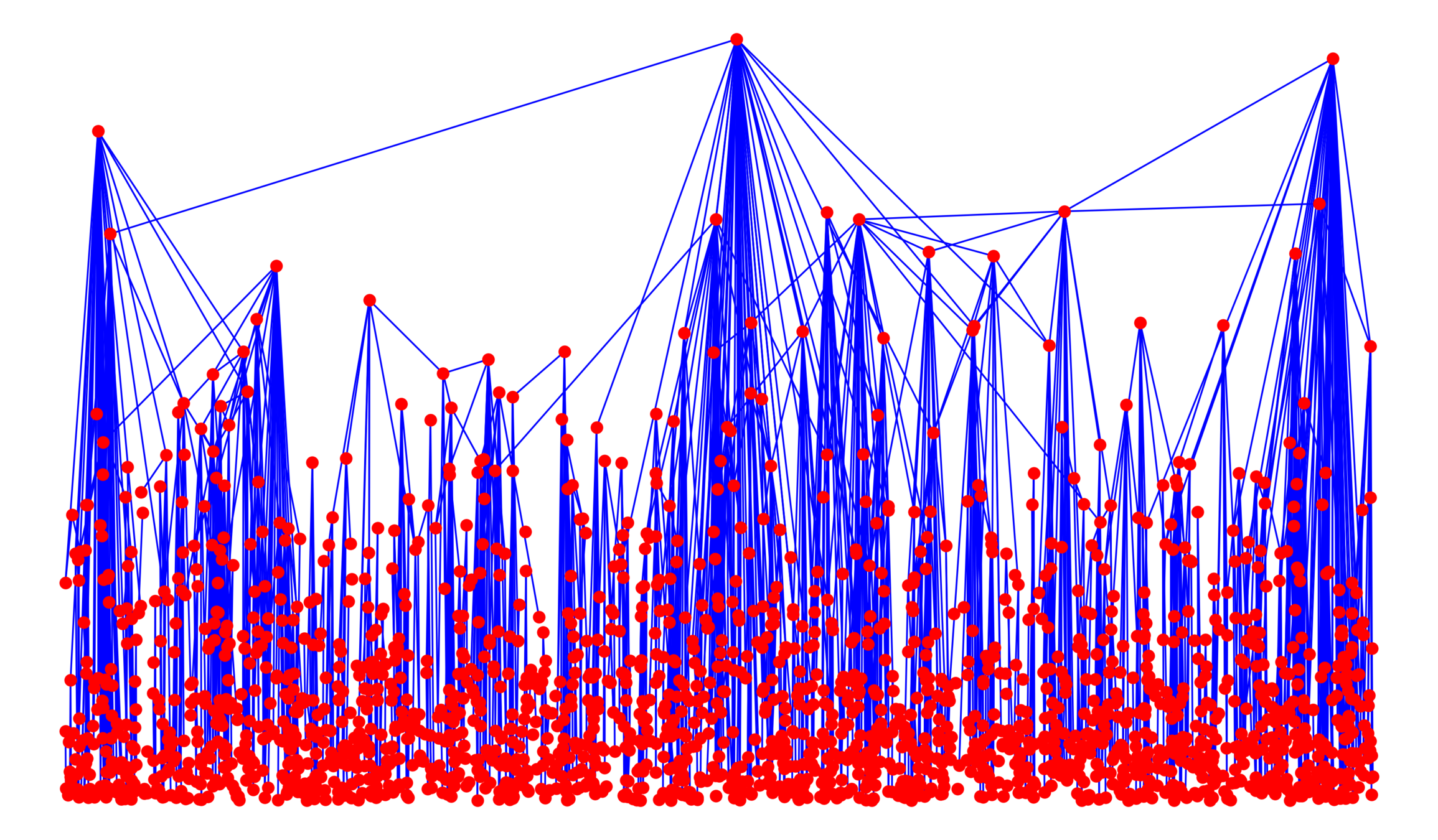
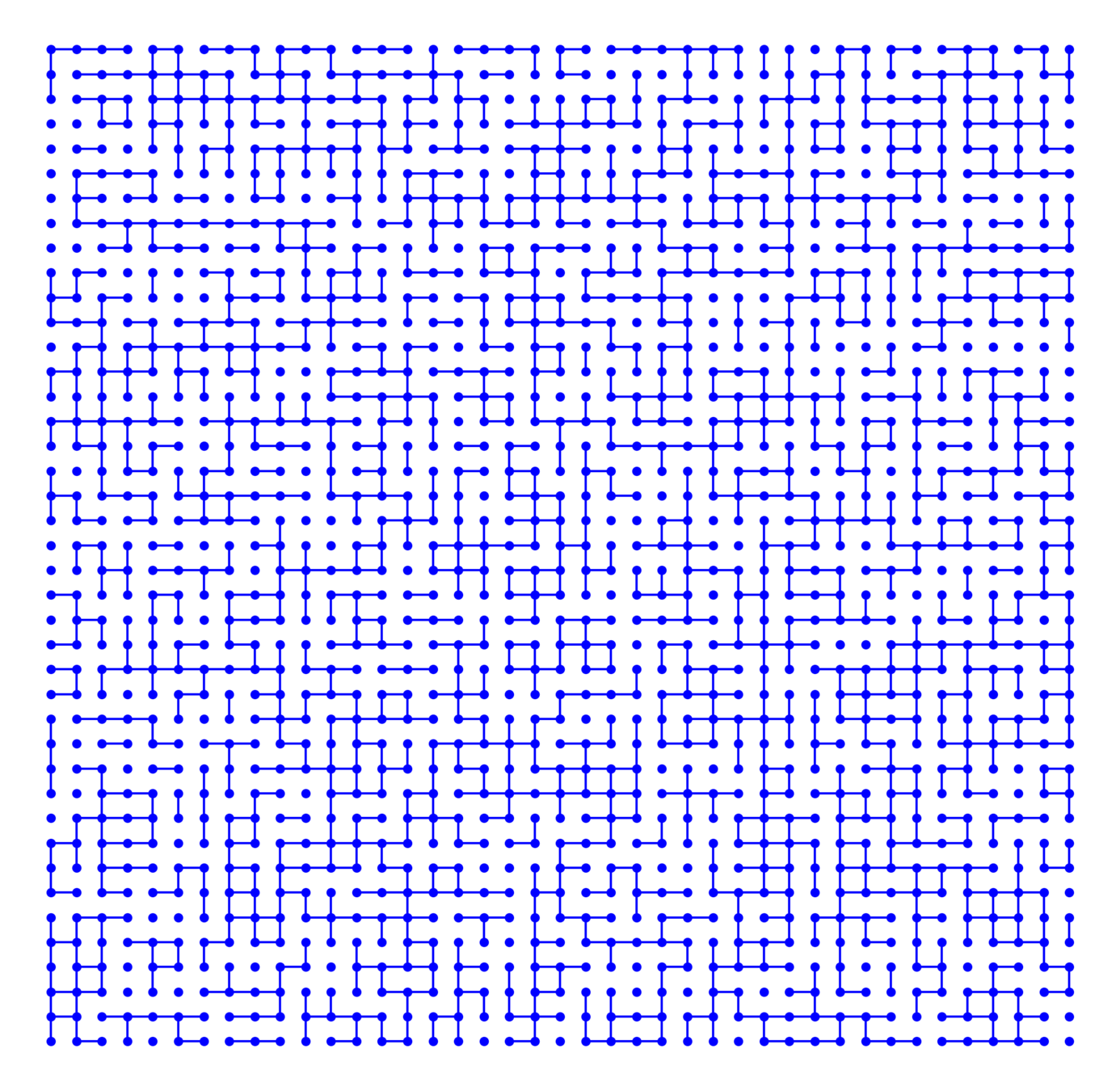
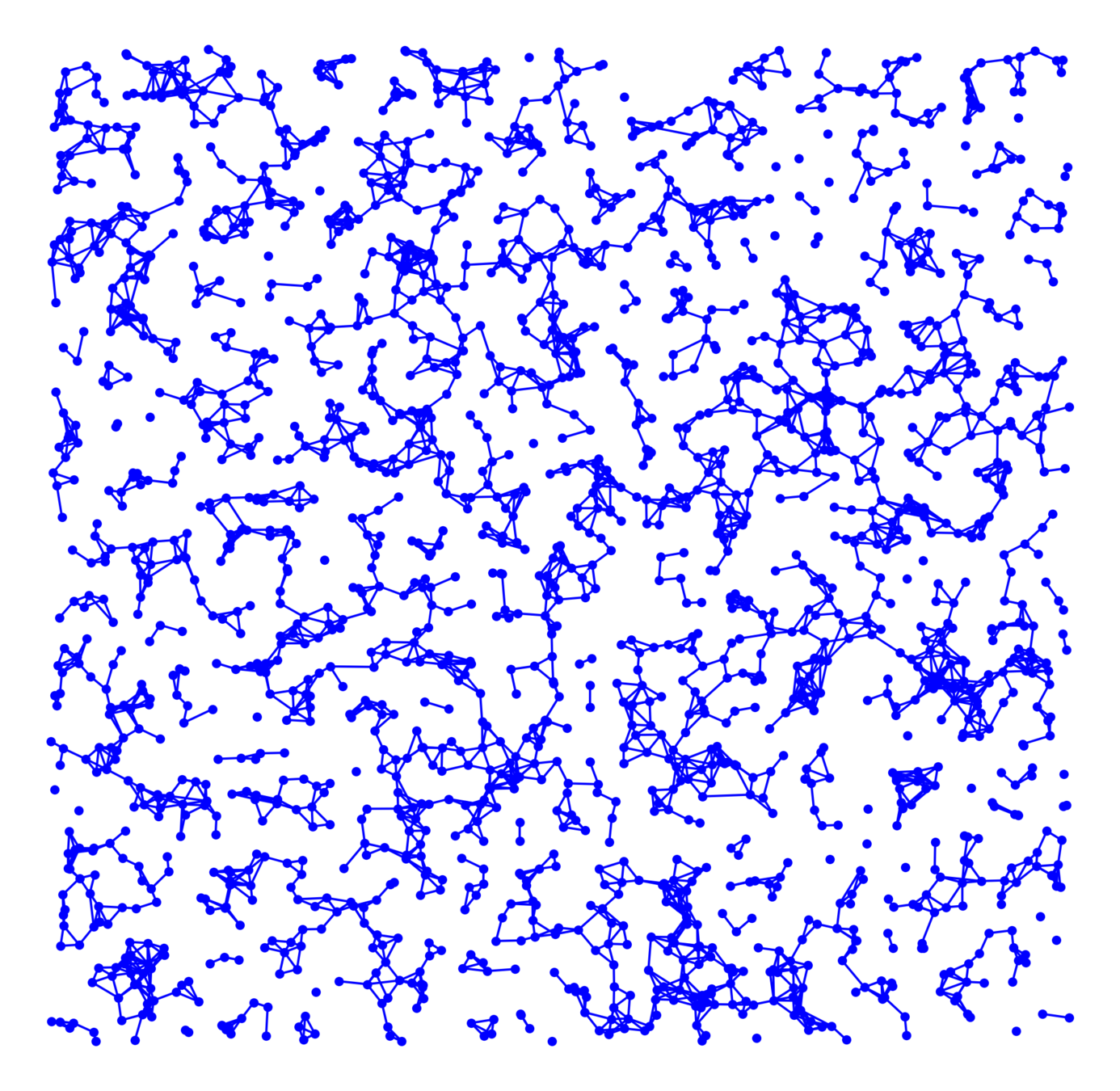
Hyperbolic random graph

Scale-free percolation

Long-range percolation

Age-dependent RCM

Random geom. graph

Nearest-neighbor percolation

Kernel-based spatial random graphs

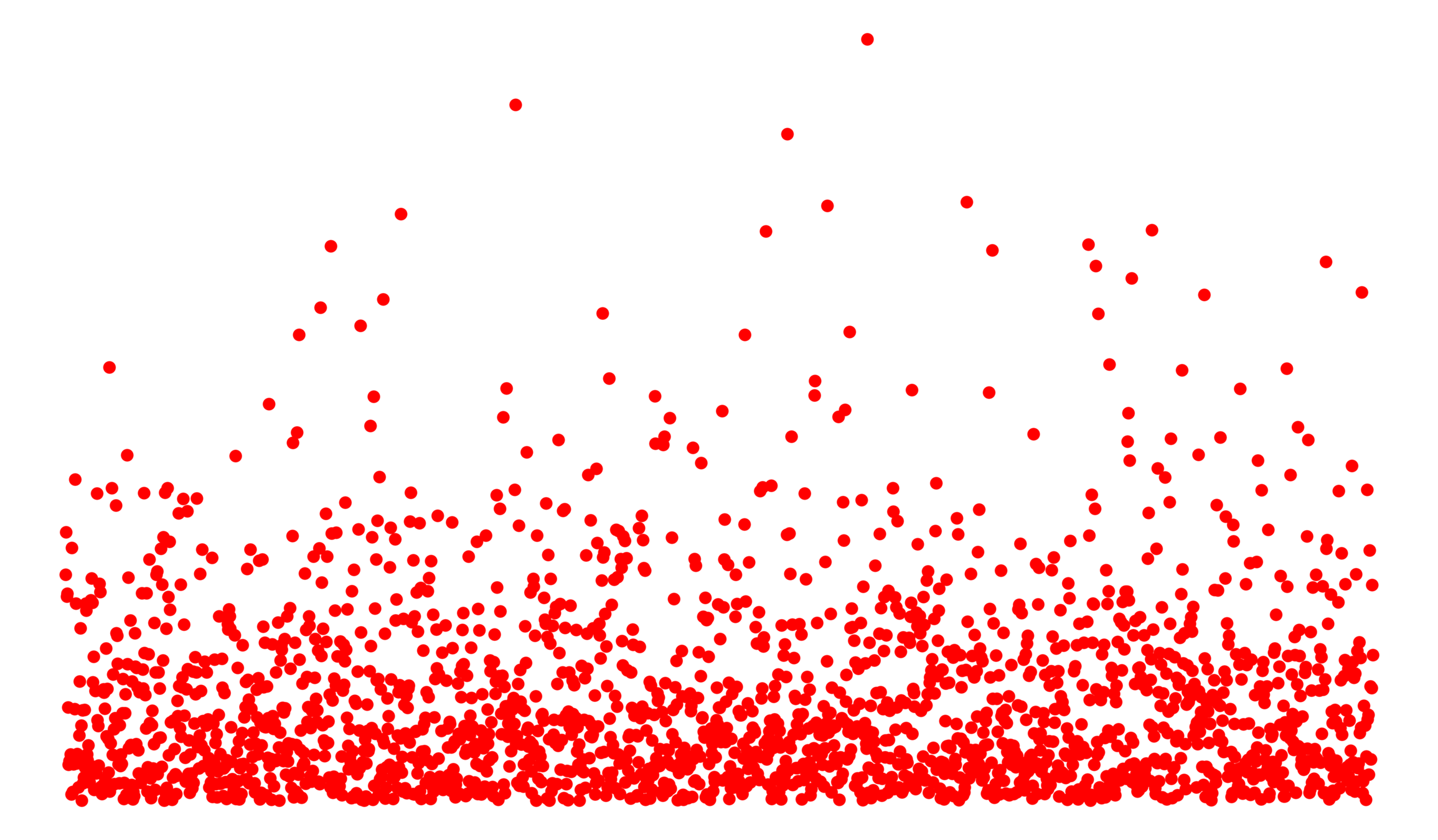
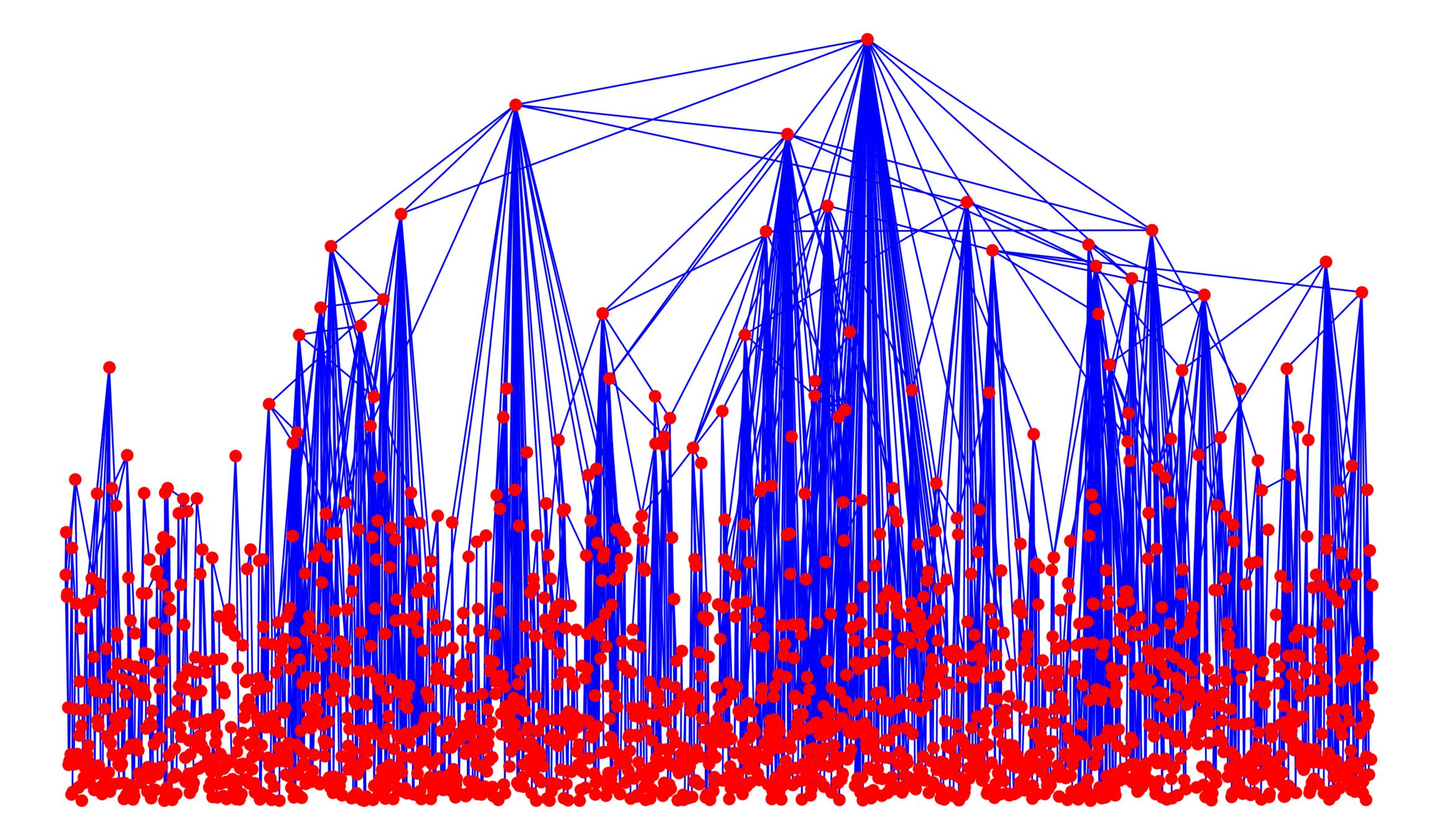
Vertex set $\mathcal{V}_\infty$

Spatial locations $x_v\in\mathbb{R}^d$: PPP(1) or $\mathbb{Z}^d$,
i.i.d. weights $w_v\ge 1$: $\mathbb{P}(w_v\ge w)=w^{-(\tau-1)}$,

Edge set $\mathcal{E}_\infty$

Symmetric kernel $\kappa(w_u, w_v)$,
Long-range parameter $\alpha>1$,
Edge-density $\beta>0$,

Connection probability

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\bigg(\beta\frac{\kappa(w_u, w_v)}{\|x_u-x_v\|^d}\bigg)^\alpha\wedge 1$$

Vertex set $\mathcal{V}_\infty$

Spatial locations $x_v\in\mathbb{R}^d$: PPP(1) or $\mathbb{Z}^d$,
i.i.d. weights $w_v\ge 1$: $\mathbb{P}(w_v\ge w)=w^{-(\tau-1)}$,

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\bigg(\beta\frac{\kappa(w_u, w_v)}{\|x_u-x_v\|^d}\bigg)^\alpha$$

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\phantom{\bigg(\beta}\frac{\kappa(w_u, w_v)}{\phantom{\|x_u-x_v\|^d}}\phantom{\bigg)^\alpha\wedge 1}$$

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\phantom{\bigg(\beta}\frac{\kappa(w_u, w_v)}{\|x_u-x_v\|^d}\phantom{\bigg)^\alpha\wedge 1}$$

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\bigg(\phantom{\beta}\frac{\kappa(w_u, w_v)}{\|x_u-x_v\|^d}\bigg)^\alpha\phantom{\wedge 1}$$

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)$$

Kernel-based spatial random graphs

Vertex set $\mathcal{V}_\infty$

Spatial locations $x_v\in\mathbb{R}^d$: PPP(1),
i.i.d. weights $w_v\ge 1$: Pareto($\tau$),

Edge set $\mathcal{E}_\infty$

Symmetric kernel $\kappa(w_1, w_2)$,
Edge-density $\beta>0$,
Long-range parameter $\alpha>1$,

Connection probability

$$\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\bigg(\beta\frac{\kappa(w_u, w_v)}{\|x_u-x_v\|^d}\bigg)^\alpha\wedge 1$$

Vertex set $\mathcal{V}_\infty$

Spatial locations $x_v\in\mathbb{R}^d$: PPP(1), or $\mathbb{Z}^d$,
i.i.d. weights $w_v\ge 1$: $\mathbb{P}(w_v\ge w)=w^{-(\tau-1)}$,

Edge set $\mathcal{E}_\infty$

Symmetric kernel $\kappa(w_1, w_2)$,
Edge-density $\beta>0$,
Long-range parameter $\alpha>1$,

The interpolating kernel

Connection probability

$${\color{grey}\mathbb{P}\big(u\leftrightarrow v\mid \mathcal{V}_\infty\big)=\bigg(\beta}\frac{\kappa(w_u, w_v)}{\color{grey}\|x_u-x_v\|^d}{\color{grey}\bigg)^\alpha\wedge 1}$$

A parameterized kernel: $\sigma\ge 0$

$$\kappa_{\sigma}(w_u, w_v):=\max\{w_u, w_v\}\min\{w_u, w_v\}^\sigma$$

$\tau: \mathbb{P}(w_v\ge w)=w^{-(\tau-1)}.$
$\sigma$: assortativity
$\sigma$: interpolation

\frac{\sigma}{\tau-1}

\frac1{\tau-1}

\sigma=\tau-1

\tau=2

\sigma=1

\sigma=\tau-2

\sigma=0

SFP/GIRG

Hyperbolic RG

Age-dep. RCM

Scale-free Gilbert

Long-range percolation

Random geom. graph

Nearest-neighbor percolation

Degree distribution

\frac{\sigma}{\tau-1}

\frac1{\tau-1}

\sigma=\tau-1

\tau=2

\sigma=1

\sigma=\tau-2

\sigma=0

\tau_\mathrm{deg}=\tau

\tau_\mathrm{deg}=\tfrac{\sigma+1}{\sigma-(\tau-2)}

\mathrm{deg}(0)=\infty

Theorem. When $\tau>2$:

Theorem. When $\tau<2$:

Infinite degrees
Bounded diameter

$$\mathbb{P}(\mathrm{deg}(0)\ge k)\sim k^{-(\tau_\mathrm{deg}-1)}.$$

[Deijfen, v.d. Hofstad, Hooghiemstra '13]

[Gracar, Grauer, Lüchtrath, Mörters '18]

[Heydenreich, Hulshof, Jorritsma '17]

[Hirsch '17]

[v.d. Hofstad, v.d. Hoorn, Maitra, '22]

[Jorritsma, K., Mitsche, '23]

[Lüchtrath '22]

Theorem. When $\tau<2$:

Theorem. When $\tau>2$:

$${\color{grey}\mathbb{P}(\mathrm{deg}(0)\ge k)\sim k^{-(\tau_\mathrm{deg}-1)}.}$$

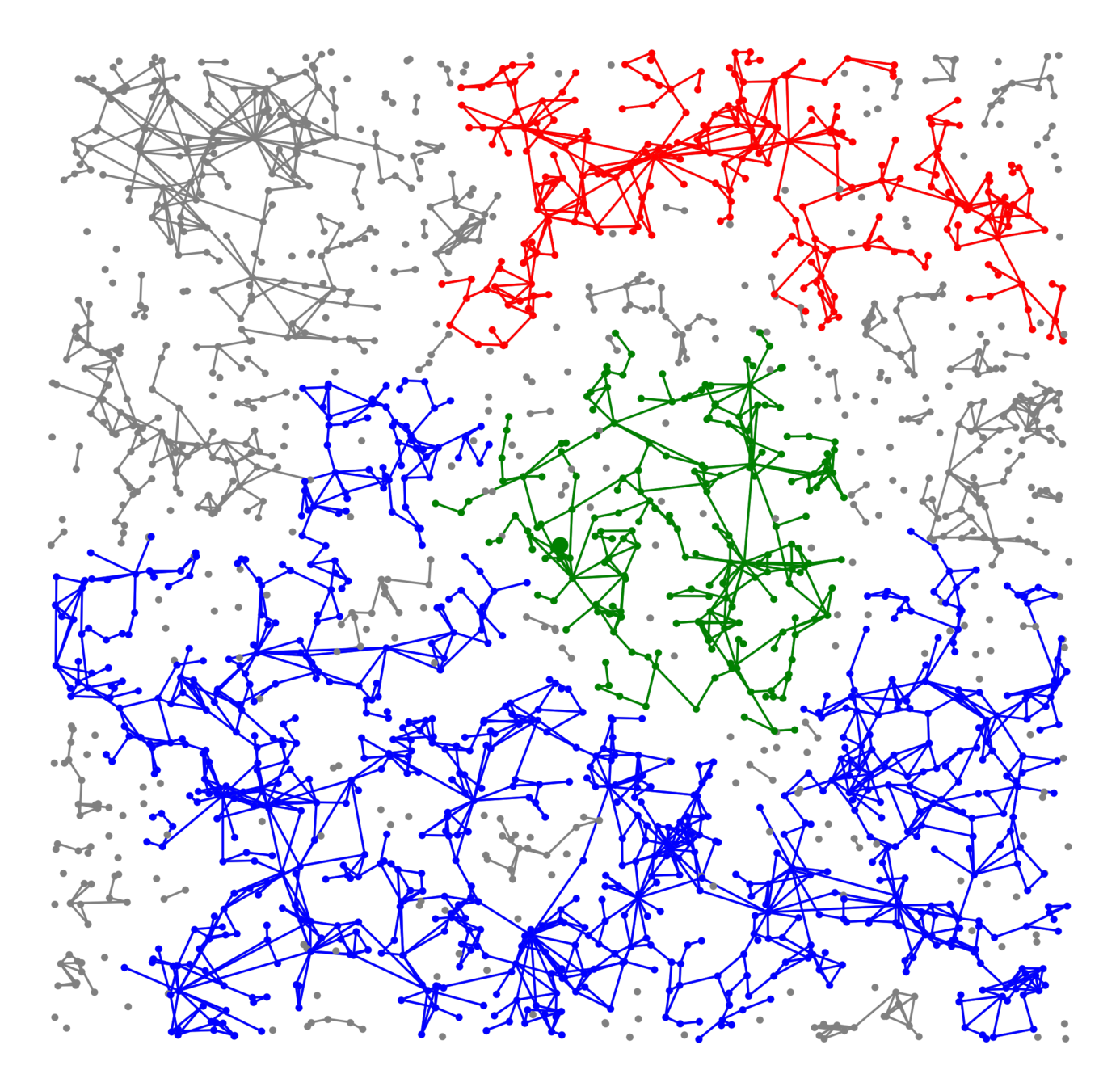
Small components in supercritical setting

Largest component $\mathcal{C}_n^{(1)}$:
Component of the origin $\mathcal{C}(0)$: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)= $$
Second-largest component: $$|\mathcal{C}_n^{(2)}|=\Theta\big(\phantom{what}\big)$$

Questions

\frac{|\mathcal{C}_n^{(1)}|}{n}\overset{\mathbb{P}}\longrightarrow

\mathbb{P}\Big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\Big)=

Some known results about $\zeta$

Speed of lower tail of large deviation:$$\mathbb{P}\big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\big)=\exp\big(-\Theta(n^\zeta)\big)$$where $|\mathcal{C}_n^{(1)}|=\Theta_\mathbb{P}(n)$.
Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)= \exp\big(-\Theta(k^{\mathbf{\zeta}})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|=\Theta\big((\log(n))^{1/\zeta}\big).$$

\zeta=\frac{d-1}d

|\mathcal{C}_n^{(2)}|=\mathcal{O}\big((\log(n))^{??}\big)

|\mathcal{C}_n^{(2)}|=\Theta\big((\log(n))^{2/(3-\tau)}\big)

[Alexander & Chayes & Chayes '90, Grimmett (& Marstrand),
Kesten & Zhang '90,

...,
Lichev, Lodewijks, Mitsche, Schapira '22,
Penrose '05]

[Sly & Crawford'12]

[Kiwi & Mitsche '17]

When $\tau>2$, there exists $\zeta\in(0,1)$ s.t.

Supercritical scaling exponent $\zeta$

Speed of lower tail of large deviation:

where $|\mathcal{C}_n^{(1)}|=\Theta_\mathbb{P}(n)$.
Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)= \exp\big(-\Theta(k^{\mathbf{\zeta}})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|=\Theta\big((\log(n))^{1/\zeta}\big).$$

When $\tau>2$, there exists $\zeta\in(0,1)$ s.t.

\frac{\sigma}{\tau-1}

\frac1{\tau-1}

\sigma=\tau-1

\tau=2

\zeta_\mathrm{nn}:=\frac{d-1}{d}

\zeta_\mathrm{hh}:=\frac{\sigma+2-\tau}{2-(\tau-1)/\alpha}

\zeta_\mathrm{lh}:=\frac{\tau-1}{\alpha}-(\tau-2)

\zeta_\mathrm{ll}:=2-\alpha

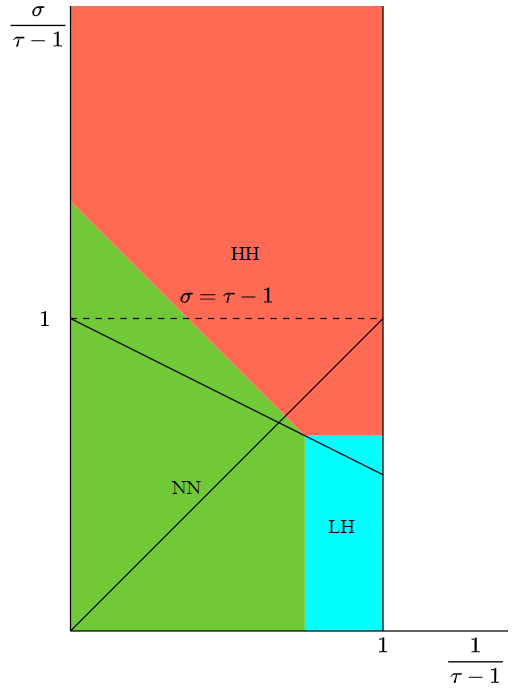
Only four possible values

\mathbb{P}\big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\big)=\exp\big(-\Theta(n^\zeta)\big)

Phase diagram of $\zeta$

\zeta=\max\{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}\}

Speed of lower tail of large deviation:

where $|\mathcal{C}_n^{(1)}|=\Theta_\mathbb{P}(n)$.
Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)= \exp\big(-\Theta(k^{\mathbf{\zeta}})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|=\Theta\big((\log(n))^{1/\zeta}\big).$$

When $\tau>2$, there exists $\zeta\in(0,1)$ s.t.

\mathbb{P}\big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\big)=\exp\big(-\Theta(n^\zeta)\big)

\zeta_\mathrm{nn}:=\frac{d-1}{d}

\zeta_\mathrm{hh}:=\frac{\sigma+2-\tau}{2-(\tau-1)/\alpha}

\zeta_\mathrm{lh}:=\frac{\tau-1}{\alpha}-(\tau-2)

\zeta_\mathrm{ll}:=2-\alpha

Only four possible values

Conjecture "almost" proven [JKM'23]

$d=1$: $\qquad\zeta_\mathrm{nn}>\max\{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}\}\quad \Longrightarrow\quad $subcritical

[Gracar, Mönch, Lüchtrath '22]

Consider supercritical interpolating model, $\sigma\le\tau-1$.

Speed of lower tail of large deviation:
Law of large numbers
Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)\sim \exp\big(-\Theta(k^{\max(Z)})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|\sim\Theta\big((\log(n))^{1/\max(Z)}\big).$$

If $\zeta_\mathrm{nn}$ is not the unique maximum of $Z:=\{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}\}$:

\mathbb{P}\big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\big)=\exp\big(-\Theta(n^\zeta)\big);

\frac{|\mathcal{C}_n^{(1)}|}{n}\overset{\mathbb{P}}\longrightarrow

\mathbb{P}\big(\mathcal{C}(0)=\infty\big);

Long-range percolation for nn-regime [JKM'23]

If $\zeta_\mathrm{nn}$ is the unique maximum of $Z$, $d>1$:

Always lower bound;
Upper bound: no weights, high edge-density $\beta\gg\beta_c$.

Gap: Monotonicity of $\zeta$

$$d=2\\\alpha=1.6$$

Proof strategy: 4 steps

\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big) \le \exp\big(-A'k^\zeta\big)

\Longrightarrow

|\mathcal{C}_n^{(2)}|\ge A(\log n)^{1/\zeta}

\Longrightarrow

(2)

(4)

\mathbb{P}\big(|\mathcal{C}_n^{(2)}|\ge k\big)\le n\exp\big(-Ak^\zeta\big)

\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big) \ge \exp\big(-A'k^\zeta\big)

(1)

(3)

Lower bounds

Upper bounds

Lower bounds: an isolated component

Aim: Find minimal $\zeta$ s.t.

\mathbb{P}\big(k \le |\mathcal{C}(0)|<\infty\big)\ge\exp\big(-Ak^\zeta\big);

Isolation events

$\mathcal{C}(0)\ge k$ in $\Theta(k)$-volume box,
$\Theta(k)$-volume box disconnected:
- Empty boundary
- High-weight vertices are dangerous
- Especially close to boundary
- No edges
Trade-off $\gamma$

k^{1/d}

2k^{1/d}

Lower bounds: an isolated component

Aim: Find minimal $\zeta$ s.t.

\mathbb{P}\big(k \le |\mathcal{C}(0)|<\infty\big)\ge\exp\big(-Ak^\zeta\big);

Isolation costs (vertices)

\mathbb{P}(\text{empty yellow})=\phantom{\exp\big(-k^{(d-1)/d}\big)}

k^{(d-1)/d}

\mathbb{E}[|\text{boundary}|]\sim k^{(d-1)/d}

\mathbb{E}[|\text{other yellow}|]\sim

k^{1-\gamma(\tau-1)}

k^{1/d}

2k^{1/d}

\mathbb{P}(\text{empty yellow})=\exp\big(-\mathbb{E}[|\text{yellow}|]\big)

\mathbb{E}[|\text{boundary}|]\sim

\Longrightarrow \quad \zeta\ge\max\big\{\tfrac{d-1}{d}, 1-\gamma(\tau-1)\big\}

\mathbb{P}(W\ge w)=w^{-(\tau-1)}

Lower bounds: an isolated component

Aim: Find minimal $\zeta$ s.t.

\mathbb{P}\big(k \le |\mathcal{C}(0)|<\infty\big)\ge\exp\big(-Ak^\zeta\big);

Isolation costs (edges)

k^{(d-1)/d}

\mathbb{P}(\text{no edges})\sim\exp\big(-\mathbb{E}[|\text{red}\leftrightarrow\text{blue}|])

\zeta\ge\max\big\{\tfrac{d-1}{d}, 1-\gamma(\tau-1)\big\}.

\begin{aligned} \mathbb{E}[|\text{red}\leftrightarrow\text{blue}|]&\sim \max_{0\le\gamma_1\le\gamma_2\le\gamma}\mathbb{E}[|k^{\gamma_1}\text{-vert.}|] \cdot\mathbb{E}[|k^{\gamma_2}\text{-vert.}|]\\ &\hspace{50pt}\cdot q\big((0, k^{\gamma_1}), (k, k^{\gamma_2})\big) \end{aligned}

\begin{aligned} \mathbb{E}[|\text{red}\leftrightarrow\text{blue}|]&\sim \end{aligned}

\begin{aligned}\Longrightarrow \quad\zeta\ge \max_{0\le\gamma_1\le\gamma_2\le\gamma} \{2-\alpha&+\gamma_1(\sigma\alpha-(\tau-1))\\&+ \gamma_2(\alpha-(\tau-1))\}\end{aligned}

\begin{aligned}\Longrightarrow \quad\zeta\ge \max_{\phantom{0\le\gamma_1\le\gamma_2\le\gamma}} \{\phantom{2-\alpha}&\phantom{+\gamma_1(\sigma\alpha-(\tau-1))}\\&\phantom{+ \gamma_2(\alpha-(\tau-1))}\}\end{aligned}

Isolation costs (edges)

\begin{aligned}\zeta\ge \max_{0\le\gamma_1\le\gamma_2\le\gamma} \{2-\alpha&+\gamma_1(\sigma\alpha-(\tau-1))\\&+ \gamma_2(\alpha-(\tau-1))\}\end{aligned}

Possible optimizers

(\gamma_1^\star, \gamma_2^\star)\in\big\{ (0, 0), \phantom{(0, \gamma), (\gamma, \gamma)} \big\}

(\gamma_1^\star, \gamma_2^\star)\in\big\{ (0, 0), (0, \gamma), \phantom{(\gamma, \gamma)} \big\}

(\gamma_1^\star, \gamma_2^\star)\in\big\{ (0, 0), (0, \gamma), (\gamma, \gamma) \big\}

(\gamma_1^\star, \gamma_2^\star)\in\big\{ \phantom{(0, 0), (0, \gamma), (\gamma, \gamma)} \big\}

\begin{aligned}\zeta\ge \max \{&\phantom{2-\alpha,}\\ &\phantom{2-\alpha + \gamma(\alpha-(\tau-1)),}\\ &\phantom{2-\alpha + \gamma((\sigma+1)-2(\tau-1))}\} \end{aligned}

\begin{aligned}\zeta\ge \max \{&2-\alpha,\\ &\phantom{2-\alpha + \gamma(\alpha-(\tau-1)),}\\ &\phantom{2-\alpha + \gamma((\sigma+1)-2(\tau-1))}\} \end{aligned}

\begin{aligned}\zeta\ge \max \{&2-\alpha,\\ &2-\alpha + \gamma(\alpha-(\tau-1)),\\ &\phantom{2-\alpha + \gamma((\sigma+1)-2(\tau-1))}\} \end{aligned}

\begin{aligned}\zeta\ge \max \{&2-\alpha,\\ &2-\alpha + \gamma(\alpha-(\tau-1)),\\ &2-\alpha + \gamma((\sigma+1)-2(\tau-1))\} \end{aligned}

Minimizing $\zeta$

\begin{aligned}\zeta=\min_\gamma \max \{&2-\alpha,\\ &2-\alpha + \gamma(\alpha-(\tau-1)),\\ &2-\alpha + \gamma((\sigma+1)-2(\tau-1)),\\ &1-\gamma(\tau-1), \\ &\tfrac{d-1}d \} \end{aligned}

Edges, vertices

Aim: Find minimal $\zeta$ s.t.

\mathbb{P}\big(k \le |\mathcal{C}(0)|<\infty\big)\ge\exp\big(-Ak^\zeta\big);

Lower bound wrap-up

Conclusion:

\begin{aligned}\zeta= \max \{&\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn} \} \end{aligned}

Aim: Find minimal $\zeta$ s.t.

\mathbb{P}\big(k \le |\mathcal{C}(0)|<\infty\big)\ge\exp\big(-Ak^\zeta\big);

\begin{aligned}\zeta= \max \{&\phantom{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}} \} \end{aligned}

\begin{aligned}\zeta= \max \{&\zeta_\mathrm{ll}, \phantom{\zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}} \} \end{aligned}

\begin{aligned}\zeta= \max \{&\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \phantom{\zeta_\mathrm{hh}, \zeta_\mathrm{nn}} \} \end{aligned}

\begin{aligned}\zeta= \max \{&\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \phantom{\zeta_\mathrm{nn}} \} \end{aligned}

Thank you!

Consider supercritical interpolating model, $\sigma\le\tau-1$.

Speed of lower tail of large deviation:
Law of large numbers
Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)\sim \exp\big(-\Theta(k^{\max(Z)})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|\sim\Theta\big((\log(n))^{1/\max(Z)}\big).$$

If $\zeta_\mathrm{nn}$ is not the unique maximum of $Z:=\{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}\}$:

\mathbb{P}\big(|\mathcal{C}_n^{(1)}|\le (1-\varepsilon)\mathbb{E}\big[|\mathcal{C}_n^{(1)}|\big]\big)=\exp\big(-\Theta(n^\zeta)\big);

\frac{|\mathcal{C}_n^{(1)}|}{n}\overset{\mathbb{P}}\longrightarrow

\mathbb{P}\big(\mathcal{C}(0)=\infty\big);

Upper bound: second-largest component

Reveal graph in stages.

Form "backbone"
with "$(k^{\gamma_1}, k^{\gamma_2})$"-edges of length $k^{1/d}$.
Merge all large components with backbone with edges of length $k^{1/d}$.

k^{1/d}

2k^{1/d}

k^{1/d}

2k^{1/d}

k^{1/d}

2k^{1/d}

Three techniques: $\zeta_\mathrm{ll}, \zeta_\mathrm{lh}$, and $\zeta_\mathrm{hh}$:

No control short edges/local geometry:

no upper bound (nn)

Prevent too large components

Consider supercritical interpolating model, $\sigma\le\tau-1$.

If $\zeta_\mathrm{nn}$ is not the unique maximum of $Z:=\{\zeta_\mathrm{ll}, \zeta_\mathrm{lh}, \zeta_\mathrm{hh}, \zeta_\mathrm{nn}\}$

Cluster-size decay: $$\mathbb{P}\big(k\le |\mathcal{C}(0)|<\infty\big)\sim \exp\big(-\Theta(k^{\max(Z)})\big);$$
Second-largest component: $$|\mathcal{C}_n^{(2)}|\sim\Theta\big((\log(n))^{1/\max(Z)}\big).$$

If $\zeta_\mathrm{nn}$ is the unique maximum of $Z$, $d>1$:

Always lower bound;
Upper bound: no weights, high edge-density $\beta\gg\beta_c$.

$\zeta_\mathrm{ll}=2-\alpha, \qquad \zeta_\mathrm{lh}=\frac{\tau-1}{\alpha}-(\tau-2), \qquad \zeta_\mathrm{hh}=\frac{3-\tau}{2-(\tau-1)/\alpha}, \qquad \zeta_\mathrm{nn}=\frac{d-1}d$

Upper bounds

Aim: Maximize $\zeta$ s.t.

\mathbb{P}\big(|\mathcal{C}_n^{(2)}|\ge k\big)\lesssim n\exp\big(-k^\zeta\big).

\mathbb{P}\big(|\mathcal{C}_n(0)\ge k, 0\notin\mathcal{C}_n^{(1)}\big)\le\mathbb{P}\big(|\mathcal{C}_n^{(2)}|\ge k\big)\lesssim\exp(-\tfrac12k^\zeta)

When $n\le\exp\big(-\tfrac12k^{\zeta}\big)$

When $k=k_n=(2\log(n))^{1/\zeta}$

\mathbb{P}\big(|\mathcal{C}_n^{(2)}|\ge (2\log(n))^{1/\zeta}\big)\lesssim n\exp\big(-2\log(n)\big).

Upper bound: second-largest component

Reveal graph in stages.

Form "backbone"
with "$(k^{\gamma_1}, k^{\gamma_2})$"-edges of length $k^{1/d}$.
Merge all large components with backbone.