Not all interventions are equal for the second peak

Joost Jorritsma,

joint with Tim Hulshof and Júlia Komjáthy

Long connections carry the curve

This talk is about

  • Epidemics like COVID-19
  • Theoretical epidemiology
  • What classical models lack
  • Effect of space/geometry/geography
  • Effect of social distancing
  • Long-term effects

This talk is not about

  • Predictions for COVID-19
  • Precise modeling of COVID-19

Outline

  • Classical models vs. Network-based
  • Intervention strategies

Compartmental model

Susceptible

Infected

Recovered

\beta \frac{I(t)S(t)}{N}
\gamma
  • ODE: Analytic expression \(I(t), S(t), R(t)\)
  • Additional states could be added (Richard)
  • "Mean-field" model: everything is averaged out:
    individuals are equal, everybody is weakly connected, individuals are "real-valued"

Two phases:

  • Subcritical: \(R_0=\beta/\gamma\le1\): epidemic vanishes
  • Supercritical: \(R_0>1\): exponential incline

Compartmental model

  • Additional states could be added (Richard)
  • "Mean-field" model: everything is averaged out:
    individuals are equal, everybody is weakly connected, individuals are "real-valued"
     
  • Only way of modeling interventions:
    Set \(\beta:=\beta(t), \gamma:=\gamma(t), N:=N(t)\).
  • Unclear how and why interventions work (assuming no data is available)
  • What is the most effective intervention?

Susceptible

Infected

Recovered

\beta \frac{I(t)S(t)}{N}
\gamma

Network-based approach

Susceptible

Infected

Recovered

Infect neighbor w.p. \(\beta\)

Heal w.p. \(\gamma\)

  • Contact network as a graph
  • Infectious nodes infect  neighbors
  • Models "coincide" for complete graph and scaling \(\beta\)
     
  • Interventions: remove edges

Network-based approach

Susceptible

Infected

Recovered

Heal w.p. \(\gamma\)

  • Contact network as a graph
  • Infectious nodes infect  neighbors
  • Models "coincide" for complete graph and scaling \(\beta\)
     
  • Interventions: remove edges
    • At random (social dist.)

Infect neighbor w.p. \(\beta\)

Network-based approach

Susceptible

Infected

Recovered

Heal w.p. \(\gamma\)

  • Contact network as a graph
  • Infectious nodes infect  neighbors
  • Models "coincide" for complete graph and scaling \(\beta\)
     
  • Interventions: remove edges
    • At random (social dist.)
    • Euclidean length (no travel)

Infect neighbor w.p. \(\beta\)

Network-based approach

Susceptible

Infected

Recovered

Heal w.p. \(\gamma\)

  • Contact network as a graph
  • Infectious nodes infect  neighbors
  • Models "coincide" for complete graph and scaling \(\beta\)
     
  • Interventions: remove edges
    • At random (social dist.)
    • Euclidean length (no travel)
    • Degree (limit # contacts)

Infect neighbor w.p. \(\beta\)

Network-based approach

No int.

Social dist.

No travel

No hubs

Classical vs. Network

Classical Network
Only two parameters Underlying network
Deterministic Stochastic
"Mean-field" Variability between nodes
No spatial component Spatial component possible
Intuitive modeling intervention
Analytic expression

Network approach

Susceptible

Infected

Recovered

Infect neighbor w.p. \(\beta\)

Heal w.p. \(\gamma\)

Susceptible w.p. \(\eta\)

  • Immunity unclear for COVID-19
  • Natural model for "second" peaks
    (also for classical model)

Network approach

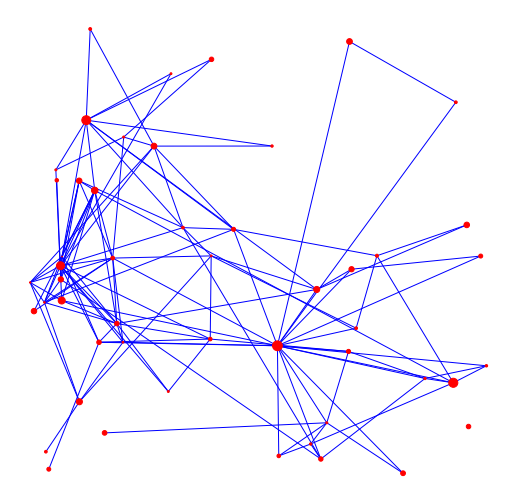
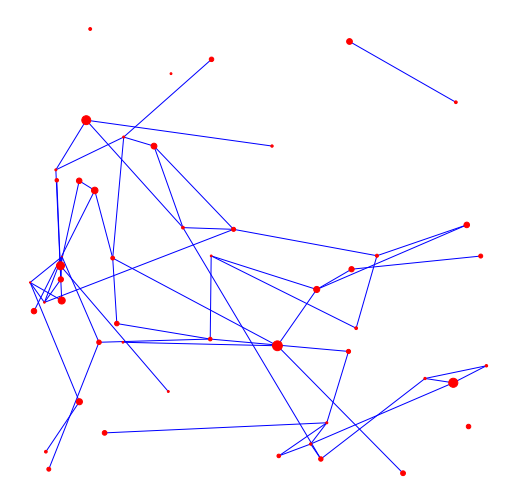
Network topology

Two graphs with mean degree 8

Network approach

Network topology

Mean degree 8, 160000 nodes

Network approach

Network topology

Two graphs with mean degree 8

Polynomial growth

"Faster" growth

Network approach

Network topology

Desired features

  • "close" nodes are
    likely to be connected
  • High variability in degrees
  • "Important" nodes are
    likely to be connected

 

Random graph model (\(\alpha>1\)):

 

p_{ij} \approx c\Big(\frac{W_iW_j}{\|x_i-x_j\|^2}\Big)^\alpha

Results

  • Network vs. Compartmental
    • New phases
    • Oscillations
  • Effect of social dist., no travel, no hubs
    • How to compare?
    • First peak
    • Later peaks
    • Probability of survival

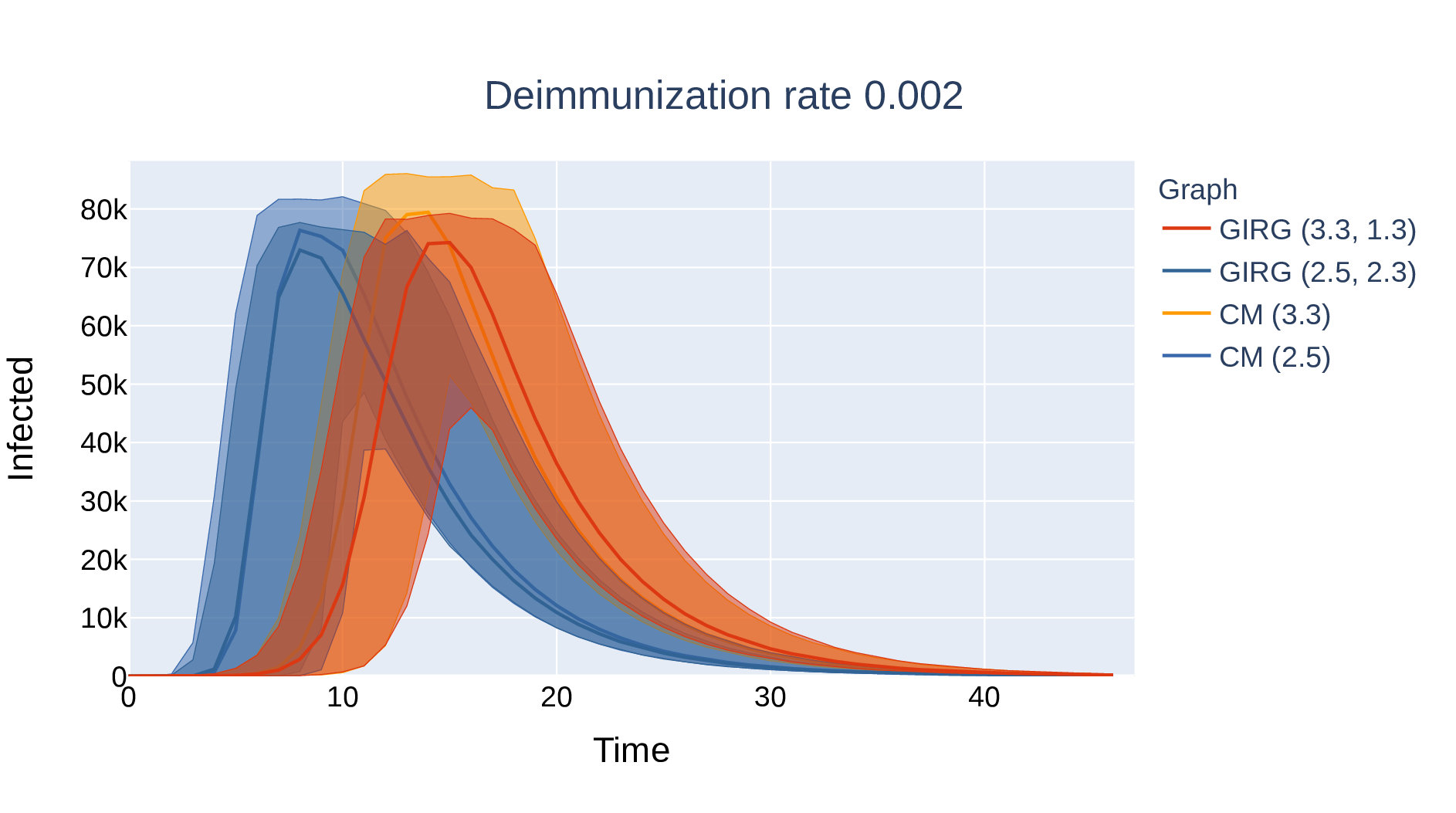
Supercritical epidemic dies out in network for \(\eta\) small

Single peak, extinction

large graphs, 100 runs

Survival

Four networks

Compartmental,

\(\eta\) small and large

Geometry introduces "immunity boundaries", herd immunity

Supercritical epidemic can survive in network for \(\eta\) large

Four networks

Compartmental,

\(\eta\) small and large

Single peak, survival

large graphs, 100 runs

Survival

Supercritical epidemic can survive in network for \(\eta\) large

Oscillations in three networks

Absence of long edges: larger amplitude

Results

  • Network vs. Compartmental
    • New phases
    • Oscillations
  • Effect of social dist., no travel, no hubs
    • How to compare?
    • First peak
    • Later peaks
    • Probability of survival

Comparing interventions

  • Remove edges permanently
    according to "rule":
    • Social dist: Random
    • No travel: Long edges
    • No hubs: Removing edges from large-degree nodes
  • Match parameters s.t. average degree is comparable
  • Start epidemic from single node
  • Resulting epidemic has at least one peak

Comparing interventions

No int. (4.8)

Social dist. (2.6)

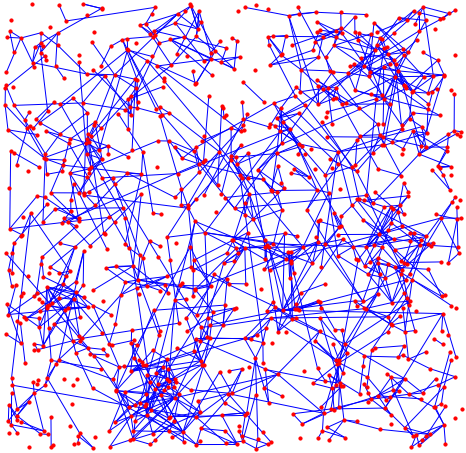
No hubs (2.6)

No travel (2.6)

No travel works best for  the first peak

Average distance from logarithmic to polynomial

No travel works best for  the first peak

Average distance from logarithmic to polynomial

No travel works well for later peaks

Higher degree-spread diminishes amplitude

No travel works well for later peaks

Higher degree-spread diminishes amplitude

Summary

Epidemics on networks with underlying geometry

  • are different from classical models.
  • allow for intuitive modeling of interventions (if no historical data is available).
     

Travel restriction works best after supercritical intervention (in a model with temporary immunity)

  • because it drastically increases average graph distance.
  • if interventions are maintained permanently and possible to implement.
  • assuming that the underlying graph is a good representation.
Made with Slides.com