Optimal transport, information, and matrix decompositions


Klas Modin

Riemannian principal bundles
Invariant Riemannian metric on \(E\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
Invariant Riemannian metric on \(E\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
left co-sets \([g] = g\cdot H \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
right co-sets \([g] = G_{b_0}\cdot g \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion


Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion



Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion





Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion





Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion





Riemannian principal bundles
left co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion






polar cone
Riemannian principal bundles
right co-sets \([g] = g\cdot G_{b_0} \)
Semi-invariant Riemannian metric on \(G\)
\(\Rightarrow\) \(\pi\) Riemannian submersion






polar cone
Examples
-
Optimal mass transport
(infinite- and finite-dimensional)
\(\Rightarrow\) polar decomposition
Vertical flows: Euler equations, free rigid body, gradient OT flow
Horizontal flows: heat flow (entropy gradient flow)
-
Information geometry
\(\Rightarrow \; QR\), Cholesky, singular value, and spectral decompositions
Vertical flows: gradient flow for \(QR\), isospectral flows
Horizontal flows: Brocket flow (entropy gradient flow)
Optimal mass transport (OMT)


Monge problem, \(L^2\) version
Optimal mass transport (OMT)


Monge problem, \(L^2\) version
Riemannian structure of OMT
Riemannian metric
Induced metric
[Benamou & Brenier (2000), Otto (2001)]
Invariance: \(\eta\in\mathrm{Diff}_{\mu_0}(M)\)



Geodesics on \(\operatorname{Diff}(\mathbb{R}^n)\)
Geodesic equation:







Easy to prove:
Polar cone \(K\) is isomorphic to strictly convex smooth functions via \(\phi \mapsto \nabla\phi\)
Hard to prove:
Polar cone \(K\) a section of principal bundle
Geodesics on \(\operatorname{Diff}(\mathbb{R}^n)\)
Geodesic equation:







Easy to prove:
Polar cone \(K\) is isomorphic to strictly convex smooth functions via \(\phi \mapsto \nabla\phi\)
Hard to prove:
Polar cone \(K\) a section of principal bundle
Brenier's decomposition of transport maps
Geodesic distance on \(\operatorname{Diff}(\mathbb{R}^n)\)
Geodesic curve:







In particular:
Monge-Ampere equation on \(\mathbb{R}^n\)
Geodesic curve:







In particular:
Monge-Ampere equation on \(\mathbb{R}^n\)
Geodesic curve:







In particular:
Linear optimal mass transport
Trivial observation: \(\varphi_0(x) = A_0 x\), \(\varphi_1(x) = A_1 x\) linear diffeomorphisms \(\Rightarrow\) geodesic consists of linear diffeomorphisms
Consequence: \(GL(n)\) is totally geodesic subgroup of \(\operatorname{Diff}(\mathbb{R}^n)\)
Corresponding subspace of densities (statistical submanifold): multivariate Gaussians with zero mean
Bundle structure




Bundle structure




Bundle structure




Monge-Ampere equation:
Bundle structure




Factorization theorem:
Bundle structure




Vertical gradient flow:

Bundle structure




Vertical gradient flow:

Bundle structure




Vertical gradient flow:

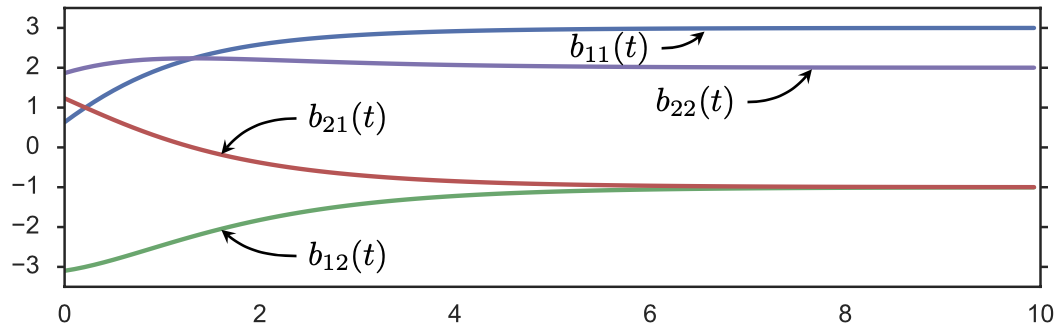
Bundle structure


Horizontal gradient (heat) flow:

Relative entropy
(Kullback-Leibler)
Bundle structure


Horizontal gradient (heat) flow:

Relative entropy
(Kullback-Leibler)
Bundle structure


Horizontal gradient (heat) flow:

Lifted gradient flow on \(K\) for
Bundle structure


Horizontal gradient (heat) flow:

Lifted gradient flow on \(K\) for
Hessian of \(F(P)\) strictly positive on \(K\) \(\Rightarrow\) unique limit!
Bundle structure


Horizontal gradient (heat) flow:

Lifted gradient flow on \(K\) for
Hessian of \(F(P)\) strictly positive on \(K\) \(\Rightarrow\) unique limit!
Bundle structure


Horizontal gradient (heat) flow:

Lifted gradient flow on \(K\) for
Hessian of \(F(P)\) strictly positive on \(K\) \(\Rightarrow\) unique limit!
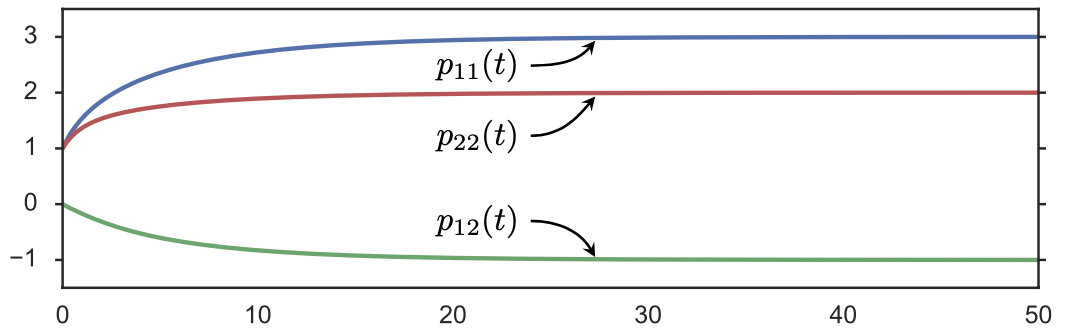
Bundle structure


Horizontal gradient (heat) flow:

Lifted gradient flow on \(K\) for
Hessian of \(F(P)\) strictly positive on \(K\) \(\Rightarrow\) unique limit!
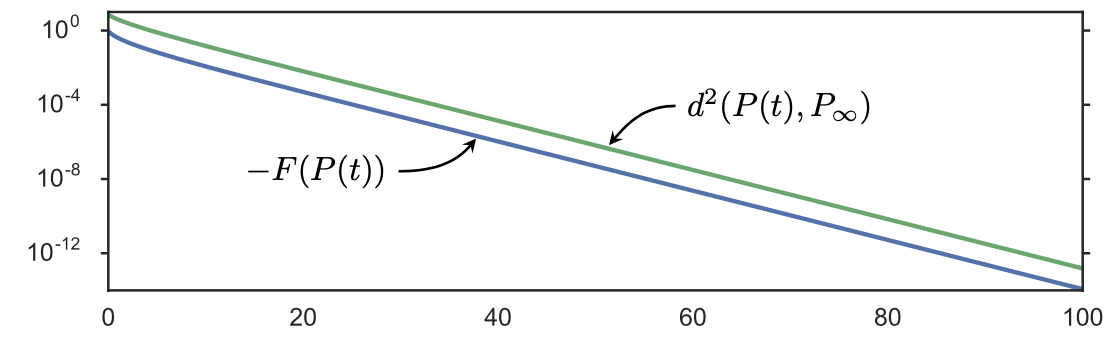
Wasserstein-Otto vs. Fisher-Rao

Wasserstein
Fisher-Rao
Dependent on Riemannian structure of \(M\)
Independent of Riemannian structure of \(M \Rightarrow \mathrm{Diff}(M)\)-invariance
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
\(K=\) subgroup of upper triangular matrices with positive entries on diagonal
Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
\(K=\) subgroup of upper triangular matrices with positive entries on diagonal

Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
\(K=\) subgroup of upper triangular matrices with positive entries on diagonal

Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
\(K=\) subgroup of upper triangular matrices with positive entries on diagonal

Bundle structure




Requirement for compatible metric on \(GL(n)\) :
- Descending, meaning
left-invariant w.r.t. \(O(n,W_0^{-1}\) ) - Right-invariant w.r.t. \(GL(n)\)
\(\mathrm{Hor}_I = \) upper triangular matrices
Lie subalgebra \(\Rightarrow\) \(\mathrm{Hor}\) is integrable
\(K=\) subgroup of upper triangular matrices with positive entries on diagonal

Entropy gradient flow (Fisher-Rao)



Relative entropy
Further reduction



Right action by \((Q,W)\mapsto Q^\top W Q \), clearly not free!
Spectral decomposition


Geodesic equation on \(D(n)\):
\(D(n)\) is totally geodesic submanifold!

Notice: \(D(n)\) intersects \(W_1\)-orbit \(n!\) times
( \(D(n)\) an \(n!\)-covering of \(\mathrm{poly}_n^+\) )
Spectral decomposition



Geodesic equation on \(D(n)\):
\(D(n)\) is totally geodesic submanifold!

Notice: \(D(n)\) intersects \(W_1\)-orbit \(n!\) times
( \(D(n)\) an \(n!\)-covering of \(\mathrm{poly}_n^+\) )
Brockett flow


\(H_N(W)\) relative entropy functional
Functional \(F(Q) = H_N(Q^\top W_1 Q)\) on \(O(n)\)
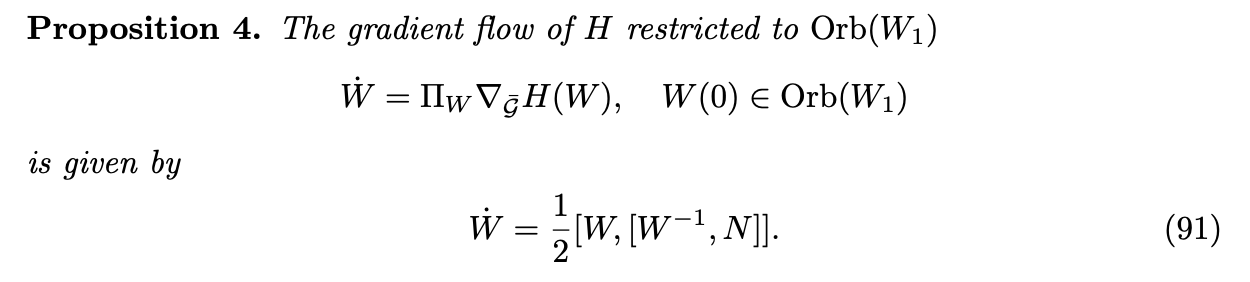


THANKS!
Reference:
- K. Modin
Geometry of Matrix Decompositions Seen Through Optimal Transport and Information Geometry, 2017
Slides available at: slides.com/kmodin