IUP Seminar on
Nonlinear Statistics
Pernille Hansen
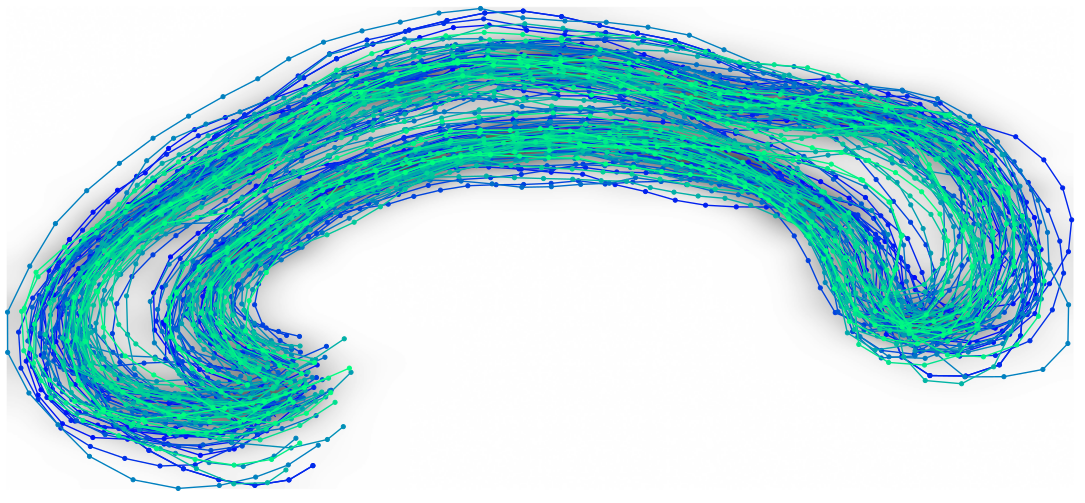

Medical Images
Alzheimer's disease (AD) Shrinkage and deformation of Corpus Callosum (CC)

Exercise: Discuss how the hypothesis can be tested from a data set of MRI's from healthy and non-healthy brains.
Hypothesis: (AD) can be detected from shape of (CC).
Mean value
Data set:
- Mean value:
Example:

Choosing a location for a Tony's Pizza!
Data set:
Mean value:

Exercise: Cooperatively come up with two properties a mean value should have and based on these, suggest a new mean value for the data set.
Mean value from distance!
Idea: Mean value should:
- be within the space
- minimize distance to the data

Def: The mean value of the points is the point that minimizes

Hypothesis: (AD) can be detected from shape of (CC).
Mean value for healthy and sick can be computed and compared!
Exercise: Come up with an example of data, that could be considered 'nonlinear'
Thank you for your attention!
ILO's
- Discuss how the Alzheimer hypothesis can be tested from a data set of MRI's from healthy and non-healthy brains.
- Cooperatively come up with two properties a mean value should have and based on these, suggest a new mean value for the data set.
- Be able to come up with an example of data, that could be considered 'nonlinear'
Mean value on circle?
Data set:
Mean value
Exercise

?
-
Compute mean value as usual and plot it in the coordinate system.
- Discuss whether the mean value is sensible as a mean value for data on the circle.
Data set:
Mean value on circle?

Limitations of
- Not an additive space
- Scaling is not allowed
Problems?
- Mean point is not located on the circle
Exercise continued :
3. Cooperatively come up with two properties a mean value should have.
4. Based on these, suggest a new point point for the data set.
(NOT A LINEAR SPACE)
Mean value from distance
Idea: Mean value should be the point that minimizes the distance to
Distance of two points :
Data set:
Mean value:
Mean value on circle
Exercise revisited

Data set:
Using only words, write down which steps are needed to find the distance mean value of a data set of points on a sphere.
