COUNTING WORDS
LEARN MATHEMATICS - CREATE NARRATIVES
Writing numbers
Rogério Lourenço
1997 - Ciências Sociais - UFRJ
2000 - Comunicação, Imagem e Informação - UFF
2001- 2008 - Empresa Municipal de Multimeios - MULTIRIO
2010 - Gramática Gerativa e Estudos de Cognição Museu Nacional -UFRJ
2010 - Rede Nacional de Pesquisa
2015 - Doutorado em Linguística - UFRJ
2017 - Laboratório de Estudo do discurso Imagem e Som - Museu Nacional - UFRJ

The image of mathematics
is a cultural practice
Mathematics

Language & Mathematics
CAGLIARI, L. C. Alfabetização e Linguística, 2000. Editora Scipione.
AN DISCOURSE ANALYSIS OF BRAZILIAN PUBLIC SCHOOL MATHEMATICS OLYMPIAD (OBMEP)
METAIMAGEM

The test
Osvaldo bought a cheese with a equilateral triangle shape.
He wants to equally divide the cheese among his four cousins and himself.
Make a draw indicating how he can make the division.
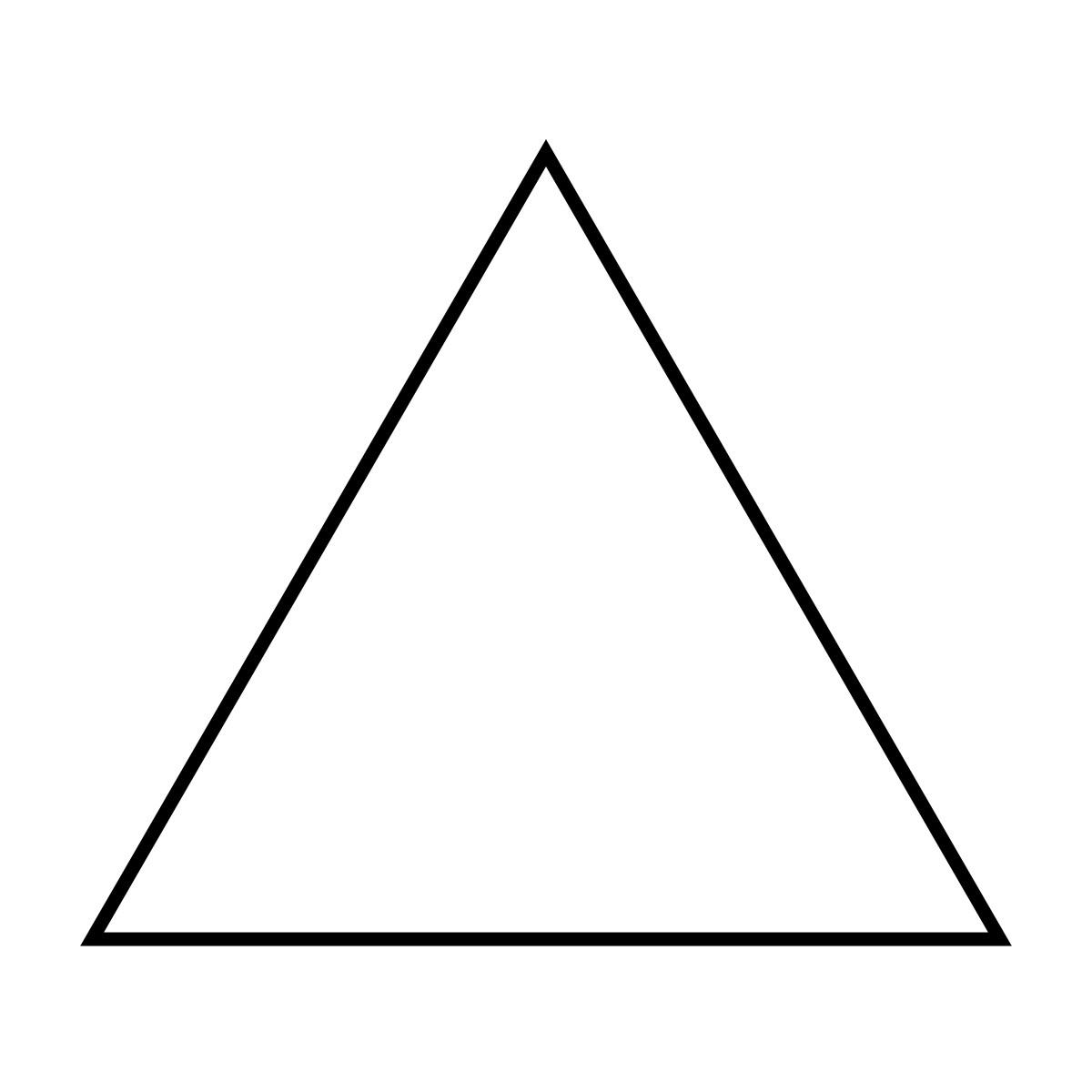
A triangular cheese
( OBEMP 2011 / questão 97)
The answer
To divide a cheese in 5 equal parts is sufficient to divide it in 5k equal parts and give k parts to each one.
One way to do this partition is shown on the figure, where the cheeses was partitioned on 25 = 5 × 5 triangles.

The project
- open platform
- to learn mathematics
- to learn how to narrate
website || app
Portuguese Mathematics
- 1 Triangle
- ÷ Division
- 4 Cousins + 1
- Cheese ( equilateral divisor )
- To divide ( verb )
- Cousins ( N dividend ) & Oswaldo
languages
grammar notation
Steps
- resources (similar initiatives)
- technologies (available)
- MVP (prototype)
resources
grammar, MathML, Parsing, Regex...
HTML, CSS3, Canvas, D3 , Javascript, SVG...
technologies
prototype
MVP:
basic operations
( + ) ( - ) ( × ) ( ÷ )
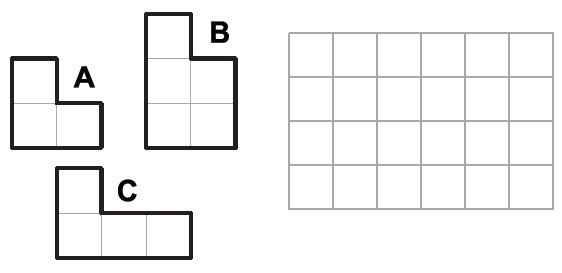
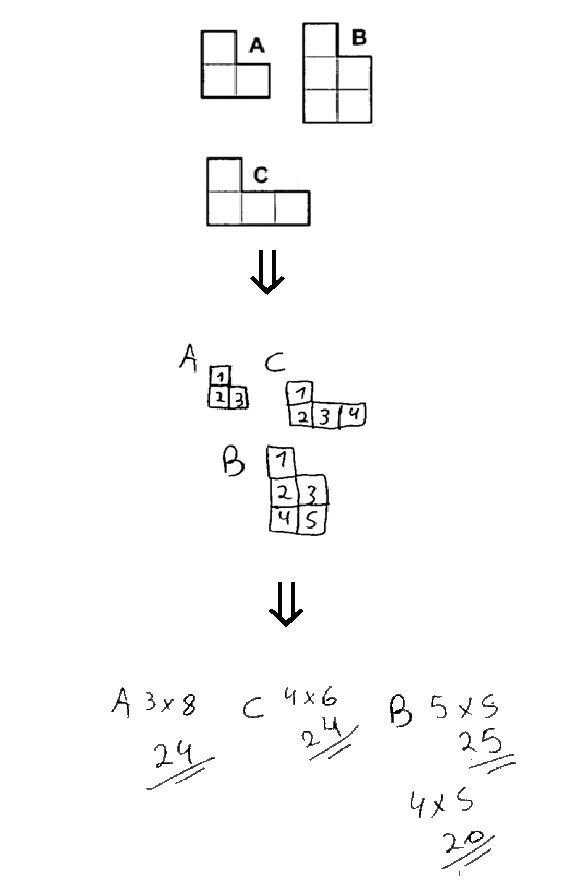
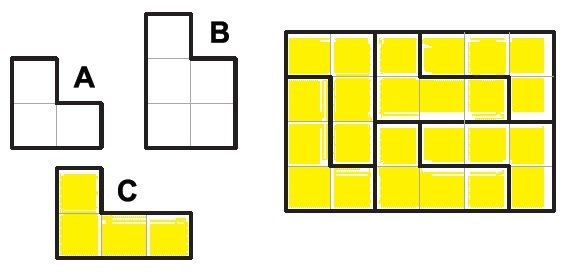
Pedro plays with boards
Pedro plays with a square checked board 4 x 6 and with pieces from types A, B andC.
Explain why it is not possible to cover the board with type B pieces.
discourse & mathematics
Pedro plays with a square checked board 4 x 6 and with pieces from types A, B andC.
The answer counts the squares on the pieces and cover the board with the c type piece as shown in the picture.
discourse & mathematics
Manipulate
the
DOM
<text>

options
circular menu

the number line
4.1.1 AN DODECAGON. (N2Q3_2010)
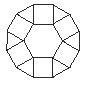
The picture shows an regular dodecagon decomposed into six equilateral triangles, six squares and one regular hexagon, all with same size sides.
a) If each triangle has the same 1 cm area, which is the hexagon´s area ?
2
“All regular hexagons can be divided into 6 equilateral triangles (6-2) - 180=720 / 6=120 ✗ 120/2=60.
So if the equilateral triangles above are equals to the sid hexagon´s side, the equilateral triangles from hexagon, have the same side of the equilateral triangles from the hexagon, have the same side from equilateral triangles from the pictures, and consequently, the same area.
Therefore the hexagon has the area: 6 x 1cm = 6 cm ”
ANSWER 1
2
2
ANSWER 2
“6 cm. As the hexagon is regular, it can be divided into equilateral triangles in the following way.
Each triangle has área 1 cm , since they are congruent to the other (all sides from all figures have the same size, and the triangles sides from the hexagon have the same side from the hexagon, having also the same side).
For 6 triangles, the área is 6 x 1 cm = 6 cm ”
2
2
2
2

ANSWER 3
“We know one regular hexagon can be divided into 6 equilateral triangules with same side of the side of the hexagon, as drawn in the figure.
As the triangles that form the hexagon have the same side from others making the dodecagon, ( equalling the hexagon) then all will have the same area: 1 cm.
As each hexagon is formed by 6 triangles, it will be igual to 6.1= 6 cm . ”
2
2
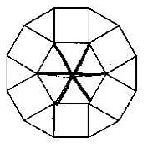
ANSWER 4
“The regular hexagon can be divided into 6 equilateal triangles. As each triangle has área of 1 cm , the hexagon has 6 cm .”
2
2

ANSWER 5
” If all have the same side measures , it is enough to divide the hexagon into six triangles.
If the area of the triangle is 1 cm , multiply by 6 (number of triangles from the hexagon) and we will have área of 6 cm pertaining to the hexágono. “
2
2

ANSWER 6
“If we connect a edge to opposite side, we will see the line will be the bisector of two angles, then each angle will have 60°,
if we do that to all edges we will have 6 congruent equilateral triangles.
As the área of the others is 1 cm , the hexago area will be 6.1= 6 cm ”
2
2
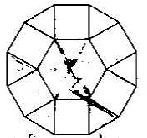

(1) To infer the square having the same side of each side of the equilateral triangle
(2) verify the picutre is composed by one of sides of the square .
The conclusion , in this case, is (6 x 1 cm = 6 cm ).
To observe the picutre... the answers use the graphical resource.
2
2


interface
The picture shows a regular dodecagon decomposed into six equilateral triangles, six squares and one regular hexagon , all with same sides mesures.
?
javascript
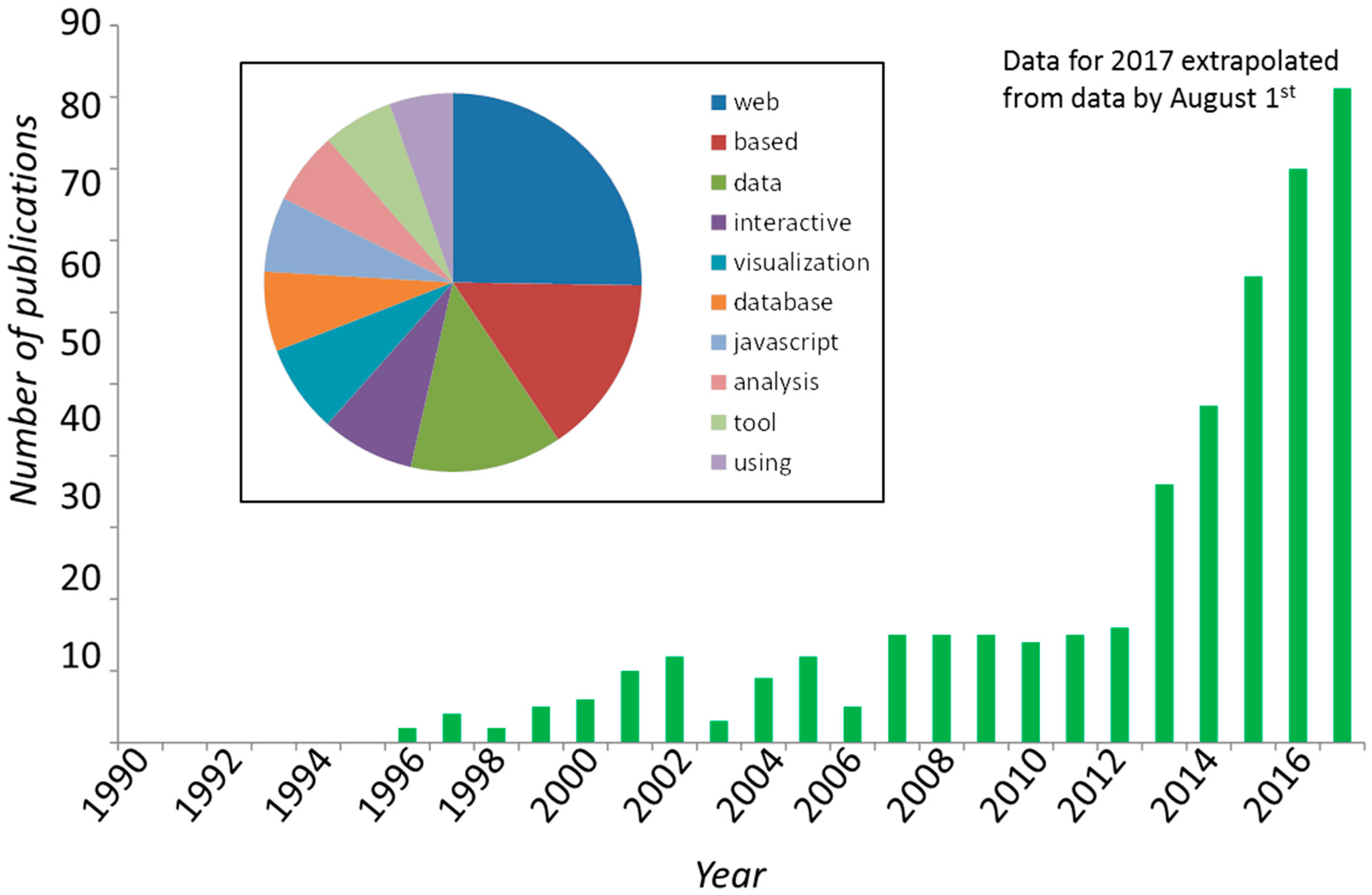
http://www.mdpi.com/2227-9709/4/3/28/htm
motivation

Thank you.

metaimagem@gmail.com