Aztec's zk\(^2\)-Rollup

Part \(1\)

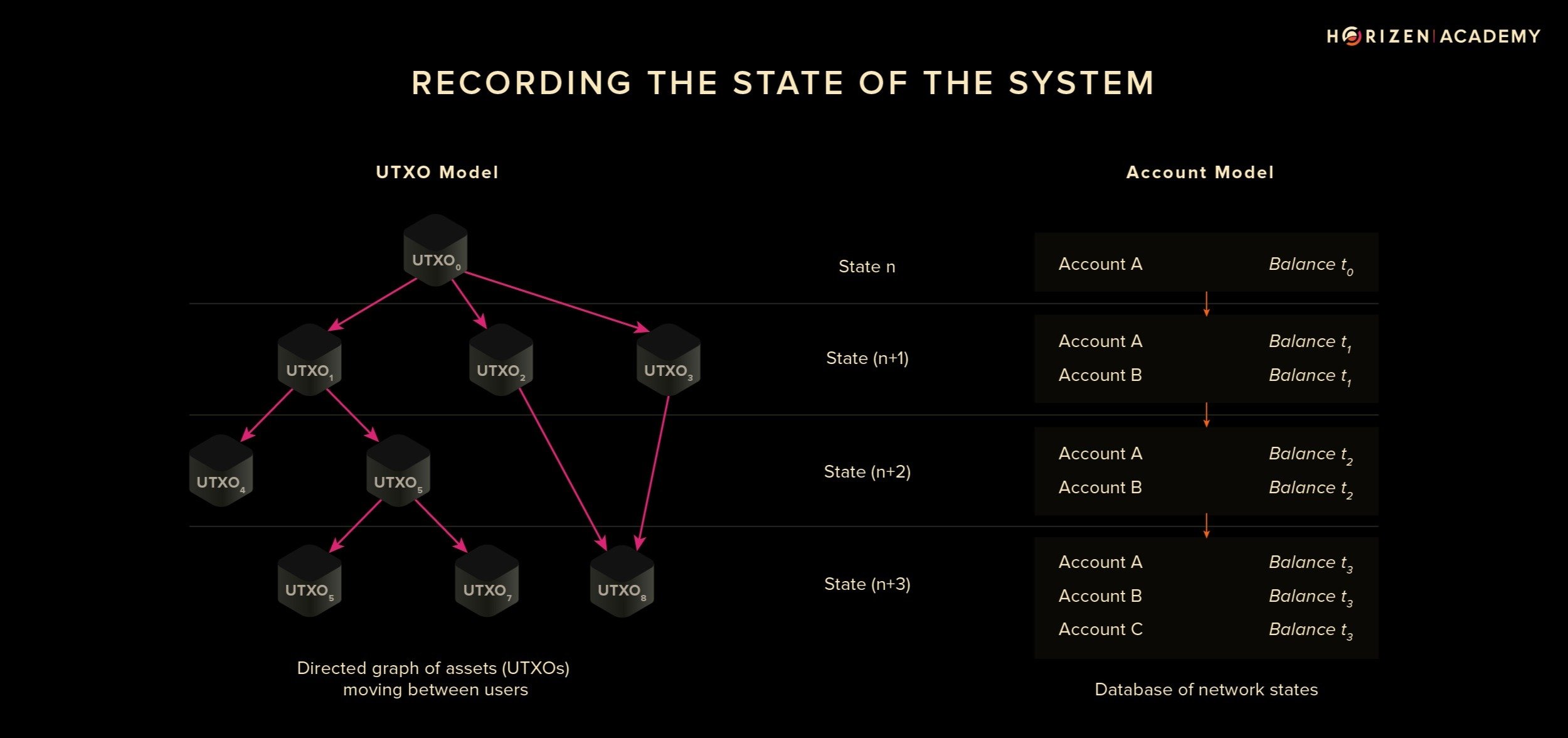
UTXO vs Account

Image: https://academy.horizen.io/technology/expert/utxo-vs-account-model/

Aztec Model

Bob

Alice

Open account

\(\texttt{bob}\)

\(\texttt{alice}\)

0.5

1.5

Shield

Rollup Contract

Account UTXO

Value UTXO

\underbrace{\hspace{2.2cm}}

Private sends

\(\text{zkETH}=8.5\)

\(\text{zkDAI}=18\)

\(\text{zkETH}=1.5\)

\(\text{zkDAI}=2\)

Withdraw

\(0\)

1.5

Aztec Notes

Account balances are calculated by adding up the available UTXOs
UTXOs are called as notes: \(\textcolor{orange}{\textsf{Account}}\) notes and \(\textcolor{violet}{\textsf{Value}}\) notes
State transition in UTXO model is tricky
A user creates an account on zk.money using an alias and a nonce \(n \in \mathbb{Z}^{32}_2\)
We compute an account identifier as:

Account information is stored in account notes

Account PK

Account id

Spending PK1

\(a_{\text{id}} \ \in \ \mathbb{Z}_2^{32}\)

\(S_1 \ \in \ \mathbb{G}_1\)

\(A \ \in \ \mathbb{G}_1\)

Account PK

Account id

Spending PK2

\(a_{\text{id}} \ \in \ \mathbb{Z}_2^{32}\)

\(S_2 \ \in \ \mathbb{G}\)

\(A \ \in \ \mathbb{G}_1\)

\(a_{\text{id}} \coloneqq \left( n \ \| \ H_{B}\left(\texttt{suyashbagad}\right)[ \ 0 : 224 \ ]\right) \in \mathbb{Z}^{256}_2\)

Spending keys are used for signing transactions

Aztec Notes

Aztec uses value notes as a basis for private transactions on Ethereum

Value

Asset id

Nonce

Owner

Secret

\(a \ \in \ \mathbb{Z}_2^{32}\)

\(A \ \in \ \mathbb{G}_1\)

\(n \ \in \ \mathbb{Z}_2^{32}\)

\(v \ \in \ \mathbb{F}_q\)

\(s \ \in \ \mathbb{F}_q\)

A value note is given as: \(\mathcal{V} = \{a, v, n, \mathcal{O}, s\}\)
The nonce here is same as the one used in an account note
A note incorporates the on-chain identity (i.e. account PK) of its owner
The secret \(s\) is the hiding factor in computing Pedersen commitment to a note:

\mathfrak{C}(\mathcal{V}) \coloneqq aG_0 + vG_1 + nG_2 + A_xG_3 + A_yG_4 + sG_5

Plonk Overview

Arithmetic Circuit

A typical computational problem: find solutions to the equation (i.e. \(\textsf{stmt}\))

\(x_1^2 \cdot x_2 + x_1 + 1 = 22\)

\times

x_1

x_2

\times

Witness: \(w \equiv (x_1=3, x_2=2)\), public inputs: \(\ell \equiv (c=1, z=22)\)
I can convince you that I know a solution \(w\) to \(\{\textsf{stmt}, \ell\}\) without revealing \(w\)
PLONK: Circuit size: \(n=4\), prover: \(\mathcal{O}(n\cdot\text{log}n)\), proof size and verifier: \(\mathcal{O}(1)\)

\iff

Plonk Arithmetisation

\textcolor{gray}{2.} a_1 \textcolor{gray}{+3.}b_1 \textcolor{gray}{-1.}c_1 \textcolor{gray}{+5} = 0

\textcolor{gray}{0.} a_3 \textcolor{gray}{+0.}b_3 \textcolor{gray}{+1.}a_3b_3 \textcolor{gray}{-1.}c_3 \textcolor{gray}{+0} = 0

(a_i,b_i) \ \textcolor{grey}{+_{\text{ecc}}} \ (c_i, d_i) = (a_{i+1},b_{i+1})

\textsf{ecc gate}:

\underbrace{\hspace{2cm}}

StandardPlonk

\underbrace{\hspace{1cm}}

TurboPlonk

\textsf{a}

\textsf{b}

\textsf{c}

\textsf{d}

a_1

b_1

c_1

d_1

a_2

b_2

c_2

d_2

a_3

b_3

c_3

d_3

\vdots

a_{i}

b_{i}

c_{i}

d_{i}

i+1

a_{i+1}

b_{i+1}

c_{i+1}

d_{i+1}

\vdots

n-1

a_{n-1}

b_{n-1}

c_{n-1}

d_{n-1}

a_{n}

b_{n}

c_{n}

d_{n}

\textsf{add gate}:

\textsf{mult gate}:

Width = \(4\)

Circuit size = \(n\)

c_1 = a_i,

d_2 = b_i,

a_{i+1} = c_{n-1},

b_{i+1} = d_{n-1},

\underbrace{\hspace{1cm}}

Copy constraints

Cell-wise permutation

Plonk Preprocessing

\textsf{a}

\textsf{b}

\textsf{c}

\textsf{d}

a_1

b_1

c_1

d_1

a_2

b_2

c_2

d_2

a_3

b_3

c_3

d_3

\vdots

a_{i}

b_{i}

c_{i}

d_{i}

i+1

a_{i+1}

b_{i+1}

c_{i+1}

d_{i+1}

\vdots

n-1

a_{n-1}

b_{n-1}

c_{n-1}

d_{n-1}

a_{n}

b_{n}

c_{n}

d_{n}

Width = \(4\)

Circuit size = \(n\)

a_1

b_1

c_1

d_1

-1

\delta_1

\delta_2

\delta_3

\delta_4

Constraint Selectors

2n+1

3n-1

3n+2

4n-1

Permutation Selectors

S_{\sigma_1}

S_{\sigma_2}

S_{\sigma_3}

S_{\sigma_4}

q_L

q_R

q_O

q_M

q_C

q_+

q_\textsf{ecc}

Plonk Preprocessing

-1

\delta_1

\delta_2

\delta_3

\delta_4

Constraint Selectors

2n+1

3n-1

3n+2

4n-1

Permutation Selectors

S_{\sigma_1}

S_{\sigma_2}

S_{\sigma_3}

S_{\sigma_4}

q_L

q_R

q_O

q_M

q_C

q_+

q_\textsf{ecc}

Compute and store coset-FFTs of selector polynomial over domain of size \(4n\)
Verification key consists of commitments to the selector polynomials
Selector polynomials are fixed for a given circuit/computation
FFT: \((7+4) \times 4n\)
MSM: \((7 + 4) \times n\)
Memory: \((7 + 4) \times 5n\)

Plonk Prover: Round 0

Convert wire polynomials to coefficient form

\left(a'_{1}, a'_{2}, a'_{3}, \dots, a'_{n}\right) \ \leftarrow \ \textcolor{lightgreen}{\textsf{iFFT}}\left(a_{1}, a_{2}, a_{3}, \dots, a_{n}\right)

\left(b'_{1}, b'_{2}, b'_{3}, \dots, b'_{n}\right) \ \leftarrow \ \textcolor{lightgreen}{\textsf{iFFT}}\left(b_{1}, b_{2}, b_{3}, \dots, b_{n}\right)

\left(c'_{1}, c'_{2}, c'_{3}, \dots, c'_{n}\right) \ \leftarrow \ \textcolor{lightgreen}{\textsf{iFFT}}\left(c_{1}, c_{2}, c_{3}, \dots, c_{n}\right)

\left(d'_{1}, d'_{2}, d'_{3}, \dots, d'_{n}\right) \ \leftarrow \ \textcolor{lightgreen}{\textsf{iFFT}}\left(d_{1}, d_{2}, d_{3}, \dots, d_{n}\right)

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{a}

\textsf{b}

\textsf{c}

\textsf{d}

a_1

b_1

c_1

d_1

a_2

b_2

c_2

d_2

a_3

b_3

c_3

d_3

\vdots

a_{i}

b_{i}

c_{i}

d_{i}

i+1

a_{i+1}

b_{i+1}

c_{i+1}

d_{i+1}

\vdots

n-1

a_{n-1}

b_{n-1}

c_{n-1}

d_{n-1}

a_{n}

b_{n}

c_{n}

d_{n}

Width = \(4\)

Circuit size = \(n\)

\omega_1

\omega_2

\omega_3

\omega_4

\dots

\omega_{n-2}

\omega_{n-1}

\omega_{n}

a_1

a_2

a_3

a_4

\dots

a_{n-2}

\dots

a_{n-1}

a_{n}

a(X)

\textsf{Round}

Plonk Prover: Round 1

Commit to wire polynomials

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{a}

\textsf{b}

\textsf{c}

\textsf{d}

a_1

b_1

c_1

d_1

a_2

b_2

c_2

d_2

a_3

b_3

c_3

d_3

\vdots

a_{i}

b_{i}

c_{i}

d_{i}

i+1

a_{i+1}

b_{i+1}

c_{i+1}

d_{i+1}

\vdots

n-1

a_{n-1}

b_{n-1}

c_{n-1}

d_{n-1}

a_{n}

b_{n}

c_{n}

d_{n}

Width = \(4\)

Circuit size = \(n\)

\textsf{Round}

\textcolor{orange}{[a]} = a'_1 \textcolor{grey}{[s_1]} + a'_2 \textcolor{grey}{[s_2]} + \dots + a'_n \textcolor{grey}{[s_n]}

\textcolor{orange}{[b]} = b'_1 \textcolor{grey}{[s_1]} + b'_2 \textcolor{grey}{[s_2]} + \dots + b'_n \textcolor{grey}{[s_n]}

\textcolor{orange}{[c]} = c'_1 \textcolor{grey}{[s_1]} + c'_2 \textcolor{grey}{[s_2]} + \dots + c'_n \textcolor{grey}{[s_n]}

\textcolor{orange}{[d]} = d'_1 \textcolor{grey}{[s_1]} + d'_2 \textcolor{grey}{[s_2]} + \dots + d'_n \textcolor{grey}{[s_n]}

Update proof: \(\pi \leftarrow (\textcolor{orange}{[a]}, \textcolor{orange}{[b]}, \textcolor{orange}{[c]}, \textcolor{orange}{[d]})\)

4 \times n

Plonk Prover: Round 2

Compute permutation polynomial \(z(X)\)

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{a}

\textsf{b}

\textsf{c}

\textsf{d}

a_1

b_1

c_1

d_1

a_2

b_2

c_2

d_2

a_3

b_3

c_3

d_3

\vdots

a_{i}

b_{i}

c_{i}

d_{i}

i+1

a_{i+1}

b_{i+1}

c_{i+1}

d_{i+1}

\vdots

n-1

a_{n-1}

b_{n-1}

c_{n-1}

d_{n-1}

a_{n}

b_{n}

c_{n}

d_{n}

Width = \(4\)

Circuit size = \(n\)

\textsf{Round}

\textcolor{orange}{[z]} = z'_1 \textcolor{grey}{[s_1]} + z'_2 \textcolor{grey}{[s_2]} + \dots + z'_n \textcolor{grey}{[s_n]}

4 \times n

\omega_1

\omega_2

\omega_3

\omega_4

\dots

\omega_{n-2}

\omega_{n-1}

\omega_{n}

z_1

z_2

z_3

z_4

\dots

z_{n-2}

\dots

z_{n-1}

z_{n}

z(X)

\small z_i = \begin{cases} 1 & i = 1 \\ \prod_{j=1}^{i} \frac{ (a_j \textcolor{grey}{+ \beta \omega^j + \gamma}) (b_j \textcolor{grey}{+ \beta k_1\omega^j + \gamma}) (c_j \textcolor{grey}{+ \beta k_2\omega^j + \gamma}) (d_j \textcolor{grey}{+ \beta k_3\omega^j + \gamma})} { (a_j \textcolor{grey}{+ \beta \sigma_1(j) + \gamma}) (b_j \textcolor{grey}{+ \beta \sigma_2(j) + \gamma}) (c_j \textcolor{grey}{+ \beta \sigma_3(j) + \gamma}) (d_j \textcolor{grey}{+ \beta \sigma_4(j) + \gamma}) } & i >1 \end{cases}

\left(z'_{1}, z'_{2}, z'_{3}, \dots, z'_{n}\right) \ \leftarrow \ \textcolor{lightgreen}{\textsf{iFFT}}\left(z_{1}, z_{2}, z_{3}, \dots, z_{n}\right)

1 \times n

Plonk Prover: Round 3

Compute quotient polynomial \(t(X)\)

\begin{aligned} t(X) =& \left(a(X)b(X)\textcolor{gray}{q_M(X)} + a(X)\textcolor{gray}{q_L(X)} + b(X)\textcolor{gray}{q_R(X)} + c(X)\textcolor{gray}{q_O(X)} + \textcolor{gray}{q_C(X)}\right){\scriptsize \frac{1}{Z_H(X)}} + \\ & (a(X) \textcolor{grey}{+ \beta X + \gamma}) (b(X) \textcolor{grey}{+ \beta k_1X + \gamma}) (c(X) \textcolor{grey}{+ \beta k_2X + \gamma}) (d(X) \textcolor{grey}{+ \beta k_3X + \gamma}) (z(X)) {\scriptsize\frac{\alpha}{Z_H(X)}} -\\ & (a(X) \textcolor{grey}{+ \beta S_{\sigma_1}(X) + \gamma}) (b(X) \textcolor{grey}{+ \beta S_{\sigma_2}(X) + \gamma}) (c(X) \textcolor{grey}{+ \beta S_{\sigma_3}(X) + \gamma}) (d(X) \textcolor{grey}{+ \beta S_{\sigma_4}(X) + \gamma}) (z(X\omega)) {\scriptsize\frac{\alpha}{Z_H(X)}} +\\ & (z(X) - 1)\textcolor{gray}{L_1(X)} {\scriptsize\frac{\alpha^2}{Z_H(X)}} \end{aligned}

\textsf{arith gate constraint}

\textsf{copy constraint}

Number of wires decide the degree of \(t(X)\): \((4n-5)\)
Lots of polynomial multiplication and division in computing \(t(X)\)
Easier to compute in evaluation form
But need the evaluation over a domain of size \(4n\) 😯
Hence need all component polynomials to be in coset-fft form

Plonk Prover: Round 3

Compute quotient polynomial \(t(X)\)

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{Round}

4 \times n

1 \times n

g\omega'_1

g\omega'_2

g\omega'_3

\dots

g\omega'_{4n}

g\omega'_{4n-1}

g\omega'_{4n-2}

\dots

\leftarrow \textcolor{olive}{\textsf{coset-FFT}}(a'_1, a'_2, \dots, a'_n)

c(X)

\vdots

d(X)

\vdots

a(X)

a''_1

a''_2

a''_3

\dots

a''_{4n-2}

a''_{4n-1}

z''_{4n}

b(X)

b''_1

b''_2

b''_3

\dots

b''_{4n-2}

\dots

b''_{4n-1}

b''_{4n}

\dots

z(X)

z''_1

z''_2

z''_3

\dots

z''_{4n-2}

\dots

z''_{4n-1}

z''_{4n}

\dots

\leftarrow \textcolor{olive}{\textsf{coset-FFT}}(b'_1, b'_2, \dots, b'_n)

\leftarrow \textcolor{olive}{\textsf{coset-FFT}}(z'_1, z'_2, \dots, z'_n)

\leftarrow \textcolor{olive}{\textsf{coset-FFT}}(c'_1, c'_2, \dots, c'_n)

\leftarrow \textcolor{olive}{\textsf{coset-FFT}}(d'_1, d'_2, \dots, d'_n)

5 \times 4n

Plonk Prover: Round 3

Compute quotient polynomial \(t(X)\)

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{Round}

4 \times n

1 \times n

5 \times 4n

\begin{aligned} t(X) =& \left(t_{1} + t_{2}\textcolor{gray}{X} + t_{3}\textcolor{gray}{X^2} + \dots + t_{n}\textcolor{gray}{X^{n-1}}\right) + \\ & \left(t_{n+1} + t_{n+2}\textcolor{gray}{X} + t_{n+3}\textcolor{gray}{X^2} + \dots + t_{2n}\textcolor{gray}{X^{n-1}}\right)\textcolor{gray}{X^n} + \\ & \left(t_{2n+1} + t_{2n+2}\textcolor{gray}{X} + t_{2n+3}\textcolor{gray}{X^2} + \dots + t_{3n}\textcolor{gray}{X^{n-1}}\right)\textcolor{gray}{X^{2n}} + \\ & \left(t_{3n+1} + t_{3n+2}\textcolor{gray}{X} + t_{3n+3}\textcolor{gray}{X^2} + \dots + t_{4n}\textcolor{gray}{X^{n-1}}\right)\textcolor{gray}{X^{3n}} \\ \end{aligned}

t_1(X)

t_2(X)

t_3(X)

t_4(X)

\textcolor{orange}{[t_1]} = t_1 \textcolor{grey}{[s_1]} + t_2 \textcolor{grey}{[s_2]} + \dots + t_n \textcolor{grey}{[s_n]}

\textcolor{orange}{[t_2]} = t_{n} \textcolor{grey}{[s_1]} + t_{n+1} \textcolor{grey}{[s_2]} + \dots + t_{2n}\textcolor{grey}{[s_n]}

\textcolor{orange}{[t_3]} = t_{2n} \textcolor{grey}{[s_1]} + t_{2n+1} \textcolor{grey}{[s_2]} + \dots + t_{3n}\textcolor{grey}{[s_n]}

\textcolor{orange}{[t_4]} = t_{3n} \textcolor{grey}{[s_1]} + t_{3n+1} \textcolor{grey}{[s_2]} + \dots + t_{4n}\textcolor{grey}{[s_n]}

4 \times n

Plonk Prover: Round 4, 5

Round 4: Compute opening evaluations

\bar{a}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((a'_1, a'_2, \dots, a'_n), \ \textcolor{gray}{\mathfrak{z}})

\bar{b}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((b'_1, b'_2, \dots, b'_n), \ \textcolor{gray}{\mathfrak{z}})

\bar{c}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((c'_1, c'_2, \dots, c'_n), \ \textcolor{gray}{\mathfrak{z}})

\bar{d}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((d'_1, d'_2, \dots, d'_n), \ \textcolor{gray}{\mathfrak{z}})

\bar{s}_{\sigma_1}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((S_{\sigma_1, 1}, \dots, S_{\sigma_1, n}), \ \textcolor{gray}{\mathfrak{z}})

\bar{s}_{\sigma_2}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((S_{\sigma_2, 1}, \dots, S_{\sigma_2, n}), \ \textcolor{gray}{\mathfrak{z}})

\bar{s}_{\sigma_3}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((S_{\sigma_3, 1}, \dots, S_{\sigma_3, n}), \ \textcolor{gray}{\mathfrak{z}})

\bar{z}_{\omega}\leftarrow \textcolor{maroon}{\textsf{evaluate}}((z'_1, z'_2, \dots, z'_n), \ \textcolor{gray}{\mathfrak{z}\omega})

Round 5: Compute linearisation polynomial \(r(X)\)

\begin{aligned} r(X) =& \left(\bar{a}\bar{b}\textcolor{gray}{q_M(X)} + \bar{a}\textcolor{gray}{q_L(X)} + \bar{b}\textcolor{gray}{q_R(X)} + \bar{c}\textcolor{gray}{q_O(X)} + \textcolor{gray}{q_C(X)}\right) + \\ & \Big[ (\bar{a} \textcolor{grey}{+ \beta \mathfrak{z} + \gamma}) (\bar{b} \textcolor{grey}{+ \beta k_1\mathfrak{z} + \gamma}) (\bar{c} \textcolor{grey}{+ \beta k_2\mathfrak{z} + \gamma}) (\bar{d} \textcolor{grey}{+ \beta k_3\mathfrak{z} + \gamma}) (z(X)) \Big] \alpha -\\ & \Big[ (\bar{a} \textcolor{grey}{+ \beta \bar{s}_{\sigma_1} + \gamma}) (\bar{b} \textcolor{grey}{+ \beta \bar{s}_{\sigma_2} + \gamma}) (\bar{c} \textcolor{grey}{+ \beta \bar{s}_{\sigma_3} + \gamma}) (\bar{d} \textcolor{grey}{+ \beta S_{\sigma_4}(X) + \gamma}) (z_\omega) \Big] \alpha +\\ & (z(X) - 1)\textcolor{gray}{L_1(\mathfrak{z})} \alpha^2 \end{aligned}

\(r(X)\) is a degree-\((n-1)\) polynomial
By combining many polynomials linearly, we avoid opening them individually

Plonk Prover: Round 5

Round 5 (contd.): Compute KZG opening proofs

W_\mathfrak{z}(X) \leftarrow \textcolor{green}{\textsf{KZG.open}}\left( \big\{r(X), a(X), b(X), c(X), d(X), S_{\sigma_1}(X), S_{\sigma_2}(X), S_{\sigma_3}(X)\big\}, \ \textcolor{grey}{\mathfrak{z}} \right)

W_{\mathfrak{z}\omega}(X) \leftarrow \textcolor{green}{\textsf{KZG.open}}\left( \big\{z(X)\big\}, \ \textcolor{grey}{\mathfrak{z}\omega} \right)

\textcolor{orange}{[W_{\mathfrak{z}}]} = w_1 \textcolor{grey}{[s_1]} + w_2 \textcolor{grey}{[s_2]} + \dots + w_n \textcolor{grey}{[s_n]}

\textcolor{orange}{[W_{\mathfrak{z}\omega}]} = w'_1 \textcolor{grey}{[s_1]} + w'_2 \textcolor{grey}{[s_2]} + \dots + w'_n \textcolor{grey}{[s_n]}

\textcolor{lightgreen}{\textsf{FFT}}

4 \times n

\textcolor{lightpink}{\textsf{MSM}}

\textsf{Round}

4 \times n

1 \times n

5 \times 4n

4 \times n

2 \times n

\(\pi = \bigg\{\underbrace{[a]_1, [b]_1, [c]_1, [d]_1, [z]_1, [t_1]_1, [t_2]_1, [t_3]_1, [t_4]_1, [W_{\mathfrak{z}}]_1, [W_{\mathfrak{z\omega}}]_1}_{\mathbb{G}_1^{2w + 3}}, \ \underbrace{\bar{a}, \bar{b}, \bar{c}, \bar{d}, \bar{z}_{\omega}, \bar{s}_{\sigma_1}, \bar{s}_{\sigma_2}, \bar{s}_{\sigma_3}}_{\mathbb{F}_p^{2w}} \bigg\}\)

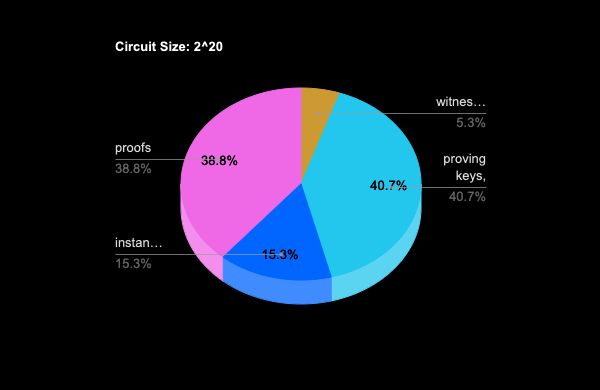
Plonk Prover: Benchmarking

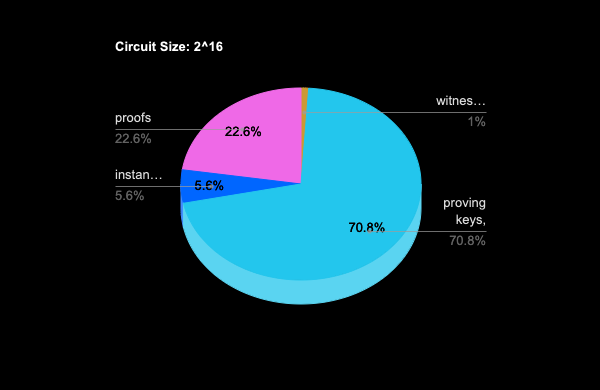
Circuit size: \(2^{16}\)

Circuit size: \(2^{20}\)

Recursion Basics

Recursive Proof Verification

A Plonk proof \(\pi\) is verified by checking equality of polynomial evaluations

\(W_{\mathfrak{z}}(x) \cdot (x - \mathfrak{z}) = F_1(x) - F_1(\mathfrak{z})\)

\(W_{\mathfrak{z\omega}}(x) \cdot (x - \mathfrak{z}\omega) = F_2(x) - F_2(\mathfrak{z}\omega)\)

\(W_{\mathfrak{z}}(x) \cdot (x - \mathfrak{z}) + u \cdot (W_{\mathfrak{z\omega}}(x) \cdot (x - \mathfrak{z}\omega))= F_1(x) - F_1(\mathfrak{z}) + u \cdot (F_2(x) - F_2(\mathfrak{z}\omega))\)

Recursive Proof Verification

A Plonk proof \(\pi\) is verified by checking equality of polynomial evaluations

\(W_{\mathfrak{z}}(x) \cdot (x - \mathfrak{z}) = F_1(x) - F_1(\mathfrak{z})\)

\(W_{\mathfrak{z\omega}}(x) \cdot (x - \mathfrak{z}\omega) = F_2(x) - F_2(\mathfrak{z}\omega)\)

\(W_{\mathfrak{z}}(x) \cdot (x - \mathfrak{z}) + u \cdot (W_{\mathfrak{z\omega}}(x) \cdot (x - \mathfrak{z}\omega))= F_1(x) - F_1(\mathfrak{z}) + u \cdot (F_2(x) - F_2(\mathfrak{z}\omega))\)

\(\underbrace{\left(W_{\mathfrak{z}}(x) + uW_{\mathfrak{z\omega}}(x)\right)}_{P_0} \cdot x = \underbrace{\left(\mathfrak{z}W_{\mathfrak{z}}(x) + u\mathfrak{z}\omega W_{\mathfrak{z\omega}}(x)) + F(x) - E\right)}_{P_1}\)

\(P_0 \cdot x \stackrel{?}{=} P_1\)

Recursive Proof Verification

Suppose we have \(n\) Plonk proofs \((\pi_1, \pi_2, \dots, \pi_m)\) with verification equations:

\(P_0^{(i)} \cdot x \stackrel{?}{=} P_1^{(i)} \quad \forall i \in [m]\)

\(\left(P_0^{(1)} + qP_0^{(2)} + \dots + q^{m-1}P_0^{(m)}\right) \cdot x \stackrel{?}{=} \left(P_1^{(1)} + qP_1^{(2)} \dots + q^{m-1}P_1^{(m)}\right)\)

A single pairing is \(\approx 300\) times costlier than a scalar multiplication
Using recursive verification, we can verify any number of Plonk proofs using a single pairing
Too good to be true? The circuit size presents a practical constraint on the number of proofs to be rolled up
Failure of the recursive check implies at least one of the \(n\) proofs is wrong

Recursive Verification Circuit

To recursively verify proofs, we only need to compute:

P_{0, \textsf{next}} = \left(P_0^{(1)} + qP_0^{(2)} + \dots + q^{m-1}P_0^{(m)}\right) \ + \left(W_{\mathfrak{z}}(x) + uW_{\mathfrak{z\omega}}(x)\right)

P_{1, \textsf{next}} = \left(P_1^{(1)} + qP_1^{(2)} + \dots + q^{m-1}P_1^{(m)}\right) \ + \left(\mathfrak{z}W_{\mathfrak{z}}(x) + u\mathfrak{z}\omega W_{\mathfrak{z\omega}}(x)) + F(x) - E\right)

Past \(n\) proofs

Current proof

So this is a scalar multiplication of size \(\approx (m + 10)\)
This involves non-native computation: i.e. computation in \(\mathbb{F}_q\) over a circuit modulo \(\mathbb{F}_p\) where \(q \gg p\).
Performing non-native arithmetic over arithmetic circuits is very costly
Therefore recursive verification circuits tend to be huge

Aztec Circuit Landscape

Transaction Proofs

Account Proofs

\pi^{\textsf{js}}_1 \qquad \quad \pi^{\textsf{js}}_2 \qquad \quad \pi^{\textsf{js}}_3 \qquad \quad \pi^{\textsf{js}}_4

\pi^{\textsf{acc}}_1 \qquad \ \ \pi^{\textsf{acc}}_2 \qquad \ \ \pi^{\textsf{acc}}_3 \qquad \ \ \pi^{\textsf{acc}}_4

\pi^{\textsf{tx}}_1

\pi^{\textsf{tx}}_2

\pi^{\textsf{root}}_1

\pi^{\textsf{std}}_1

Root Rollup Proof:

Tx Rollup Proofs:

Root Verifier Proof:

2^{16}

2^{15}

2^{21}

2^{22}

2^{23}

\textsf{standard plonk}

\textsf{turbo plonk}