Bijective Rotations on Hexagonal Lattice
by K. Pluta, T. Roussillon, D. Cœurjolly,
P. Romon, Y. Kenmochi, V. Ostromoukhov
21/06/2018, JIG 2018, Lyon
Motivations
Digitized rotations on the square grid are burdened with an incompatibility between rotations and the geometry of the grid.
Agenda
-
Introduction to the Bees' Point of View
-
Quick Introduction to Rigid Motions
- Bijective Digitized Rotations
- Contributions
- Conclusions & Perspectives
The beehive figure's source and author unknown (if you recognize it, please let me know). The image of the bee comes from http://karenswhimsy.com/public-domain-images (public domain)
Contributions in Short
- Characterization of bijective digitized rotations on the regular hexagonal lattice
- Comparison of frequencies of such rotations on the square and the regular hexagonal lattices
Pure, extracted honey
Related Studies
- Pluta, K., Romon, P., Kenmochi, Y., Passat, N.: Bijective Digitized Rigid Motions on Subsets of the Plane. Journal of Mathematical Imaging and Vision (2017)
- Pluta, K., Romon, P., Kenmochi, Y., Passat, N.: Bijectivity Certification of 3D Digitized Rotations. CTIC (2016)
- Roussillon, T., Cœurjolly, D.: Characterization of Bijective Discretized Rotations by Gaussian Integers. Research report, LIRIS UMR CNRS 5205 (2016). URL: https://hal.archives-ouvertes.fr/hal-01259826
Introduction to the Bees' Point of View
The figure comes from http://thegraphicsfairy.com/vintage-clip-art-bees-with-honeycomb
The Lattices
\mathbb{Z}[\omega] = \mathbb{Z} \oplus \mathbb{Z}\omega, \omega = -\frac{1}{2} + \frac{\sqrt{3}}{2} i
\mathbb{Z}[i] = \mathbb{Z} \oplus \mathbb{Z}i
Eisenstein:
and Gaussian:
integers
Properties
| Property | Gaussian integers | Eisenstein integers |
|---|---|---|
| conjugate | ||
| squared modulus | ||
| units |
\bar{\mathbf{x}} = a - b i
\bar{\mathbf{x}} = (a - b) - b \omega
| \mathbf{x} | = \mathbf{x} \cdot \bar{\mathbf{x}} = a^2 + b^2
| \mathbf{x} | = \mathbf{x} \cdot \bar{\mathbf{x}} = a^2 - a b + b^2
\Upsilon = \{ \pm 1, \pm i \}
\Upsilon = \{ \pm 1, \pm \omega, \pm \bar{\omega} \}
| divisibility |
\mathbf{y} | \mathbf{x} \text{ if } \exists \mathbf{z} \in \mathbb{Z}[\varkappa] \text{ such that } \mathbf{x} = \mathbf{y}
\cdot \mathbf{z} \text{ and } \mathbf{y} \in \mathbb{Z}[\varkappa]
| greatest common divisor |
|
\gcd(\mathbf{x}, \mathbf{y}) = \mathbf{z} \in \mathbb{Z}[\varkappa]\text{ is defined as a largest }
\mathbf{z} \in \mathbb{Z}[\varkappa]\text{ (up to multiplications by units) which }
\text{divides both } \mathbf{x}\text{ and }\mathbf{y}
\text{Giving } a, b \in \mathbb{Z}\text{ and }\mathbf{x},\mathbf{y}, \in \mathbb{Z}[\varkappa], \varkappa \in \{i, \omega\}
Digitization Model
A hexagonal cell of
\kappa
denoted by
\mathcal{C}_{\zeta}(\kappa),
\kappa\in \mathbb{Z}[\omega], \zeta \in \mathbb{C}.
\kappa
Digitization Model
The digitization operator is defined as
\mathcal{D}: \mathbb{C} \rightarrow \mathbb{Z}[\omega]
such that
\forall \mathbf{x} \in \mathbb{C}, \exists! \mathcal{D}(\mathbf{x}) \in \mathbb{Z}[\omega]
\mathbf{x} \in \mathcal{C}_1(\mathcal{D}(\mathbf{x})).
and
\mathcal{D}(\mathbf{x})
Quick Lesson on Rigid Motions
Or how to become a beekeeper. Part I - Equipment
The figure comes from Wikimedia. Original source The New Student's Reference Work (public domain)
\left|
\begin{array}{lllll}
\mathcal{U} & : & \mathbb{C} & \to & \mathbb{C} \\
& & \mathbf{x} & \mapsto & \mathbf{a} \cdot \mathbf{x}
\end{array}
\right.
Rotations on
\mathbb{C}
Properties
- Do preserve distances
- Bijective
\mathbf{a}
– a unit modulus complex number
\theta = \arg(\mathbf{a})
– a rotation angle
U = \mathcal{D}\ \circ \mathcal{U}_{|\mathbb{Z}[\omega]}
\mathbb{Z}[\omega]
Properties
- Non-injective
- Non-surjective
- Do not preserve distances
\mathcal{U}(\mathbb{Z}[\omega])
Rigid Motions on
A digitized rotation is bijective if and only if
\forall \mathbf{p} \in \mathbb{Z}[\omega] \; \exists ! \mathbf{q} \in \mathbb{Z}[\omega]
such that
\mathcal{U}(\mathbf{q}) \in \mathcal{C}_1(\mathbf{p}).
\mathcal{U}
- a continuous rotation
\mathcal{C}_{\mathbf{a}}(\mathbf{p})
- a digitization cell centered at
\mathbf{p}
\mathbb{Z}[\omega]
- the hexagonal lattice
Conditions for Bijectivity
and multiplied by
\mathbf{a}
Bijective Digitized Rotations on Hexagonal Lattice
The figure comes from Wikimedia. The original source The honey bee: a manual of instruction in apiculture (public domain)
The figure of bumble bee comes from http://www.ase.org.uk (public domain), The bee figure by Pearson Scott Foresman, Wikimedia.
Set of Remainders
\forall \mathbf{p} \in \mathbb{Z}[\omega]\ \exists! \mathbf{q} \in \mathbb{Z}[\omega], S_{\mathbf{a}}(\mathbf{p},\mathbf{q}) \in \mathcal{C}_1(0)
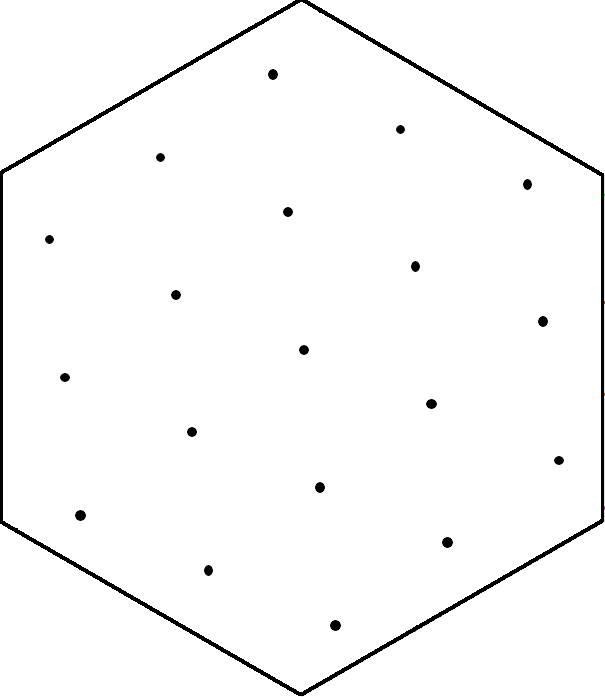
A 2D digitized rotation is then bijective when
Double Surjectivity
Double Surjectivity
Double Surjectivity
The double surjectivity condition is then
provided that
S_{\mathbf{a}}(\mathbb{Z}[\omega], \mathbb{Z}[\omega]) \cap \mathcal{C}_1(0) =
S_{\mathbf{a}}(\mathbb{Z}[\omega], \mathbb{Z}[\omega]) \cap \mathcal{C}_{\frac{\mathbf{a}}{|\mathbf{a}|}}(0).
Primitive Eisenstein Integers
s, t \in \mathbb{Z}
0 < s < t
and
t - s
not being divisible by 3.
a = s^2 + 2 s t, b = t^2 + 2 s t
and
c = s^2 + t^2 + s t,
such that
Set of Remainders
The double surjectivity condition is then
provided that
\check{S}_{\mathbf{a}}(\mathbb{Z}[\omega], \mathbb{Z}[\omega]) \cap \mathcal{C}_{\mathbf{a}}(0) =
\check{S}_{\mathbf{a}}(\mathbb{Z}[\omega], \mathbb{Z}[\omega]) \cap \mathcal{C}_{|\mathbf{a}|}(0).
Primitive Eisenstein Integers
A bunch of facts
Set of Remainders
The double surjectivity condition is then
provided that
Proving Bijectivity
Proving Bijectivity
For which s and t,
or
are in the green but not in the
black hexagonal cell?
Proving Bijectivity
From the equation of the line passing through the vertices of the green line-segment we obtain:
Proving Bijectivity
Then, we substitute t with s + e to arrive at
which are violated when s = 1 or when s > 1 and e = 1.
From the equation of the line passing through the vertices of the green line-segment we obtain:
Proving Bijectivity
At the end we have to check if
\bar{\gamma}+ \omega
or
\bar{\gamma}-1
are members
of the lattice that spans the values of
S'_{\mathbf{a}}.
Proving Bijectivity
Step 2: Check if for s = 1 or s > 1 and e = 1, the uncommon space of the hexagonal cells does not contain Eisenstein integers.
s = 1
s > 1, t = s + 1
Proving Bijectivity
The Square Grid
The Hexagonal Grid
Conclusion & Perspectives
Or extracting the pure, organic honey
The figure comes from Wikimedia. The original comes from A practical treatise on the hive and honey-bee (public domain)
- Characterization of bijective digitized rotations on the regular hexagonal lattice
- Comparison of frequencies of such rotations on the square and the regular hexagonal lattices
Conclusion
Perspectives
Investigate bijectivity of digitized rigid motions on finite subsets of Eisenstein integers.
Thank you for your attention and see you again!
Bijective Rotations on Hexagonal Lattice
By Kacper Pluta
Bijective Rotations on Hexagonal Lattice
Presentation created for JIG 18 in Lyon.
