DESI DR2: Cosmological Constraints from the Baryon Acoustic Oscillations
Arnaud de Mattia
on behalf of the DESI collaboration
Leiden, March 26th



Thanks to our sponsors and
72 Participating Institutions!


DESI 3D Map

Physics program
- Galaxy and quasar clustering
- Lyman-alpha forest
- Clusters and cross-correlations
- Galaxy and quasar physics
- Milky Way Survey
- Transients and low-z

DESI 3D Map

Physics program
- Galaxy and quasar clustering
- Lyman-alpha forest
- Clusters and cross-correlations
- Galaxy and quasar physics
- Milky Way Survey
- Transients and low-z

DESI Y5 galaxy samples
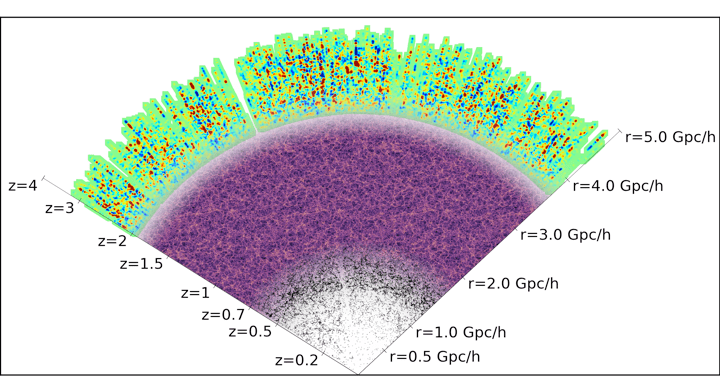
Bright Galaxies: 14M (SDSS: 600k)
0 < z < 0.4
LRG: 8M (SDSS: 1M)
0.4 < z < 0.8
ELG: 16M (SDSS: 200k)
0.6 < z < 1.6
QSO: 3M (SDSS: 500k)
Lya \(1.8 < z\)
Tracers \(0.8 < z < 2.1\)
Y5 \(\sim 40\)M galaxy redshifts!
\(z = 0.4\)
\(z = 0.8\)
\(z = 0\)
\(z = 1.6\)
\(z = 2.0\)
\(z = 3.0\)

Mayall Telescope
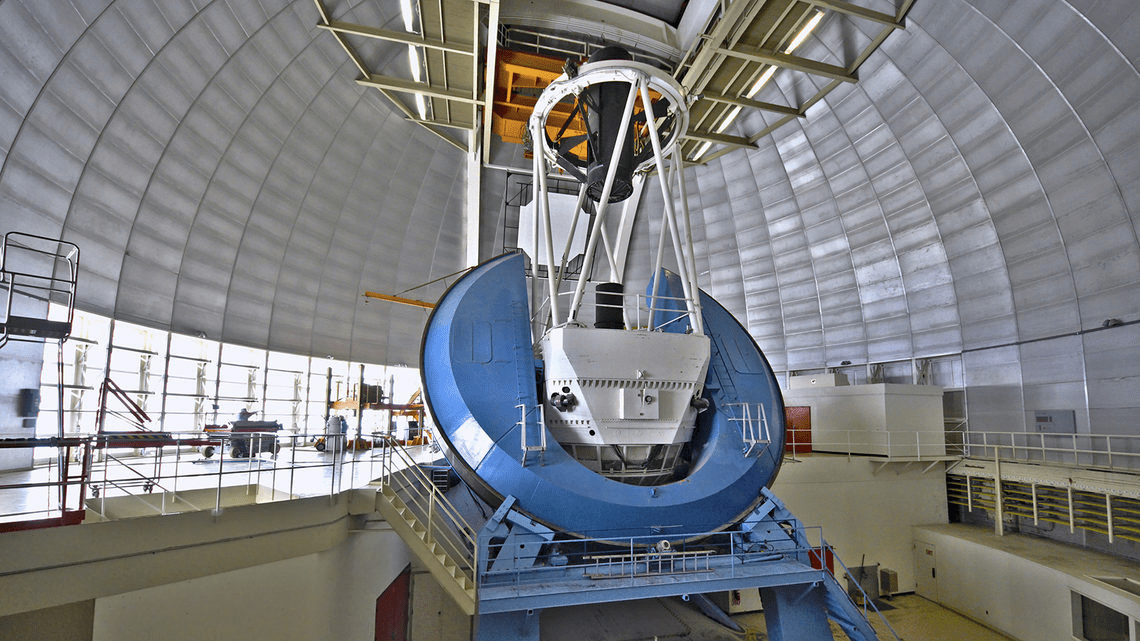

focal plane 5000 fibers
fiber view camera
wide-field corrector FoV \(\sim 8~\mathrm{deg}^{2}\)
ten 3-channel spectrographs
49 m, 10-cable fiber run
Kitt Peak, AZ

Focal plane: 5000 robotic positioners



86 cm
0.1 mm
robots w/ 2 rotation axes
position spectroscopic fibers

Spectroscopic pipeline

wavelength
fiber number

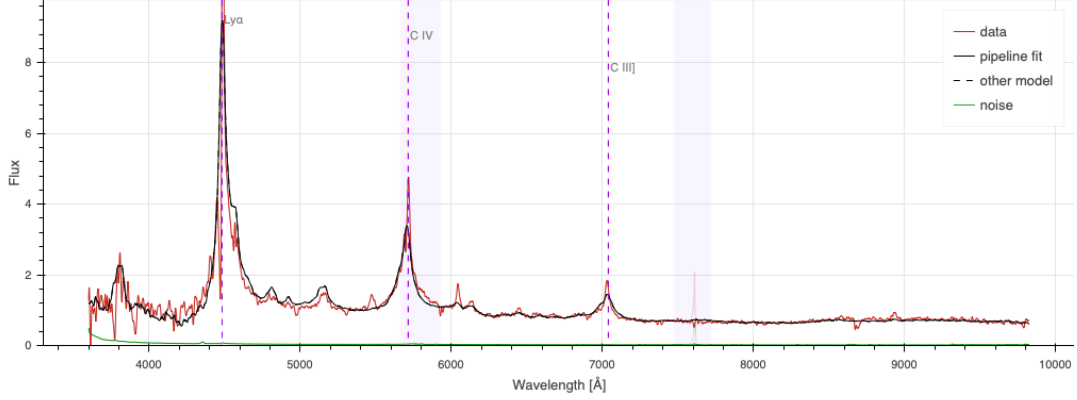
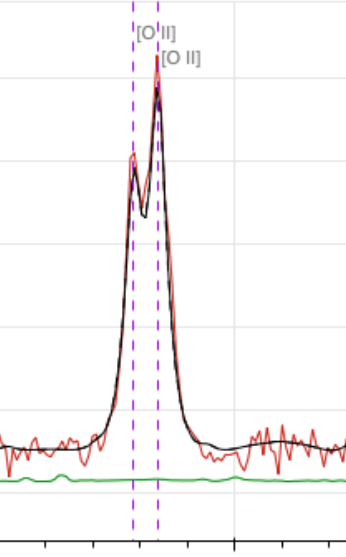
\(z = 2.1\) QSO
\(z = 0.9\) ELG
Ly\(\alpha\)
CIV
CIII
[OII] doublet at \(3727 \AA\) up to \(z = 1.6\)
[OII]
Ly\(\alpha\) at \(1216 \AA\) down to \(z = 2.0\)

DESI data release 2 (DR2)

Observations from May 14th 2021 to April 9th 2024
approved
construction started
first light
survey started
DR1 data sample
DR1 results
DR2 sample secured
end of the survey...
... DESI-2
DR2 results
Final survey
- dark time (LRG, ELG, QSO): 7 visits
- bright time (BGS): 5 visits
- 14,000 \(\mathrm{deg}^2\)
2015
16
17
18
19
20
22
23
24
21
25
26
27

DESI data release 2 (DR2)
- 30M galaxy and QSO redshifts in 3 years of operation
- 14M used in the DR2 analysis (6M in DR1)
- Including 820,000 Ly\(\alpha\) QSO at \(z > 2.09\) (420,000 in DR1)
- \(> 2\times\) increase in number of tracers

higher completeness (deeper)
extended mag cut

Release of DESI DR2 (BAO) results
March 19th 2025
First batch of DESI DR2 cosmological analyses: https://data.desi.lbl.gov/doc/papers/
• DESI Collaboration et al. (2025), DESI DR2 Results I: Baryon Acoustic Oscillations from the Lyman Alpha Forest
• DESI Collaboration et al. (2025), DESI DR2 Results II: Measurements of Baryon Acoustic Oscillations and Cosmological Constraints
Companion supporting papers:
Lodha et al. (2025), Extended Dark Energy analysis
Elbers et al. (2025), Constraints on Neutrino Physics
Andrade et al. (2025), Validation of the DESI DR2 BAO mesurements
Casas et al. (2025), Validation of the DESI DR2 Lyα BAO analysis using synthetic datasets
Brodzeller et al. (2025), Construction of the Damped Lyα Absorber Catalog for DESI DR2 Lyα BAO
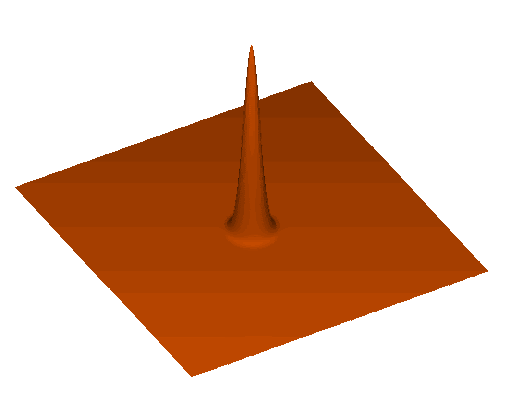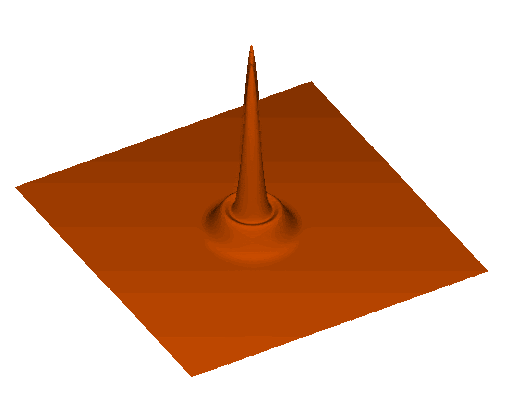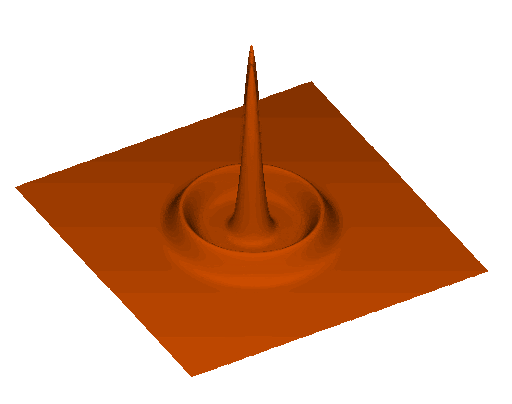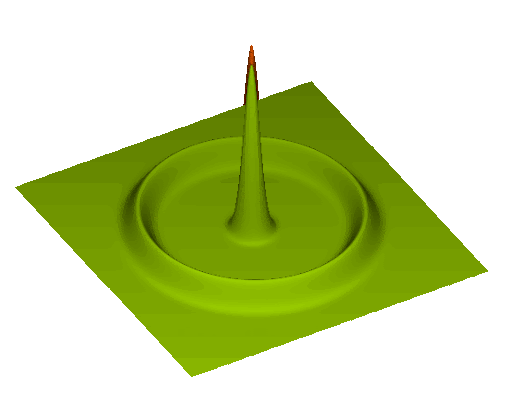
Baryon Acoustic Oscillations
Sound waves in primordial plasma
At recombination (\(z \simeq 1100\))
- plasma changes to optically thin
- baryons decouple from photons
- sound wave stalls after travelling \(r_\mathrm{d}\)
Sound horizon scale at the drag epoch
\(r_\mathrm{d} \simeq 150\; \mathrm{Mpc}\)
standard ruler
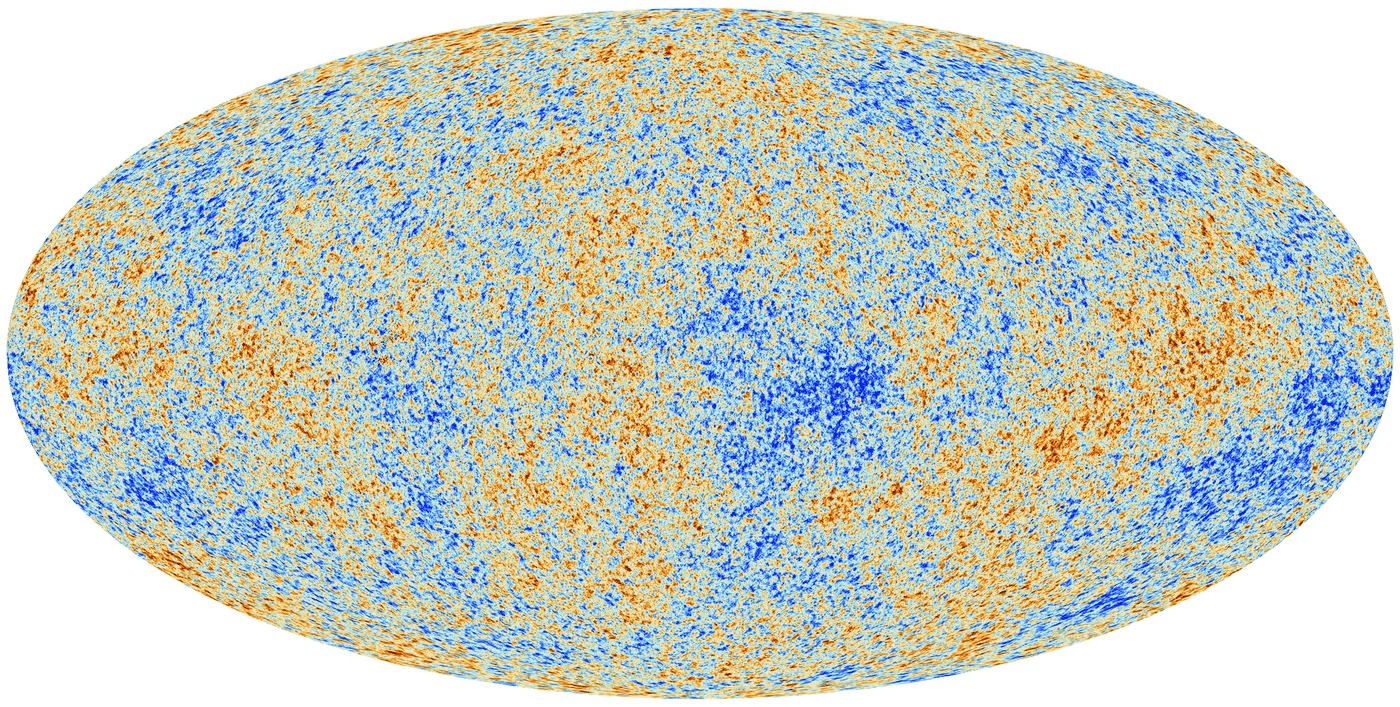

Baryon Acoustic Oscillations
CMB (\(z \simeq 1100\))
Sound waves in primordial plasma
At recombination (\(z \simeq 1100\))
- plasma changes to optically thin
- baryons decouple from photons
- sound wave stalls after travelling \(r_\mathrm{d}\)
Sound horizon scale at the drag epoch
\(r_\mathrm{d} \simeq 150\; \mathrm{Mpc}\)
standard ruler


CMB (\(z \simeq 1100\))
LSS



Baryon Acoustic Oscillations

BAO measurements

line of sight
distribution of galaxies (cartoonish)


BAO measurements
correlation function



BAO peak
line of sight
monopole

BAO measurements
correlation function



BAO peak
line of sight
monopole
isotropic
comoving transverse distance
Hubble distance \(c/H(z)\)
sound horizon (standard ruler)

BAO measurements
isotropic
anisotropic





BAO peak
line of sight
line of sight
monopole
quadrupole


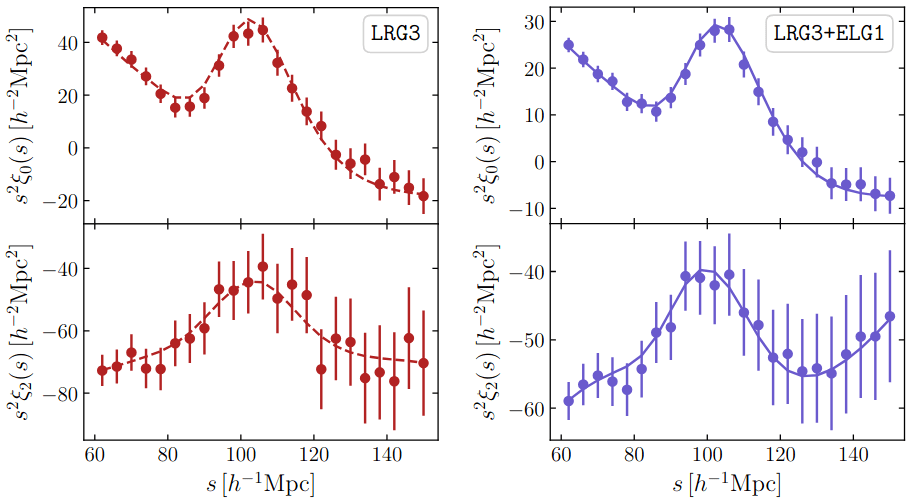
low S/N
BAO detection: \(14.7\sigma\)
0.1 < z < 0.4
0.4 < z < 0.6
0.6 < z < 0.8
0.8 < z < 1.1
1.1 < z < 1.6


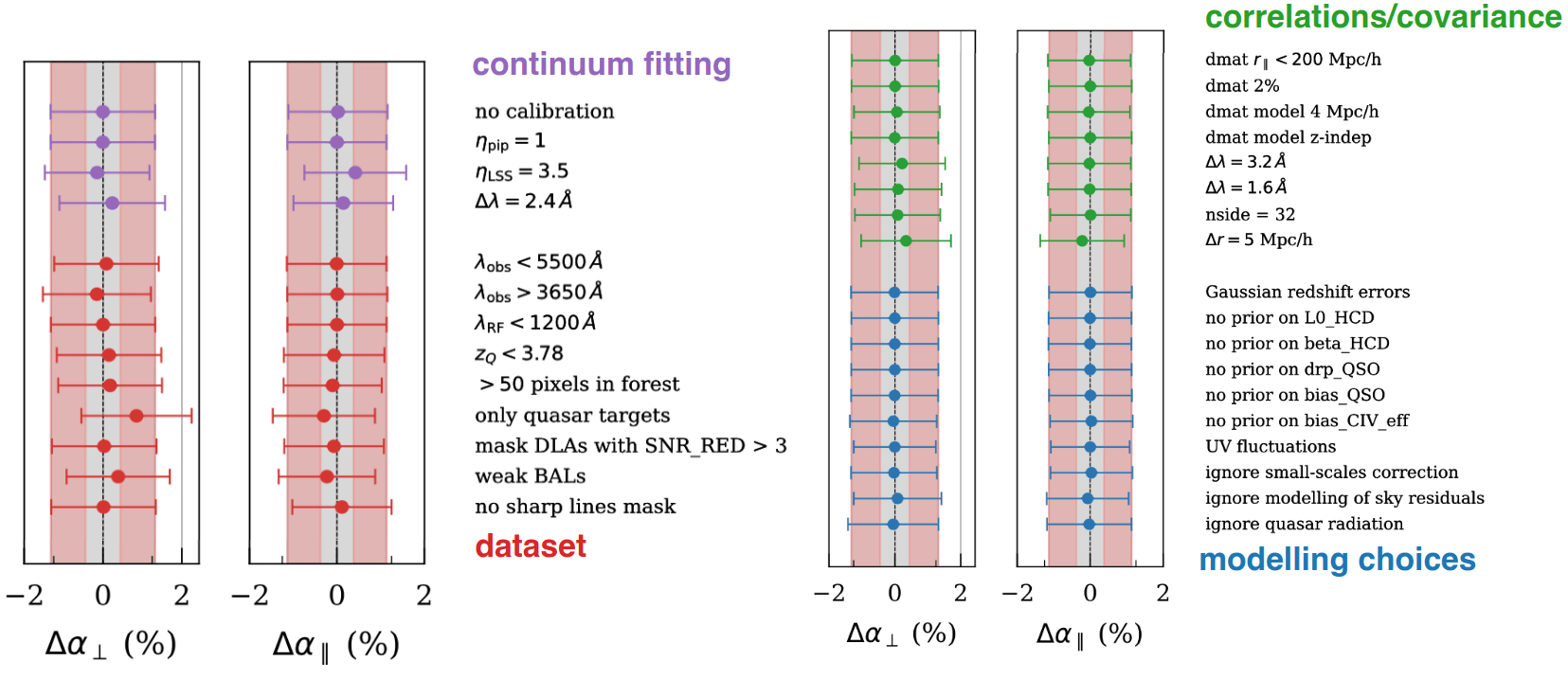
Robustness tests
tracers / redshift bins
data vector


Robustness tests
tracers / redshift bins
BAO modelling


Robustness tests
tracers / redshift bins
imaging systematics


Robustness tests
tracers / redshift bins
data splits




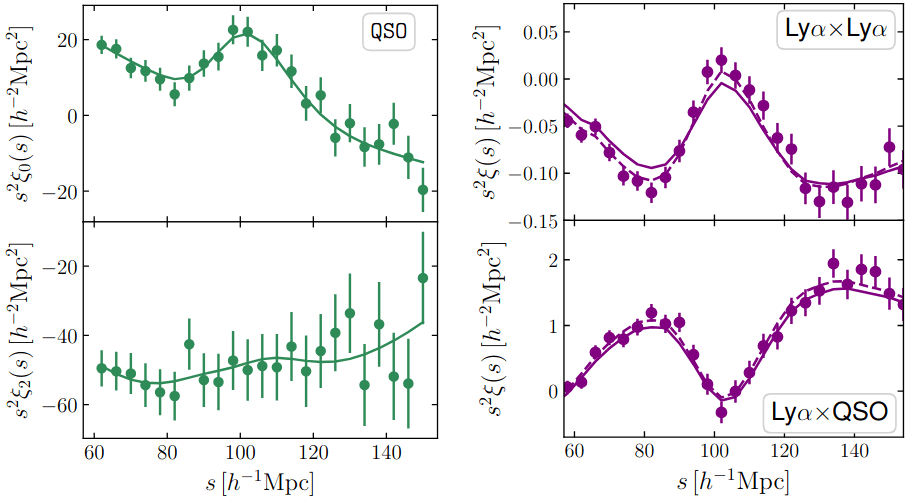
Ly\(\alpha\) forest
Ly\(\alpha\) forest
Absorption in QSO spectra by neutral hydrogen in the intergalactic medium: \(\lambda_\mathrm{abs} = (1 + z_\mathrm{HI}) \times 1215.17 \; \AA \)
Transmitted flux fraction \(F = e^{-\tau}\) probes the fluctuation in neutral hydrogen density, \(\tau \propto n_\mathrm{HI} \)
credit: Andrew Pontzen

Correlation functions
Ly\(\alpha\) forest auto-correlation
\(\langle \delta_F(\mathbf{x}) \delta_F(\mathbf{x + s}) \rangle\)
Ly\(\alpha\) forest - QSO cross-correlation
\(\langle \delta_F(\mathbf{x}) Q(\mathbf{x + s}) \rangle\)

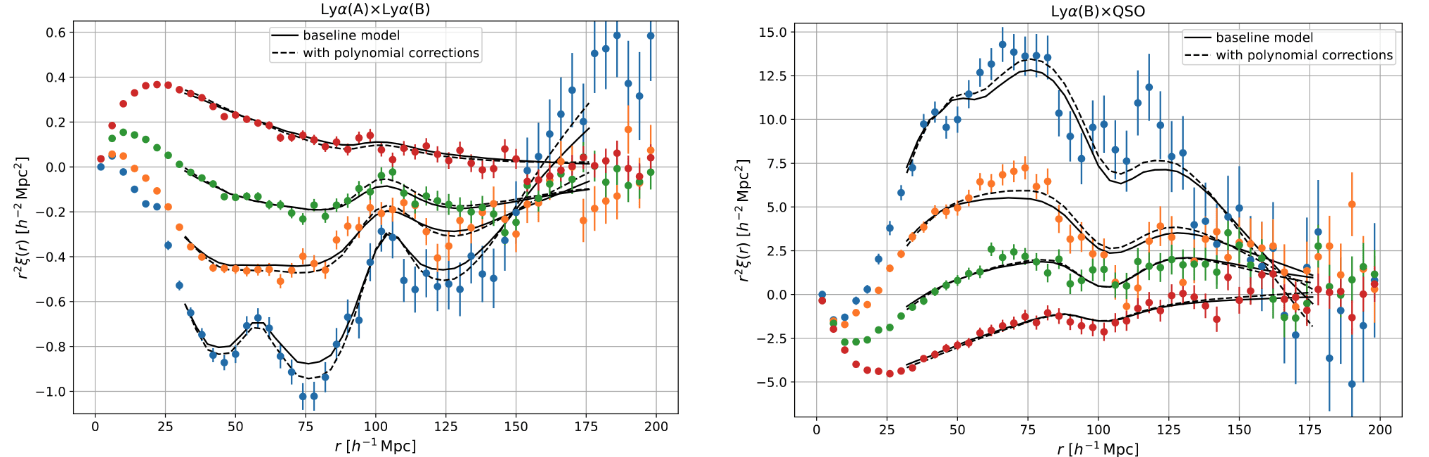
Robustness tests
data vector / covariance

Robustness tests

modelling choices

Robustness tests

continuum fitting

Robustness tests

data splits

What's new in the analyses?
Analysis pipelines mostly the same as DR1
Again, blind analyses:
- discrete tracers: catalog-level blinding
- Ly\(\alpha\): data vector-level blinding
Specifics:
- discrete tracers: more robustness tests, increased BGS density
- Ly\(\alpha\): improved mocks / modelling (DLA, metals, continuum fitting)
Some updates in BAO fitting
Subdominant systematics: \(\sigma_\mathrm{syst} / \sigma_\mathrm{stat} < 9\%\) for discrete tracers, \(40\%\) for Ly\(\alpha\)

DESI DR2 BAO
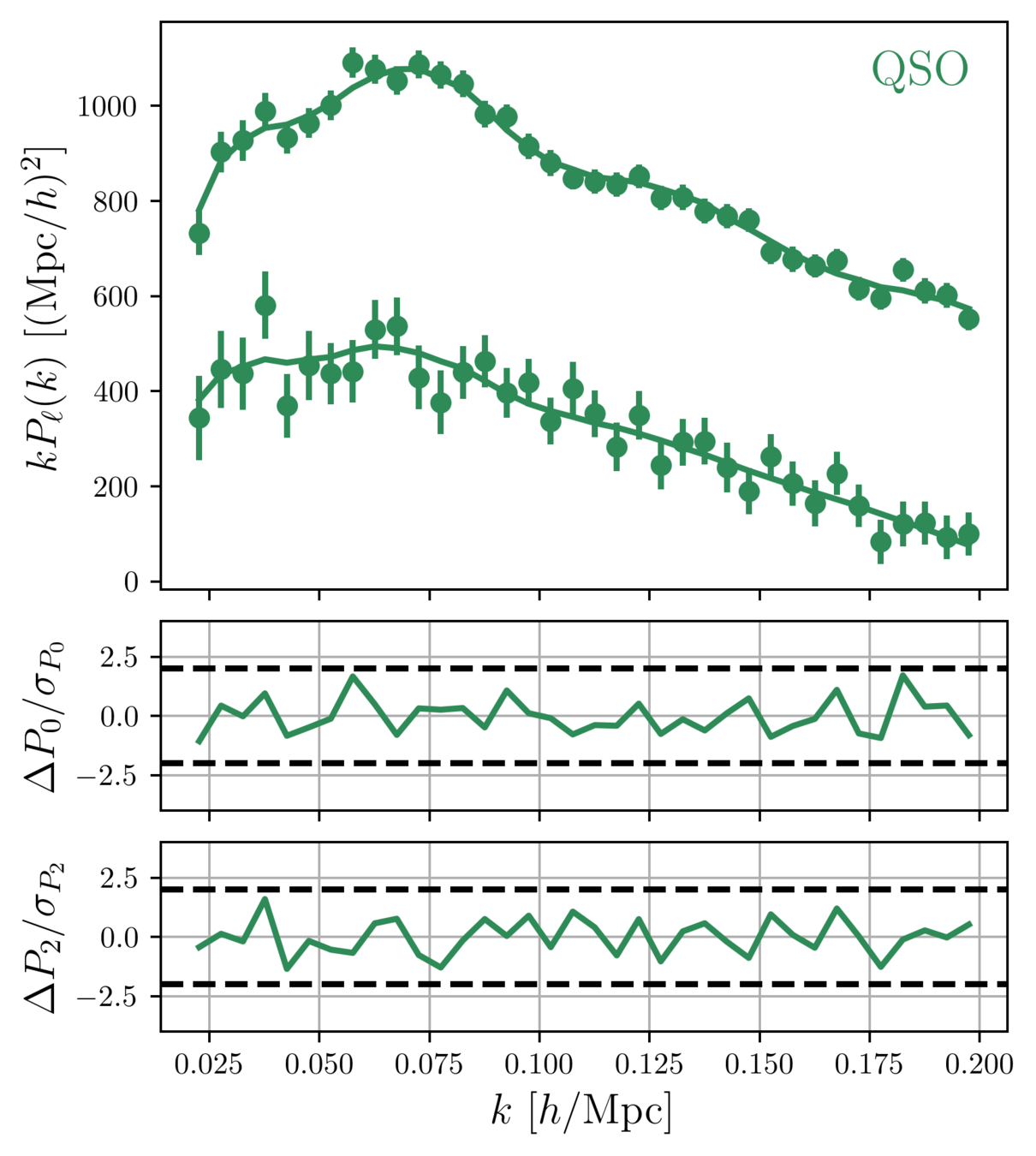
DESI DR2 BAO measurements
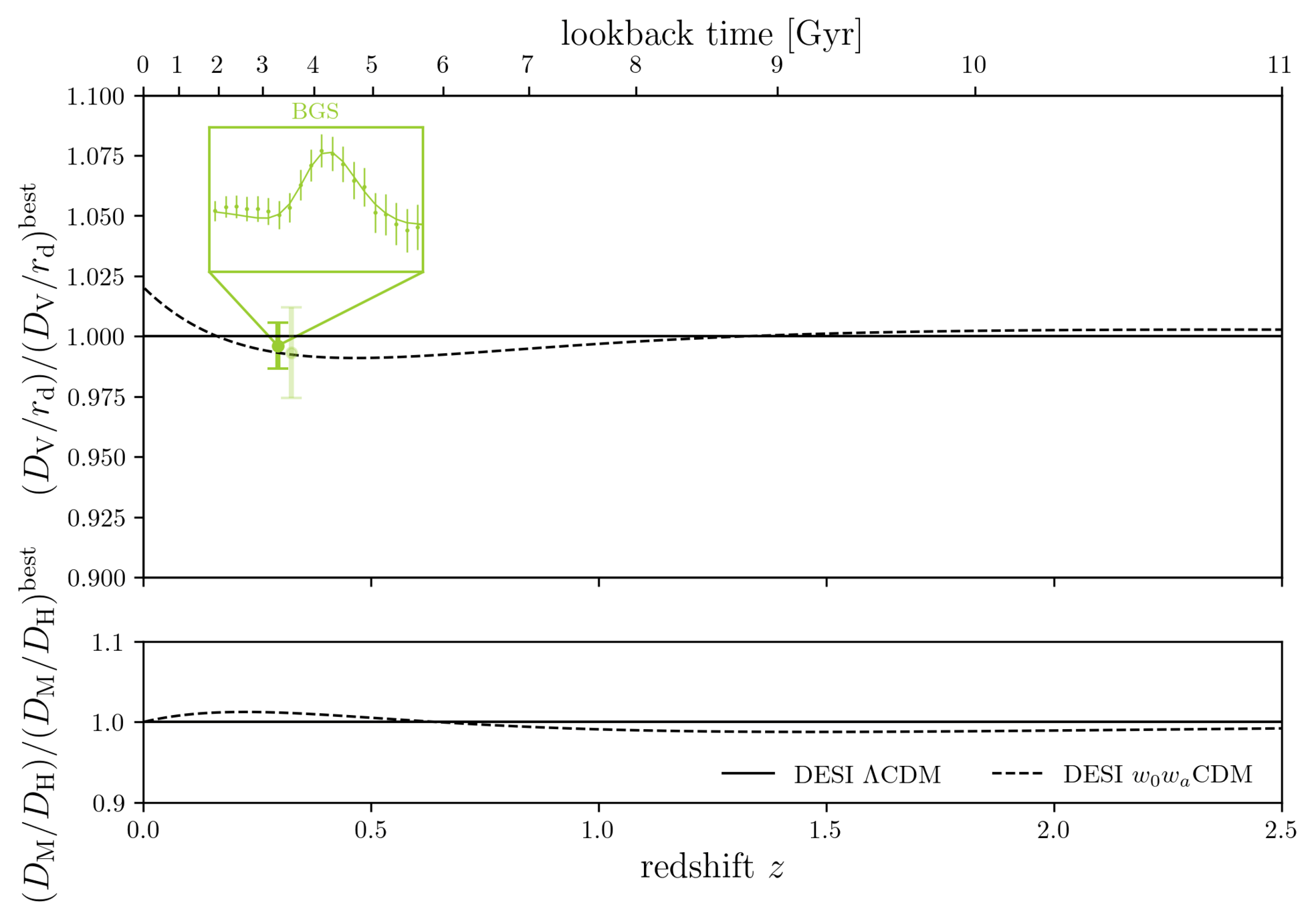
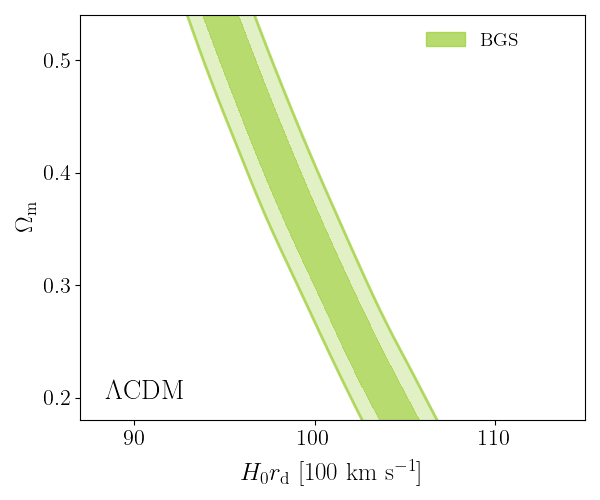

DESI DR2 BAO
DESI DR2 BAO measurements
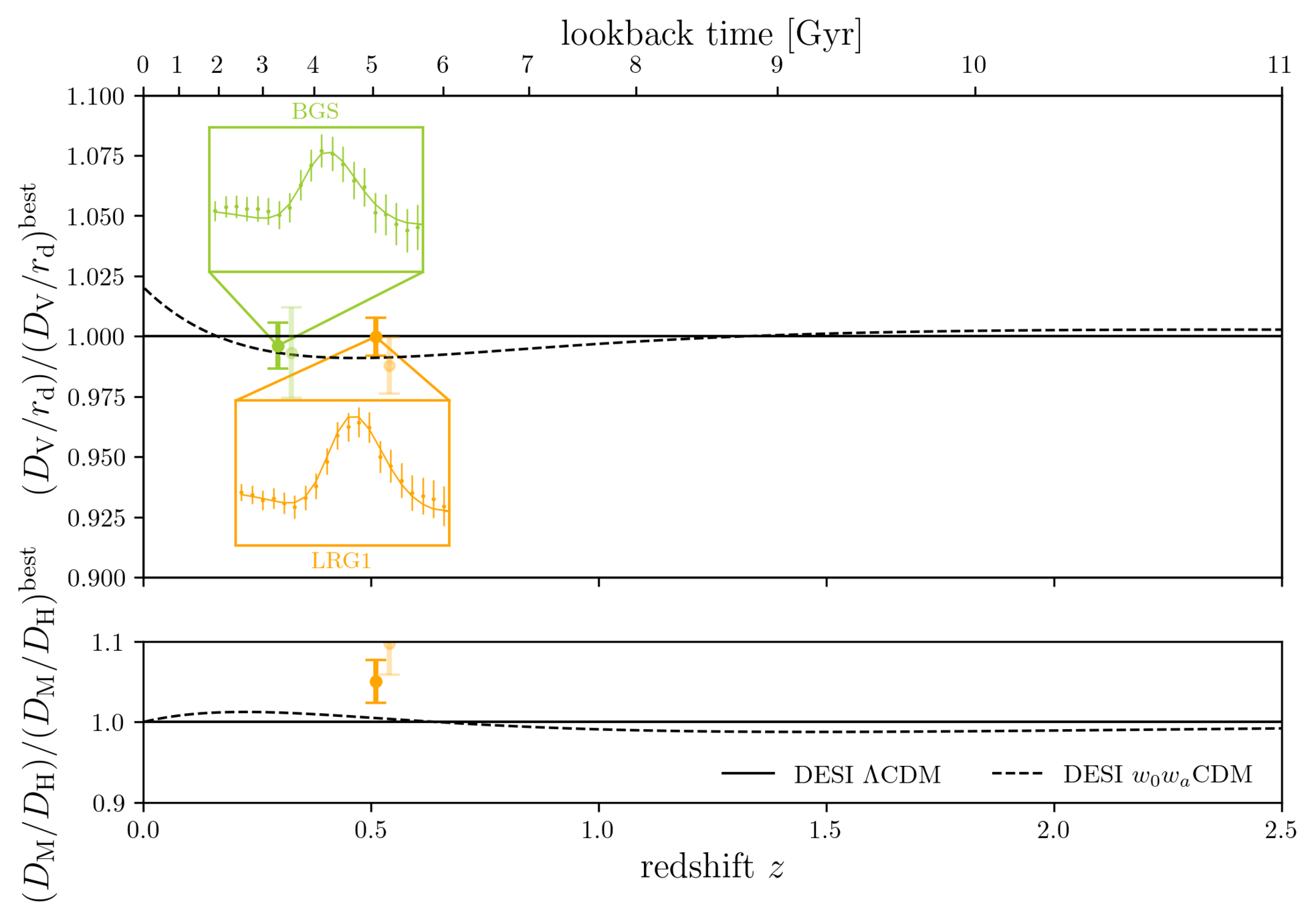
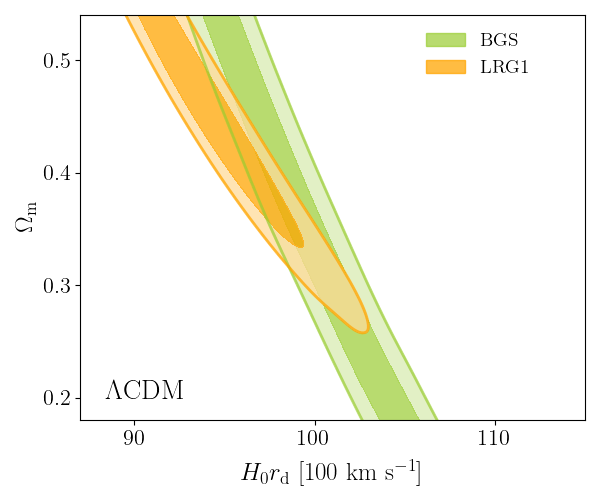

DESI DR2 BAO
DESI DR2 BAO measurements
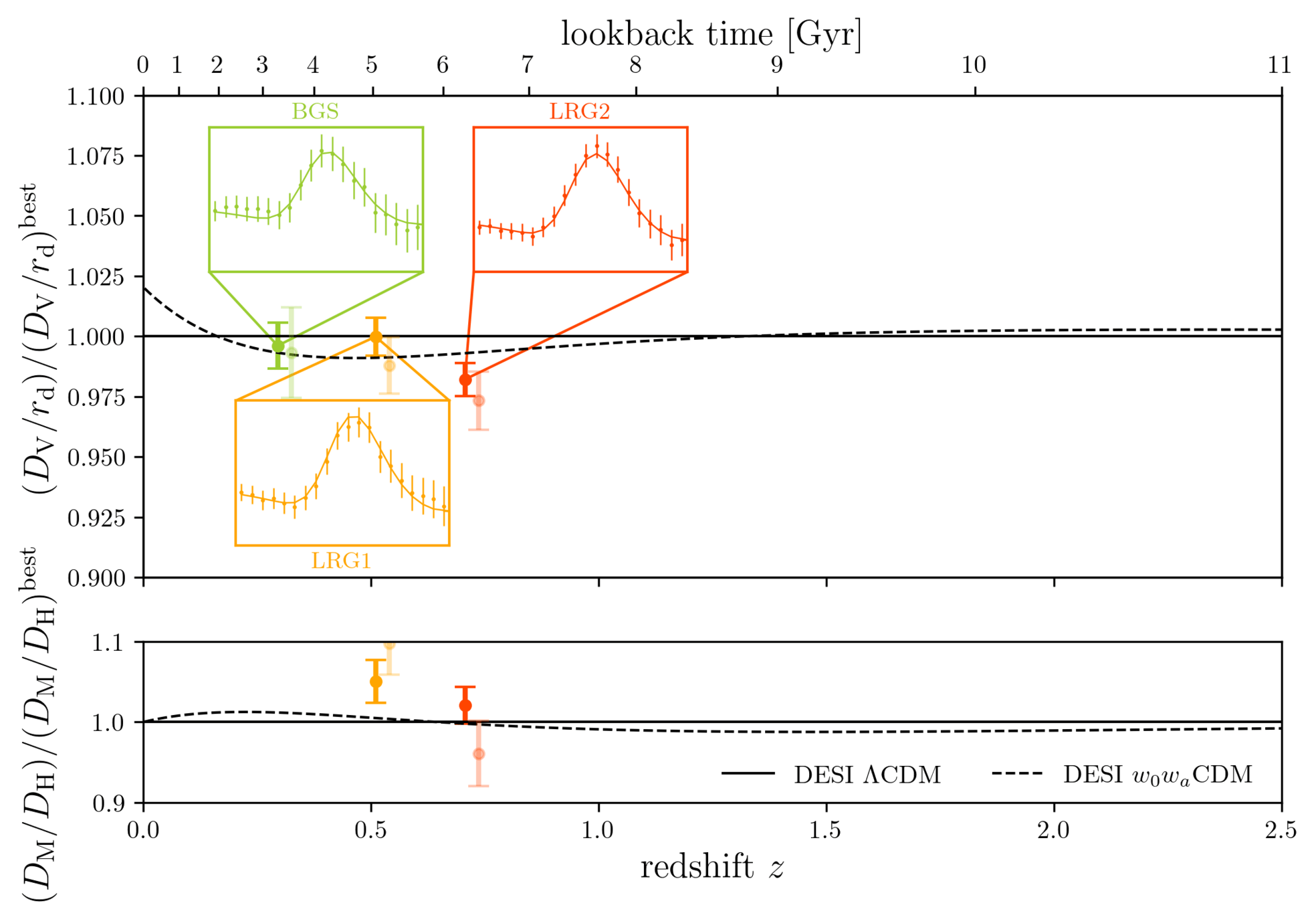

DESI DR2 BAO
DESI DR2 BAO measurements
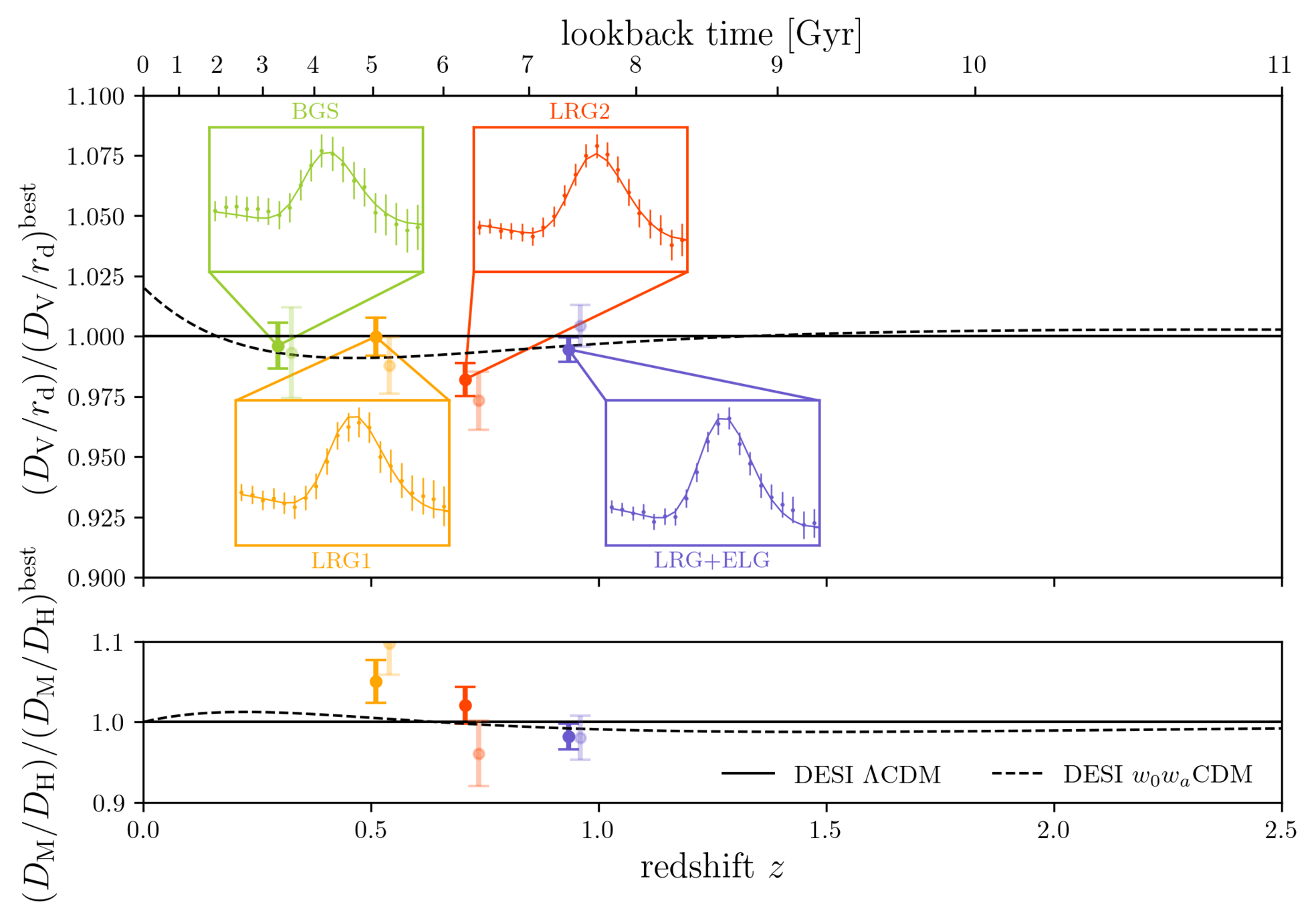

DESI DR2 BAO
DESI DR2 BAO measurements

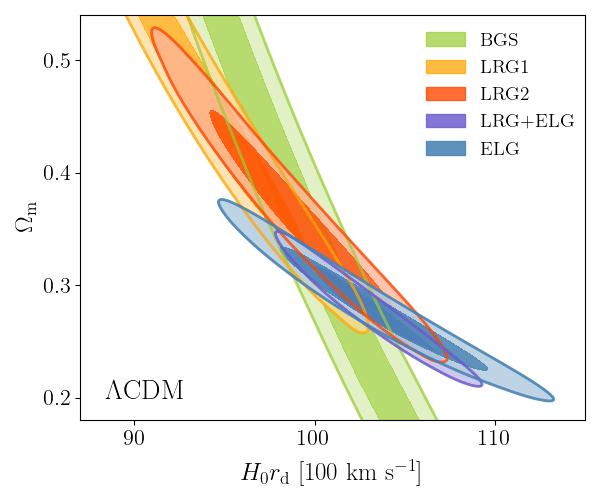

DESI DR2 BAO
DESI DR2 BAO measurements

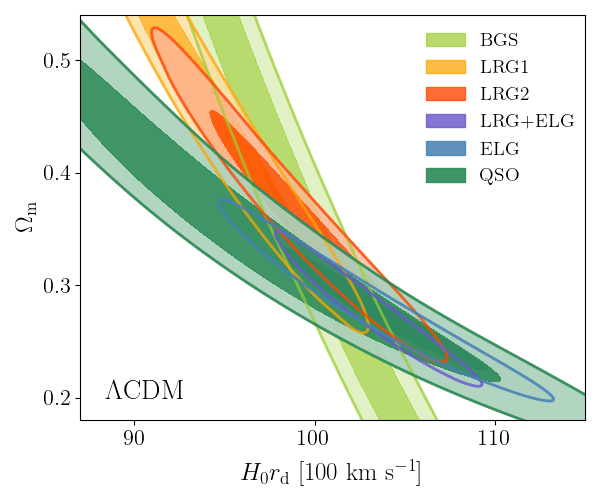

DESI DR2 BAO
DESI DR2 BAO measurements



Consistent with each other,
and complementary
DESI DR2 BAO
DESI DR2 BAO measurements
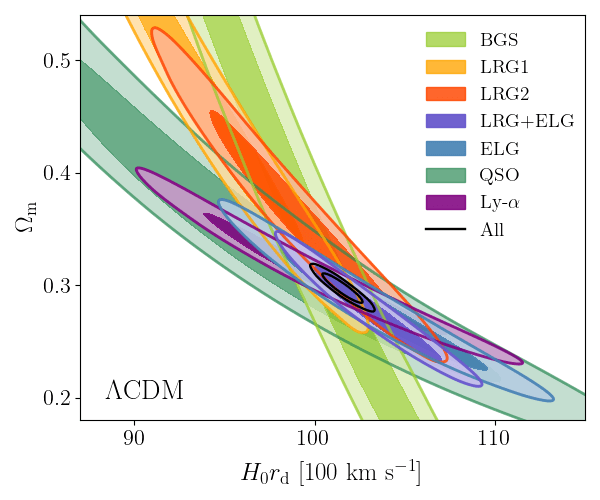

- DESI DR2 BAO fully consistent with DESI DR1
- Improvement of \(\simeq 40\%\)
- \(2.3 \sigma\) discrepancy with primary CMB¹ + CMB lensing²
Consistency with other data

1. Planck PR4 CamSpec
2. Planck PR4 + ACT DR6 lensing

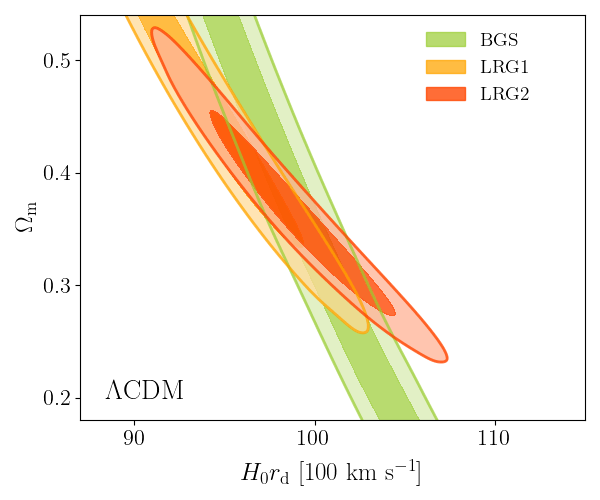
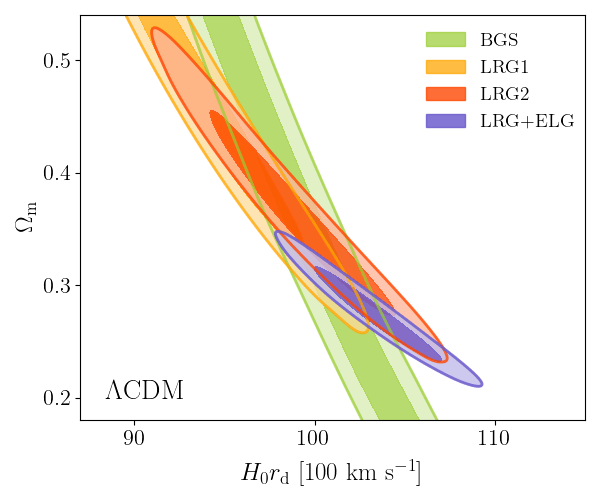
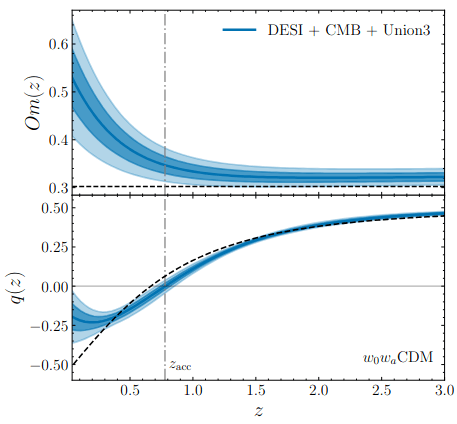
- BAO constrains \(\Omega_\mathrm{m}\), \(h \times r_d(\Omega_\mathrm{b}h^2, \Omega_\mathrm{m}h^2)\)
- Calibrating BAO relative distance measurements using BBN \(\Omega_\mathrm{b} h^2\)
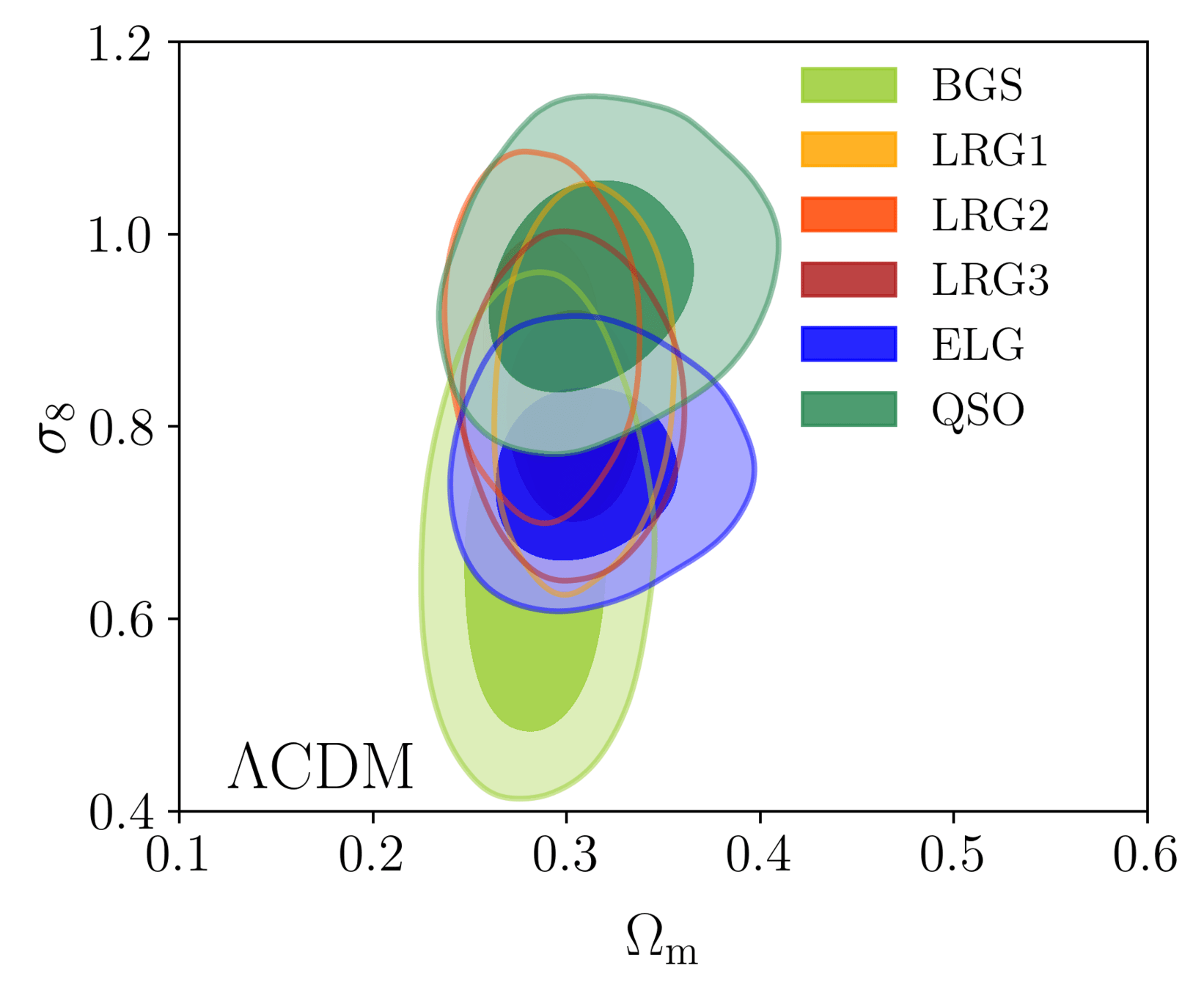
\(\Lambda\mathrm{CDM}\) constraints
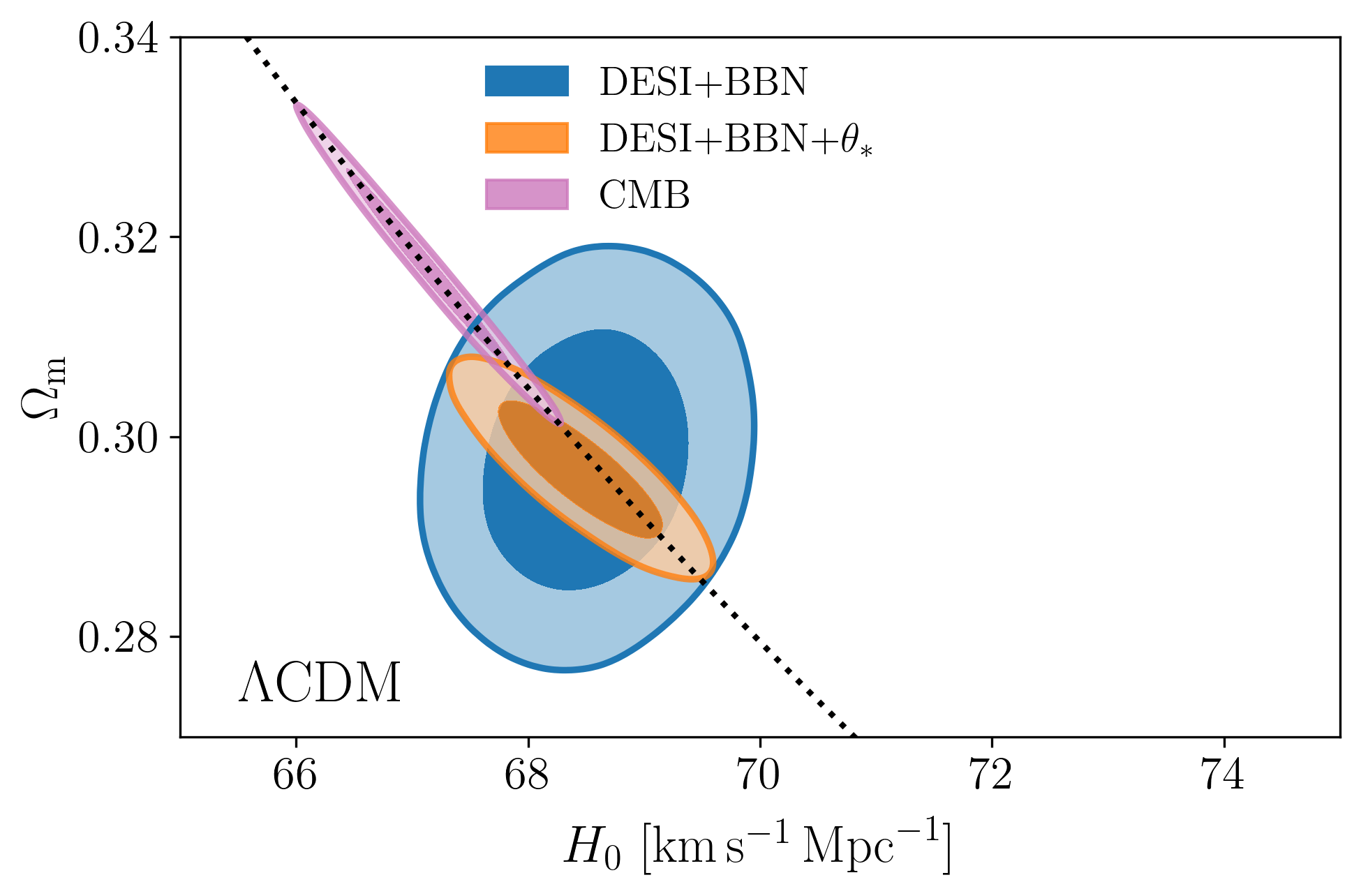

\(\Lambda\mathrm{CDM}\) constraints

- BAO constrains \(\Omega_\mathrm{m}\), \(h \times r_d(\Omega_\mathrm{b}h^2, \Omega_\mathrm{m}h^2)\)
- Calibrating BAO relative distance measurements using BBN \(\Omega_\mathrm{b} h^2\)
- Adding very precise CMB acoustic angular scale

\(\Lambda\mathrm{CDM}\) constraints
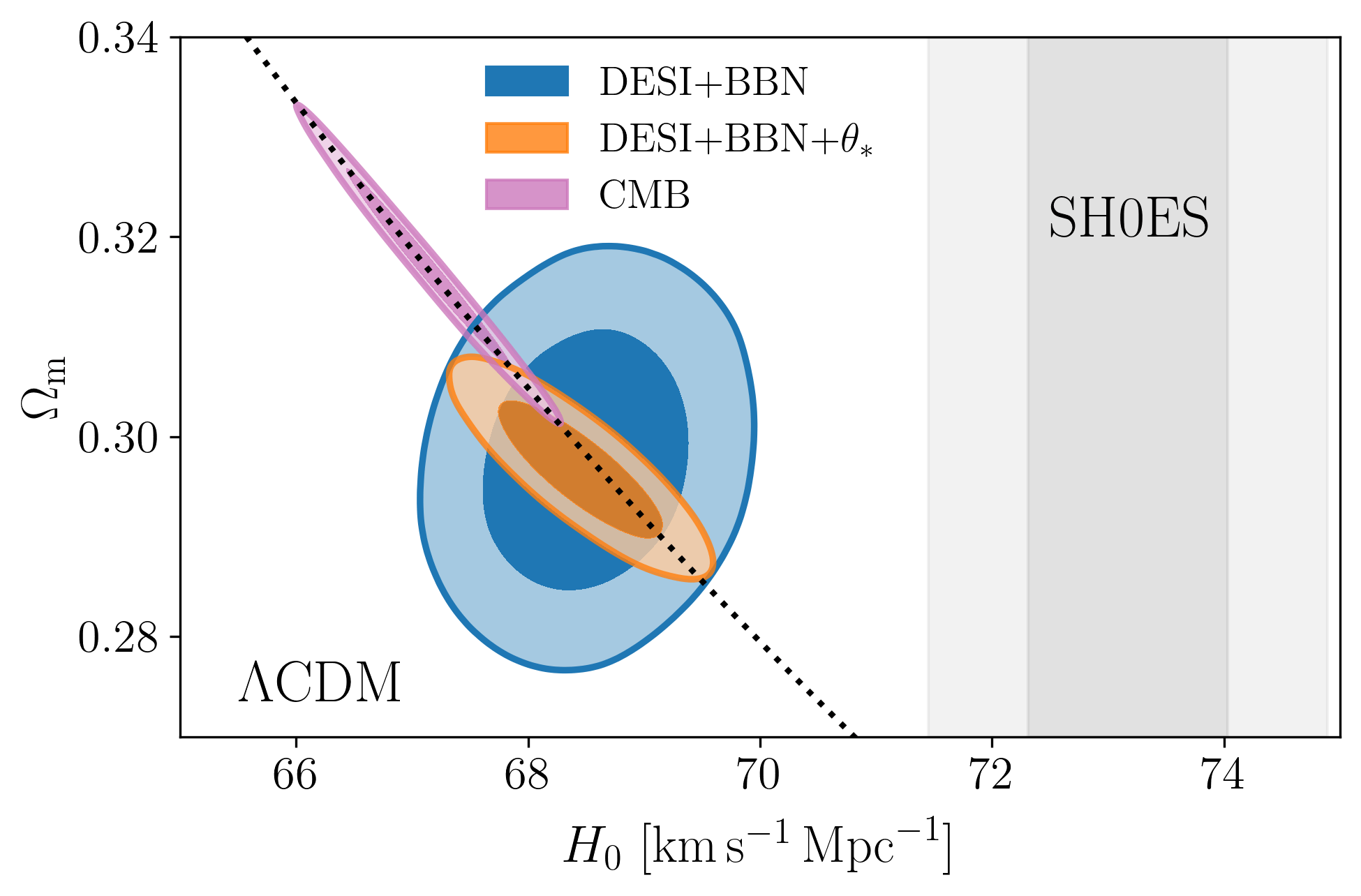
- In \(4.5\sigma\) tension with SH0ES (Breuval et al. 2024) (independently of the CMB)
- BAO constrains \(\Omega_\mathrm{m}\), \(h \times r_d(\Omega_\mathrm{b}h^2, \Omega_\mathrm{m}h^2)\)
- Calibrating BAO relative distance measurements using BBN \(\Omega_\mathrm{b} h^2\)

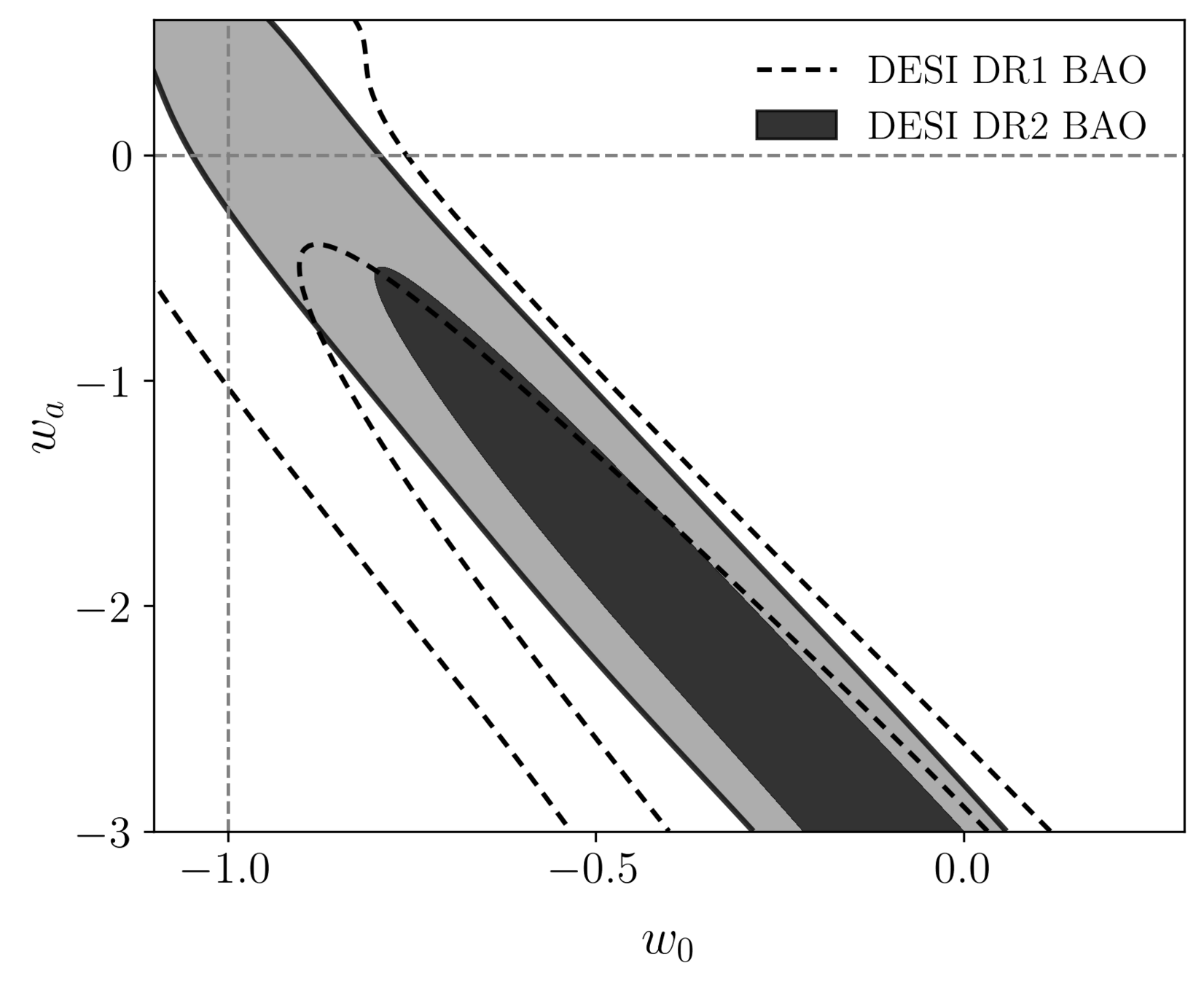
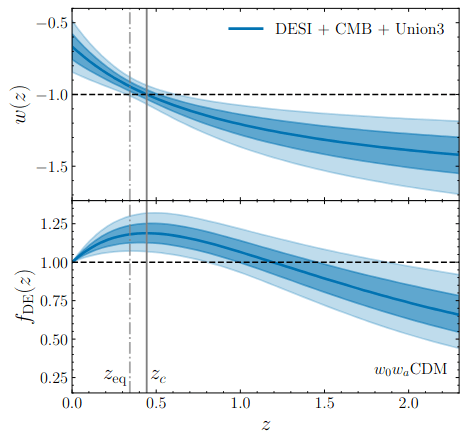
- Dark energy fluid
- No strong preference for dark energy evolution: \(1.7\sigma\) from DESI data alone
Dark Energy Equation of State
\(\Lambda\)
pressure
density
CPL

- Combining DESI + CMB:
Dark Energy Equation of State
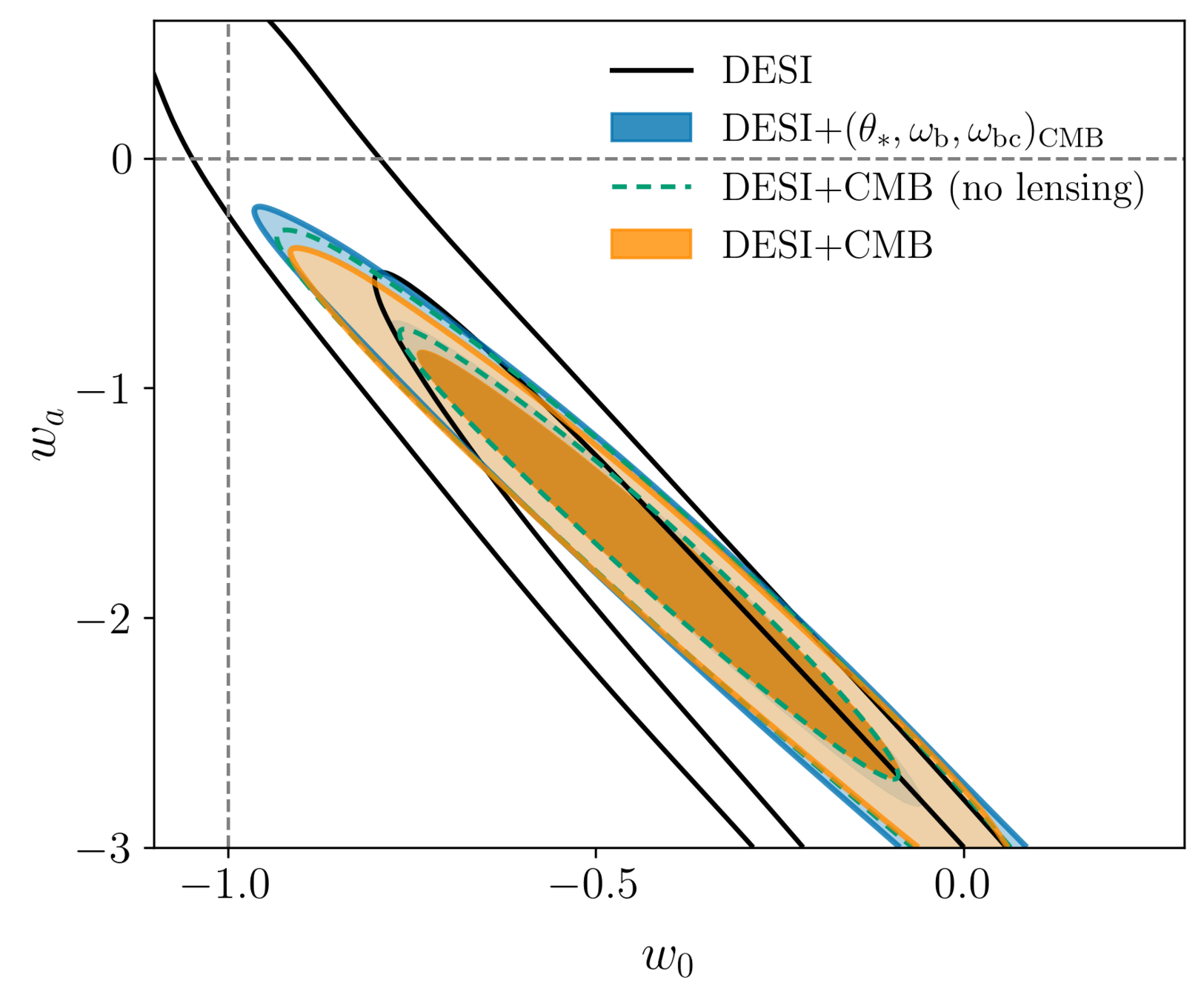
- CMB priors (marginalizing over late-time physics): \(2.4\sigma\)
- Without CMB lensing \(2.7\sigma\)
\(+0.5\sigma\) compared to DR1

Dark Energy Equation of State
Combining all DESI + CMB + SN

\(+0.3\sigma\) compared to DR1

Robustness tests
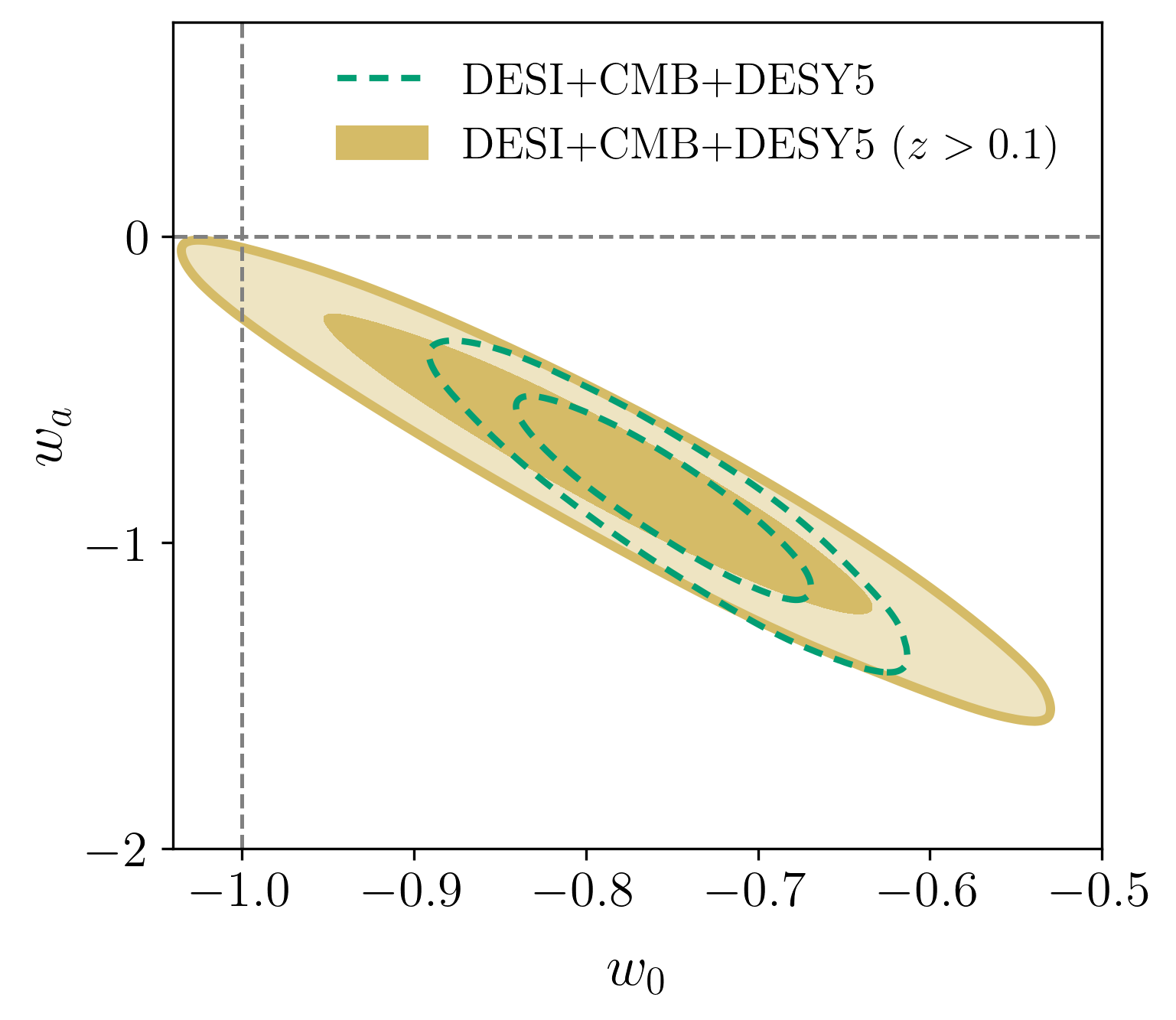
Removing low-\(z\) SN
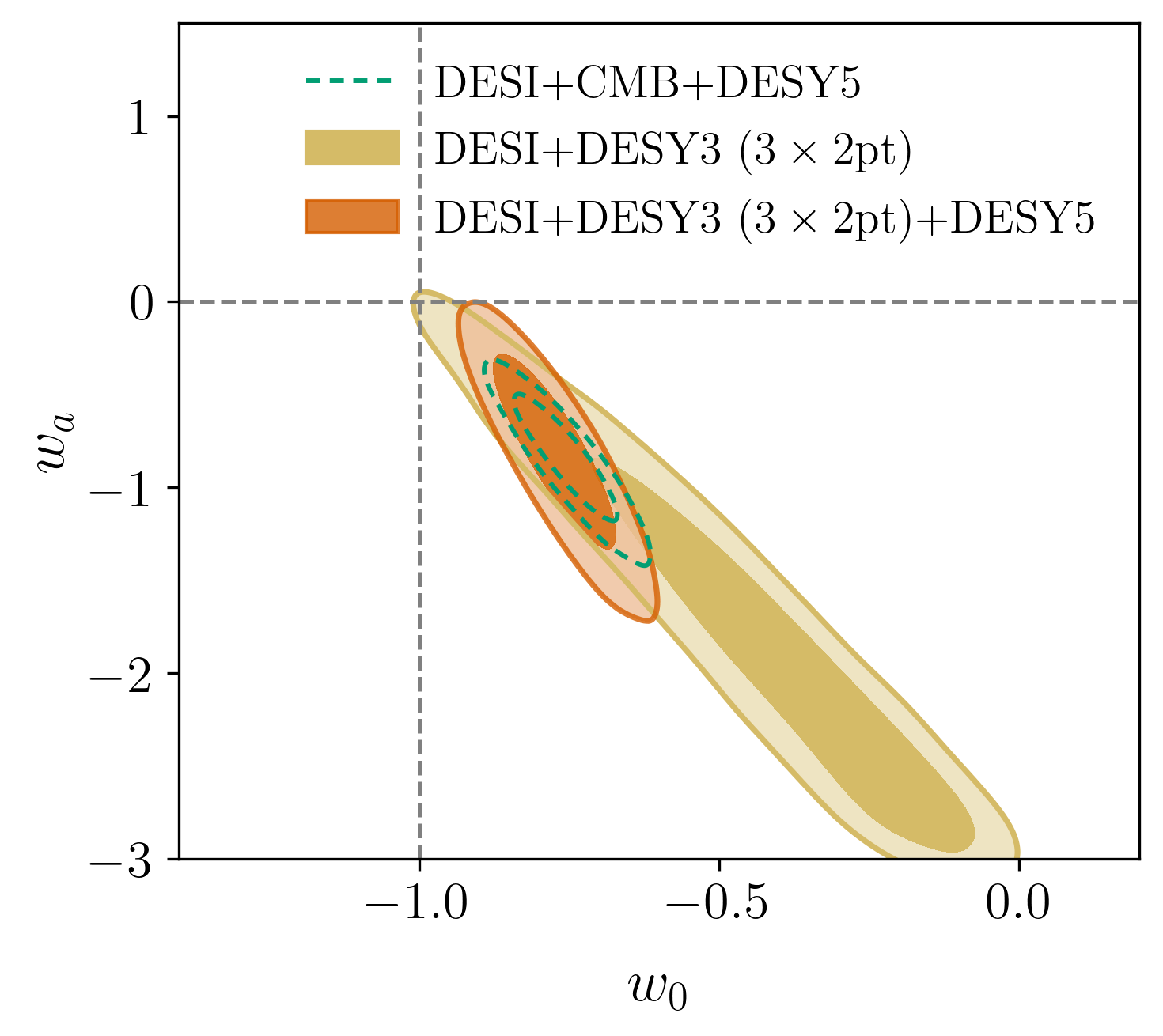
"Replacing CMB": DESY3 \(3\times2\)pt
\(3.3\sigma\)

Understanding tensions
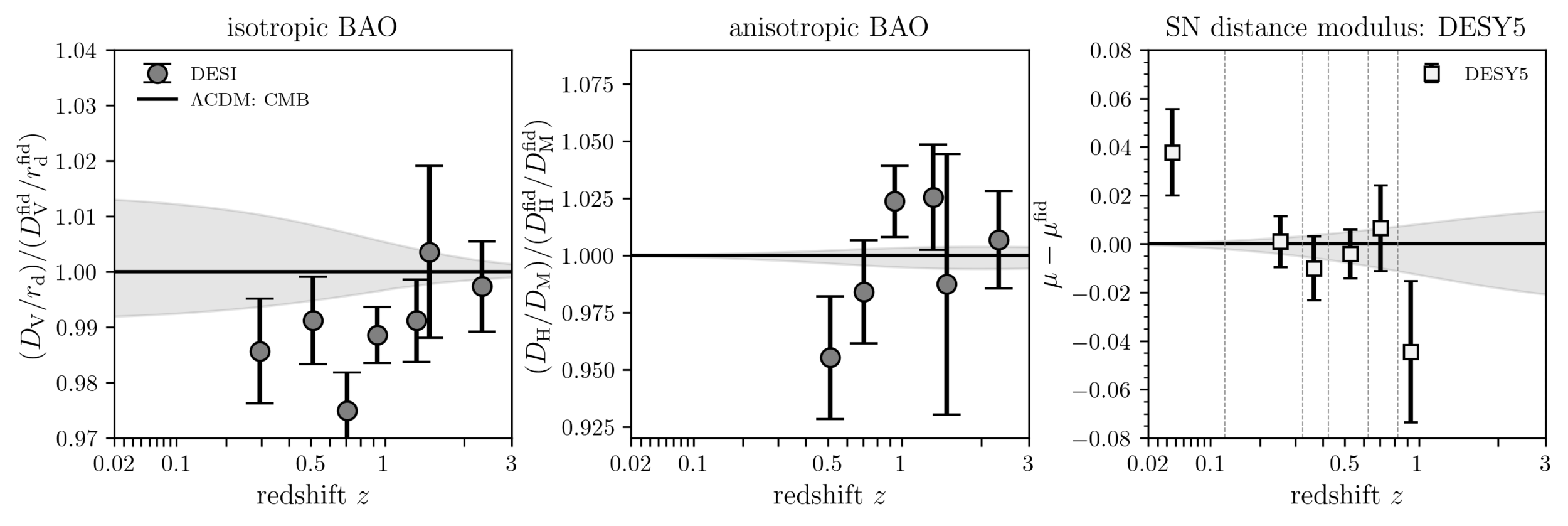

Understanding tensions
doesn't fit the SN!
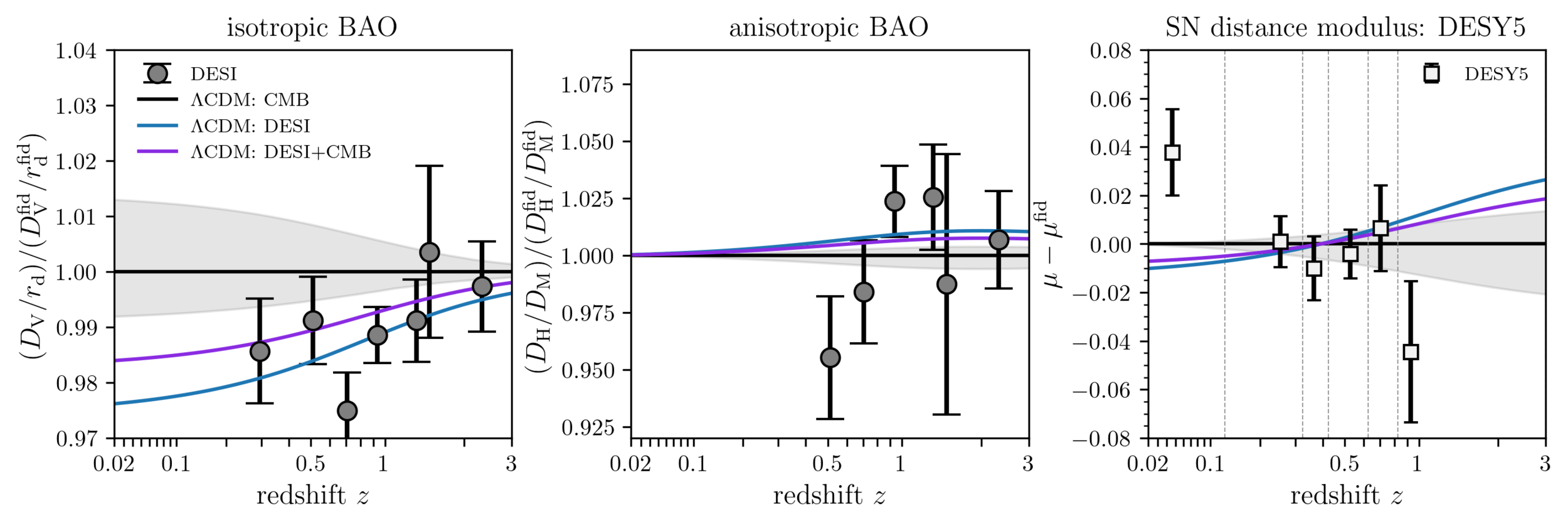

Understanding tensions
doesn't fit the BAO!
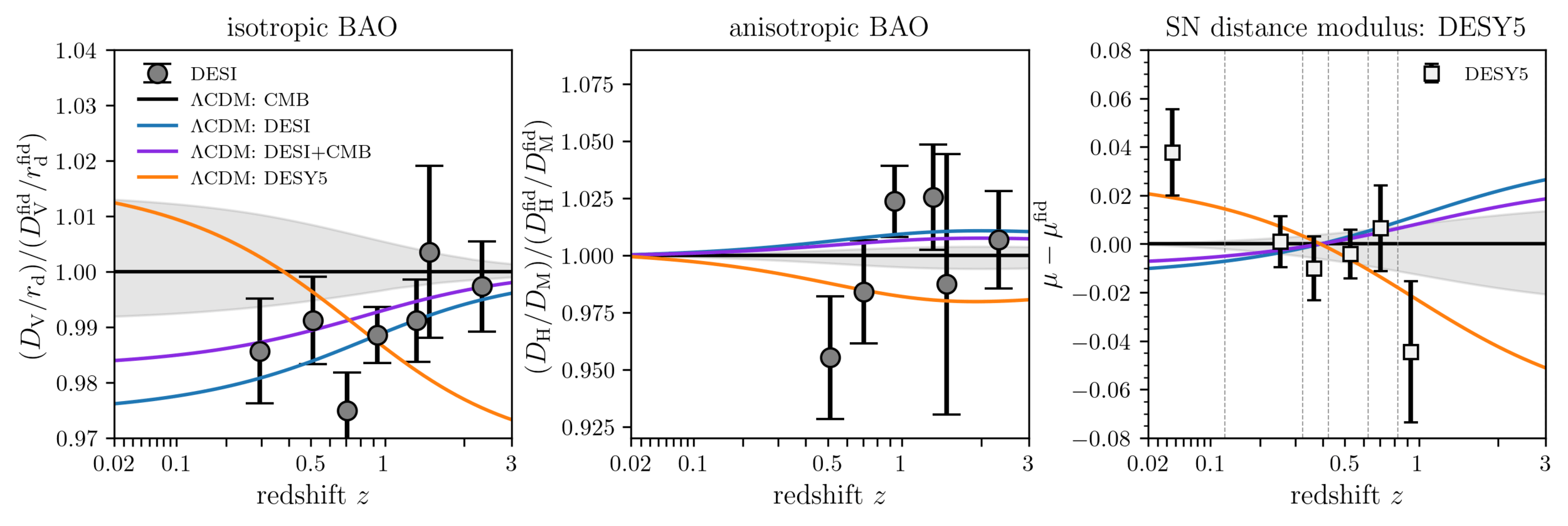

Understanding tensions
\(w\mathrm{CDM}\) not flexible enough to fit all 3 datasets!
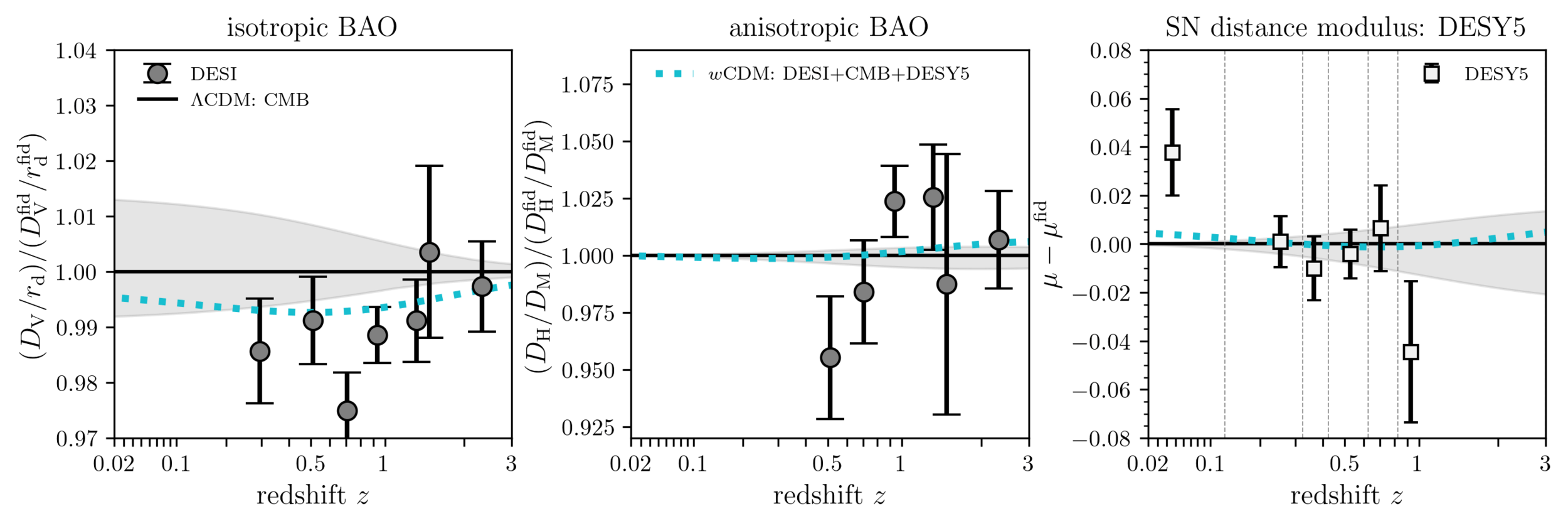

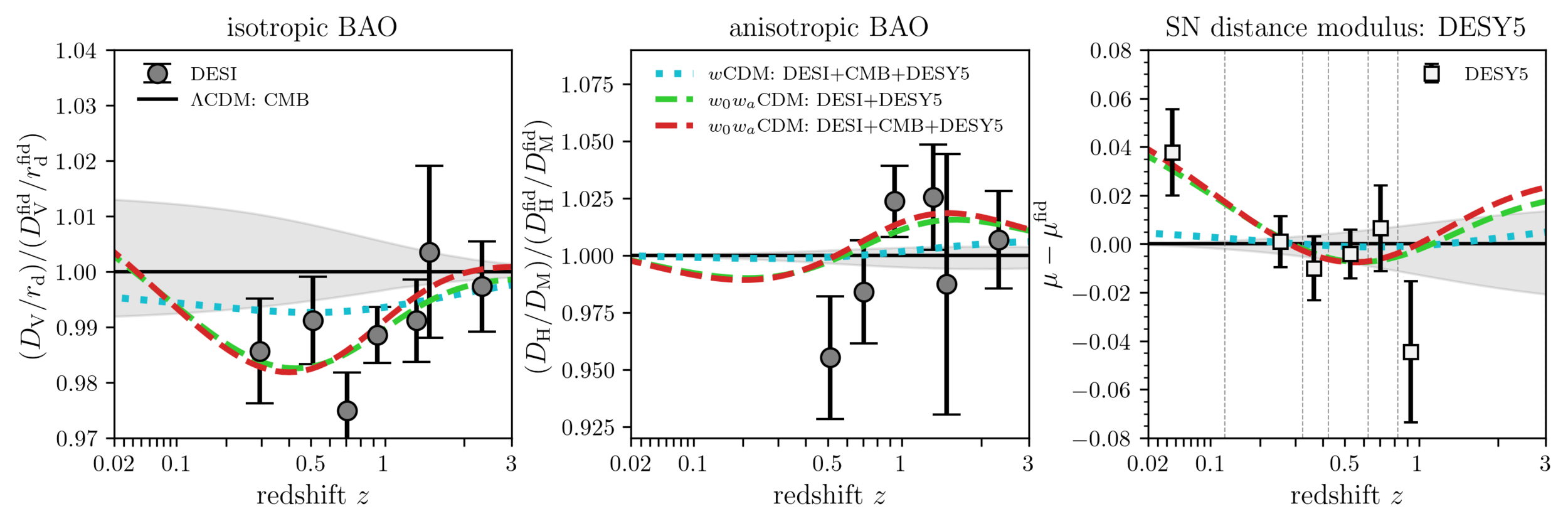
Understanding tensions
\(w_0w_a\mathrm{CDM}\) fits all 3 datasets!
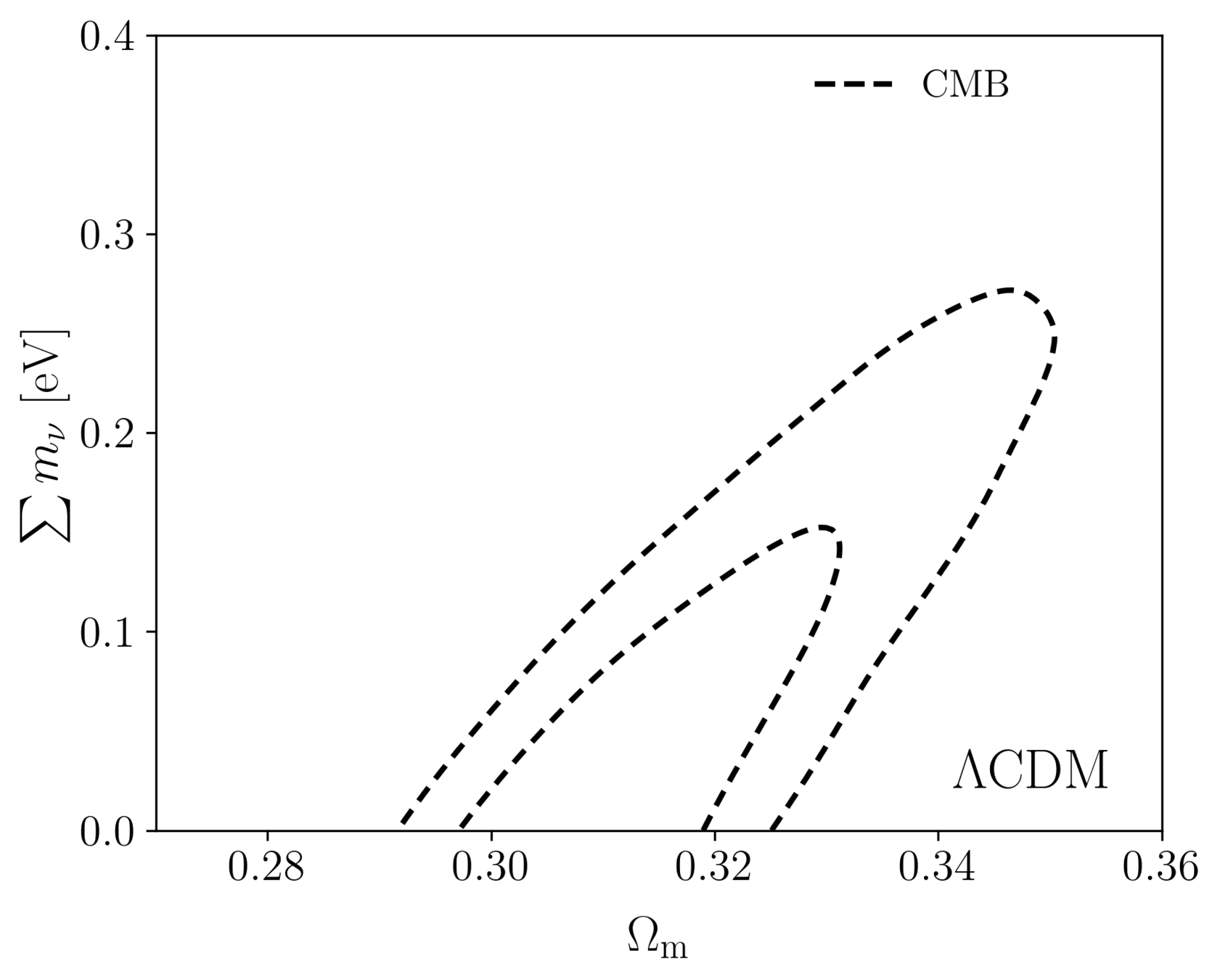
Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by BAO
Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
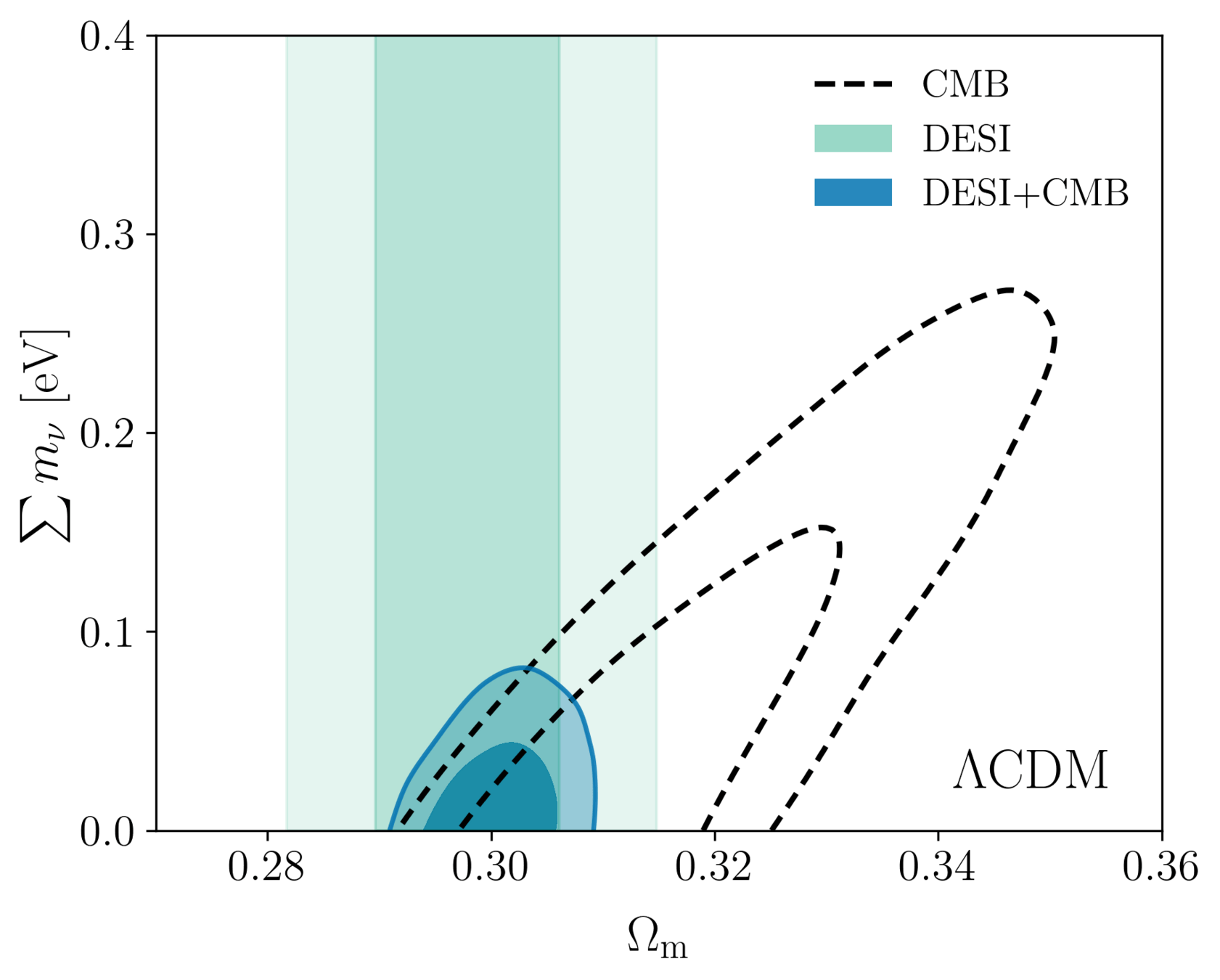
Broken by BAO, which favors low \(\Omega_\mathrm{m}\)
Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by BAO, which favors low \(\Omega_\mathrm{m}\)
Variations of the CMB likelihood
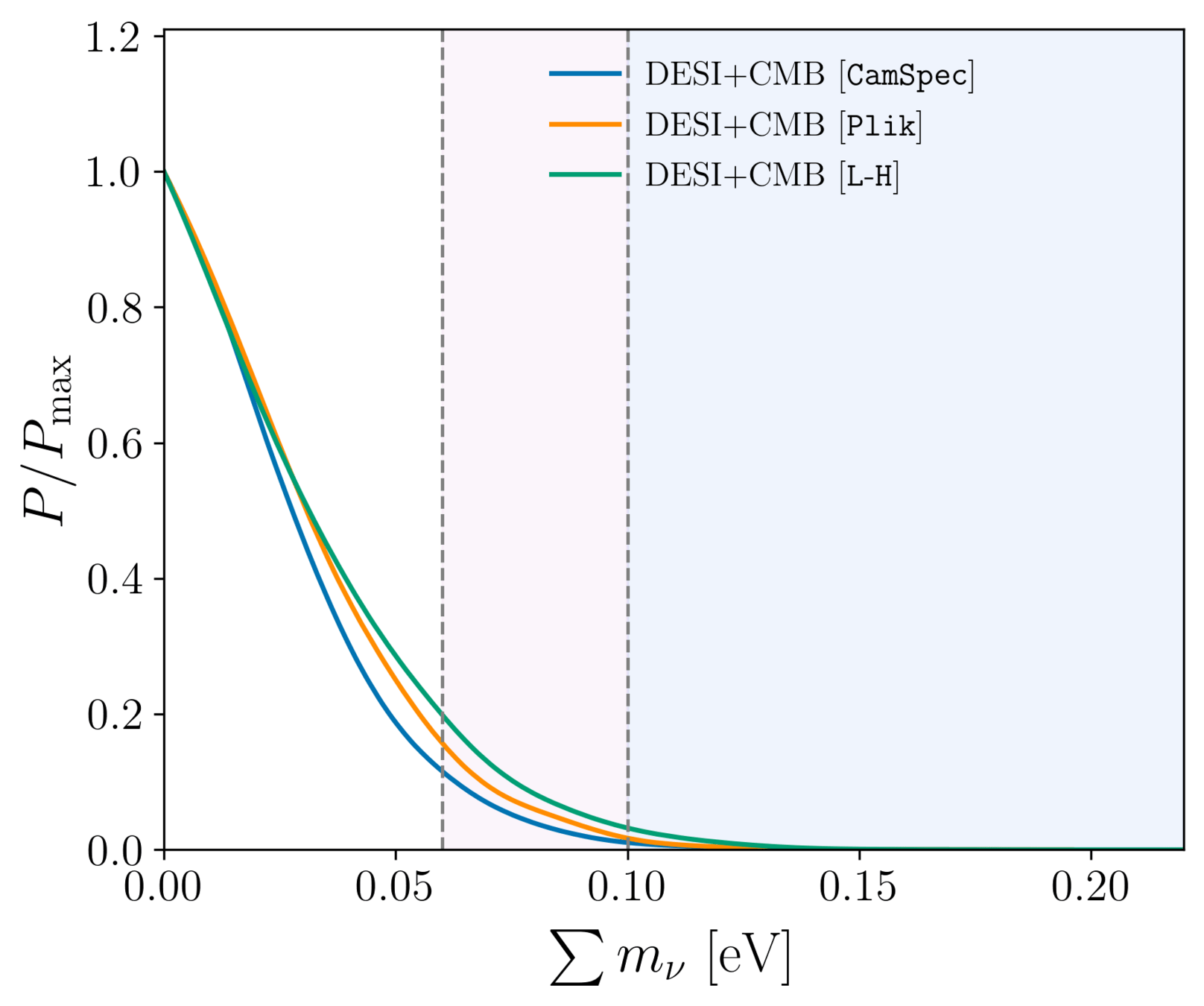
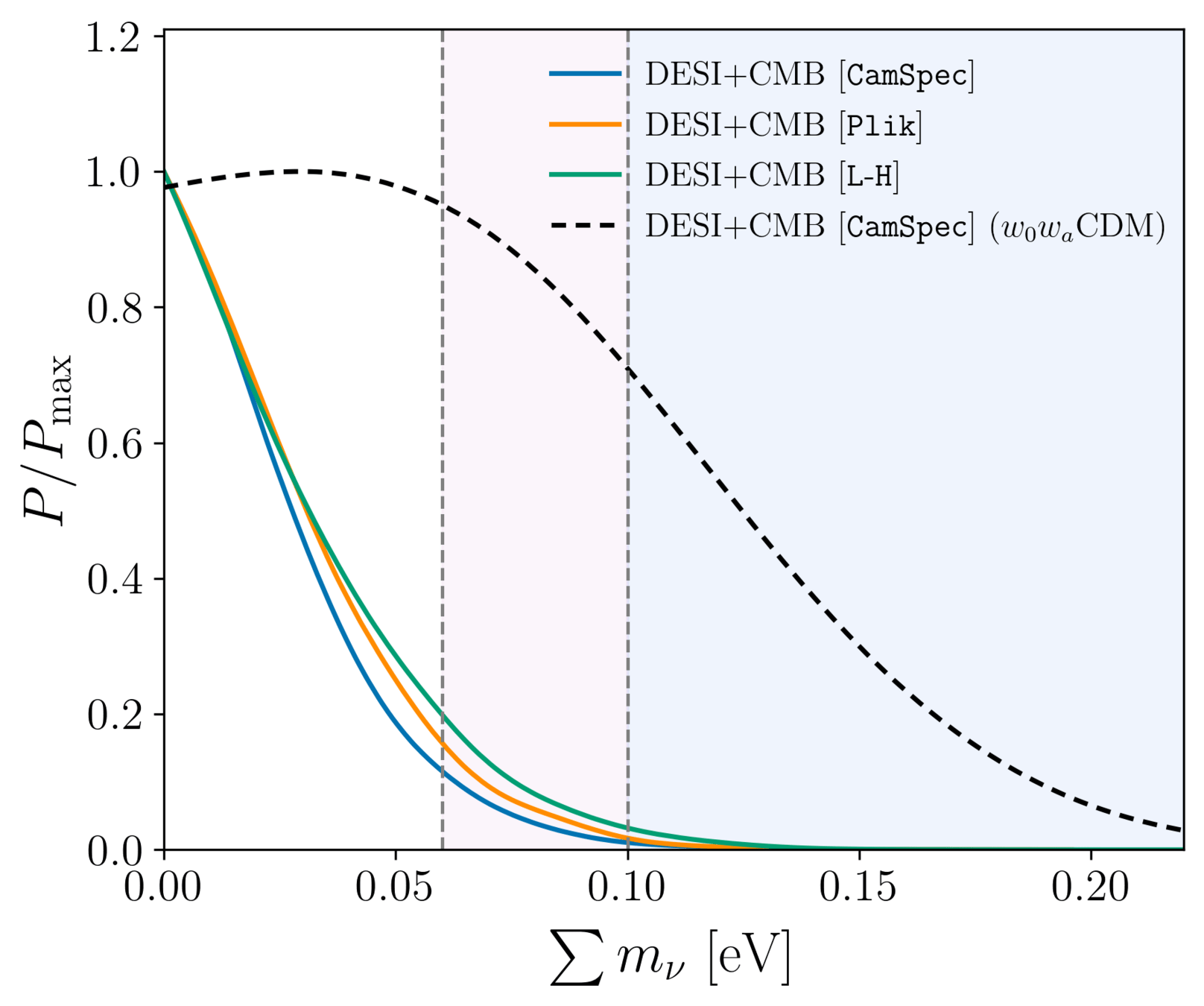
Sum of neutrino masses

Internal CMB degeneracies limiting precision on the sum of neutrino masses
Broken by BAO, which favors low \(\Omega_\mathrm{m}\)
Limit relaxed for \(w_0w_a\mathrm{CDM}\)
Summary

DESI already has the most precise BAO measurements ever (40% more precise than DR1)
DESI in mild, growing, tension with Planck \((2.3\sigma)\) and SN \((\sim 2\sigma)\) when interpreted in the ΛCDM model
Tightest upper bound on \(\sum m_\nu\), increasing tension with neutrino oscillations
Evidence for time-varying Dark Energy equation of state has increased with the DR2 BAO data by \(0.3\sigma\): CMB: \(3.1\sigma\), SN: \(2.8 - 4.2\sigma\). \(w_0w_a\mathrm{CDM}\) fixes above tensions (not \(H_0\)!).
But it's not the end yet!

In November 2024: DR1 Full-Shape results (probing the growth of structure)
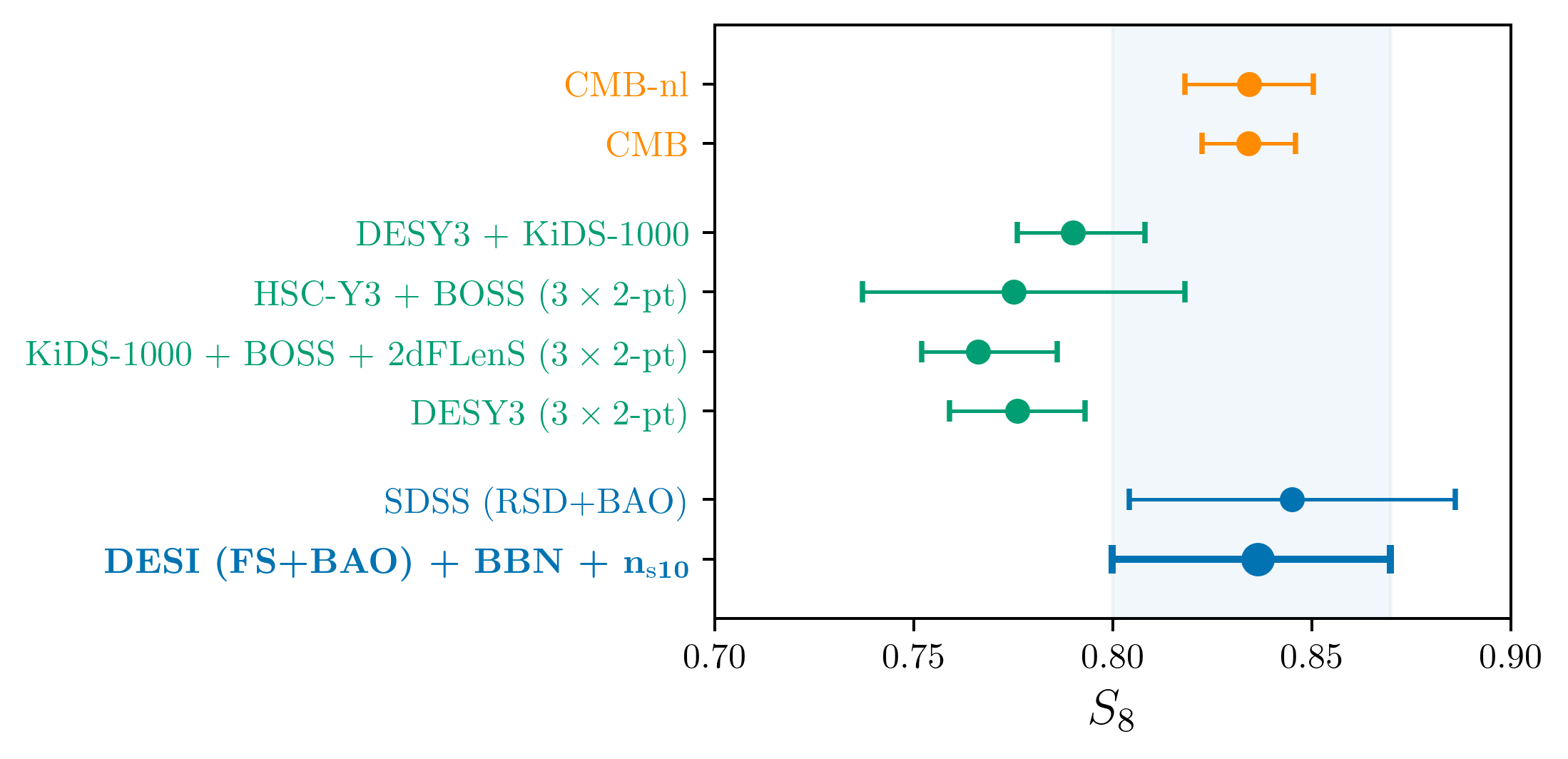
\(S_8 = \sigma_8(\Omega_\mathrm{m} / 0.3)^{0.5}\)
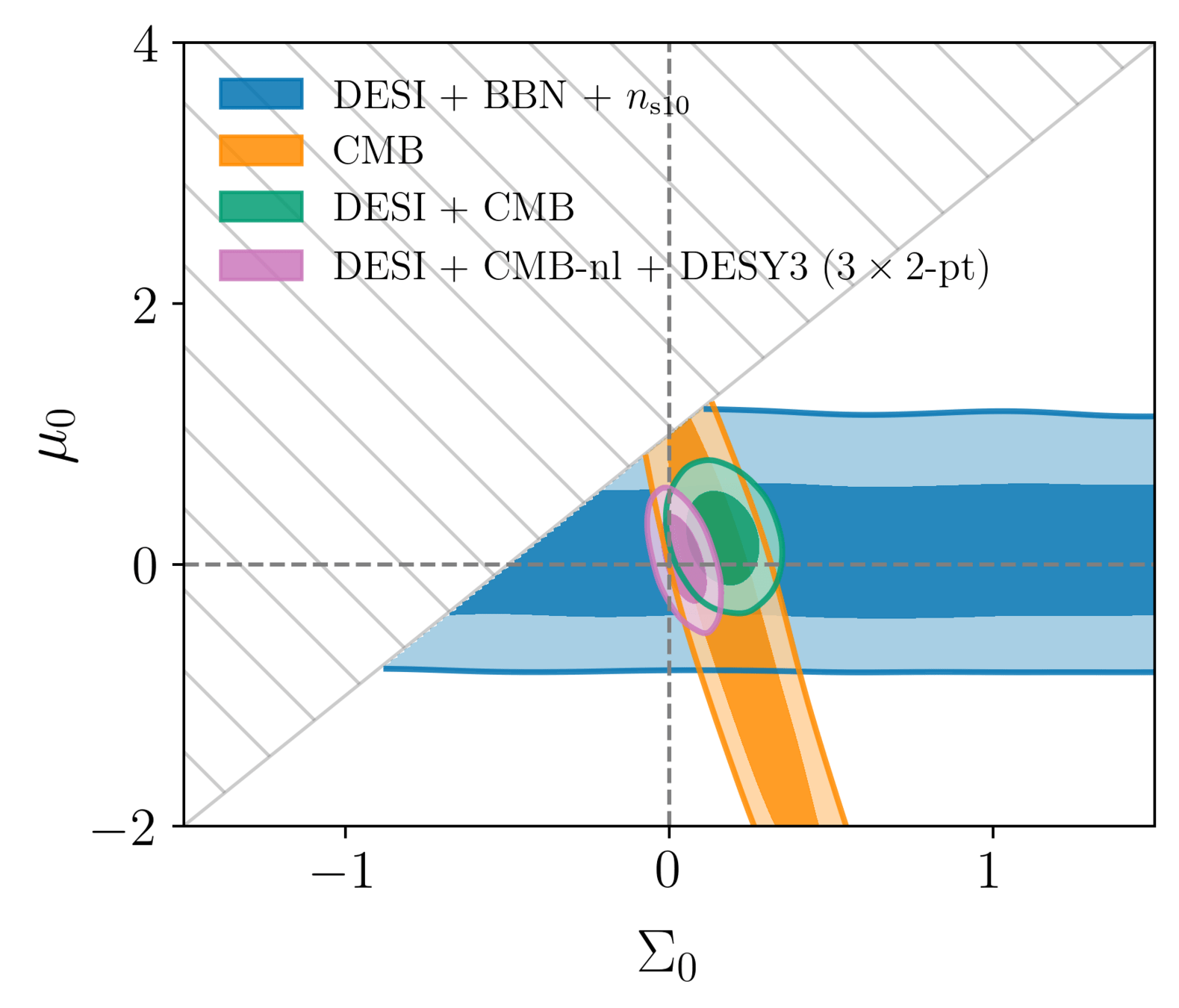
General Relativity
But it's not the end yet!

In November 2024: DR1 Full-Shape results (probing the growth of structure)
With DR2 - stay tuned!
- Full-Shape from power spectrum
- Bispectrum
- Primordial non-Gaussianity
- x CMB lensing
- Low-\(z\) growth of structure with peculiar velocities

Back-up

Full Shape measurements
clustering



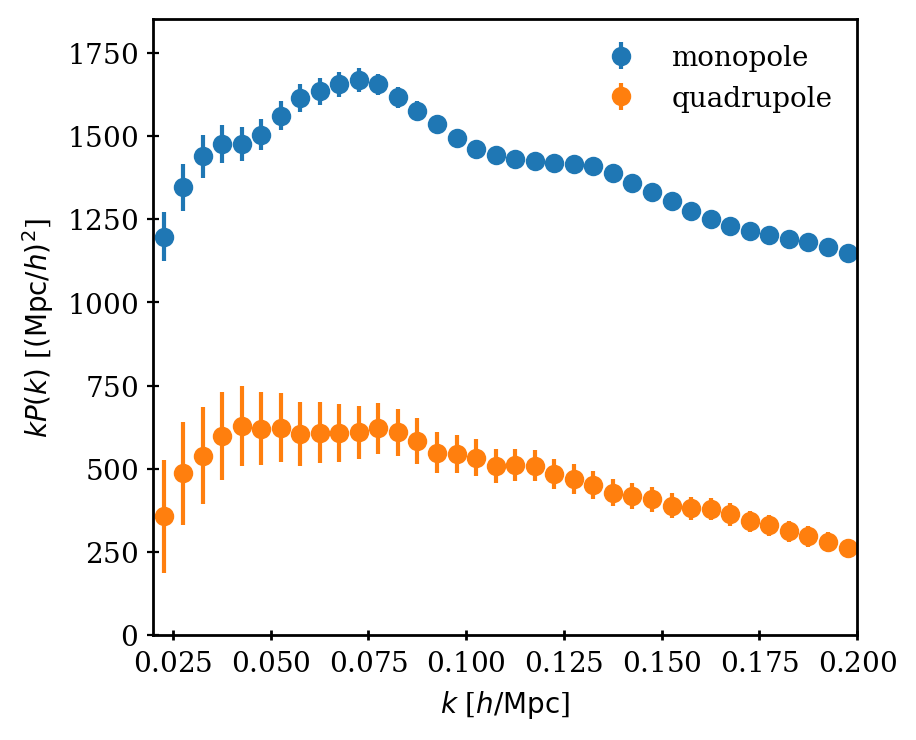
We fit the "full shape" (FS) of the galaxy power spectrum multipoles

Full Shape measurements






RSD
observed redshift = Hubble flow and peculiar velocities (RSD = "redshift space distortions")
shape
(\( \Omega_\mathrm{cdm} h^2, \Omega_\mathrm{b} h^2, n_\mathrm{s}, \sum m_\nu \))
growth of structure \(f\sigma_8\) sensitive to the theory of gravity and dark energy
We fit the "full shape" (FS) of the galaxy power spectrum multipoles

DR1 Full Shape + BAO
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
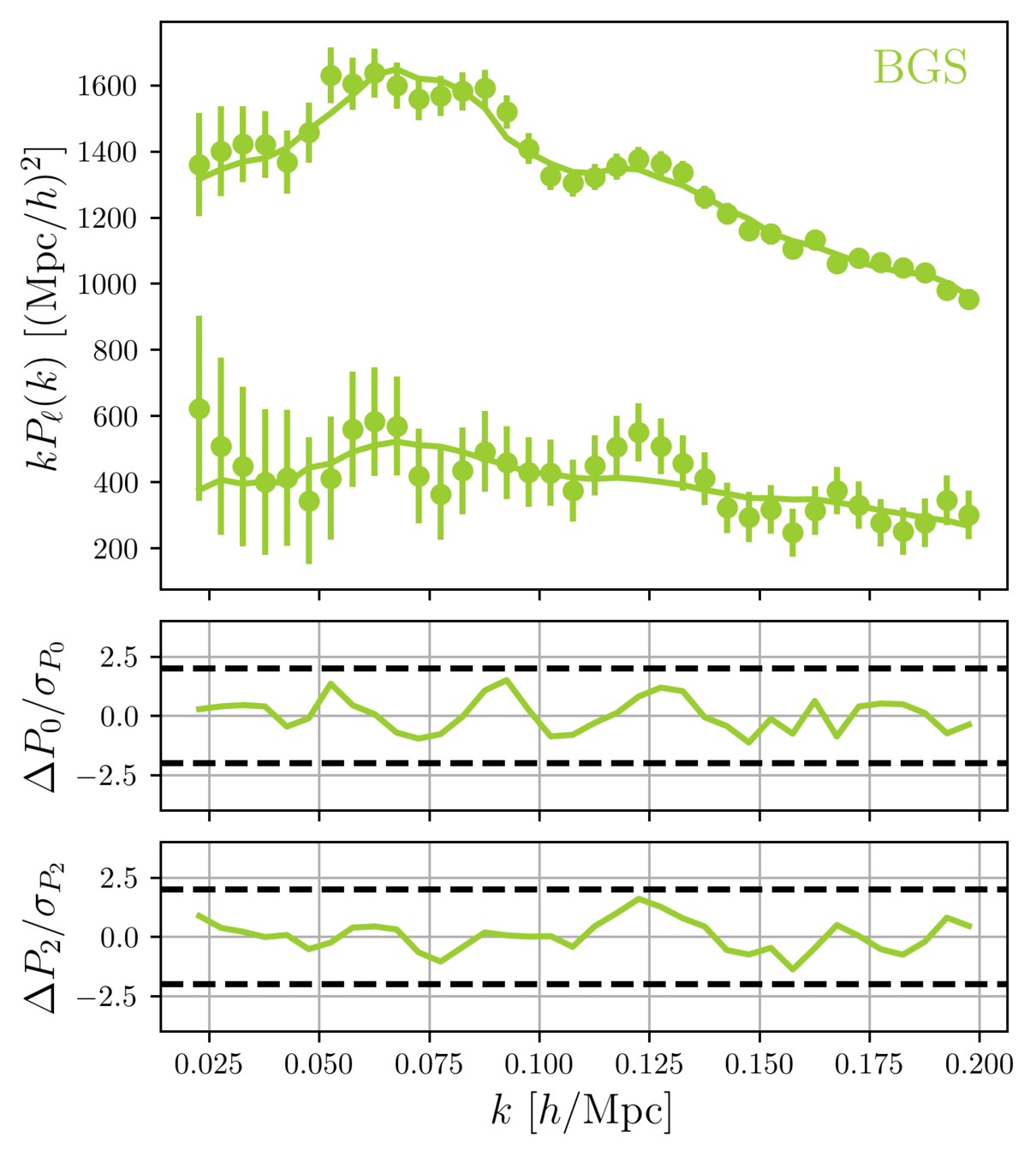
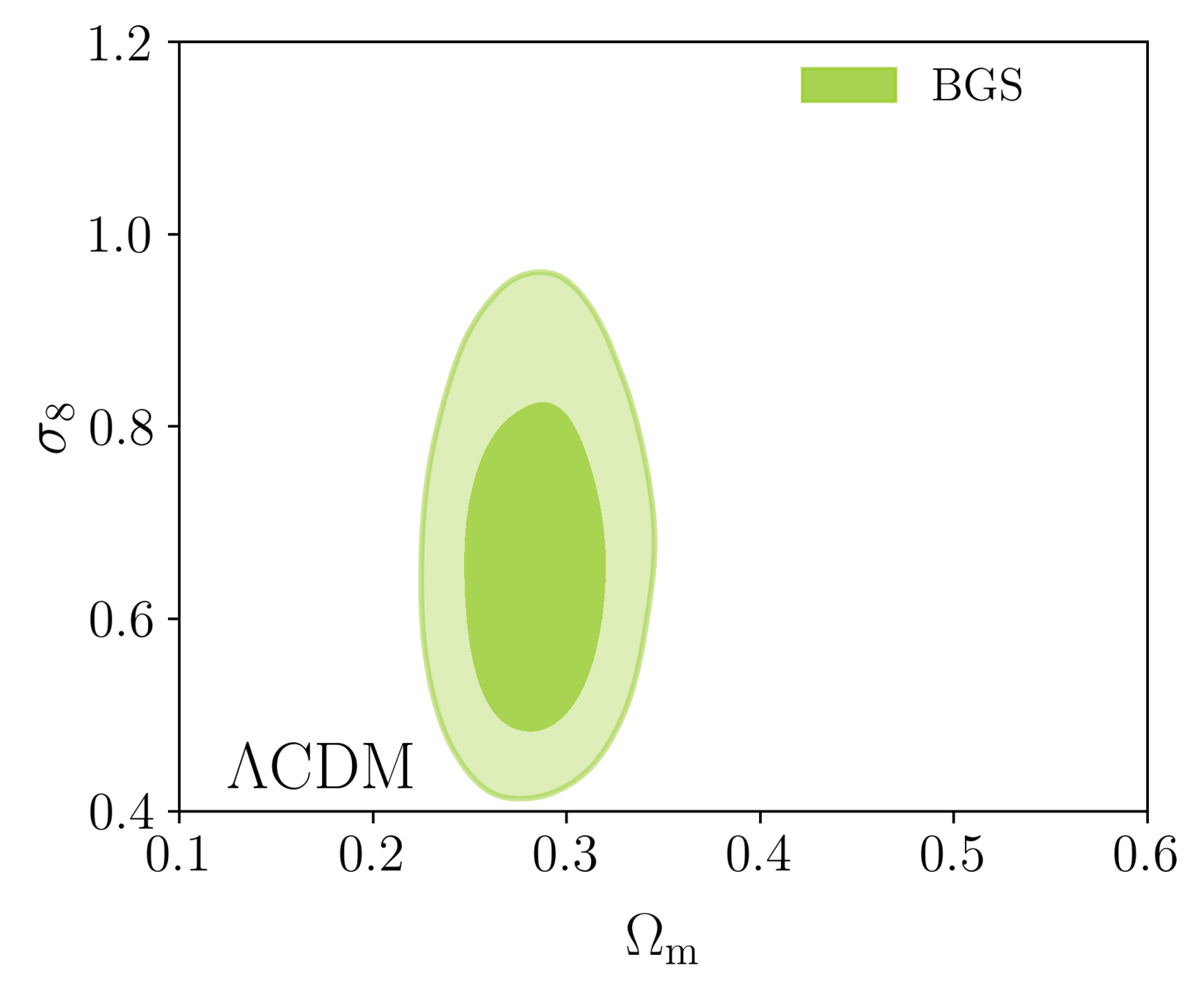


\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)



DR1 Full Shape + BAO

\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
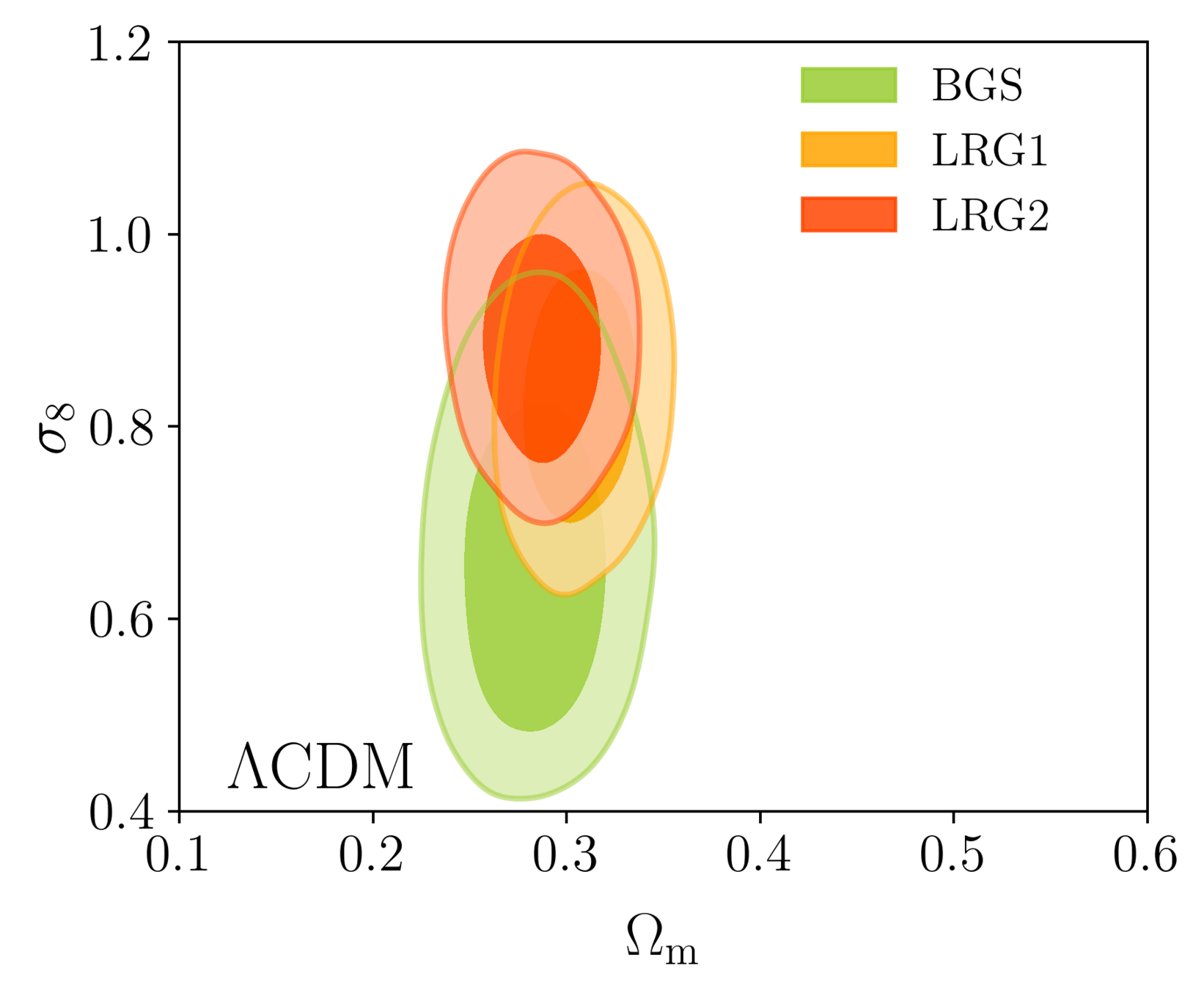

DR1 Full Shape + BAO

\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
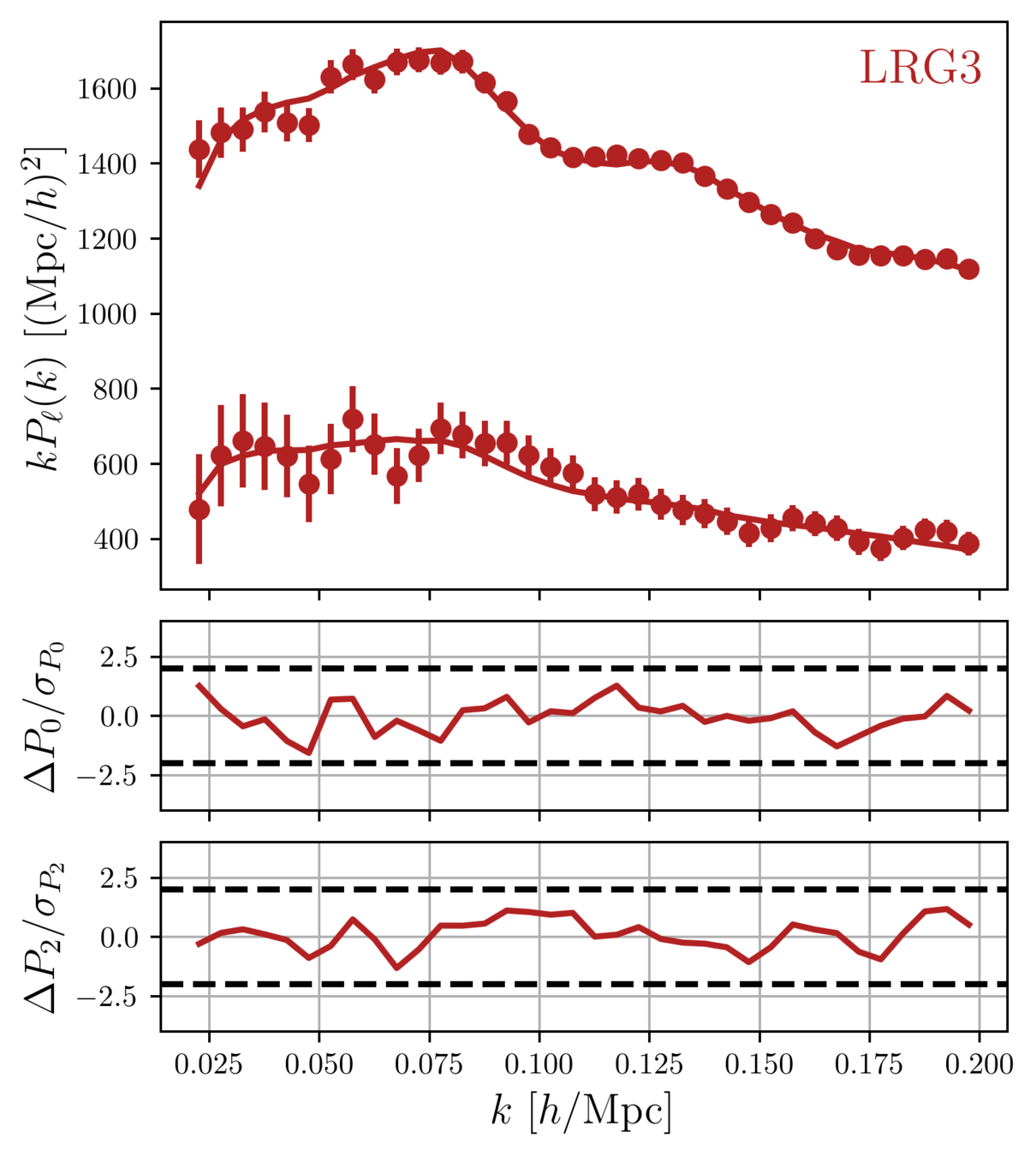

DR1 Full Shape + BAO

\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)

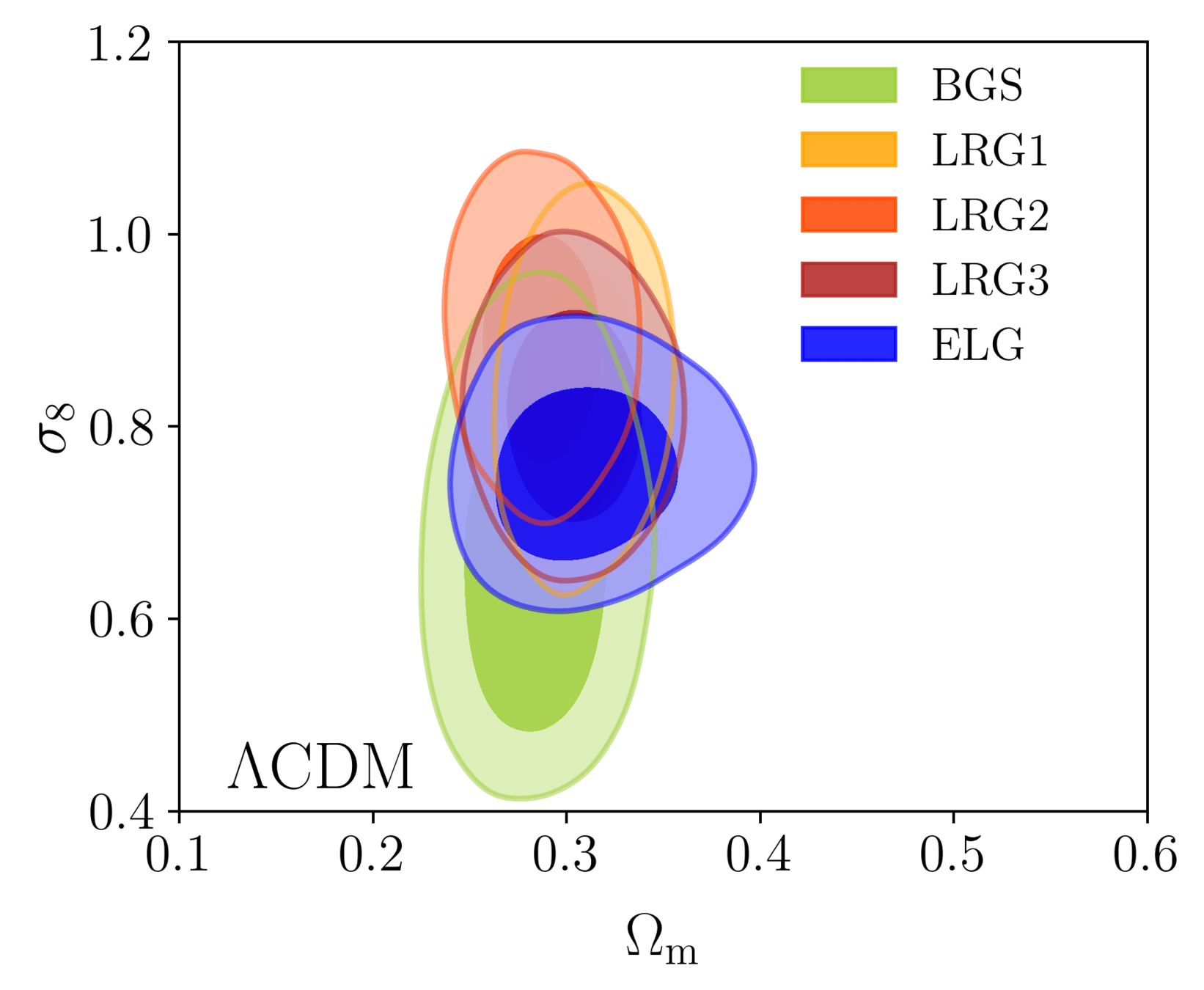
DR1 Full Shape + BAO

\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
DR1 Full Shape + BAO


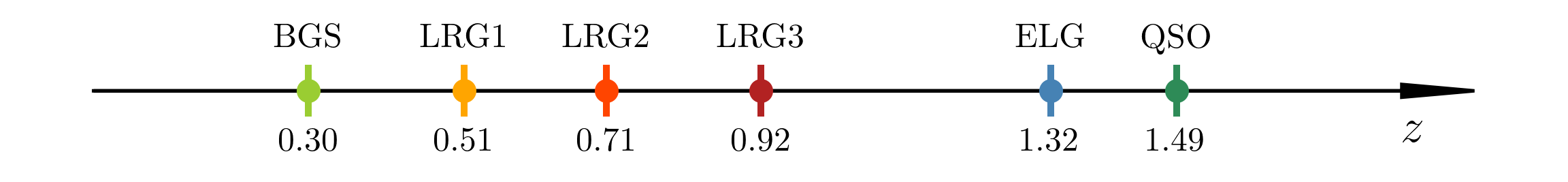
\(\omega_\mathrm{b}\): BBN, \(n_\mathrm{s} \sim \mathcal{G}(0.9649, 0.042^2)\)
DR1 Full Shape + BAO

\(S_8\) constraints

\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

\(S_8\) constraints
- Consistency with SDSS
- In agreement with CMB
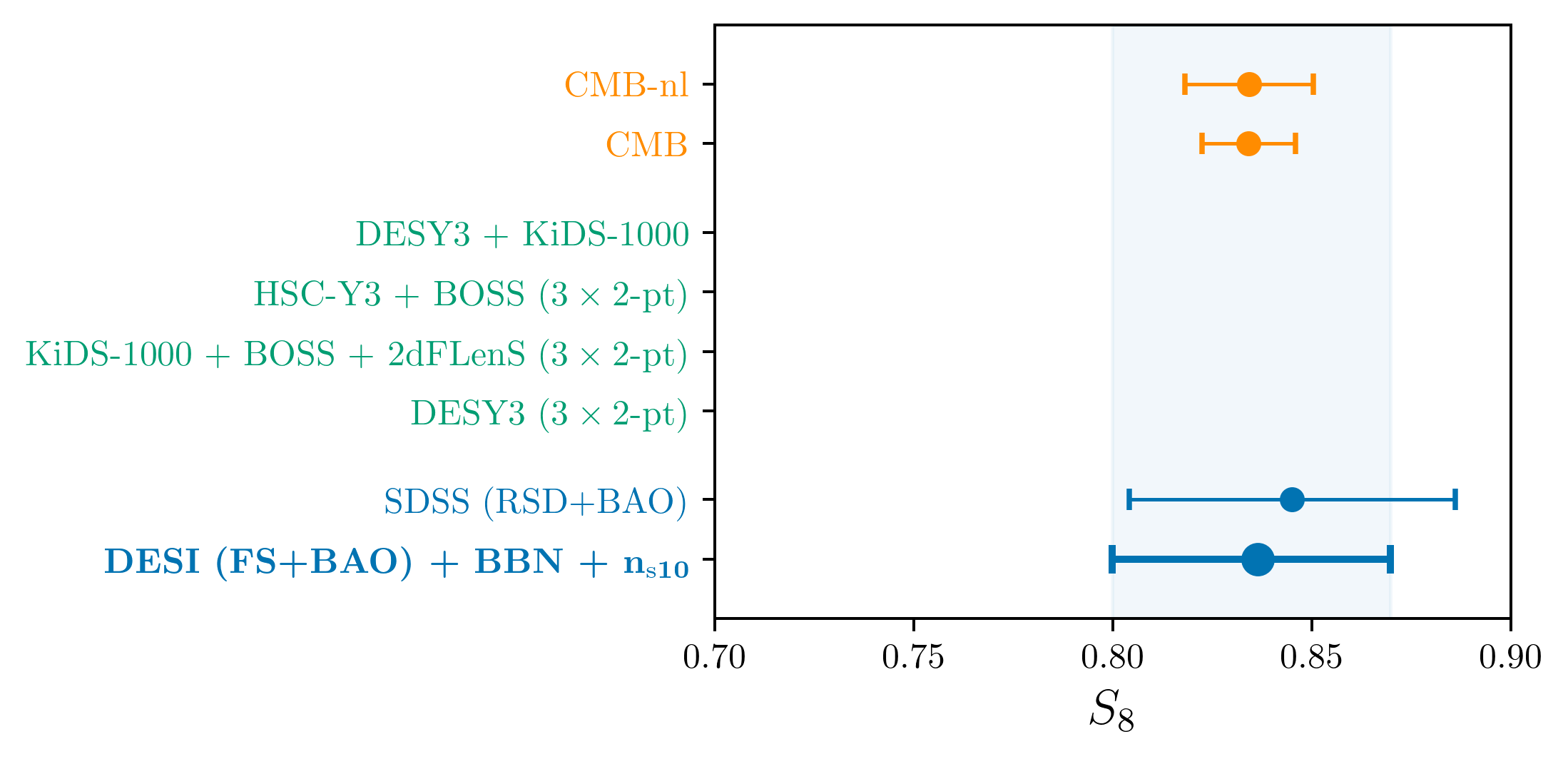
\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

\(S_8\) constraints
- Consistency with SDSS
- In agreement with CMB
- Weak lensing prefers lower \(S_8\), but still consistent

\(S_8 = \sigma_8 (\Omega_\mathrm{m} / 0.3)^{0.5}\) best constrained by weak lensing surveys

Modified gravity constraints
Perturbed FLRW metric
\(ds^2=a(\tau)^2[-(1+2\orange{\Psi})d\tau^2+(1-2\orange{\Phi})\delta_{ij}dx^i dx^j]\)
At late times:
(mass) \(k^2\orange{\Psi} = -4\pi G a^2 \green{\mu(a,k)} \blue{\sum_i\rho_i\Delta_i}\)
(light) \(k^2(\orange{\Phi} + \orange{\Psi})=-8\pi G a^2 \green{\Sigma(a,k)} \blue{\sum_i\rho_i\Delta_i}\)
gravitational potentials
density perturbations

Modified gravity constraints

DESI constrains
GR

Modified gravity constraints
\(\Sigma_0\) constrained by
- CMB (ISW and lensing)
- galaxy lensing
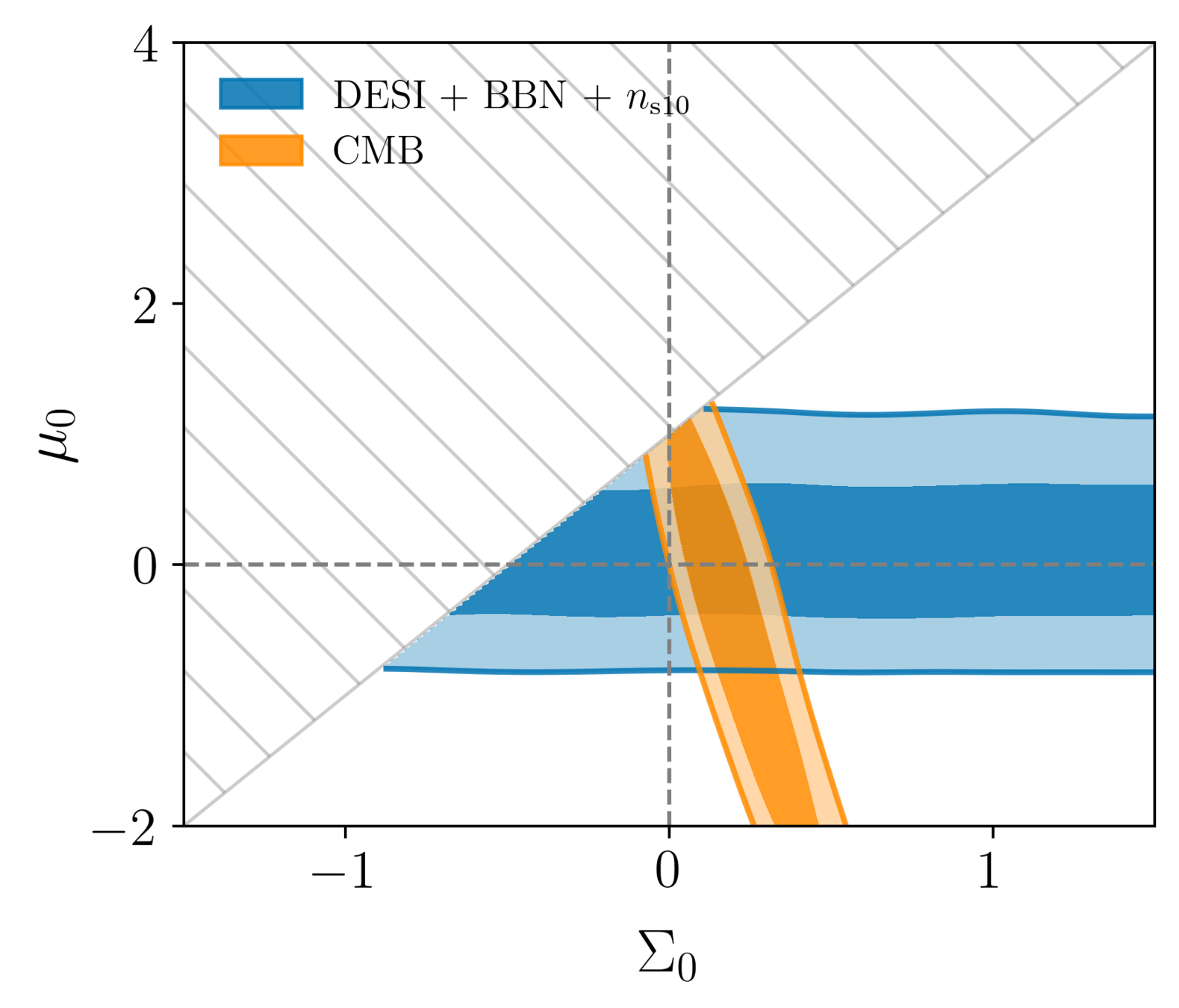
DESI constrains

Modified gravity constraints
\(\Sigma_0\) constrained by
- CMB (ISW and lensing)
- galaxy lensing

compared to CMB-nl + DESY3 (3x2pt) only: \(\sigma(\mu_0) / 2.5\), \(\sigma(\Sigma_0) / 2\)
DESI constrains
Other datasets

- SDSS BAO (for comparisons only): eBOSS Collaboration, 2020
- Primary CMB: CamSpec PR4, HiLLiPoP/LoLLiPoP, Planck Collaboration, 2018
- CMB lensing: Planck PR4 + ACT DR6 lensing ACT Collaboration, 2023, Carron, Mirmelstein, Lewis, 2022
- BBN: Schöneberg et al., 2024
- SN: Pantheon+ Brout, Scolnic, Popovic et al., 2022, Union3 Rubin, Aldering, Betoule et al. 2023, DES-SN5YR DES Collaboration

Analysis pipeline mostly the same as DR1
Again, blind analysis to mitigate observer / confirmation biases (catalog-level blinding)
Anisotropic BAO measurements for QSO (and low-\(z\) ELG)
Minor updates:
- revised min fitting range (\(60 < s / [\mathrm{Mpc}/h] < 150\))
- revised systematic budget (theory, fiducial cosmology, HOD): \(\sigma_\mathrm{syst} / \sigma_\mathrm{stat} < 9\%\)
Many more robustness tests
What's new in the BAO analysis?

BAO reconstruction
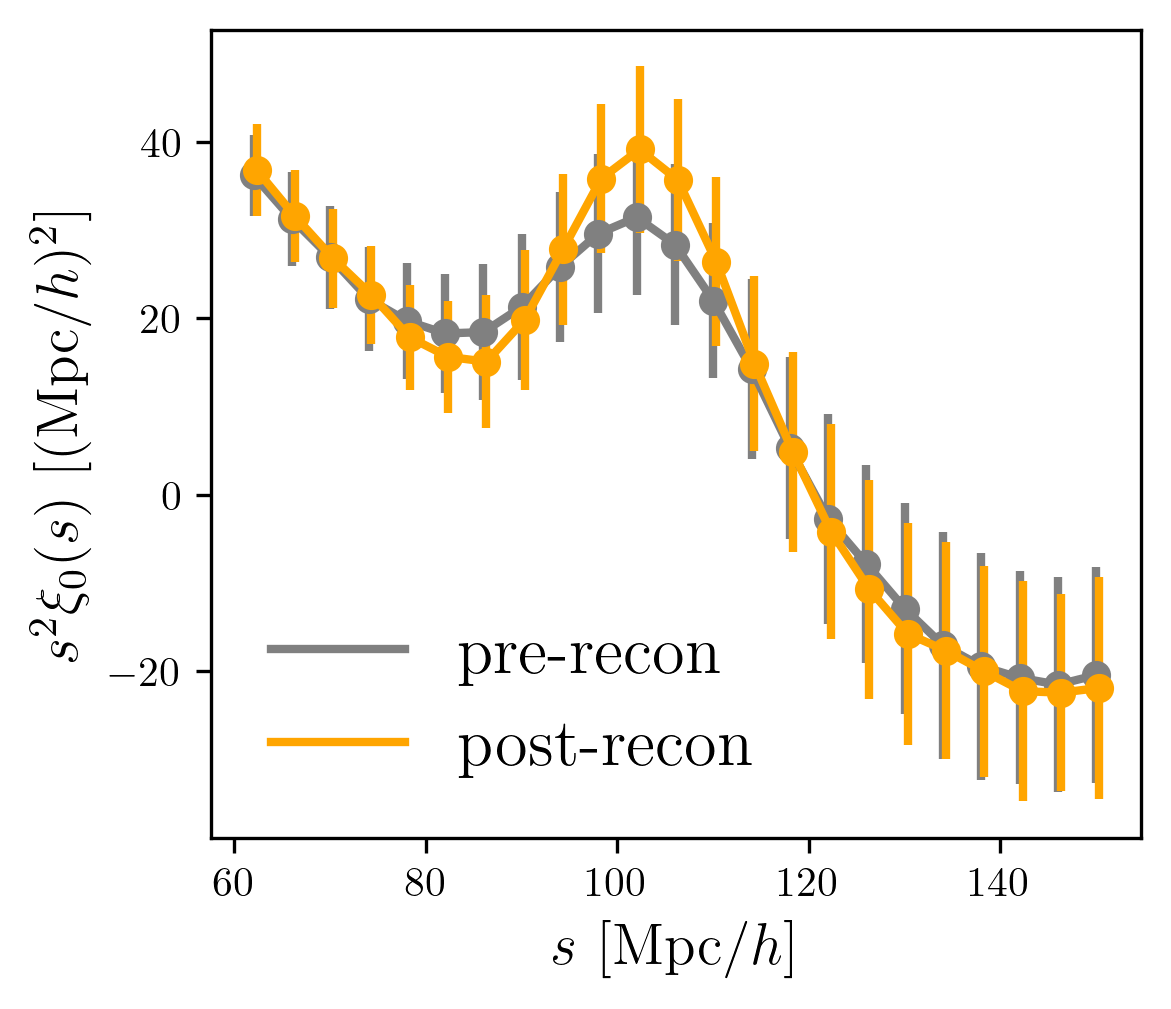
- Non-linear structure growth and peculiar velocities smear (and slightly displace) the BAO peak
- Reconstruction: estimate Zeldovich displacements from observed field and move galaxies back \(\rightarrow\) refurbishes the ruler (improves precision and accuracy)

Analysis pipeline mostly the same as DR1
Again, blind analysis to mitigate observer / confirmation biases (data vector-level blinding)
Improved modelling of metals and continuum-fitting distortions

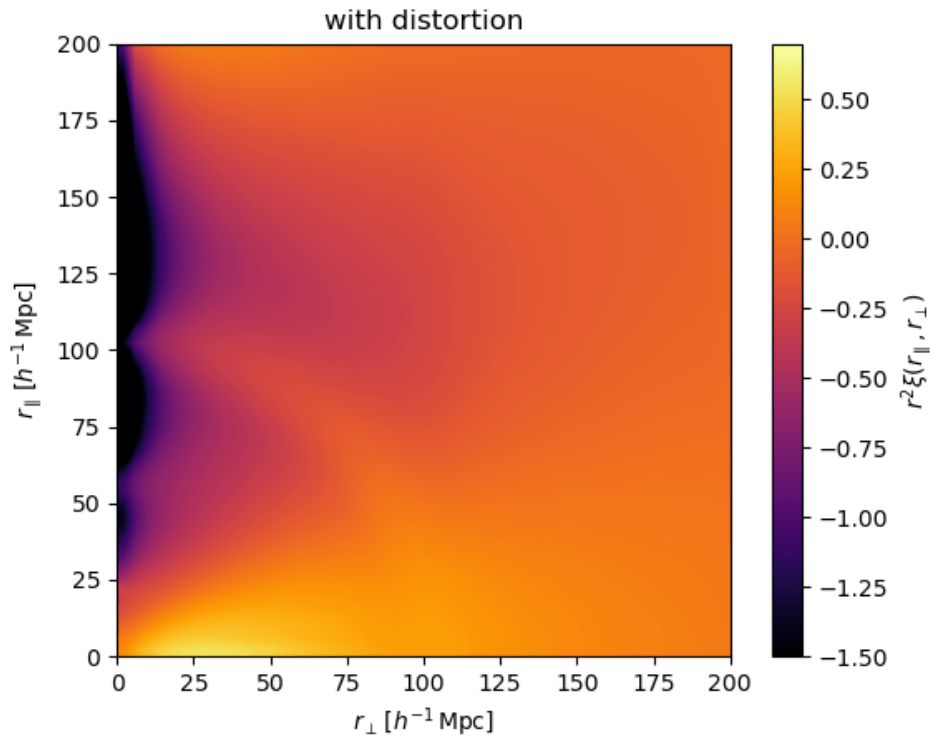
What's new in the Ly\(\alpha\) analysis?

Analysis pipeline mostly the same as DR1
Again, blind analysis to mitigate observer / confirmation biases (data vector-level blinding)
Improved modelling of metals and continuum-fitting distortions
New catalog of Damped Lyman-\(\alpha\) systems (masked)
Improved mocks and associated studies
Revised fitting range and priors on nuisance parameters
Include a small (0.3%) theory systematic uncertainty for non-linear BAO shift \(\sigma_\mathrm{syst} / \sigma_\mathrm{stat} \simeq 40 \%\)
What's new in the Ly\(\alpha\) analysis?

Robustness tests

Robust to various Planck likelihoods:
- CamSpec (baseline)
- Plick (PR3)
- LiLLiPoP-LolliPoP (PR4)

\(\Lambda\mathrm{CDM}\) constraints
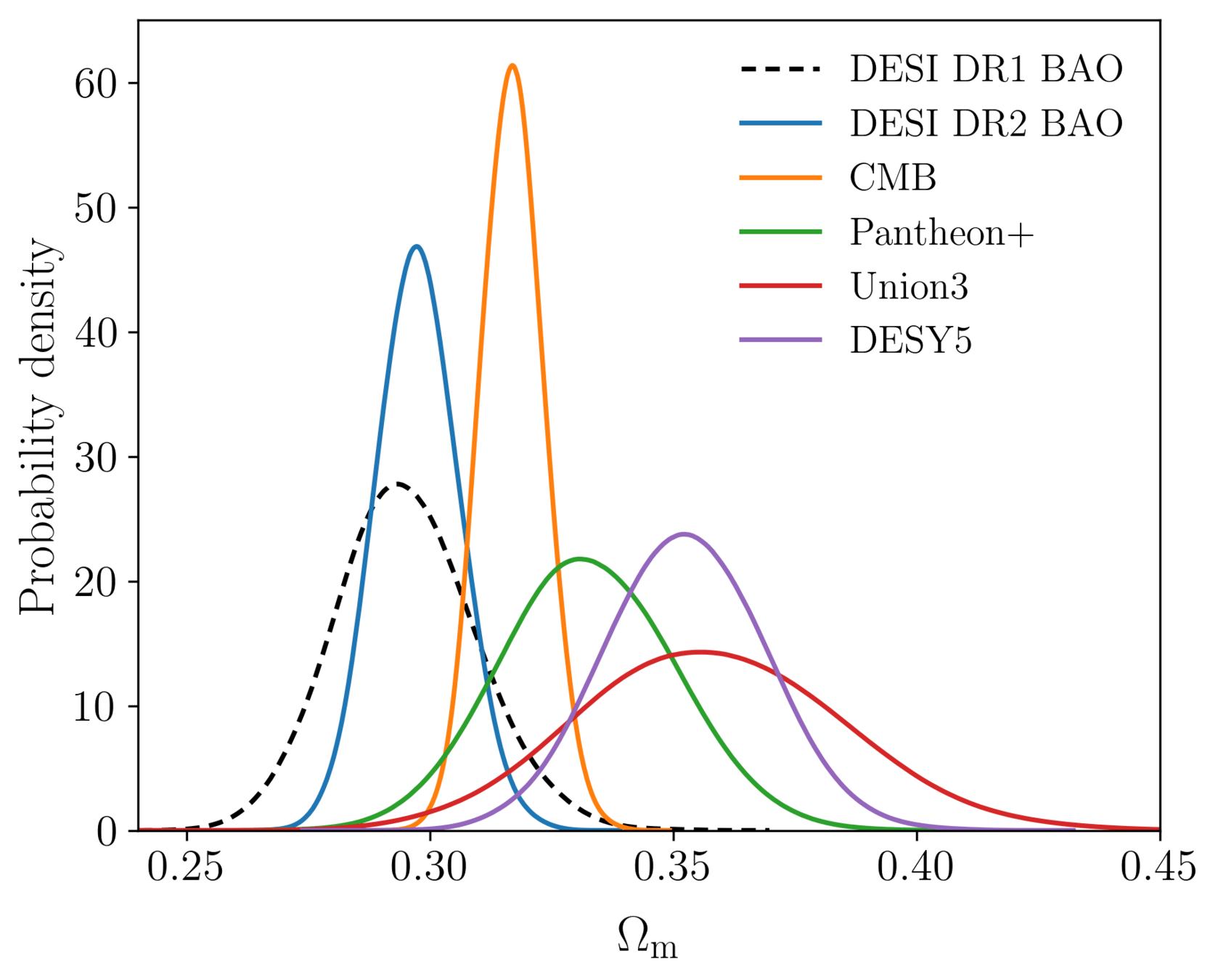
- DESI \(\Omega_\mathrm{m}\) lower than the CMB (\(1.8\sigma\))
-
DESI \(\Omega_\mathrm{m}\) lower than SN:
- Pantheon+: \(1.7\sigma\)
- Union3: \(2.1\sigma\)
- DESY5: \(2.9\sigma\)

Understanding tensions
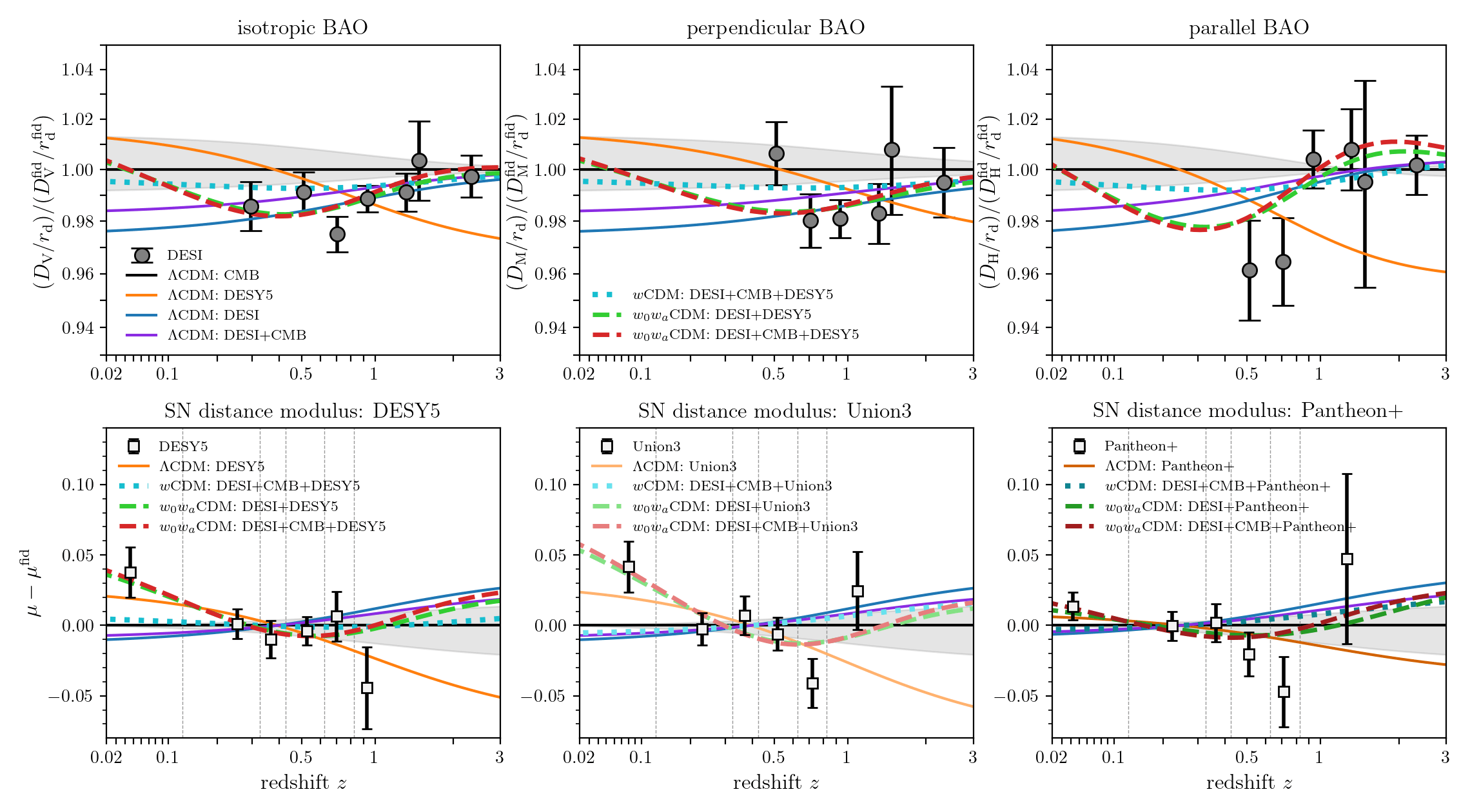

Spectroscopic redshift surveys
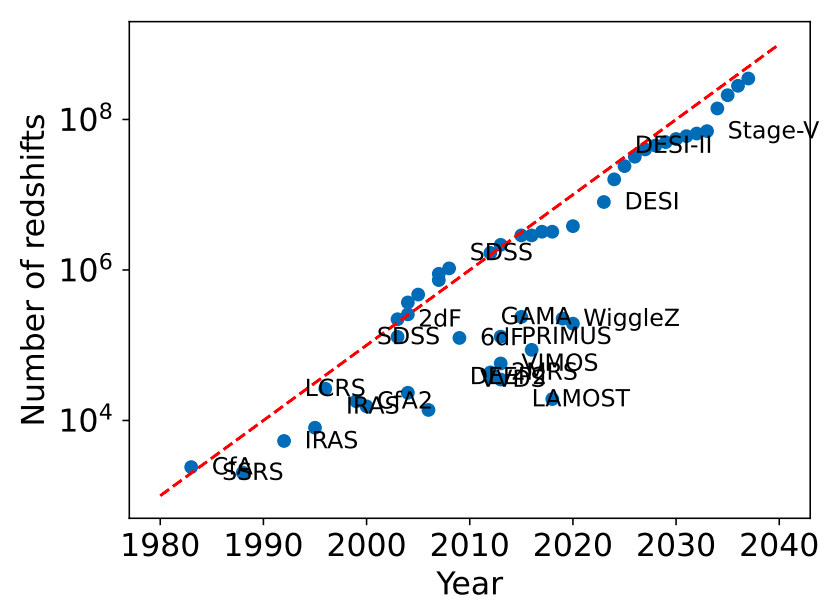
10 years = \(10 \times \)

DESI data release 1 (DR1)

Observations from May 14th 2021 to June 12th 2022

DESI data release 2 (DR2)
| asgn. comp. DR1 | # good z DR1 |
asgn. comp. DR2 | z. comp DR2 |
# of good z DR2 | |
| BGS | 64% | 0.3M | 76% | 99% | 1.2M |
| LRG | 69% | 2.1M | 83% | 99% | 4.5M |
| ELG | 35% | 2.4M | 54% | 74% | 6.5M |
| QSO | 87% | 1.2M | 94% | 68% | 2M |
more observations

Ly\(\alpha\) forest
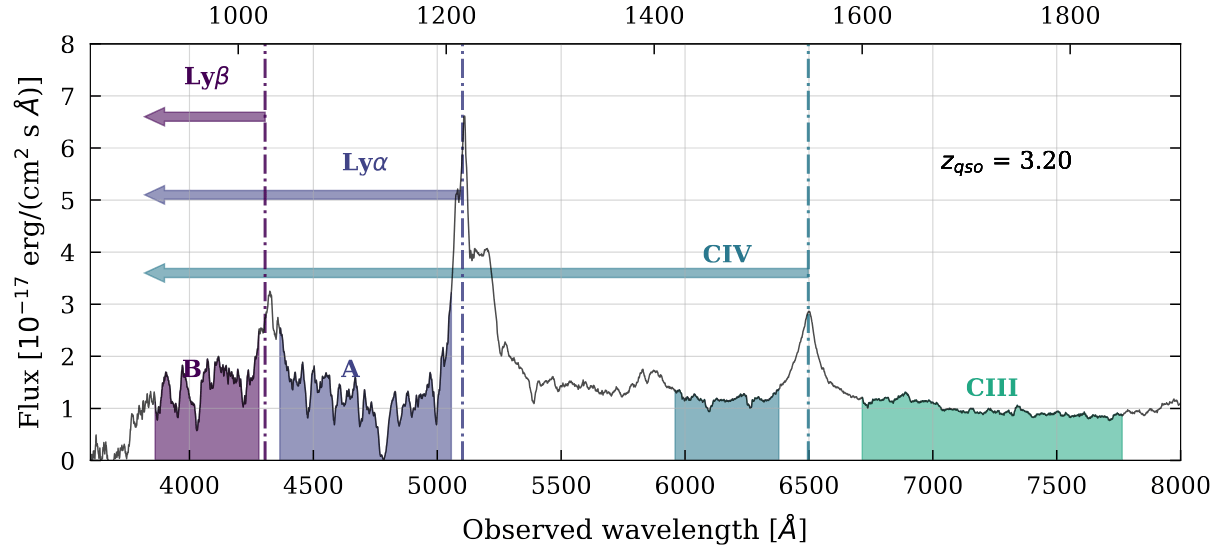

- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)


BAO measurements

transverse comoving distance
sound horizon \(r_d\)

- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)
BAO measurements



Hubble distance
sound horizon \(r_d\)

- transverse to the line-of-sight: \(D_\mathrm{M}(z) / r_\mathrm{d}\)
- along the line-of-sight: \(D_\mathrm{H}(z) / r_\mathrm{d} = c / (H(z) r_\mathrm{d}) \)
At multiple redshifts \(z\)
BAO measurements







Probes the expansion history, hence the energy content (DE)
Absolute size at \(z = 0\): \(H_0 r_d\)

Binned dark energy

- Binned reconstruction of \(w(z)\)
without assuming a functional form for the EoS - \(\simeq 4\sigma\) preference for \(w > -1\) in the first redshift bin
- Consistent with \(w_0, w_a\) parameterization
Pre/post DESI

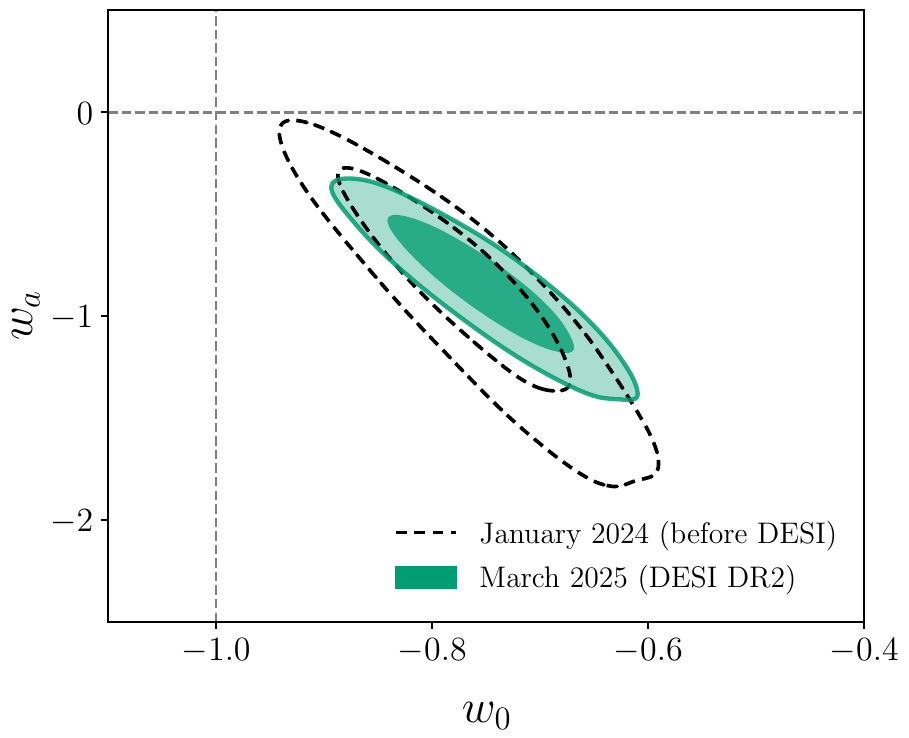
DESI vs BOSS/eBOSS

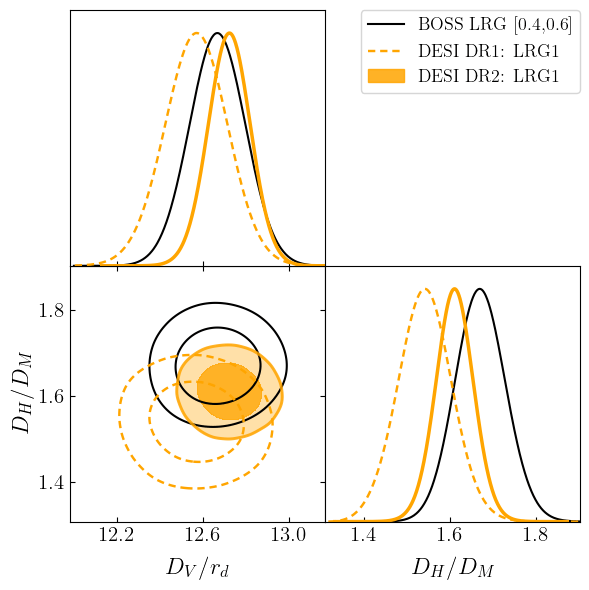
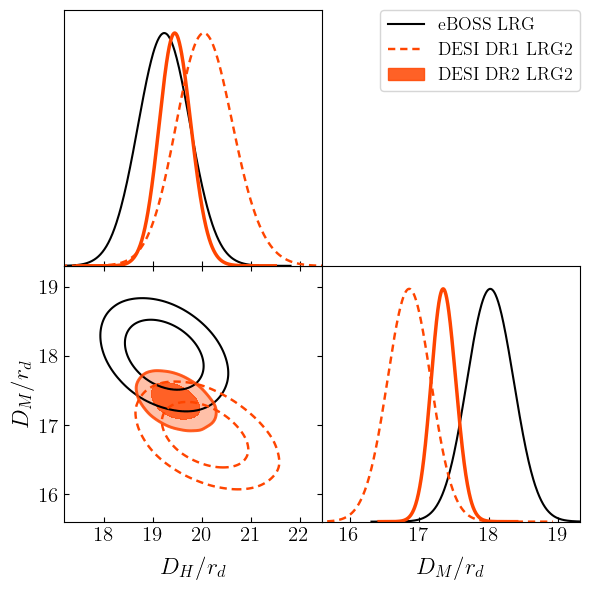
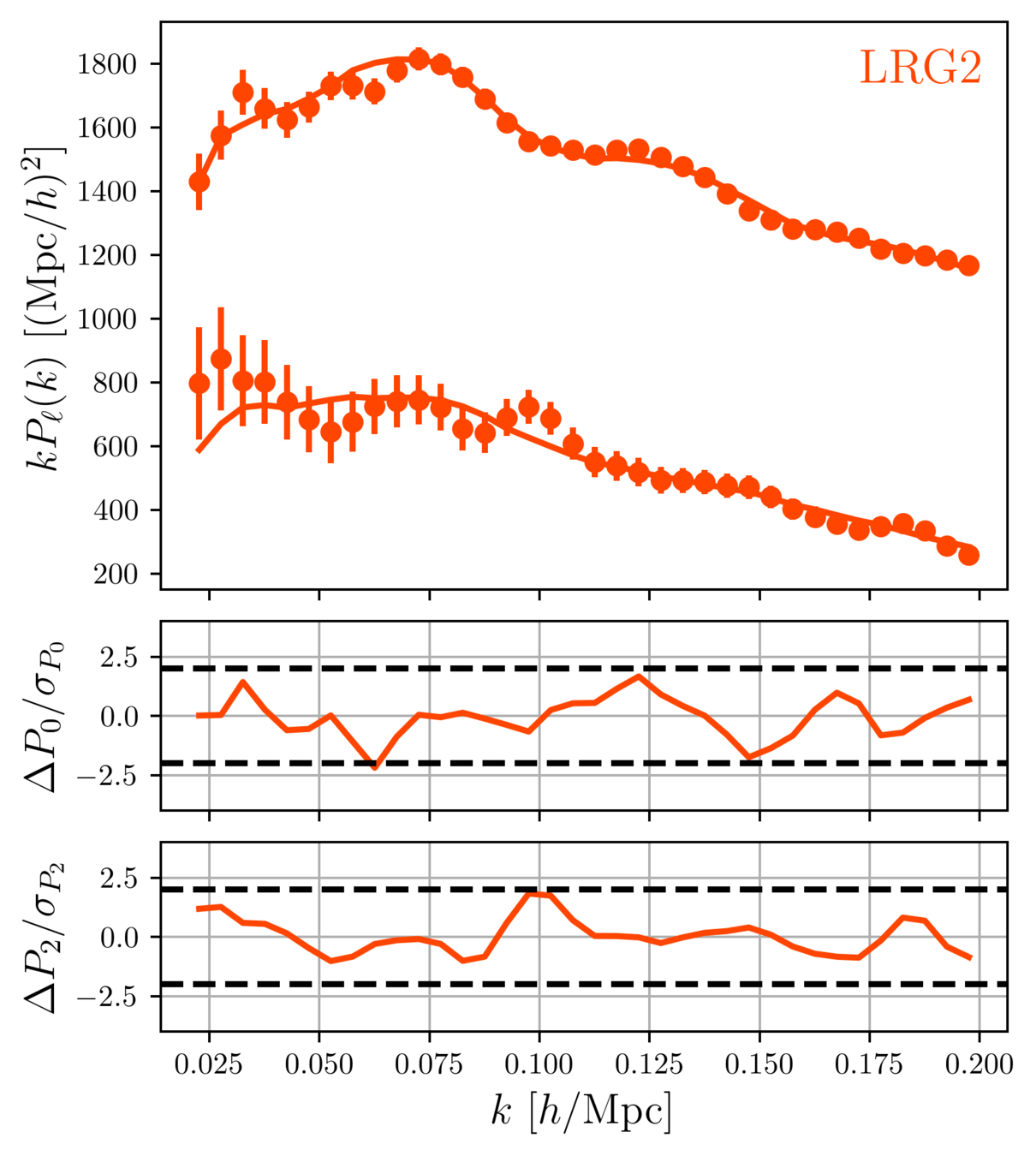
LRG2 (worst case)
\(2.8\sigma \, (\mathrm{DR1}) \Rightarrow 2.3\sigma \, (\mathrm{DR2})\)

Dark energy
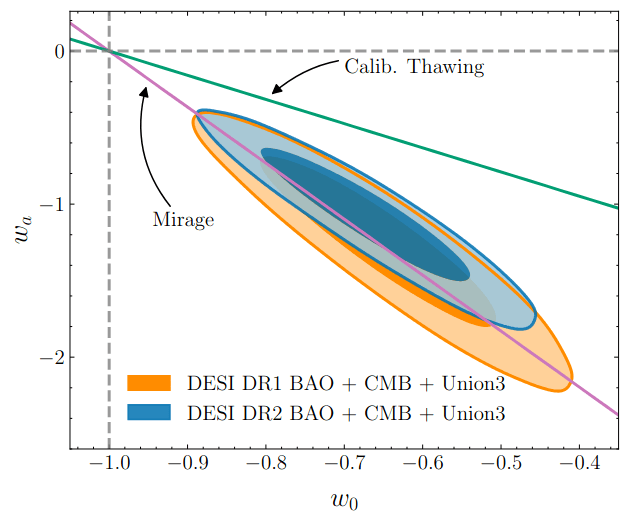
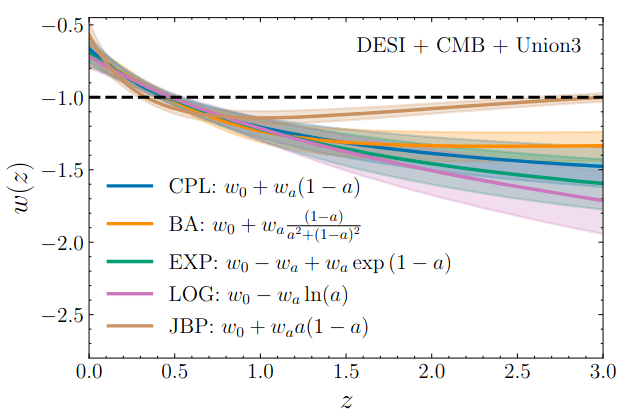
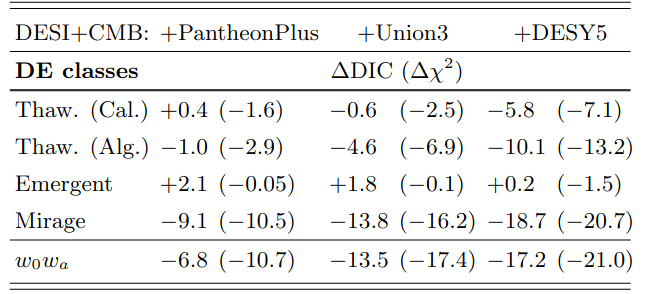
DE parameters

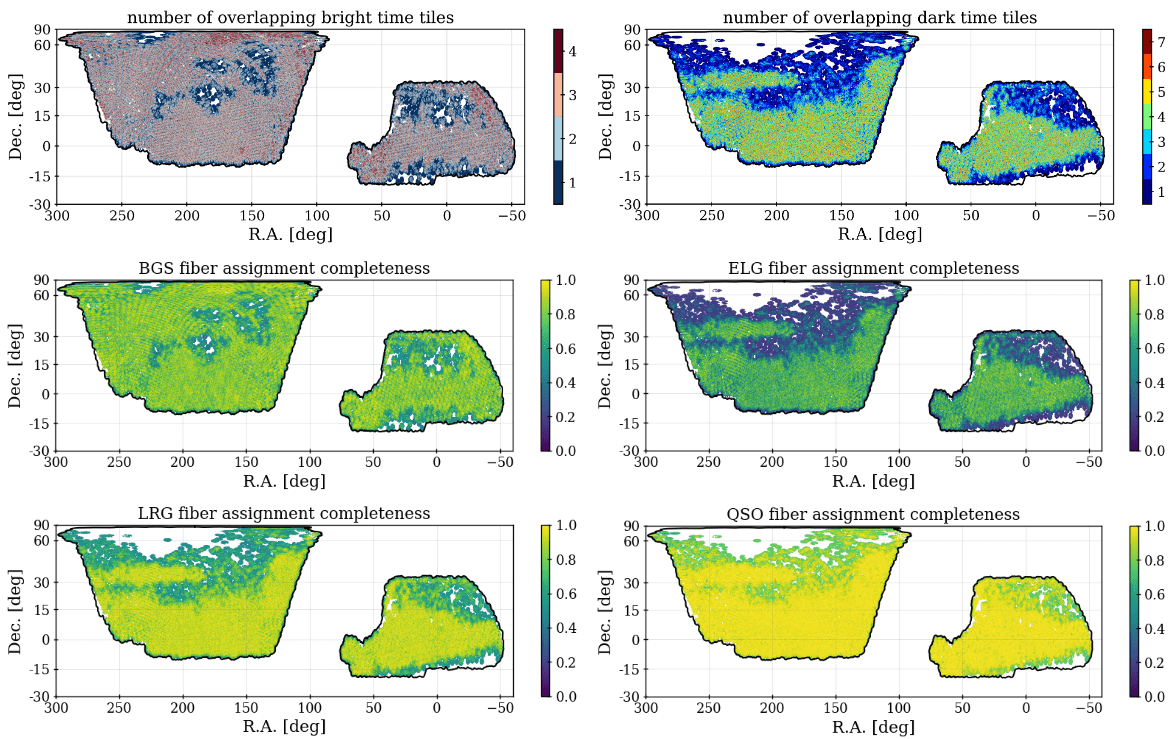
DR2 footprint

BAO_March2025
By Arnaud De Mattia
BAO_March2025
- 57



