Saverio Bolognani
Senior Scientist at ETH Zurich
Control problems in
low-inertia power systems
Scenario
-
Fewer synchronous generators to provide
- "electro-mechanical" inertia
- real-time power balancing
-
More generation from renewable sources
- fluctuating power generation
- reduced reliability in case of large disturbances
-
More power converters
- higher flexibility
- hard operational constraints
Typical frequency response analysis
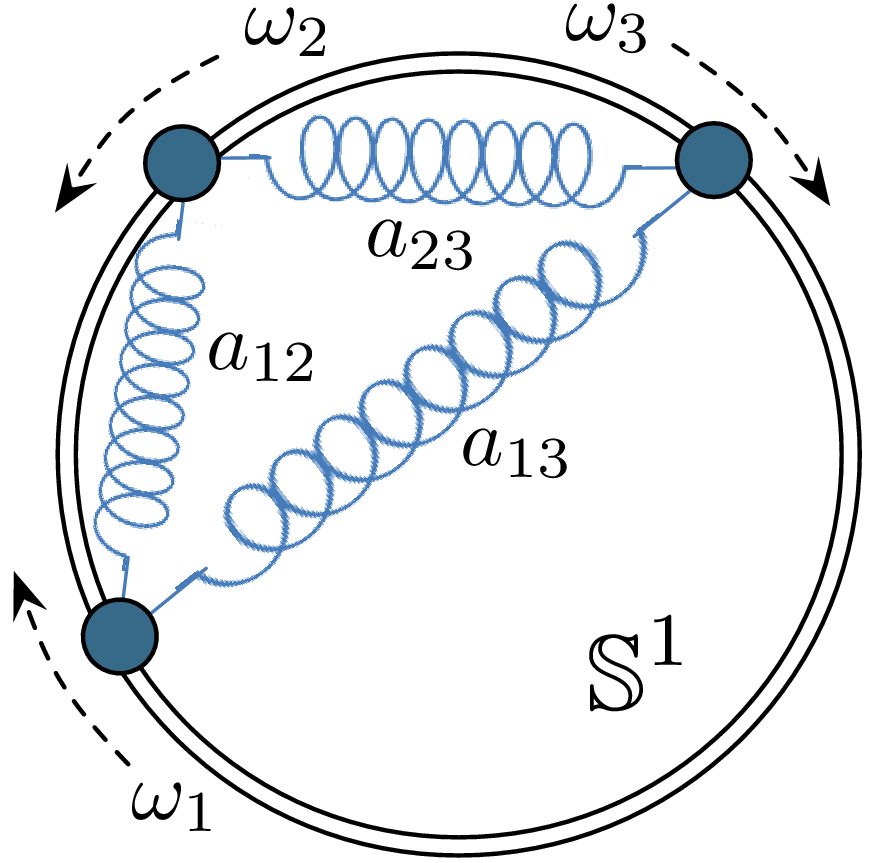
Based on electro-mechanical synchronous generators
-
Definition of angles / frequency
- Rotor angle / speed
-
Specifications
- Generators' frequency / ROCOF limits
-
Dynamical models
- 2nd order Swing equations and more
Still valid?
Definition of angle / frequency
Three-phase voltages
u_a(t), u_b(t), u_c(t)
Balanced operation
u_a(t) + u_b(t) + u_c(t) = 0
Vector notation
\mathbf{u}(t) = \frac{2}{3}
\left[
u_a(t) + u_b(t) e^{j \frac{2\pi}{3}} + u_c(t) e^{j \frac{4\pi}{3}}
\right]
\mathbf{u}(t)
\in \mathbb{C}
Vector notation (rotating frame)
Synchronous power system
\theta_{dq} = \int_0^t \omega d\tau = \omega t
\mathbf{u^{dq}}(t) = \mathbf{u}(t) e^{-j \theta_{dq}}
\begin{bmatrix}
u_d \\ u_q
\end{bmatrix}
=
\begin{bmatrix}
\cos \theta_{dq} & \sin \theta_{dq} \\
- \sin \theta_{dq} & \cos \theta_{dq}
\end{bmatrix}
\begin{bmatrix}
u_\alpha \\ u_\beta
\end{bmatrix}
\mathbf{u}(t) = U e^{j (\theta_0 + \omega t)}
\mathbf{u^{dq}}(t) = U e^{j \theta_0}
Phasor notation
Time varying frequency
\mathbf{u}(t) = U(t) e^{j \theta(t)}
\theta(t) = \theta_0 + \int_0^t \omega(\tau) d\tau
- No sinusoidal assumption
- At each time, magnitude and angle are well defined
- We can use the vector notation in ODEs
- Frequency: tracking a ramp signal
Time varying frequency
u_a, u_b, u_c
T_{abc}^{dq}
\hat \theta(t)
u_d
u_q
\hat U(t)
\frac{k_1}{s}
k_2
\frac{1}{s}
\hat \omega(t)
Specifications
Frequency response to a fault
- "Features" inspired by electro-mechanical dynamics
- ROCOF (inertia)
- bounded frequency deviation (primary control)
- frequency restoration (secondary control)
Specifications
Frequency response to a fault
- "Features" inspired by electro-mechanical dynamics
- ROCOF (inertia)
- bounded frequency deviation (primary control)
- frequency restoration (secondary control)
Not very different from step response analysis
of a PID controller (and a nonlinear plant)
P
I
D
\displaystyle p_G = p_G^\text{ref} + k_D \dot \delta \omega + k_P \delta \omega + k_I \int \delta \omega
Specifications
Small-signal amplification
- Disturbance model
- Power demand of loads
- Fluctuation of renewable generation
- Amplification from disturbance to output
- Norm of frequency deviation (which norm?)
Frequency in low inertia power systems
-
Definition of angles / frequency
-
Specifications
- Response to large disturbances
- Small signal response (system norms)
-
Dynamical models
- Phasor-free models
Control problems in low-inertia power systems
By Saverio Bolognani
