Marcos de referencia y transformaciones homogéneas
BE3027 - Robótica Médica
¿Por qué?
diversos agentes ⇒ diversas perspectivas
interacción e intercambio de información
lenguaje estándar y sistemático
⇒ Álgebra lineal
diversos agentes ⇒ diversas perspectivas
interacción e intercambio de información
lenguaje estándar y sistemático
Cuerpos rígidos
rigidez ⇒d(pi,pj)=cte.
\text{rigidez } \Rightarrow d(p_i,p_j)=\mathrm{cte.}
y
y
x
x
z
z
y
y
x
x
z
z
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
=
\boldsymbol{=}
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
Marcos de referencia
marco inercial
body frame
Marcos de referencia
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
marco inercial
body frame
vectores en {B} forman un espacio vectorial
x^B,y^B,z^B base ortonormal para este espacio
Emplearemos marcos de referencia para ubicar cuerpos rígidos, describir su interacción y permitir el intercambio de información
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
p
p
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
Bp
{^B}\mathbf{p}
p
p
Mx
{^M}\mathbf{x}
nombre del vector
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
Bp
{^B}\mathbf{p}
p
p
Mx
{^M}\mathbf{x}
marco de referencia en donde se define
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
Bp
{^B}\mathbf{p}
p
p
Ip
{^I}\mathbf{p}
Bp
{^B}\mathbf{p}
Ip
{^I}\mathbf{p}
distintos espacios vectoriales
≡
\equiv
y
y
x
x
z
z
{I}
\left\{I\right\}
y^B
\hat{\mathbf{y}}_B
x^B
\hat{\mathbf{x}}_B
z^B
\hat{\mathbf{z}}_B
{B}
\left\{B\right\}
Bp
{^B}\mathbf{p}
p
p
Ip
{^I}\mathbf{p}
ITB
{^I}\mathcal{T}_B
debe existir para transformar de un espacio al otro
T
\mathcal{T}
ITB
{^I}\mathcal{T}_B
marco destino
marco fuente
cuerpos rígidos ≡ transformaciones rígidas
Ip=ITB{Bp}
{^I}\mathbf{p}={^I}\mathcal{T}_B\left\{{^B}\mathbf{p}\right\}
Ip=ITB{Bp}
{^I}\mathbf{p}={^I}\mathcal{T}_B\left\{{^B}\mathbf{p}\right\}
xR
x_R
yR
y_R
zR
z_R
{R}
\{R\}
xS
x_S
yS
y_S
zS
z_S
{S}
\{S\}
xC
x_C
yC
y_C
zC
z_C
{C}
\{C\}
xN
x_N
zN
z_N
yN
y_N
{N}
\{N\}
p
p
xR
x_R
yR
y_R
zR
z_R
{R}
\{R\}
xS
x_S
yS
y_S
zS
z_S
{S}
\{S\}
xC
x_C
yC
y_C
zC
z_C
{C}
\{C\}
xN
x_N
zN
z_N
yN
y_N
{N}
\{N\}
p
p
Cp
{^C}\mathbf{p}
Sp
{^S}\mathbf{p}
xR
x_R
yR
y_R
zR
z_R
{R}
\{R\}
xS
x_S
yS
y_S
zS
z_S
{S}
\{S\}
xC
x_C
yC
y_C
zC
z_C
{C}
\{C\}
xN
x_N
zN
z_N
yN
y_N
{N}
\{N\}
p
p
Cp
{^C}\mathbf{p}
Sp
{^S}\mathbf{p}
RTS
{^R}\mathcal{T}_S
NTS
{^N}\mathcal{T}_S
NTC
{^N}\mathcal{T}_C
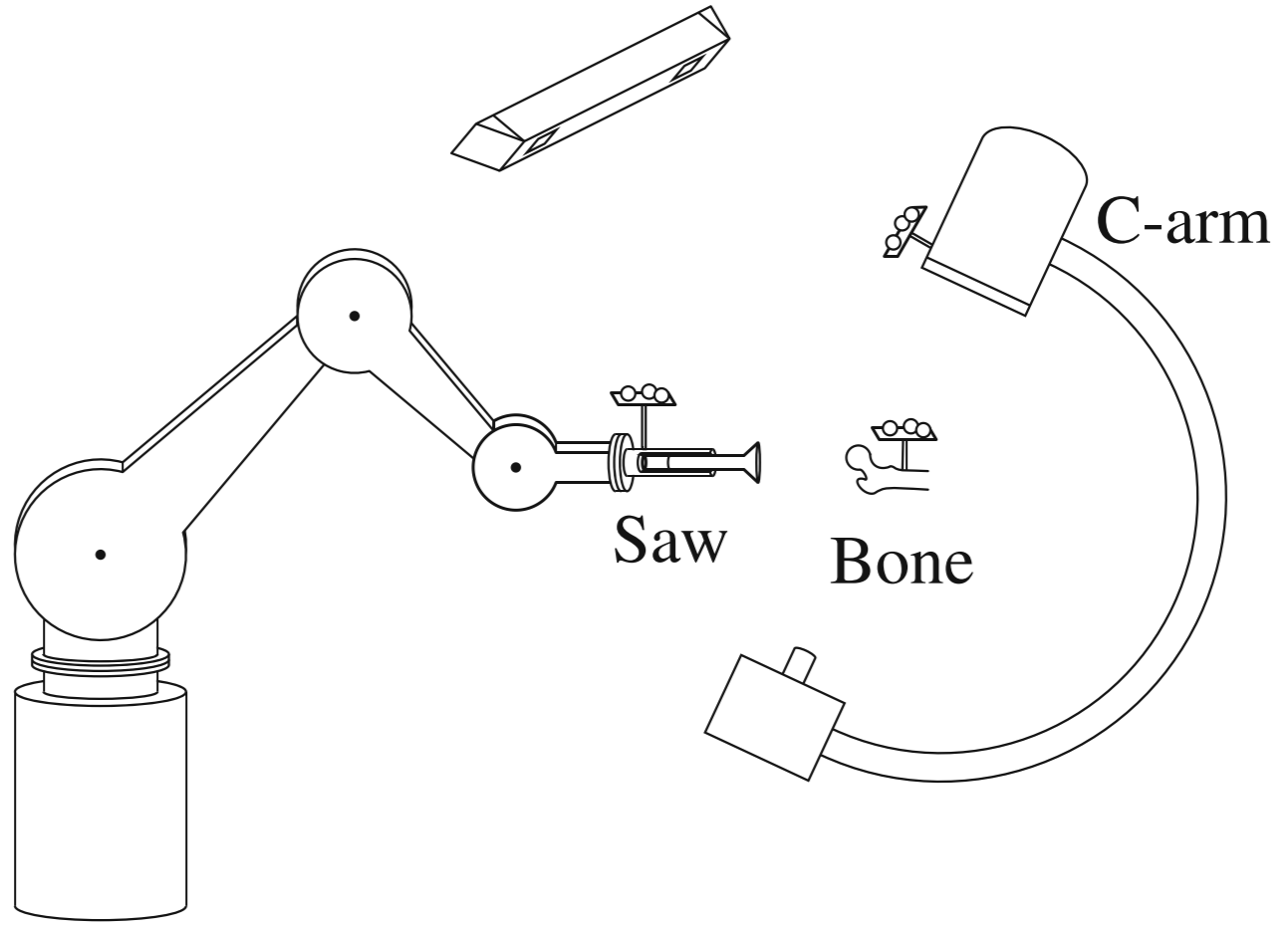
¿Posición del hueso con respecto al sistema de navegación?
¿Posición del hueso con respecto a la base del robot?
Matriz de transformación homogénea
ITB∼ITB
{^I}\mathcal{T}_B \sim {^I}\mathbf{T}_B
toda transformación lineal tiene asociada una matriz
Pose = posición + orientación
ITB
{^I}\mathbf{T}_B
pose de {B} con respecto de {I}
la matriz como tal también posee información
xR
x_R
yR
y_R
zR
z_R
{R}
\{R\}
xS
x_S
yS
y_S
zS
z_S
{S}
\{S\}
xC
x_C
yC
y_C
zC
z_C
{C}
\{C\}
xN
x_N
zN
z_N
yN
y_N
{N}
\{N\}
p
p
Cp
{^C}\mathbf{p}
Sp
{^S}\mathbf{p}
RTS
{^R}\mathcal{T}_S
NTS
{^N}\mathcal{T}_S
NTC
{^N}\mathcal{T}_C
¿Ubicación de la base del robot con respecto al sistema de navegación?
¿Pose (posición + orientación) de la sierra con respecto del brazo en C?
Marcos de referencia y transformaciones homogéneas BE3027 - Robótica Médica
BE3027 - Lecture 1 (2024)
By Miguel Enrique Zea Arenales
BE3027 - Lecture 1 (2024)
- 221
