pollev.com/chrismakler
Which Econ-related song is playing
right now?
Preferences and Utility
Christopher Makler
Stanford University Department of Economics
Econ 50: Lecture 7
🐟
🥥
Production Possibilities Fronier
Feasible
Last Wednesday: The PPF
Last Monday: Production Functions
Labor
Fish
🐟
Capital
Coconuts
🥥
[GOODS]
⏳
⛏
[RESOURCES]
🐟
🥥
🙂
😀
😁
😢
🙁
Today: Preferences
How does Chuck rank
all possible combinations
of fish and coconuts?
Goal: find the best combination within his production possibilities set.
Feasible
Today's Agenda
Today: Modeling Preferences with Utility Functions
Wednesday: Characteristics and Examples of Utility Functions
Preferences: Definition and Axioms
Indifference curves
The Marginal Rate of Substitution
Quantifying Happiness
The Mathematics of Utility Functions
Monotonicity and Convexity
Perfect Substitutes
Cobb-Douglas
Quasilinear
Preferences
Preferences: Ordinal Ranking of Options
Given a choice between option A and option B, an agent might have different preferences:
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
The agent strictly prefers A to B.
The agent strictly disprefers A to B.
The agent weakly prefers A to B.
The agent weakly disprefers A to B.
The agent is indifferent between A and B.
Sidebar: “Strictly" vs. “Weakly"
A \succ B
A \succeq B
The agent strictly prefers A to B.
The agent weakly prefers A to B.
2
3
4
5
6
x \ge 3
x \gt 3
Preference Axioms
Complete
Transitive
Any two options can be compared.
If \(A\) is preferred to \(B\), and \(B\) is preferred to \(C\),
then \(A\) is preferred to \(C\).
\text{For any options }A\text{ and }B\text{, either }A \succeq B \text{ or } B \succeq A
\text{If }A \succeq B \text{ and } B \succeq C\text{, then } A \succeq C
Together, these assumptions mean that we can rank
all possible choices in a coherent way.
Special case: choosing between bundles
containing different quantities of goods.
Preferences over Quantities
A=(4,3,6)
Example: “good 1” is apples, “good 2” is bananas, and “good 3” is cantaloupes:
🍏🍏🍏🍏
🍌🍌🍌
🍈🍈🍈🍈🍈🍈
B=(3,8,2)
🍏🍏🍏
🍌🍌🍌🍌🍌🍌🍌🍌
🍈🍈
General framework: choosing between anything
Special Case: Two Goods
Good 1 \((x_1)\)
Good 2 \((x_2)\)
Completeness axiom:
any two bundles can be compared.
Implication: given any bundle \(A\),
the choice space may be divided
into three regions:
preferred to A
dispreferred to A
indifferent to A
Indifference curves cannot cross!
A
The indifference curve through A connects all the bundles indifferent to A.
Indifference curve
through A
Marginal Rate of Substitution
X = (10,24)
🍏🍏
🍏🍏
🍏🍏
🍏🍏
🍏🍏
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
Y=(12,20)
🍏🍏
🍏🍏
🍏🍏
🍏🍏
🍏🍏
🍏🍏
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
🍌🍌🍌🍌
Suppose you were indifferent between the following two bundles:
Starting at bundle X,
you would be willing
to give up 4 bananas
to get 2 apples
Let apples be good 1, and bananas be good 2.
Starting at bundle Y,
you would be willing
to give up 2 apples
to get 4 bananas
MRS = {4 \text{ bananas} \over 2 \text{ apples}}
= 2\ {\text{bananas} \over \text{apple}}
Visually: the MRS is the magnitude of the slope
of an indifference curve
Quantifying Preferences
How do I love thee? Let me count the ways.
I love thee to the depth and breadth and height
My soul can reach, when feeling out of sight
For the ends of Being and ideal Grace.
I love thee to the level of everyday’s
Most quiet need, by sun and candlelight.
I love thee freely, as men strive for Right;
I love thee purely, as they turn from Praise.
I love thee with the passion put to use
In my old griefs, and with my childhood’s faith.
I love thee with a love I seemed to lose
With my lost saints,—I love thee with the breath,
Smiles, tears, of all my life!—and, if God choose,
I shall but love thee better after death.
Elizabeth Barrett Browning
Sonnets from the Portugese 43
18th/19th Centuries: Utilitarianism
the greatest happiness of the greatest number
is the foundation of morals and legislation.
Jeremy Bentham
the utilitarian standard...
is not the agent's own greatest happiness,
but the greatest amount of happiness, altogether.
John Stuart Mill
Introduction to the Principles of Morals and Legislation (1789)
Utilitarianism (1861)
How do we model preferences mathematically?
Approach: assume consuming goods "produces" utility
Production Functions
Labor
Fish
🐟
Capital
⏳
⛏
[RESOURCES]
Utility Functions
Utility
😀
[GOODS]
Fish
🐟
Coconuts
🥥
Preferences: Ordinal Ranking of Options
Given a choice between option A and option B, an agent might have different preferences:
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
The agent strictly prefers A to B.
The agent strictly disprefers A to B.
The agent weakly prefers A to B.
The agent weakly disprefers A to B.
The agent is indifferent between A and B.
Representing Preferences with a Utility Function
u(a_1,a_2) > u(b_1,b_2)
u(a_1,a_2) \ge u(b_1,b_2)
u(a_1,a_2) = u(b_1,b_2)
u(a_1,a_2) \le u(b_1,b_2)
u(a_1,a_2) < u(b_1,b_2)
u(x_1,x_2)
"A is strictly preferred to B"
Words
Preferences
Utility
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
"A is weakly preferred to B"
"A is indifferent to B"
"A is weakly dispreferred to B"
"A is strictly dispreferred to B"
Suppose the "utility function"
assigns a real number (in "utils")
to every possible consumption bundle
We get completeness because any two numbers can be compared,
and we get transitivity because that's a property of the operator ">"
An indifference curve is a set of all bundles between which a consumer is indifferent.
If a consumer is indifferent between two bundles (A ~ B), then \(u(a_1,a_2) = u(b_1,b_2)\)
Therefore, an indifference curve is a set of all consumption bundles which are assigned the same number of "utils" by the function \(u(x_1,x_2)\)
Likewise, set of bundles preferred to some bundle A is the a set of all consumption bundles which are assigned a greater number of "utils" by \(u(x_1,x_2)\)
Do we have to take the
number of "utils" seriously?
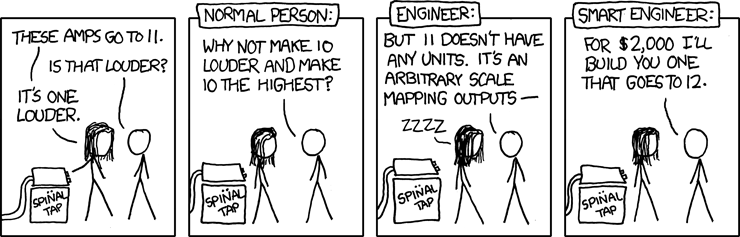
From XKCD:
Just like the "volume" on an amp, "utils" are an arbitrary scale...saying you're "11" happy from a bundle doesn't mean anything!
All we want to use utility functions for
is to describe preference orderings.
It doesn't matter that “utils" are nonsense.
As long as the utility function generates the correct indifference map,
it doesn't matter what the level of utility at each indifference curve is.
The Mathematics of Utility Functions
Marginal Utility
MU_1(x_1,x_2) = {\partial u(x_1,x_2) \over \partial x_1}
MU_2(x_1,x_2) = {\partial u(x_1,x_2) \over \partial x_2}
Given a utility function \(u(x_1,x_2)\),
we can interpret the partial derivatives
as the "marginal utility" from
another unit of either good:
Indifference Curves and the MRS
Along an indifference curve, all bundles will produce the same amount of utility
In other words, each indifference curve
is a level set of the utility function.
The slope of an indifference curve is the MRS. By the implicit function theorem,
MRS(x_1,x_2) = \frac{\partial u(x_1,x_2) \over \partial x_1}{\partial u(x_1,x_2) \over \partial x_2} = {MU_1 \over MU_2}
(Note: we'll treat this as a positive number, just like the MRTS and the MRT)
If you give up \(\Delta x_2\) units of good 2, how much utility do you lose?
If you get \(\Delta x_1\) units of good 1, how much utility do you gain?
\Delta u \approx \Delta x_2 \times MU_2
\Delta u \approx \Delta x_1 \times MU_1
If you end up with the same utility as you begin with:
\Delta x_2 \times MU_2 \approx \Delta x_1 \times MU_1
{\Delta x_2 \over \Delta x_1} \approx {MU_1 \over MU_2}
pollev.com/chrismakler
What is the MRS of the utility function \(u(x_1,x_2) = x_1x_2\)?
\displaystyle{MRS = {MU_1 \over MU_2} =}
u(x_1,x_2) = x_1x_2
\displaystyle{MU_1 = {\partial u(x_1,x_2) \over \partial x_1} =}
\displaystyle{MU_2 = {\partial u(x_1,x_2) \over \partial x_1} =}
4 \times 16
A = (4,16)
B = (8,8)
8 \times 8
x_2
x_1
\displaystyle{x_2 \over x_1}
= 64
= 64
16
8
4
8
\displaystyle{16 \over 4}
=4
\displaystyle{8 \over 8}
=1
MRS = 4
MRS = 1
Transforming Utility Functions
Applying a positive monotonic transformation to a utility function doesn't affect
the way it ranks bundles.
u(x_1,x_2) = x_1^{1 \over 2}x_2^{1 \over 2}
\hat u(x_1,x_2) = 2x_1^{1 \over 2}x_2^{1 \over 2}
Example: \(\hat u(x_1,x_2) = 2u(x_1,x_2)\)
u(4,16) = 8
u(9,4) = 6
u(4, 16) = 2 \times 8 = 16
u(9,4) = 2 \times 6 = 12
Transformations and the MRS
Applying a positive monotonic transformation to a utility function doesn't affect
its MRS at any bundle (and therefore generates the same indifference map).
u(x_1,x_2) = x_1^{1 \over 2}x_2^{1 \over 2}
\hat u(x_1,x_2) = 2x_1^{1 \over 2}x_2^{1 \over 2}
MU_1 = {1 \over 2}x_1^{-{1 \over 2}}x_2^{1 \over 2}
MU_2 = {1 \over 2}x_1^{{1 \over 2}}x_2^{-{1 \over 2}}
MU_1 = x_1^{-{1 \over 2}}x_2^{1 \over 2}
MU_2 = x_1^{{1 \over 2}}x_2^{-{1 \over 2}}
MRS =
\displaystyle{= {x_2 \over x_1}}
\hat{MRS} =
\displaystyle{= {x_2 \over x_1}}
Example: \(\hat u(x_1,x_2) = 2u(x_1,x_2)\)
Transformations and the MRS
Applying a positive monotonic transformation to a utility function doesn't affect
its MRS at any bundle (and therefore generates the same indifference map).
u(x_1,x_2) = x_1^{1 \over 2}x_2^{1 \over 2}
\hat u(x_1,x_2) = {1 \over 2}\ln x_1 + {1 \over 2}\ln x_2
MU_1 = {1 \over 2}x_1^{-{1 \over 2}}x_2^{1 \over 2}
MU_2 = {1 \over 2}x_1^{{1 \over 2}}x_2^{-{1 \over 2}}
MU_1 = {1 \over 2x_1}
MU_2 = {1 \over 2x_2}
MRS =
\displaystyle{= {x_2 \over x_1}}
\hat{MRS} =
\displaystyle{= {x_2 \over x_1}}
Example: \(\hat u(x_1,x_2) = \ln(u(x_1,x_2))\)
pollev.com/chrismakler
The utility function \(u(x_1,x_2) = x_1x_2^2\) represents the same preferences as which of the following utility functions? You may select more than one answer.
Summary
- Preferences are real; "utils" are not.
- We can use utility functions to represent preferences.
- Any two utility functions which generate the same difference curves represent the same preferences.
- After today, you can do exercises 2 and 3 on the homework. You'll be able to do exercise 1 after Wednesday's class.
Desirable Properties of Preferences
We've asserted that all (rational) preferences are complete and transitive.
There are some additional properties which are true of some preferences:
- Monotonicity
- Convexity
- Continuity
- Smoothness
Monotonic Preferences: “More is Better"
\text{For any bundles }A=(a_1,a_2)\text{ and }B=(b_1,b_2)\text{, }A \succeq B \text{ if } a_1 \ge b_1 \text{ and } a_2 \ge b_2
\text{In other words, all marginal utilities are positive: }MU_1 \ge 0, MU_2 \ge 0
Nonmonotonic Preferences and Satiation
Some goods provide positive marginal utility only up to a point, beyond which consuming more of them actually decreases your utility.
Strict vs. Weak Monotonicity
Strict monotonicity: any increase in any good strictly increases utility (\(MU > 0\) for all goods)
Weak monotonicity: no increase in any good will strictly decrease utility (\(MU \ge 0\) for all goods)
Example: Pfizer's COVID-19 vaccine has a dose of 0.3mL, Moderna's has a dose of 0.5mL
Goods vs. Bads
\text{Good: }MU > 0
\text{Bad: }MU < 0
Convex Preferences: “Variety is Better"
Take any two bundles, \(A\) and \(B\), between which you are indifferent.
Would you rather have a convex combination of those two bundles,
than have either of those bundles themselves?
If you would always answer yes, your preferences are convex.
Concave Preferences: “Variety is Worse"
Take any two bundles, \(A\) and \(B\), between which you are indifferent.
Would you rather have a convex combination of those two bundles,
than have either of those bundles themselves?
If you would always answer no, your preferences are convex.
Common Mistakes about Convexity
1. Convexity does not imply you always want equal numbers of things.
2. It's preferences which are convex, not the utility function.
Other Desirable Properties
Continuous: utility functions don't have "jumps"
Smooth: marginal utilities don't have "jumps"
Counter-example: vaccine dose example
Counter-example: Leontief/Perfect Complements utility function
Well-Behaved Preferences
If preferences are strictly monotonic, strictly convex, continuous, and smooth, then:
Indifference curves are smooth, downward-sloping, and bowed in toward the origin
The MRS is diminishing as you move down and to the right along an indifference curve
Good 1 \((x_1)\)
Good 2 \((x_2)\)
"Law of Diminishing MRS"
Next Steps
- Section: practice drawing indifference curves from utility functions
- Friday: look at specific utility functions and talk about the preferences they model
- Homework due Saturday night
- Next: maximizing utility subject to a PPF
Econ 50 | Lecture 07
By Chris Makler
Econ 50 | Lecture 07
Preferences and Utility Functions
