Profit Maximization
Christopher Makler
Stanford University Department of Economics
Econ 50: Lecture 18
Today's Agenda
- Overview of market structures
- Quick review of revenue, marginal revenue, and elasticity
- Profit maximization with market power
- Profit maximization for a competitive firm
Market Structures
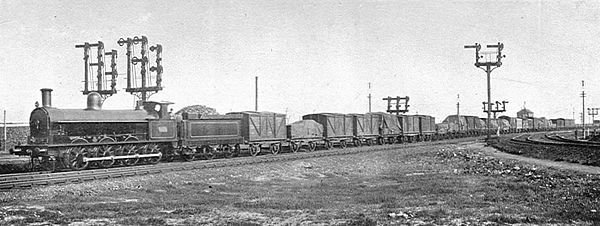
What were economists modeling when they came up with all these models?
Farmers producing commodities: price takers, no market power.
Railroads transporting goods:
price setters, lots of market power.
"Market Power" doesn't actually require a monopoly
Competition
- Lots of "small" firms selling basically the same thing
Market Power
- One or a few "medium" or "large" firms selling differentiated products
- Firms face essentially horizontal demand curve
- Firms face downward sloping demand curve
Monopoly as Metaphor
- True "monopolies" are rare
- Firms with some (at least local) market power are common
- We'll use "monopoly" as a metaphor
to analyze any firm that doesn't take prices as given,
and therefore faces a downward-sloping demand curve.
Review: Revenue, Marginal Revenue, and Elasticity
Demand and Inverse Demand
Demand curve:
quantity as a function of price
Inverse demand curve:
price as a function of quantity
QUANTITY
PRICE
Demand
Inverse Demand
q(p) = 20 - p
p(q) = 20-q
Revenue
r(q) =
MR(q)=
AR(q)=
20q - q^2
20 - 2q
20 - q
pollev.com/chrismakler
Suppose instead that the firm faced the demand function
(not inverse demand!)
\(q(p) = 20 - 2p\).
What would their marginal revenue function \(MR(q)\) be?
Correctness matters on this one...
Demand
Inverse Demand
q(p) = 20 - p
q(p) = 20 - 2p
p(q) = 20-q
p(q) = 10 - \tfrac{1}{2}q
Revenue
r(q) =
MR(q)=
AR(q)=
r(q) =
MR(q)=
AR(q)=
20q - q^2
20 - 2q
20 - q
10q - \tfrac{1}{2}q^2
10 - q
10 - \tfrac{1}{2}q
\text{marginal revenue} = {dr \over dq} = {dp \over dq} \times q + p
dr = dp \times q + dq \times p
p
p(q)
q
The total revenue is the price times quantity (area of the rectangle)
\text{marginal revenue} = {dr \over dq} = {dp \over dq} \times q + p
dr = dp \times q + dq \times p
p
p(q)
q
dp
dq
Note: \(MR < 0\) if
dq \times p
{dq \over q} < {dp \over p}
\% \Delta q < \% \Delta p
|\epsilon| < 1
dp \times q
<
The total revenue is the price times quantity (area of the rectangle)
If the firm wants to sell \(dq\) more units, it needs to drop its price by \(dp\)
Revenue loss from lower price on existing sales of \(q\): \(dp \times q\)
Revenue gain from additional sales at \(p\): \(dq \times p\)
\text{marginal revenue} = {dr \over dq} = {dp \over dq} \times q + p
Marginal Revenue and Elasticity
= \left[{dp \over dq} \times {q \over p}\right] \times p + p
= {p \over {dq \over dp} \times {p \over q}} + p
= p\left(1 - {1 \over |\epsilon_{q,p}|}\right)
(multiply first term by \(p/p\))
(simplify)
(since \(\epsilon < 0\))
Notes
Elastic demand: \(MR > 0\)
Inelastic demand: \(MR < 0\)
In general: the more elastic demand is, the less one needs to lower ones price to sell more goods, so the closer \(MR\) is to \(p\).
MR = p\left(1 - {1 \over |\epsilon_{q,p}|}\right)
The more elastic demand is, the less MR is different than price.
Which part of a linear demand curve is more elastic?
Profit Maximization with Market Power
\pi(q) = r(q) - c(q)
Optimize by taking derivative and setting equal to zero:
\pi'(q) = r'(q) - c'(q) = 0
\Rightarrow r'(q) = c'(q)
Profit is total revenue minus total costs:
"Marginal revenue equals marginal cost"
Example:
p(q) = 20 - q \Rightarrow
c(q) = 64 + {1 \over 4}q^2
What is the profit-maximizing value of \(q\)?
r(q) = 20q - q^2
\pi(q) = \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ -
Optimize by taking derivative and setting equal to zero:
\pi'(q) = \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ - \ \ \ \ \ \ \ \ \ = 0
\Rightarrow \ \ \ \ \ \ \ \ \ \ \ \ \ \ =
Profit is total revenue minus total costs:
"Marginal revenue equals marginal cost"
(64 + {1 \over 4}q^2)
(20q - q^2)
r'(q)
c'(q)
{1 \over 2}q
20 - 2q
r'(q)
c'(q)
{1 \over 2}q
20 - 2q
40 - 4q = q
40 = 5q
q^* = 8
CHECK YOUR UNDERSTANDING
p(q)=20-2q
c(q)=10+5q+{1 \over 2}q^2
Find the profit-maximizing quantity.
Average Profit Analysis
\pi(q) = r(q) - c(q)
Multiply right-hand side by \(q/q\):
\pi(q) = \left[{r(q) \over q} - {c(q) \over q}\right] \times q
= (AR - AC) \times q
Profit is total revenue minus total costs:
"Profit per unit times number of units"
AVERAGE PROFIT
Elasticity and Profit Maximization
MR = p\left[1 - \frac{1}{|\epsilon_{q,p}|}\right]
Recall our elasticity representation of marginal revenue:
MR = MC
Let's combine it with this
profit maximization condition:
p\left[1 - \frac{1}{|\epsilon_{q,p}|}\right] = MC
p = \frac{MC}{1 - \frac{1}{|\epsilon_{q,p}|}}
Really useful if MC and elasticity are both constant!
Inverse elasticity pricing rule:
p = \frac{MC}{1 - \frac{1}{|\epsilon_{q,p}|}}
If a firm has the cost function $$c(q) = 200 + 4q$$ and faces the demand curve $$D(p) = 6400p^{-2}$$ what is its optimal price?
Inverse elasticity pricing rule:
One more way of slicing it...
P = \frac{MC}{1 - \frac{1}{|\epsilon|}}
1 - \frac{1}{|\epsilon|} = \frac{MC}{P}
\frac{P - MC}{P} = \frac{1}{|\epsilon|}
Fraction of price that's markup over marginal cost
(Lerner Index)
What if \(|\epsilon| \rightarrow \infty\)?
Competitive (Price-Taking) Firms
Demand and Inverse Demand
Demand curve:
quantity as a function of price
Inverse demand curve:
price as a function of quantity
QUANTITY
PRICE
Special case: perfect substitutes
Demand and Inverse Demand
Demand curve:
quantity as a function of price
Inverse demand curve:
price as a function of quantity
QUANTITY
PRICE
Special case: perfect substitutes
For a small firm, it probably looks like this...
\text{marginal revenue} = {dr \over dq} = {dp \over dq} \times q + p
Marginal Revenue for Perfectly Elastic Demand
= \left[{dp \over dq} \times {q \over p}\right] \times p + p
= {p \over {dq \over dp} \times {p \over q}} + p
= p \left (1 - {1 \over |\epsilon_{q,p}|} \right)
(multiply first term by \(p/p\))
(simplify)
(since \(\epsilon < 0\))
Note
Perfectly elastic demand: \(MR = p\)
c(q) = 64 + {1 \over 4}q^2
r(q) = pq = 12q
\text{Let's assume }p = 12:
\text{Profit function is:}
\text{Total cost}
\pi(q) = 12q - [64 + {1 \over 4}q^2]
\text{Take derivative with respect to } q \text{ and set equal to zero:}
\pi'(q) = \ \ \ \ \ \ - \ \ \ \ \ \ = 0
12
{1 \over 2}q
Price
MC
\(q\)
$/unit
P = MR
12
MC = {1 \over 2}q
24
\Rightarrow q^* = 24
Summary
- All firms maximize profits by setting MR = MC
- If a firm faces a downward-sloping demand curve,
the marginal revenue is less than the price. - The more elastic a firm's demand curve,
the less it will optimally raise its price above marginal cost. - A competitive firm faces a perfectly elastic demand curve,
so its marginal revenue is equal to the price. - Next week: establish a competitive firm's output supply and labor demand as functions of \(p\) and \(w\)
Econ 50 | Lecture 18
By Chris Makler
Econ 50 | Lecture 18
Profit Maximization, With and Without Market Power
