Characteristics of
Production Functions
Christopher Makler
Stanford University Department of Economics
Econ 50: Lecture 3
Today's Agenda
Part 1: Elasticity of Substitution
Part 2: Scaling
Production functions and their MRTS
Elasticity of substitution
Scaling in the long run (returns to scale)
Scaling in the short run (holding K fixed)
What happens as you move
along an isoquant
What happens as you move
between isoquants
Examples of Production Functions
f(L,K) = aL + bK
Linear
f(L,K) = \min\{aL, bK\}
Leontief
(Fixed Proportions)
Cobb-Douglas
f(L,K) = AL^aK^b
Constant Elasticity of Substitution (CES)
f(L,K) = (aL^\rho + bK^\rho)^{1 \over \rho}
Linear Production Function
MP_L =
MP_K =
MRTS =
\displaystyle{a\ {\text{output} \over \text{unit of L}}}
\displaystyle{b\ {\text{output} \over \text{unit of K}}}
\displaystyle{= {a \text{ units of K} \over b \text{ units of L}}}
q=f(L,K)=aL + bK
Leontief (Fixed Proportions) Production Function
f(L,K)=\begin{cases}aL \text{\ \ if }aL \le bK\\bK \text{\ \ if }aL \ge bK\end{cases}
=\min\{aL,bK\}
MP_L =
MP_K =
MRTS =
\begin{cases}a \text{\ \ if }aL < bK\\0 \text{\ \ if }aL > bK\end{cases}
\begin{cases}0 \text{\ \ if }aL < bK\\b \text{\ \ if }aL > bK\end{cases}
= \begin{cases}\infty \text{\ \ if }aL < bK\\0 \text{\ \ if }aL > bK\end{cases}
Cobb-Douglas Production Function
q=f(L,K)=AL^aK^b
MP_L = aAL^{a-1}K^b
MP_K = bAL^aK^{b-1}
MRTS =
\displaystyle{= {a \over b} \times {K \over L}}
CES Production Function
q=f(L,K)=(aL^\rho + bK^\rho)^{1 \over \rho}
\displaystyle{MRTS= {a \over b} \times \left({K \over L}\right)^{1-\rho}}
MRTS for Different Production Functions
aL + bK
Linear
\min\{aL, bK\}
Leontief
(Fixed Proportions)
Cobb-Douglas
AL^aK^b
CES
(aL^\rho + bK^\rho)^{1 \over \rho}
f(L,K)
MRTS
\frac{a}{b}
\begin{cases}\infty \text{\ \ if }aL < bK\\0 \text{\ \ if }aL > bK\end{cases}
\frac{a}{b}\times \frac{K}{L}
\frac{a}{b}\times \left(\frac{K}{L}\right)^{1-\rho}
Interpreting the MRTS
(slope of an isoquant)
"What is the rate at which
one can substitute
one input for another
and keep output the same?"
Another way of thinking about it:
"If we fired one worker and wanted to keep output the same,
how many additional machines would we need to buy?"
Elasticity of Substitution
- Measures the substitutability of one input for another
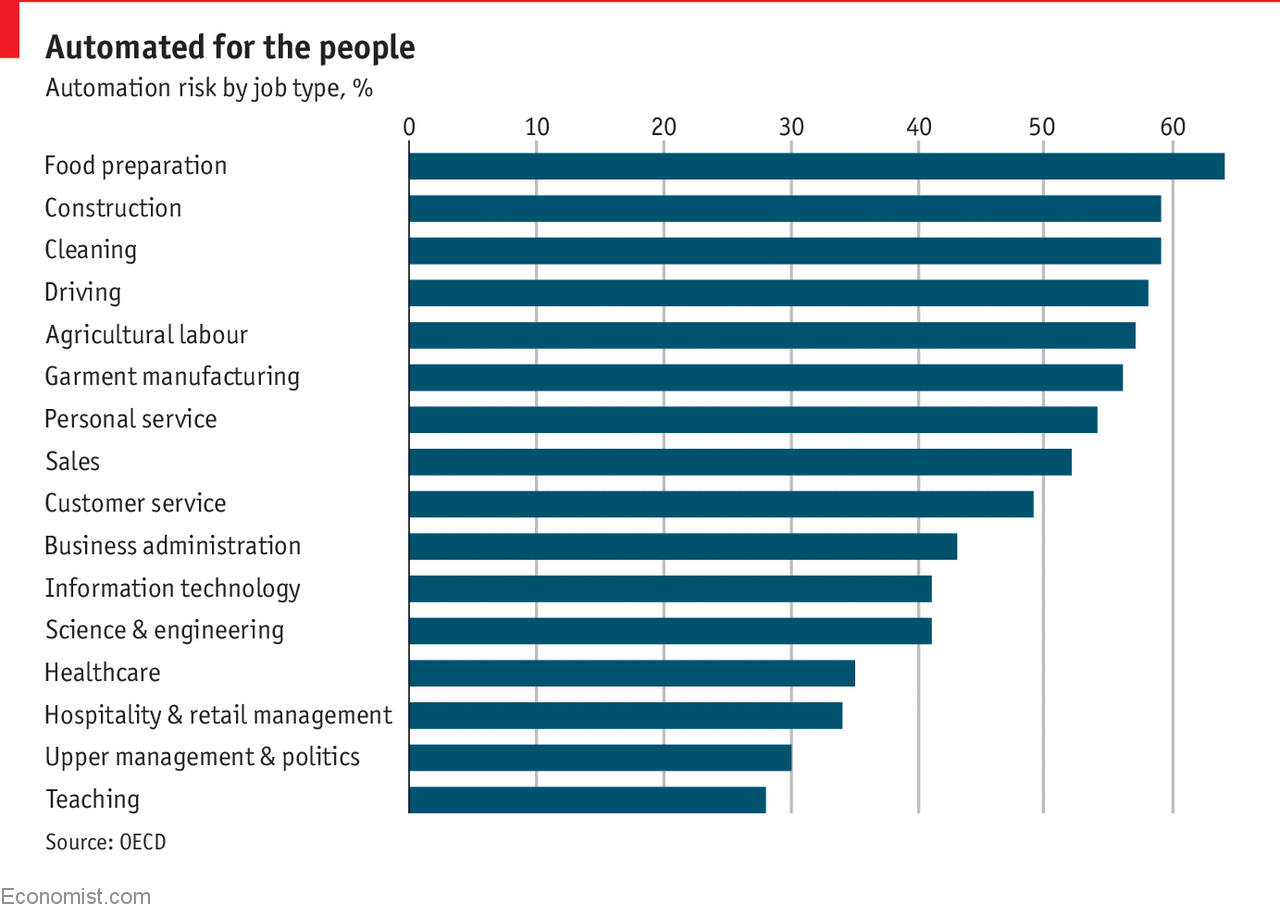
- Key to answering the question: "will my job be automated?"
- Formal definition: the inverse of the percentage change in the MRTS
per percentage change in the ratio of capital to labor, K/L - Intuitively: how "curved" are the isoquants for a production function?
Scaling Production
How does a technology respond to increasing production?
Short run: only some resources can be reallocated
Long run: all resources can be reallocated
It depends on the time horizon:
Scaling Production in the Short Run
Suppose \(K\) is fixed at some \(\overline K\) in the short run.
Then the production function becomes \(f(L\ |\ \overline K)\)
pollev.com/chrismakler
When does the production function
f(L) = 10L^a
exhibit diminishing marginal product of labor?
Scaling Production in the Long Run
What happens when we increase all inputs proportionally?
For example, what happens if we double both labor and capital?
Does doubling inputs -- i.e., getting \(f(2L,2K)\) -- double output?
f(2L,2K) > 2f(L,K)
f(2L,2K) = 2f(L,K)
f(2L,2K) < 2f(L,K)
Decreasing Returns to Scale
Constant Returns to Scale
Increasing Returns to Scale
f(L,K) = 4L^{1 \over 2}K
Does this exhibit diminishing, constant or increasing MPL?
Does this exhibit decreasing, constant or increasing returns to scale?
pollev.com/chrismakler
When does the production function
f(L,K) = AL^aK^b
exhibit constant returns to scale?
Next Steps
- Homework for the last two classes is due on Saturday night
- Readings (and old videos) for next week have been posted
- Quizzes for next week will be posted later today
Econ 50 | Lecture 03
By Chris Makler
Econ 50 | Lecture 03
Characteristics of Production Functions
