Intertemporal Choice

Christopher Makler

Stanford University Department of Economics

Econ 51: Lecture 3

Today's Agenda

Part 1: Baseline Case

Part 2: Extensions and Applications

Modeling present-future tradeoffs

The intertemporal budget constraint

Preferences over time

Optimal saving and borrowing

Different interest rates for borrowing and saving

Credit constraints

Real and nominal interest rates

Beyond two time periods

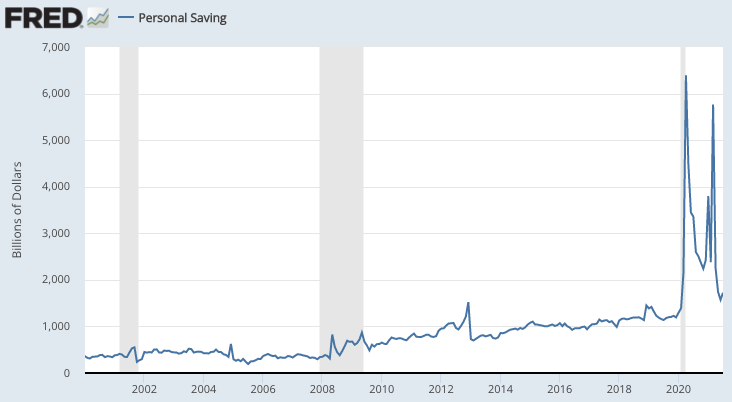
Saving and borrowing is a huge part of the U.S. economy.

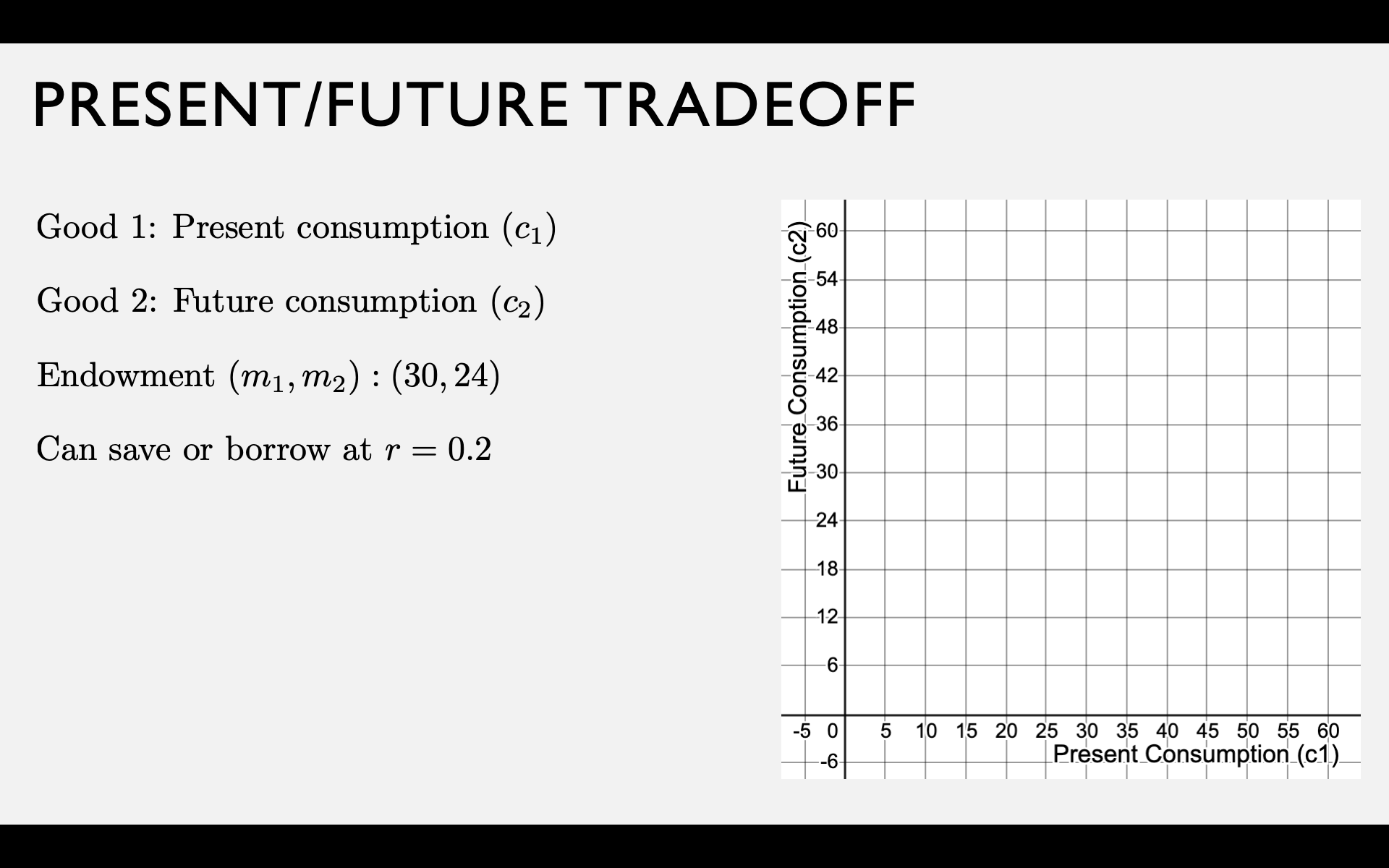
Endowment of time and money.

Today: Present vs. Future Consumption

Endowment of money in different time periods (an "income stream")

Last time: Leisure vs. Consumption

Working = trading time for money

Saving = trading present consumption for future consumption

Borrowing = trading future consumption for present consumption

For Each Context:

Determine the budget line

Analyze preferences

Solve for optimal choice

Comparative statics: analyze net supply and demand

Do you have to spend all the money you earn in the period when you earn it?

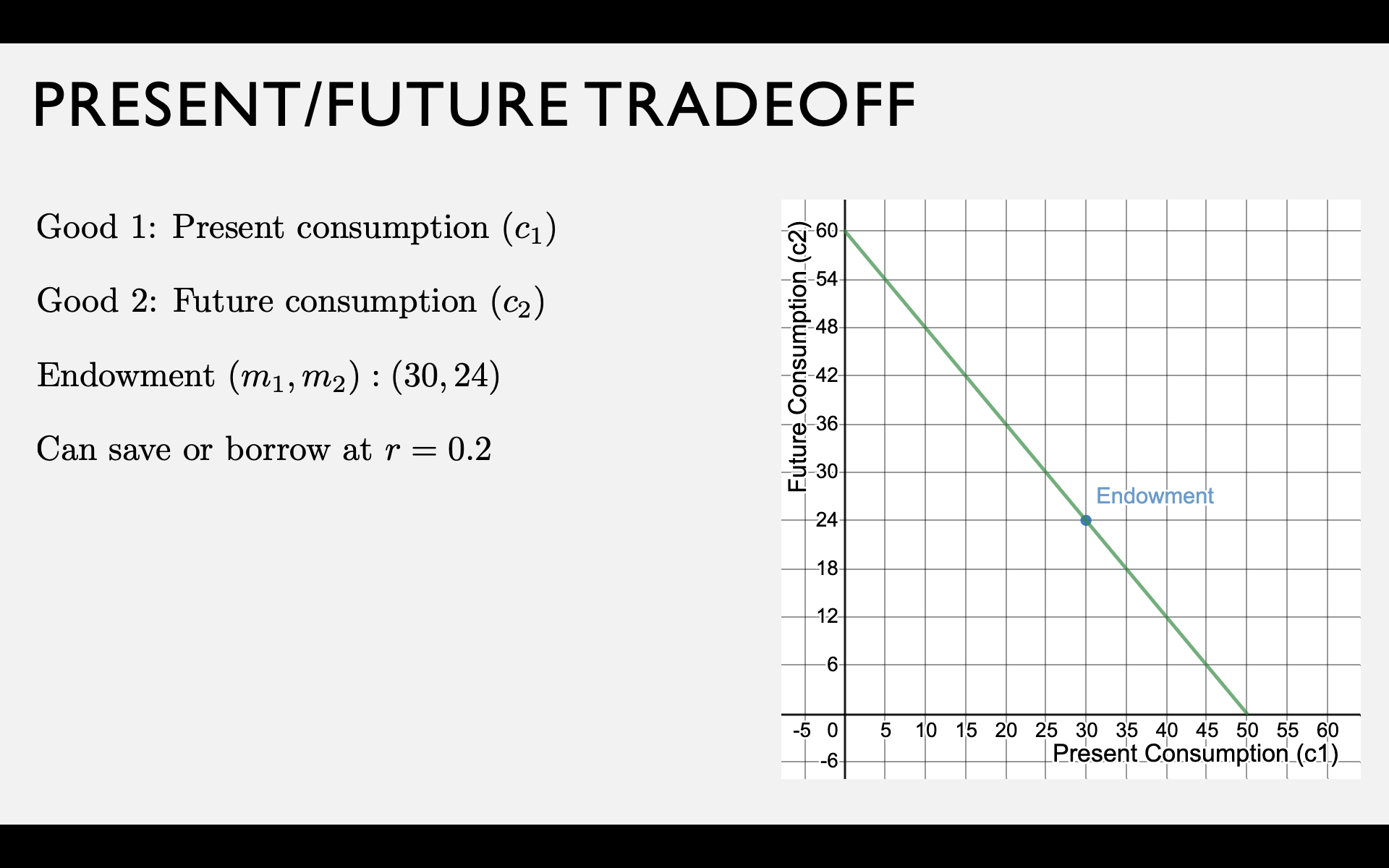
Present-Future Tradeoff

Your endowment is an income stream of $m_1$ dollars now and $m_2$ dollars in the future.

What happens if you don't consume all $m_1$ of your present income?

Two "goods" are present consumption $c_1$ and future consumption $c_2$.

c_1 = m_1 - s

c_2 = m_2 + s

Let $s = m_1 - c_1$ be the amount you save.

Saving and Borrowing with Interest

If you save at interest rate $r$,
for each dollar you save today,
you get $1 + r$ dollars in the future.

You can either save some of your current income, or borrow against your future income.

If you borrow at interest rate $r$,
for each dollar you borrow today,
you have to repay $1 + r$ dollars in the future.

c_1 = m_1 - s

c_2 = m_2 + (1+r)s

c_2 = m_2 + (1+r)(m - c_1)

(1+r)c_1 + c_2 = (1+r)m_1 + m_2

c_1 = m_1 + b

c_2 = m_2 - (1+r)b

c_2 = m_2 - (1+r)(c_1 - m_1)

(1+r)c_1 + c_2 = (1+r)m_1 + m_2

(1+r)c_1 + c_2 = (1 + r)m_1 + m_2

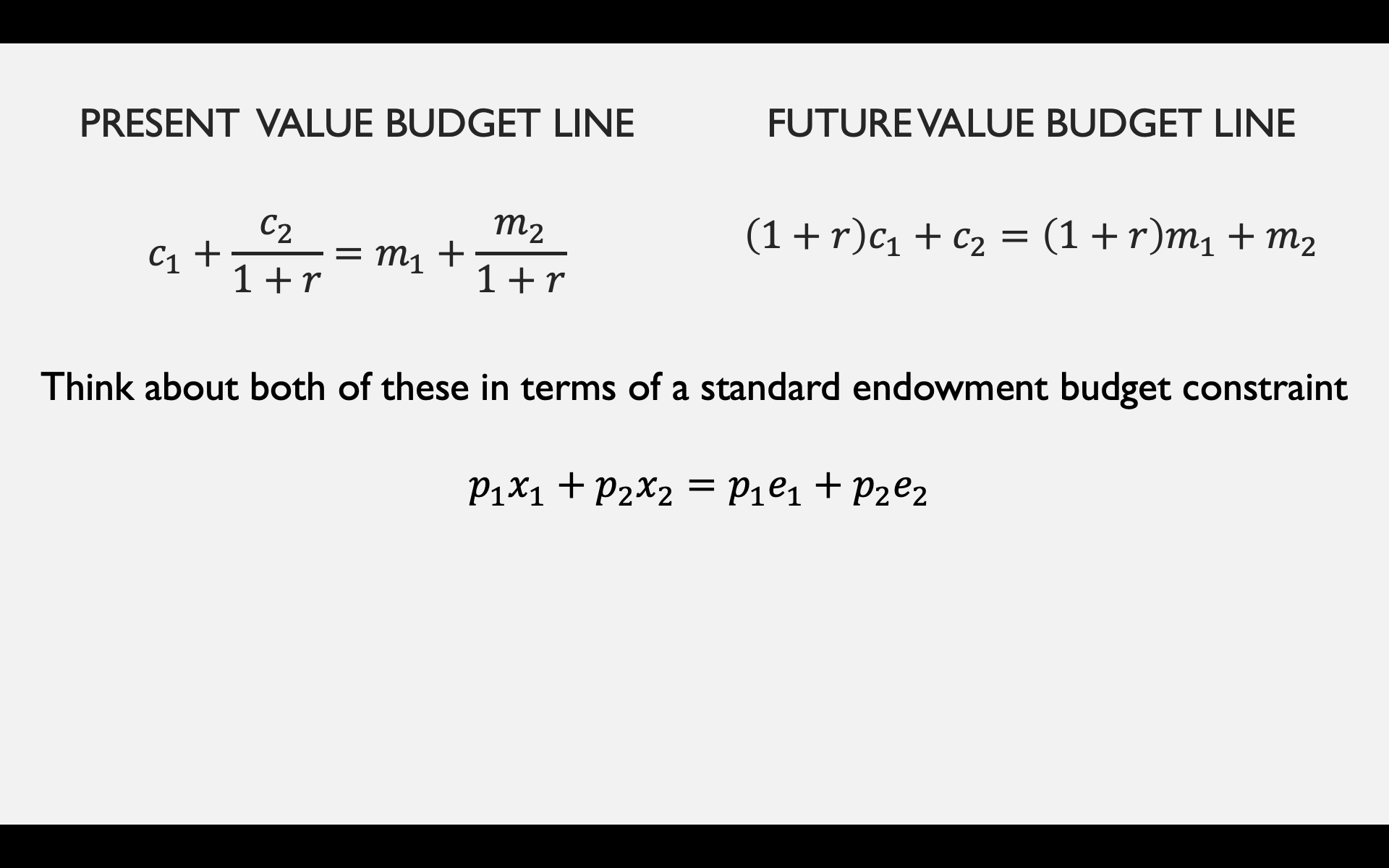
"Future Value"

1.2c_1 + c_2 = 1.2 \times 30 + 24

1.2c_1 + c_2 = 60

c_1 + \frac{c_2}{1+r} = m_1 + \frac{m_2}{1 + r}

"Present Value"

c_1 + \frac{c_2}{1.2} = 30 + \frac{24}{1.2}

c_1 + \frac{c_2}{1.2} = 50

Preferences over Time

u(c_1,c_2) = v(c_1)+\beta v(c_2)

v(c) = \text{“within-period" utility}

\beta = \text{“between-period" discount factor}

v(c) = \ln c

v(c) = c

u(c_1,c_2) = \ln c_1 + \beta \ln c_2

Examples:

v(c) = \sqrt{c}

u(c_1,c_2) = c_1 + \beta c_2

u(c_1,c_2) = \sqrt{c_1} + \beta \sqrt{c_2}

When to borrow and save?

u(c_1,c_2) = v(c_1)+\beta v(c_2)

MRS \text{ at endowment }= {v^\prime (m_1) \over \beta v^\prime (m_2)}

Save if MRS at endowment < $1 + r$

Borrow if MRS at endowment > $1 + r$

(high interest rates or low MRS)

(low interest rates or high MRS)

If we assume $v(c)$ exhibits diminishing marginal utility:
MRS is higher if you have less money today ($m_1$ is low)
and/or more money tomorrow ($m_2$ is high)

MRS is lower if you are more patient ($\beta$ is high)

u(c_1,c_2) = v(c_1)+\beta v(c_2)

MRS \text{ at endowment }= {v^\prime (m_1) \over \beta v^\prime (m_2)}

Save if MRS at endowment < $1 + r$

Borrow if MRS at endowment > $1 + r$

pollev.com/chrismakler

MRS(c_1,c_2) = {c_2 \over \beta c_1}

\text{Example: }v(c_t) = \ln c_t

u(c_1,c_2) = \ln c_1 + \beta \ln c_2

If $m_1 = 30$, $m_2 = 24$, and $\beta = 0.5$,
what is the highest interest rate at which you would borrow money?

Borrow or Save?

MRS(c_1,c_2) = {c_2 \over \beta c_1}

\text{MRS at endowment } =

\text{Price Ratio } =

\text{Example: }v(c_t) = \ln c_t \Rightarrow u(c_1,c_2) = \ln c_1 + \beta \ln c_2

m_1 = 30, m_2 = 24, \beta = 0.5

\text{Generic }m_1,m_2,r

\text{Borrow if } :

MRS(m_1,m_2) = {m_2 \over \beta m_1}

MRS(30,24) = {24 \over 0.5 \times 30}

1 + r

= 1.6

1 + r

1.6

1 + r

MRS

r < 0.6

1 + r

Optimal Bundle

\text{Example: }v(c_t) = \ln c_t \Rightarrow u(c_1,c_2) = \ln c_1 + \beta \ln c_2

Tangency condition:

Budget line:

MRS(c_1,c_2) = {c_2 \over \beta c_1}

{c_2 \over \beta c_1} = 1 + r

(1 + r)c_1 + c_2 = (1 + r)m_1 + m_2

\Rightarrow c_2 = (1+r)\beta c_1

(1 + r)c_1 + (1+r)\beta c_1 = (1 + r)m_1 + m_2

c_1^* = {1 \over 1 + \beta}(m_1 + {m_2 \over 1 + r})

c_2^* = {\beta \over 1 + \beta}((1 + r)m_1 + m_2)

If $m_1 = 30$, $m_2 = 24$, $\beta = 0.25$, and $r = 0.2$,
what is your optimal choice?

pollev.com/chrismakler

Optimal Bundle

\text{Example: }v(c_t) = \ln c_t \Rightarrow u(c_1,c_2) = \ln c_1 + \beta \ln c_2

Tangency condition:

Budget line:

m_1 = 30, m_2 = 24, \beta = 0.25, r = 0.2

{c_2 \over \beta c_1} = 1 + r

{c_2 \over 0.25 c_1} = 1.2

(1 + r)c_1 + c_2 = (1 + r)m_1 + m_2

1.2c_1 + c_2 = 1.2 \times 30 + 24

1.2c_1 + c_2 = 60

\Rightarrow c_2 = 0.3 c_1

\Rightarrow c_2 = (1+r)\beta c_1

(1 + r)c_1 + (1+r)\beta c_1 = (1 + r)m_1 + m_2

1.2c_1 + 0.3 c_1 = 60

c_1^* = {1 \over 1 + \beta}(m_1 + {m_2 \over 1 + r})

c_2^* = {\beta \over 1 + \beta}((1 + r)m_1 + m_2)

c_1^* = 40

Since you start with $m_1 = 30$, this means you borrow 10.

MRS(c_1,c_2) = {c_2 \over \beta c_1}

Supply of Savings and
Demand for Borrowing

In general, net demand is $x_1^* - e_1$

In this context, net demand is the demand for borrowing.

If it's negative, then it's the supply of saving.

Different Interest Rates

MRS > 1 + r

MRS < 1 + r

BORROW

SAVE

What if the interest rate is different for borrowing and saving?

When a Budget Line Ends...

c_2 = m_2 + (1 + r)(m_1 - c_1)

Inflation and Real Interest Rates

Suppose there is inflation,
so that each dollar saved can only buy
$1/(1 + \pi)$ of what it originally could:

c_2 = m_2 + \left({1 + r\over 1 + \pi}\right)(m_1 - c_1)

Up to now, we've been just looking at
dollar amounts in both periods

\text{let }\rho = {1 + r \over 1 + \pi} - 1

c_2 = m_2 + (1 + \rho)(m_1 - c_1)

We call $r$ the "nominal interest rate" and $\rho$ the "real interest rate"

For low values of $r$ and $\pi$, $\rho \approx r - \pi$

c_1 + \frac{c_2}{1+r} = m_1 + \frac{m_2}{1 + r}

"Present Value" for two periods

Beyond Two Periods

If you save $s$ now, you get $x = s(1 + r)$ next period.

The amount you have to save in order to get $x$ one period in the future is

s(1 + r) = x

s = {x \over 1 + r}

Remember how we got this...

If you save $s$ now, you get $x = s(1 + r)$ next period.

The amount you have to save in order to get $x$ one period in the future is

s(1 + r) = x

s = {x \over 1 + r}

If you save for two periods, it grows at interest rate $r$ again, so $x_2 = (1+r)(1+r)s = (1+r)^2s$

Therefore, the amount you have to save in order to get $x_2$ two periods in the future is

s(1 + r)^2 = x_2

s = {x_2 \over (1 + r)^2}

If you save for two periods, it grows at interest rate $r$ again, so $x_2 = (1+r)(1+r)s = (1+r)^2s$

Therefore, the amount you have to save in order to get $x_2$ two periods in the future is

s(1 + r)^2 = x_2

s = {x_2 \over (1 + r)^2}

If you save for $t$ periods, it grows at interest rate $r$ each period, so $x_t = (1+r)^ts$

Therefore, the amount you have to save in order to get $x_t$, $t$ periods in the future, is

s(1 + r)^t = x_t

s = {x_t \over (1 + r)^t}

Therefore, the amount you have to save in order to get $x_t$, $t$ periods in the future, is

s(1 + r)^t = x_t

s = {x_t \over (1 + r)^t}

We call this the present value of a payoff of $x_t$

PV(x_t) = {x_t \over (1 + r)^t}

Application: Social Cost of Carbon

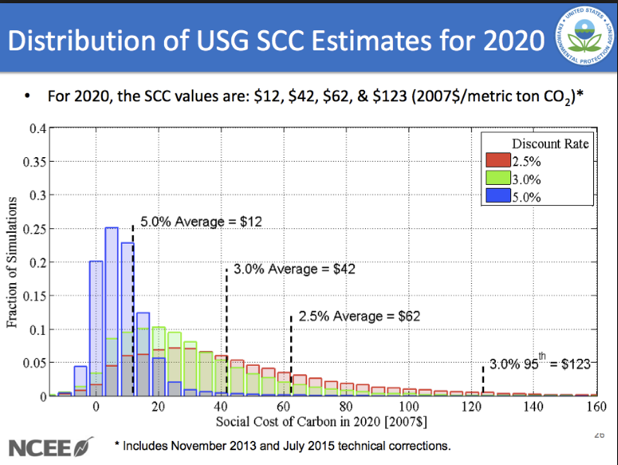
Obama Admin: $45

Uses a 3% discount rate; includes global costs

Trump Admin: less than $6

Uses a 7% discount rate; only includes American costs

PV of $1 Trillion in 2100:
$86B for Obama, $4B for Trump

Most of what we did was to extend the endowment framework to another context.

The price ratio is the real interest rate: i.e., the cost of increasing consumption today, which is foregone consumption tomorrow - based on interest rate and inflation.

The utility function is a combination of a within-period utility $v(c_t)$ and between-utility discounting $\beta$.

Conclusion and Next Steps

Other important new feature: kinked budget constraints representing different prices for buying and selling.

Next time: similar utility function for consumption in different states of the world.

Econ 51 | 03 | Intertemporal Choice

By Chris Makler

Econ 51 | 03 | Intertemporal Choice

We apply the framework of consumer choice theory to the choice of how to allocation money across time, investigating saving and borrowing.

Intertemporal Choice