Dynamic Games of Incomplete Information
Christopher Makler
Stanford University Department of Economics
Econ 51: Lecture 15
- Beliefs and Updating
- Perfect Bayesian Equilibrium
- Job Market Signaling
Today's Agenda
- Complete information: everyone knows everything about the game
- Incomplete information: players have private information
(e.g., they know what their valuation of a good is)
- Incomplete information: players have private information
- Perfect information: everyone can perfectly observe everyone else's moves
- Imperfect information: players' moves are hidden from other players
(e.g. I cannot observe how much time you spend studying)
- Imperfect information: players' moves are hidden from other players
Information
Time
Information
Static
(Simultaneous)
Dynamic
(Sequential)
Complete
Incomplete
WEEKS 5 & 6
WEEK 7
LAST TIME
TODAY
Prisoners' Dilemma
Cournot
Entry Deterrence
Stackelberg
Auctions
Job Market Signaling
Collusion
Cournot with Private Information
Poker
Time
Information
Static
(Simultaneous)
Dynamic
(Sequential)
Complete
Incomplete
Strategy: an action
Equilbirium: Nash Equilibrium
Strategy: a mapping from the history of the game onto an action.
Equilibrium: Subgame Perfect NE
Strategy: a plan of action that
specifies what to do after every possible history of the game, based on one's own private information and (updating) beliefs about other players' private information.
Equilibrium: Perfect Bayesian Equilibrium
Strategy: a mapping from one's private information onto an action.
Equilibrium: Bayesian NE
WEEKS 5 & 6
WEEK 7
LAST TIME
TODAY
Prisoners' Dilemma
Cournot
Entry Deterrence
Stackelberg
Auctions
Job Market Signaling
Collusion
Cournot with Private Information
Poker
Bayes' Rule and Conditional Beliefs
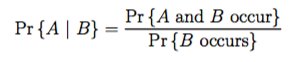
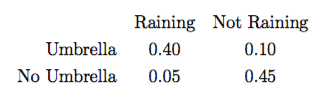
Suppose you don't know whether it's raining out,
but you can observe whether
I'm carrying an umbrella or not.
Ex ante, you believe the joint probabilities
of these events are given by this table:
Bayes' Rule:
Before you see whether I'm carrying an umbrella, with what probability do you believe it's raining?

Bayes' Rule and Conditional Beliefs
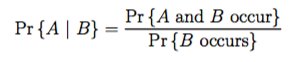

Suppose you don't know whether it's raining out,
but you can observe whether
I'm carrying an umbrella or not.
Ex ante, you believe the joint probabilities
of these events are given by this table:
Bayes' Rule:

Suppose you see me with an umbrella. Now with what probability do you think it's raining?
Conditional Beliefs and Strategies
- Suppose you're at an information set; you don't know which of several nodes you might be at.
- How can you use what you know about other players' strategies to form beliefs about the true state of the world?
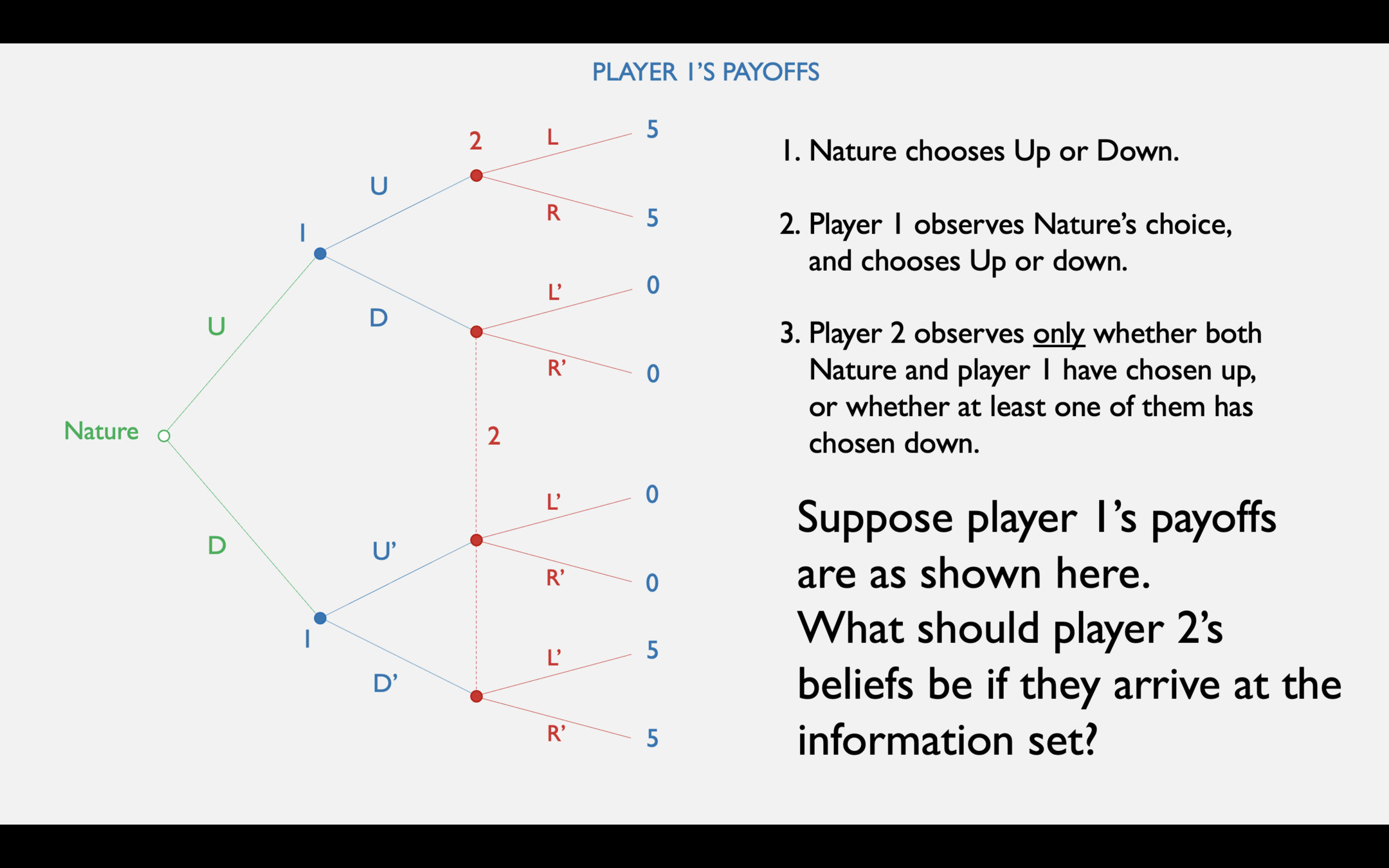
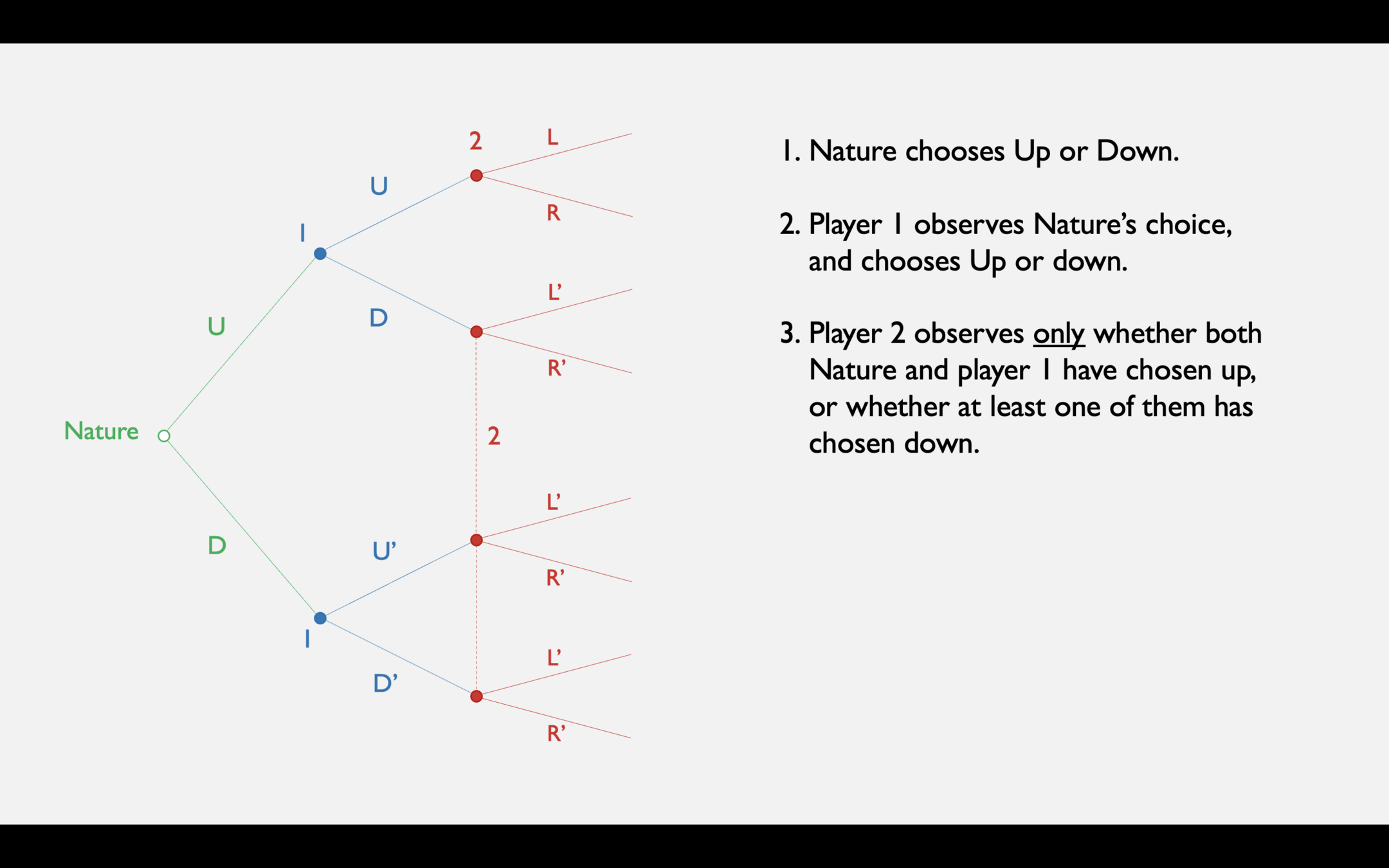
Perfect Bayesian Equilibrium
Consider a strategy profile for the players, as well as beliefs over the nodes at all information sets.
These are called a perfect Bayesian Equilibrium (PBE) if:
- Each player’s strategy is optimal to them at each infoset, given beliefs at this infoset and opponents’ strategies (“Sequential Rationality”)
- The beliefs are obtained from strategies using Bayes’ Rule wherever possible (i.e. at each infoset that is reached with a positive probability) (“consistency of beliefs”)
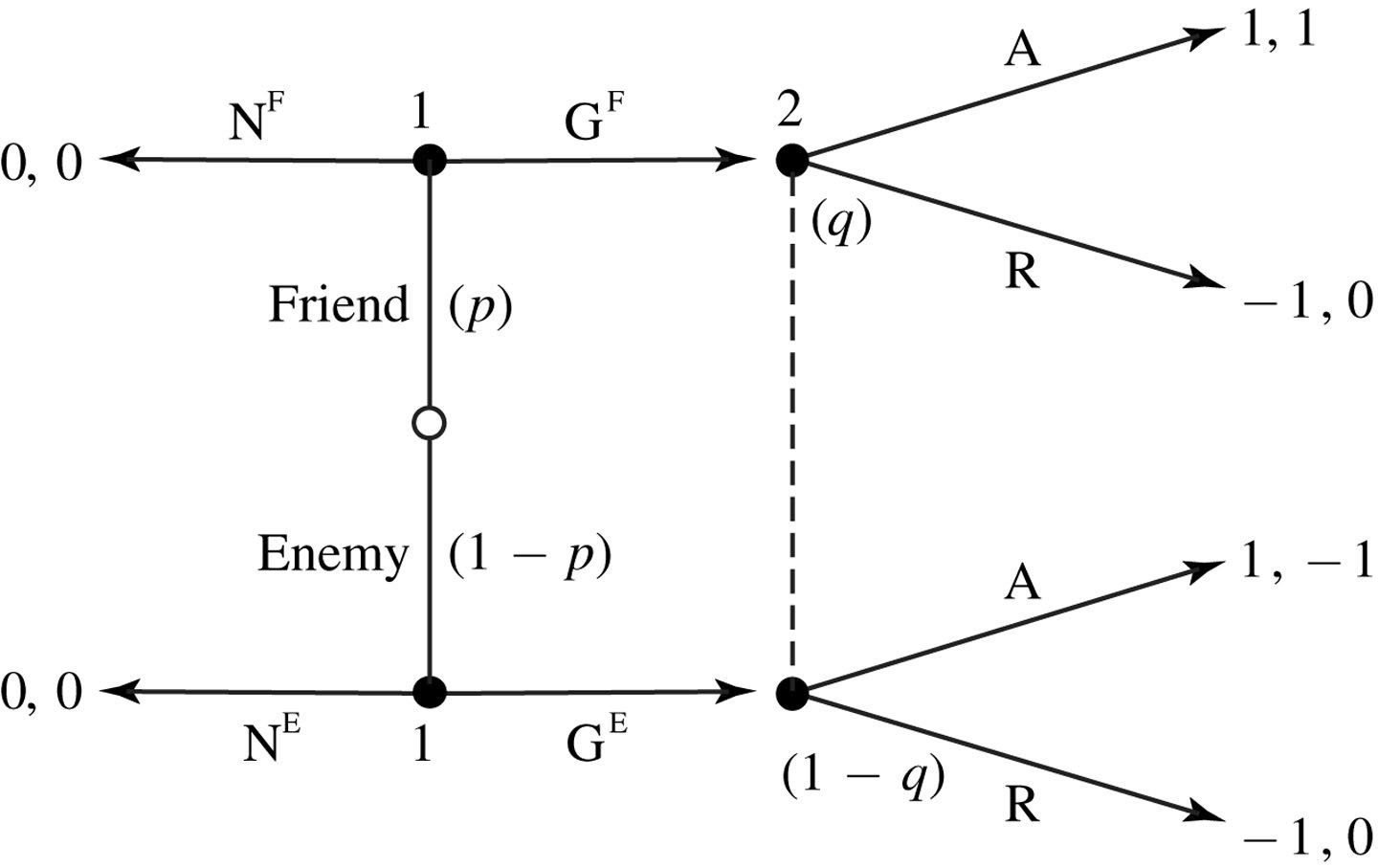
"Gift Giving Game"
Nature determines whether player 1 is a "friend" or "enemy" to player 2.
Player 1, knowing their type, can decide to give a gift to player 2 or not.
If player 1 gives a gift, player 2 can choose to accept it or not. Player 2 wants to accept a gift from a friend, but not from an enemy.
Whenever a player reaches an information set, they have some updated beliefs over which node they are.
Based on these beliefs, they should choose the action that maximizes their expected payoff.

"Gift Giving Game"
Nature determines whether player 1 is a "friend" or "enemy" to player 2.
Player 1, knowing their type, can decide to give a gift to player 2 or not.
If player 1 gives a gift, player 2 can choose to accept it or not. Player 2 wants to accept a gift from a friend, but not from an enemy.

"Gift Giving Game"
Nature determines whether player 1 is a "friend" or "enemy" to player 2.
Player 1, knowing their type, can decide to give a gift to player 2 or not.
If player 1 gives a gift, player 2 can choose to accept it or not. Player 2 wants to accept a gift from a friend, but not from an enemy.
In equilibrium, players' beliefs should be consistent with the strategies being played.
What is \(q\) if player 1 plays \(G^FN^E\)?
What is \(q\) if player 1 plays \(N^FG^E\)?
What is \(q\) if player 1 plays \(G^FG^E\)?
What is \(q\) if player 1 plays \(N^FN^E\)?
Separating and Pooling Equilibria
Separating Equilibrium: Each type of informed player chooses differently,
thereby conveying information about their type to the uninformed player
Pooling Equilibrium: Each type of informed player chooses the same,
thereby leaving the uninformed player with their prior belief.
Steps for calculating perfect Bayesian equilibria: Guess and Check!
- Start with a strategy for player 1 (pooling or separating).
- If possible, calculate updated beliefs (q in the example) by using Bayes’ rule.
In the event that Bayes’ rule cannot be used, you must arbitrarily select an updated belief; here you will generally have to check different potential values for the updated belief with the next steps of the procedure. - Given the updated beliefs, calculate player 2’s optimal action.
- Check whether player 1’s strategy is a best response to player 2’s strategy.
If so, you have found a PBE.
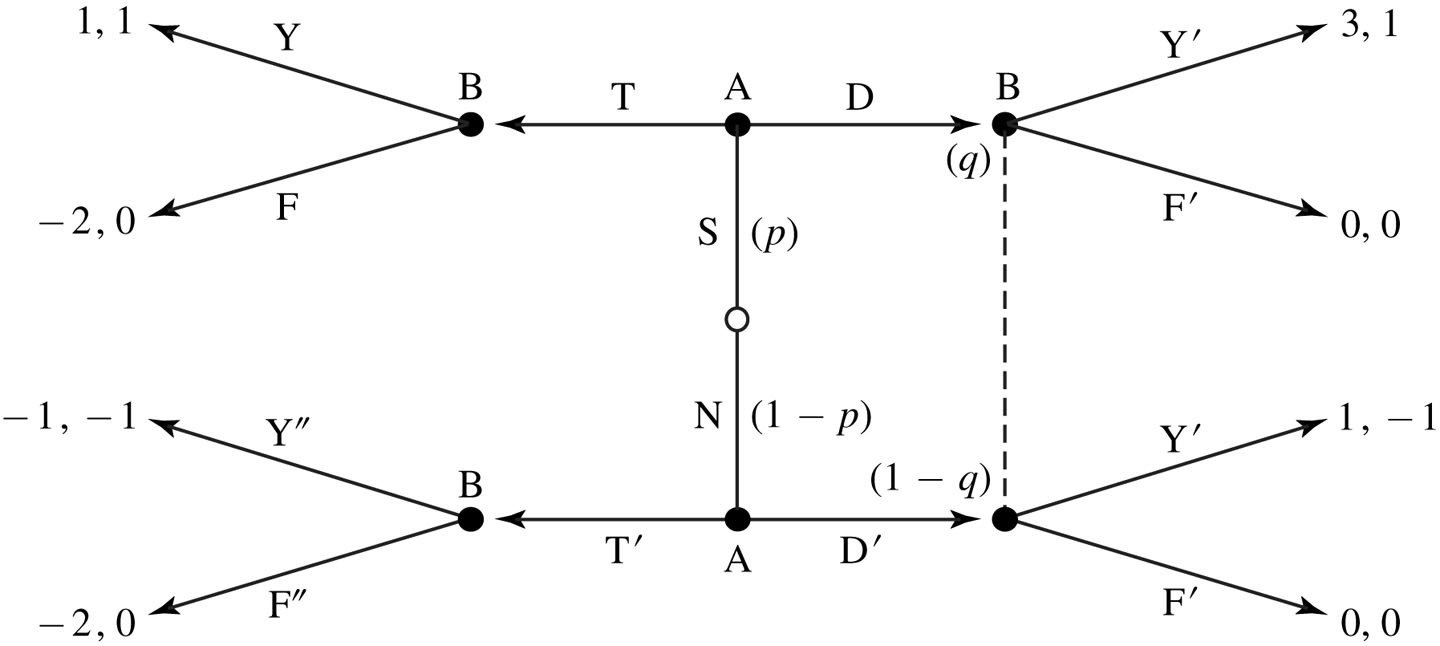
Guided Exercise from Watson (p. 385)
There are two types of workers: "high-ability" and "low-ability."
High-ability workers
are worth \(y_H\) to a firm
Low-ability workers
are worth \(y_L\) to a firm
Assume both firms and high-ability workers would be better off if firms could observe their ability.
Need some mechanism to create a separating equilibrium.

Job Market Signaling
Econ 51 | 15 | Dynamic Games of Incomplete Information
By Chris Makler
Econ 51 | 15 | Dynamic Games of Incomplete Information
Perfect Bayesian Equilibrium and Signaling Models
- 689



