pollev.com/chrismakler
Introduction to
Game Theory
Christopher Makler
Stanford University Department of Economics
Econ 51: Lecture 8
- Motivation: why game theory?
- Overview of the next 6 weeks
- Components of a game
- The extensive form (ch. 2)
- Strategies and the normal form (ch. 3)
- Beliefs, mixed strategies, and expected payoffs (ch. 4)
Today's Agenda
- Up until now: agents only (really) interact with "the market" via prices
- In real life, people, firms, countries ("players") interact with each other.
- Our economic lives are interconnected: our well-being doesn't depend only on our own actions, but on the actions taken by others
- Questions:
- OPTMIZATION: How do you operate in a world like this?
- EQUILIBRIUM: What is our notion of "equilibrium" in a world like this, and how is it different from competitive equilibrium?
- POLICY: Given how people behave in strategic settings, how can we design "mechanisms" to achieve policy goals?
Motivation
- The branch of economics that studies strategic interactions between economic agents.
- Everyone's payoffs depend on the actions chosen by all agents
- To "play the game," each agent thinks strategically about how the other agents are playing
Game Theory
-
Industrial organization: situations where a few firms dominate the market,
and each firm's decisions affect others -
Political economy: campaigning, governing, international diplomacy,
provision of public goods - Contract negotiations: incentive structures, credible threats, negotiating over price
- Interpersonal relationships: team dynamics, division of chores within a family
Applications
-
Week 5: Analyzing a Game from the Player's Point of View
- Today: Notation and terminology [Watson Ch. 1-5]
- Thursday: Dominance, best response, rationalizability [Watson Ch. 6-7]
-
Week 6: Equilibrium in a One-Shot Game
- Tuesday: Nash equilibrium [Watson Ch. 9-10]
- Thursday: Application to imperfect competition [Watson Ch. 11]
-
Week 7: Dynamic Games
- Tuesday: Dynamic games & subgame perfection [Watson Ch. 14-15]
- Thursday: Repeated games & collusion [Watson Ch. 22-23]
Next Three Weeks:
Games of Complete & Perfect Information
- Players: who is playing the game?
- Actions: what can the players do at different points in the game?
- Information: what do the players know when they act?
- Outcomes: what happens, as a function of all players' choices?
- Payoffs: what are players' preferences over outcomes?
Components of a Game
- Outcomes: what happens, as a function of all players' choices?
- Payoffs: what are players' preferences over outcomes?
1
2
1
1
,
0
0
,
1
1
,
0
0
,
Left
Right
Left
Right
1
2
Left
Right
Left
Right
Both OK
Both OK
Crash
Crash
Outcomes
Two bikers approach on an unmarked bike path.
Payoffs
i = \text{Player }i
-i = \text{Player(s) other than }i
\text{Example: Consider a strategy profile for four players }s = (s_1, s_2, s_3, s_4)
\text{If we consider player 2, then }s_i = s_2 \text{ and } s_{-i}=(s_1, s_3, s_4)
u_i(s_i,s_{-i}) = \text{Player $i$'s utility from playing $s_i$ when others play $s_{-i}$}
Notation Convention
The Extensive Form
(Watson, Chapter 2)
Extensive Form
Nodes:
Branches:
Initial node: where the game begins
Decision nodes: where a player makes a choice; specifies player
Terminal nodes: where the game ends; specifies outcome
Individual actions taken by players; try to use unique names for the same action (e.g. "left") taken at different times in the game
Information sets:
Sets of decision nodes at which the decider and branches are the same, and the decider doesn't know for sure where they are.
A "tree" representation of a game.
Example: Gift-Giving
She chooses to give one of three gifts:
X, Y, or Z.
1
X
Y
Z
Player 1 makes the first move.
Initial node
Player 1's actions at her decision node
(and decision node)
Example: Gift-Giving
Twist: Gift X is unwrapped,
but Gifts Y and Z are wrapped.
(Player 1 knows what they are,
but player 2 does not.)
After each of player 1's moves,
player 2 has the move: she can either accept the gift or reject it.
2
Accept X
Reject X
2
1
X
Y
Z
We represent this by having an information set connecting
player 2's decision nodes
after player 1 chooses Y or Z.
2
2
Player 2's actions
Player 2's decision nodes
Information set
Accept Y
Reject Y
Accept Z
Reject Z
Also: player 2 cannot make her action contingent on Y or Z; her actions must be "accept wrapped" or "reject wrapped"
Accept Wrapped
Reject Wrapped
Accept Wrapped
Reject Wrapped
Example: Gift-Giving
After player 2 accepts or rejects the gift, the game ends (terminal nodes) and payoffs are realized.
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
Accept X
Reject X
Accept Wrapped
Reject Wrapped
Accept Wrapped
Reject Wrapped
Terminal Nodes
Player 1's payoffs
Player 2's payoffs
In this game, both players get a payoff of
0 if any gift is rejected,
1 if gift X is accepted, and
2 if gift Y is accepted.
If gift Z is accepted, player 1 gets a payoff of 3, but player 2 gets a payoff of –1.
Strategies and the Normal Form
(Watson, Chapter 3)
Strategies and Strategy Spaces
A strategy is a complete, contingent plan of action for a player in a game.
This means that every player
must specify what action to take
at every decision node in the game tree!
A strategy space is the set of all strategies available to a player.
Strategies & Strategy Spaces
Player 1 has a single decision:
which gift to give (X, Y, or Z).
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
Accept X
Reject X
Accept Wrapped
Reject Wrapped
Accept Wrapped
Reject Wrapped
Player 2 might have to make one of two decisions: accept or reject gift X,
and accept or reject a wrapped gift.
Let's abbreviate these as A/R and A'/R'.
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
Then player 2's strategy space is
Therefore player 1's strategy space is
Strategy Profiles
A strategy profile \(s = (s_1,s_2)\) is a vector showing which strategy from their strategy space is chosen by each player.
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
s_1 = Z
s_2 = AR'
The outcome of this is that gift Z is given and rejected, and both players receive a payoff of 0.
\text{Example: }s = (Z, AR')
Note: the strategy profile specifies which action is taken at every decision node!
Notation
Strategy for player \(i\):
Strategy space for player \(i\):
S_1 = \{X,Y,Z\}
s_1 = Z
Strategy profile:
s_i
S_i
(s_1, s_2, ..., s_n)
S_2 = \{AA', AR', RA', RR'\}
s_2 = AR'
(\ \ \ ,\ \ \ \ \ \ \ )
Z
AR'
(a complete, contingent plan for how player \(i\) will move)
(set of all possible strategies for player \(i\))
(list of strategies chosen by each player \(i = 1,2,...,n\))
Player 1's Strategy Space:
Player 2's Strategy Space:
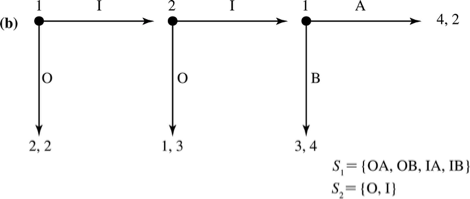
pollev.com/chrismakler
How many strategies does player 1 have
in her strategy space?
Player 1's Strategy Space:
Player 2's Strategy Space:
Player 1's Strategy Space:
Player 3's Strategy Space:
Player 2's Strategy Space:
Continuous Strategies
Strategy for player \(i\):
Strategy space for player \(i\):
S_1 = \mathbb{R}_+
q_1 = 6
Strategy profile:
s_i
S_i
(q_1, q_2)
S_2 = \mathbb{R}_+
q_2 = 3
(\ \ \ ,\ \ \ )
6
3
(set of all possible strategies for player \(i\))
(list of strategies chosen by each player \(i\))
Payoffs for both players, as a function of what strategies are played
\pi_1(q_1,q_2) = 12q_1 - q_1^2 - q_1q_2
\pi_2(q_1,q_2) = 12q_2 - q_2^2 - q_1q_2
Suppose two firms each simultaneously choose a quantity \(q_i\) to produce.
Normal-Form Game
List of players: \(i = 1, 2, ..., n\)
Strategy spaces for each player, \(S_i\)
Payoff functions for each player \(i: u_i(s)\),
where \(s = (s_1, s_2, ..., s_n)\) is a strategy profile
listing each player's chosen strategy.
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
\(X\)
\(AA'\)
1
2
\(AR'\)
\(RA'\)
\(RR'\)
\(Y\)
\(Z\)
Normal Form Representation
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
\(X\)
\(AA'\)
1
2
\(AR'\)
\(RA'\)
\(RR'\)
\(Y\)
\(Z\)
0
0
,
Normal Form Representation
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
\(X\)
\(AA'\)
1
2
\(AR'\)
\(RA'\)
\(RR'\)
\(Y\)
\(Z\)
0
0
,
3
–1
,
Normal Form Representation
1
0
1
0
2
0
2
0
3
0
–1
0
2
2
1
X
Y
Z
,
,
,
,
,
,
A
R
S_1 = \{X,Y,Z\}
S_2 = \{AA', AR', RA', RR'\}
A'
R'
A'
R'
\(X\)
\(AA'\)
1
2
\(AR'\)
\(RA'\)
\(RR'\)
\(Y\)
\(Z\)
1
1
,
1
1
,
0
0
,
0
0
,
2
2
,
0
0
,
2
2
,
0
0
,
3
–1
,
0
0
,
3
–1
,
0
0
,
Normal Form Representation
Normal Form Representation
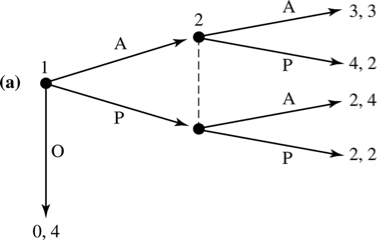
\(OA\)
\(I\)
1
2
\(O\)
\(OB\)
\(IA\)
\(IB\)
2
2
,
2
2
,
4
2
,
3
4
,
2
2
,
2
2
,
1
3
,
1
3
,
Normal Form Representation
\(A\)
\(C\)
1
2
\(D\)
\(B\)
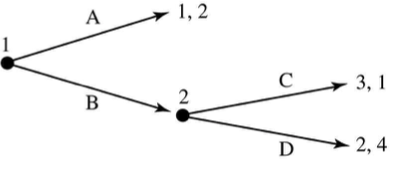
\(A\)
\(C\)
1
2
\(D\)
\(B\)
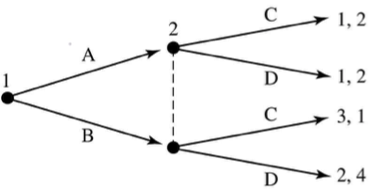
1
2
,
3
1
,
1
2
,
2
4
,
3
1
,
2
4
,
1
2
,
1
2
,
Relationship between Normal-Form
and Extensive-Form Games
Both include the players, strategies, and payoffs.
The extensive form also includes information
on timing and information.
We usually use the normal form for
static (simultaneous-move) games of complete information.
Different extensive forms might have the same normal form.
Mixed Strategies, Beliefs, and Expected Payoffs
(Watson, Chapter 4)
Mixed Strategy
- Play one element of your strategy space
with probability 1, others with probability 0 - Example: "Play heads" or "play tails"
Pure Strategy
- Place positive probability on more than one element of your strategy space
- Example: "Flip a coin and play whatever comes up on top."
Equilibria with mixed strategies are sometimes the only equilibrium!
- Your probability distribution over another player's strategies
- Represents the probability you believe they'll play each strategy (for whatever reason)
Beliefs
- Your probability distribution over your own strategies.
- Represents the probability with which you intend to play each strategy
Mixed Strategies
A
B
X
Y
1
2
5
4
5
0
0
4
4
4
Mixed strategy for player 1:
probability distribution
over {A, B}
Belief for player 1:
probability distribution over {X, Y}
2
Expected Payoffs
\({1 \over 6}\)
\({1 \over 3}\)
\({1 \over 2}\)
\(0\)
Player 1's beliefs
\({1 \over 6} \times 6 + {1 \over 3} \times 3 + {1 \over 2} \times 2 + 0 \times 7\)
\(=3\)
Player 1's expected payoffs from each of their strategies
\(X\)
\(A\)
1
\(B\)
\(C\)
\(D\)
\(Y\)
\(Z\)
6
6
,
3
6
,
2
8
,
7
0
,
12
6
,
6
3
,
0
2
,
5
0
,
6
0
,
0
9
,
6
8
,
11
4
,
Your expected payoff from playing one of your strategies
is the weighted average of the payoffs, weighted by your beliefs about what the other person is playing
2
\({1 \over 6}\)
\({1 \over 3}\)
\({1 \over 2}\)
\(0\)
Player 1's beliefs
\(X\)
\(A\)
1
\(B\)
\(C\)
\(D\)
\(Y\)
\(Z\)
6
6
,
3
6
,
2
8
,
7
0
,
12
6
,
6
3
,
0
2
,
5
0
,
6
0
,
0
9
,
6
8
,
11
4
,
pollev.com/chrismakler
Given these beliefs, what is player 1's expected payoff from playing Y?
Expected Payoffs
2
Your expected payoff from playing one of your strategies
is the weighted average of the payoffs, weighted by your beliefs about what the other person is playing
Expected Payoffs
\({1 \over 6}\)
\({1 \over 3}\)
\({1 \over 2}\)
\(0\)
Player 1's beliefs
\({1 \over 6} \times 6 + {1 \over 3} \times 3 + {1 \over 2} \times 2 + 0 \times 7\)
\(=3\)
\({1 \over 6} \times 12 + {1 \over 3} \times 6 + {1 \over 2} \times 0 + 0 \times 5\)
\(=4\)
\({1 \over 6} \times 6 + {1 \over 3} \times 0 + {1 \over 2} \times 6 + 0 \times 11\)
\(=4\)
Player 1's expected payoffs from each of their strategies
\(X\)
\(A\)
1
\(B\)
\(C\)
\(D\)
\(Y\)
\(Z\)
6
6
,
3
6
,
2
8
,
7
0
,
12
6
,
6
3
,
0
2
,
5
0
,
6
0
,
0
9
,
6
8
,
11
4
,
\(X\)
\(A\)
1
2
\(B\)
\(C\)
\(D\)
\(Y\)
\(Z\)
6
6
,
3
6
,
2
8
,
7
0
,
12
6
,
6
3
,
0
2
,
5
0
,
6
0
,
0
9
,
6
8
,
11
4
,
If you are playing a mixed strategy, and the other player is playing a pure strategy, your expected payoff is the weighted average given the way you are mixing.
Expected Payoffs
\({1 \over 6}\)
\({1 \over 3}\)
\({1 \over 2}\)
\(0\)
Player 2's strategy
\({1 \over 6} \times 6 + {1 \over 3} \times 6 + {1 \over 2} \times 8 + 0 \times 0\)
\(=7\)
\({1 \over 6} \times 6 + {1 \over 3} \times 3 + {1 \over 2} \times 2 + 0 \times 0\)
\(=3\)
\({1 \over 6} \times 0 + {1 \over 3} \times 9 + {1 \over 2} \times 8 + 0 \times 4\)
\(=7\)
Player 2's expected payoffs given each of 1's strategies
This Unit: Complete & Perfect Information
Complete Information
Perfect Information
All players, strategies, and payoffs are common knowledge.
(Everyone knows what game they are playing, and all relevant attributes of the other players in the game.)
All players know all events in a sequential game which have previously occurred,
including random events.
Next Steps
- Thursday: Analyzing a single player's optimal behavior
- There are sections this week
- The homework this week sets up the homework for next week. Recommend doing all questions (they're pretty short)
Econ 51 | 08 | Introduction to Game Theory
By Chris Makler
Econ 51 | 08 | Introduction to Game Theory
Notation and definitions
