A Competitive Firm's
Labor Demand
Christopher Makler
Stanford University Department of Economics
Econ 50 | Lecture 21
We'll stick with our same function
f(L,K) = \sqrt{LK}
\overline K = 32
L(q) = {q^2 \over K}
MP_L = \frac{1}{2}\sqrt{K \over L}
L(q) = {q^2 \over 32}
MP_L = \sqrt{8 \over L}
f(L) = \sqrt{32L}
This Week's Agenda
Review of firm profit maximization, with and without market power
Edge cases
Deriving output supply
as a function of \(p\),
holding \(w\) constant
Deriving labor demand
as a function of \(w\),
holding \(p\) constant
Analyzing how a change in \(w\)
shifts the supply curve
Analyzing how a change in \(p\)
shifts the labor demand curve
[ MOVEMENT
ALONG CURVES]
[ SHIFTS OF
CURVES]
Wednesday
Friday
Are people paid their marginal value?
c(q) = 64 + {1 \over 4}q^2
r(q) = pq = 12q
\text{Let's assume }p = 12:
\text{Profit function is:}
\text{Total cost}
\pi(q) = 12q - [64 + {1 \over 4}q^2]
\text{Take derivative with respect to } q \text{ and set equal to zero:}
\pi'(q) = \ \ \ \ \ \ - \ \ \ \ \ \ = 0
12
{1 \over 2}q
Price
MC
\(q\)
$/unit
P = MR
12
MC = {1 \over 2}q
24
\Rightarrow q^* = 24
Key insight for today: the profit-maximizing choice of \(q\) is also a profit-maximizing choice of \(L\).
\pi = pq - (wL + rK)
The production function tells us the relationship between labor and output:
q = f(L)
L = L^c(q)
q = \sqrt{L \overline K}
L = {q^2 \over \overline K}
Profit is total revenues minus total costs:
e.g.
We can write this profit in terms of either choice variable:
\pi(q) = pq - (wL^c(q) + r \overline K)
\pi(L) = pf(L) - (wL + r \overline K)
CONDITIONAL DEMAND FOR LABOR
Profit as a function of quantity
Profit as a function of labor
1. Costs and Revenues
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
\pi(q) = 12q - (64 + \tfrac{1}{4}q^2)
\pi^\prime(q) = 12 - {1 \over 2}q = 0
r(L) = p \times f(L) = 12 \sqrt{32L}
1. Costs and revenues
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
\pi(L) = 12\sqrt{32L} - (8L + 64)
\pi^\prime(L) = 12 \times \sqrt{8 \over L} - 8 = 0
q^* = 24
L^* = 18
Profit two ways when \(p = 12\), \(w = 8\), \(r = 2\), and \(\overline K = 32\)
c(q) = wL(q) + r\overline K = {1 \over 4}q^2 + 64
r(q) = pq = 12q
c(L) = wL + r\overline K = 8L + 64
PROFIT-MAXIMIZING OUTPUT CHOICE
PROFIT-MAXIMIZING INPUT CHOICE
MP_L = \tfrac{2}{3}
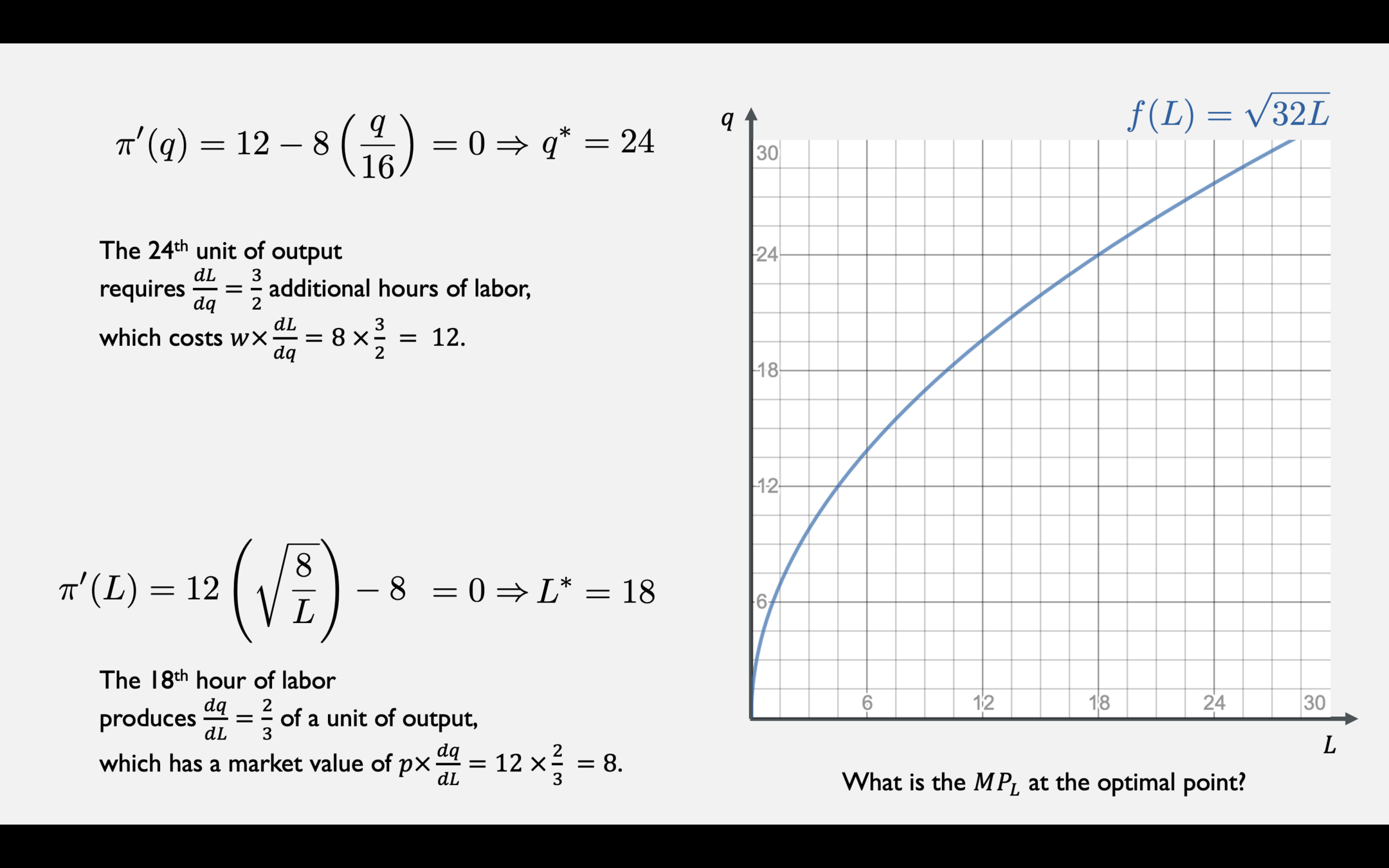
When price is fixed at 12
For a general price
1. Costs and Revenues
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
\pi(q) = 12q - (64 + \tfrac{1}{4}q^2)
\pi^\prime(q) = 12 - {1 \over 2}q = 0
r(q) = pq
\pi(q) = pq - (64 + \tfrac{1}{4}q^2)
\pi^\prime(q) = p - {1 \over 2}q = 0
q^* = 24
q^*(p) = 2p
Profit-Maximizing Output Choice when \(w = 8\), \(r = 2\), and \(\overline K = 32\)
c(q) = wL(q) + r\overline K = {1 \over 4}q^2 + 64
r(q) = pq = 12q
c(q) = wL(q) + r\overline K = {1 \over 4}q^2 + 64
NUMBER
FUNCTION
Labor Demand as a Function of \(w\) with Fixed \(p\)
When wage is fixed at 8
For a general wage
1. Costs and Revenues
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
Profit-Maximizing Labor Choice when \(p = 12\), \(r = 2\), and \(\overline K = 32\)
NUMBER
FUNCTION
r(L) = p \times f(L) = 12 \sqrt{32L}
\pi(L) = 12\sqrt{32L} - (wL + 64)
\pi^\prime(L) = 12 \times \sqrt{8 \over L} - w = 0
L^*(w) = {1152 \over w^2}
c(L) = wL + r\overline K = wL + 64
r(L) = p \times f(L) = 12 \sqrt{32L}
\pi(L) = 12\sqrt{32L} - (8L + 64)
\pi^\prime(L) = 12 \times \sqrt{8 \over L} - 8 = 0
L^* = 18
c(L) = wL + r\overline K = 8L + 64
f(L,K) = \sqrt{LK}, \overline K = 32
wL + 64
w
12\sqrt{32L}
\pi(L) = \ \ \ \ \ \ \ \ \ \ \ \ \ - [\ \ \ \ \ \ \ \ \ \ \ \ \ \ ]
\pi^\prime(q) = \ \ \ \ \ \ \ \ \ \ - \ \ \ \ = 0
12\sqrt{8 \over L}
TR
TC
MRPL
MC
Take derivative and set = 0:
Solve for \(L^*\):
L^*(w) = {1152 \over w^2}
PROFIT-MAXIMIZING
LABOR DEMAND FUNCTION
Output Supply and Labor Demand for General \(p\) and \(w\)
Profit as a function of output \(q\)
Profit as a function of labor \(L\)
c(q) = {wq^2 \over \overline K} + r \overline K
1. Costs for general \(w\) and revenue for general \(p\)
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
\pi(q) = pq - [{wq^2 \over 32} + 32r]
\pi^\prime(q) = p - {wq \over 16} = 0
r(L) = p\sqrt{32L}
\pi(L) = p\sqrt{32L} - [wL + 32r]
\pi^\prime(L) = p \times \sqrt{8 \over L} - w = 0
q^*(p\ |\ w) = {16 p \over w}
L^*(w\ |\ p) = {8p^2 \over w^2}
\text{Profit }\pi = pq - [wL + rK]
r(q) = p \times q
c(L) = wL + r \overline K
PROFIT-MAXIMIZING OUTPUT SUPPLY
PROFIT-MAXIMIZING LABOR DEMAND
f(L,K) = \sqrt{LK}, \overline K = 32
L^*(w\ |\ p) = 8 \left({p \over w}\right)^2
PROFIT-MAXIMIZING
LABOR DEMAND FUNCTION
q^*(p\ |\ w) = {16p \over w}
SUPPLY FUNCTION
the conditional labor demand
for the profit-maximizing supply:
L^*(w\ |\ p) = L^c(q^*(p\ |\ w))
L^c(q) = {1 \over 32} q^2
= {1 \over 32}\left(16p \over w\right)^2
= 8\left(p \over w\right)^2
The profit-maximizing labor demand is
CONDITIONAL LABOR DEMAND FUNCTION
Profit as a function of output \(q\)
Profit as a function of labor \(L\)
c(q) = wL(q) + r \overline K
1. Costs for general \(w\) and revenue for general \(p\)
2. Profit = total revenues minus total costs
3. Take derivative of profit function, set =0
\pi(q) = pq - [wL(q) + r\overline K]
\pi^\prime(q) = p - w \times {dL \over dq} = 0
r(L) = p \times f(L)
\pi(L) = p\times f(L) - [wL + r\overline K]
\pi^\prime(L) = p \times {dq \over dL} - w = 0
p = w \times {dL \over dq}
p \times {dq \over dL} = w
\text{Profit }\pi = pq - [wL + rK]
r(q) = p \times q
c(L) = wL + r \overline K
[cost of labor required for \(q\) units of output]
[revenue of output produced by \(L\) hours of labor]
MARGINAL COST (MC)
MARGINAL REVENUE PRODUCT OF LABOR (MRPL)
"Keep producing output as long as the marginal revenue from the last unit produced is at least as great as the marginal cost of producing it."
"Keep hiring workers as long as the marginal revenue from the output of the last worker is at least as great as the cost of hiring them."
p = MC
w = MRP_L
Profit as a function of output \(q\)
Profit as a function of labor \(L\)
p = w \times {1 \over MP_L}
w = p \times MP_L
What does this model tell us?
A competitive firm takes input prices \(w\) and \(r\), and the output price \(p\), as given.
We can therefore characterize its optimal choices of inputs and outputs
as functions of those prices: the supply of output \(q^*(p\ |\ w)\),
and the demand for inputs (e.g. \(L^*(w\ |\ p)\)).
We can find the optimal input-output combination either by finding the optimal quantity of output and determining the inputs required to produce it, or to find the profit-maximizing inputs and determine the resulting output. These two methods are equivalent.
Profit is increasing when marginal revenue is greater than marginal cost, and vice versa.
In most cases, the profit-maximizing choice occurs where \(MR = MC\).
If \(p\) is below the minimum value of AVC, the profit-maximizing choice is \(q = 0\).
In which MR or MC is discontinuous, logic must be applied. (There is an old exam question on the homework that explores this...and this kind of thing often shows up on exams...)
Is it true?
Are people paid the value of their marginal product?
What determines the value of people's work?
What should?
What determines how much people are paid?
Econ 50 | Lecture 21
By Chris Makler
Econ 50 | Lecture 21
Output Supply and Labor Demand for a Competitive Firm
