A Solution, a Clicker, and the Zero Product Property Walk into a Bar...

Anurag Katyal

Professor I, Mathematics

Palm Beach State College, FL

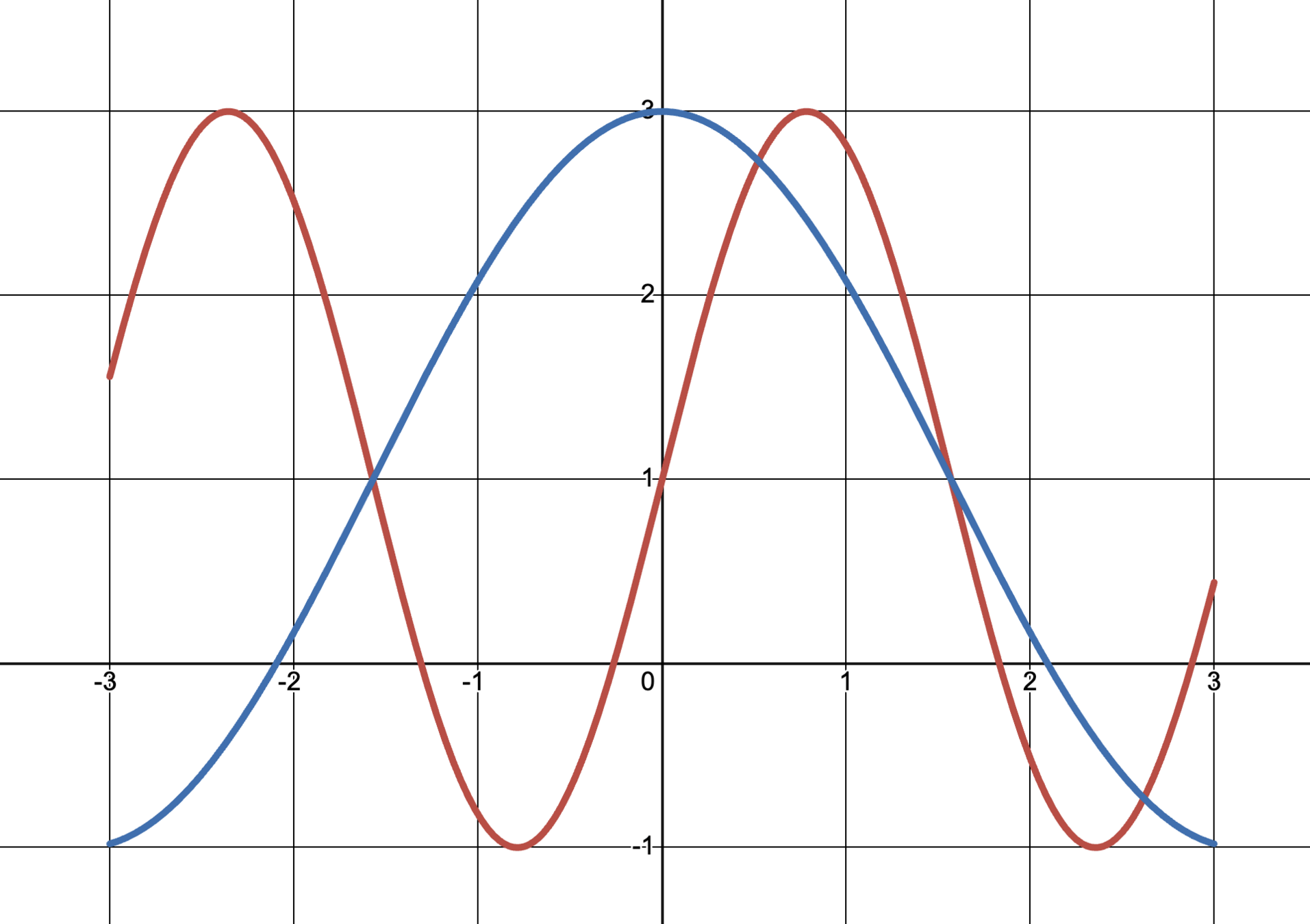
Q1. How many solutions does the above system of equations have in \([-3,3]\)?

Q2. Find the value(s) of \(k\) such that the line \(5 x+y=-9\) is tangent to the graph of \(f(x)=2 x^2+k x-1\).

Heat

Oxygen

A (Potential) Solution

The Serendipitous Fire Triangle of Math™

(circa Summer 2023)

Fuel

Head

Head

Body

Tail

Body

Tail

Tail

Body

Tail

Reorder content and Delivery

Week 1, Day 2

Week 15, Day 1

The Problem(s)

Head

Head

Body

Tail

Body

Tail

Tail

Body

Tail

Reorder content and Delivery

Team BAsed Inquiry Learning

• Permanent teams selected by the instructor

• Students held accountable through Readiness Assurance Process

• Students receive frequent and immediate feedback

• Teams work collaboratively on activities.

Students Collaborate
(while i listen)

Hands must remain in pockets (As much as possible)

TBIL Activities

• Free and open-source interactive activities

• Immediate feedback in class

• Open-ended response

• Try different approaches

Collaborative Research: Empowering faculty to run online learning experiments

So...what exactly is a solution?

The Activity

What do students think?

• 4 Intermediate Algebra sections (76 students)
• Week 1, Fall 2023 - "What do you think it means for a number to be a solution to an equation?"
• 8 out of 72 (~11%): "If you substitute a solution into an equation, it satisfies the equation, making the equation true."
• 12 out of 72 students (~17%) alluded to substituting a solution into an equation but did not expand further.

Intro/Test 1

• Week 1 - Students engage with Doenet activity and scored an average of 87.13%.
• Week 4 - Solve \(\color{blue}\frac{41}{9}=\frac{5}{2}\left(x+\frac{2}{3}\right)-\frac{1}{3} x\) for \(x\). Explain whether the number you found for \(x\) was a solution to the equation. 
• 17 out of 74 students (~23%) were awarded at least half credit.

Test 2

• Week 7 - Which of the following values are solutions to the inequality \(\color{blue}9 \leq 3 x+4\) ?
  I. \(\color{blue}-7\)                    II. \(\color{blue}8\)                    III. \(\color{blue}2\)
• Students encouraged but not required to revisit the activity from the start of the course. 
• 59 out of 74 students (~80%) were awarded at least half credit.

Test 3

• Week 11 - Solve \(\color{blue}\frac{n}{n+3}+\frac{1}{n-4}=\frac{6-5 n}{n^2-n-12}\) for \(n\).  
• Initially asked to work on this problem independently.
• Without being prompted, 36 out of 51 students (~71%) verified \(n=1\) was the only solution to the equation and also wrote an explanation.

Final Exam

• Week 15 - Solve \(\color{blue}\sqrt{2 x+2}=3+\sqrt{2 x-1}\) for \(x\).  
• 33 out of 50 students (66%) correctly identified the equation has no solutions. 4 more students solved the question correctly and verified the candidates but did not explain their findings.
• Historically, maximum of ~25% with semesters with at least 65 students and average of ~19%. 
• Passing rates went up 20% from 35% to 55%.

〞

I personally liked Doenet activities because it helped me get hands on learning as a group. The way the course was set up allowed me to open up in ways I haven’t before and also hear my peers talk. It also broke down steps that helped answer complex questions later in the semester.

– Intermediate Algebra student

〞

I hate this man so much. He could have just taught in a normal way.

\(100\%\)

(n=6)

Let's take a walk...

Acknowledgements

• Doenet (NSF Award # 1915363)
  • PI - Duane Nykamp, Uni. of Minnesota
  • Doenet Learning Experiment Mini-Grant
  • www.doenet.org
• Team Based Inquiry Learning (NSF Award # 2011807)
  • PI - Steven Clontz, Uni. of South Alabama
  • www.tbil.org

www.pitimespi.com

Sabbatical until May 2025.

Please reach out if you have ideas or suggestions!