Causal Dynamical Triangulations with CGAL
Adam Getchell
acgetchell@ucdavis.edu
University of California, Davis
C++Now 2016
Causal Dynamical Triangulations
A candidate theory of quantum gravity
(Not )
Why is this hard?
(In English)
Everything else is simpler at short distances.
Gravity is more complicated.
- No local observables
- Mass is really only defined at the horizon
- Energy is not conserved
- Time is a problem
- No universal clock
- Closed timelike curves possible
And a bunch of other highly entertaining issues ...
Does CDT have a Newtonian Limit?
CDT looks like GR at cosmological scales, does it have a Newtonian limit?
At first glance, this is hard:
- CDT is not well-suited for approximating smooth classical space-times
- We don't have the time or resolution to watch objects fall
Path Integral
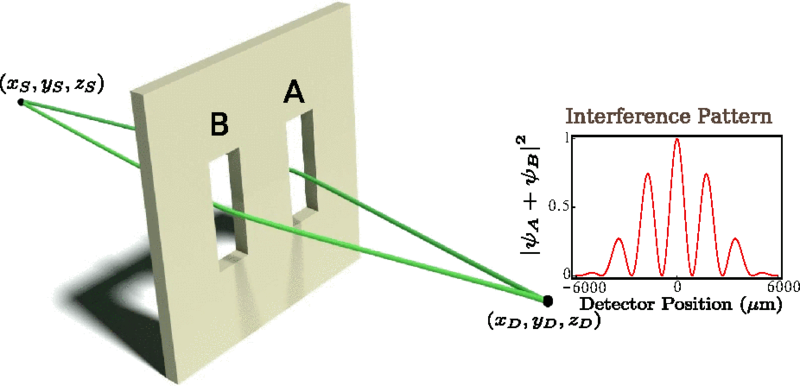
Path Integral
Equations of Motion
Ricci scalar
Cosmological constant
Ricci tensor
Ricci scalar
Stress-Energy tensor
Transition probability amplitude
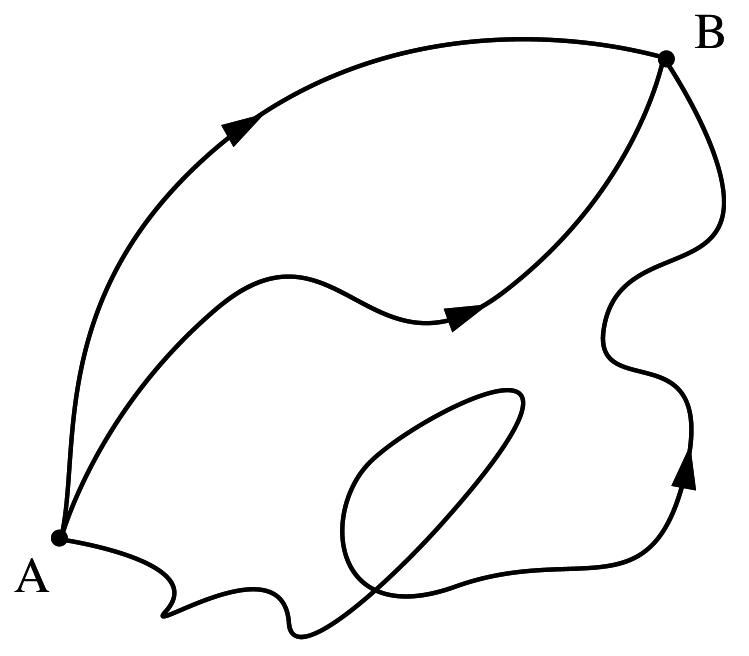
Calculating the Path Integral
Quickly gets complicated!


DT Path Integral
Inequivalent Triangulations
Regge Action
Partition Function
Transition probability amplitude
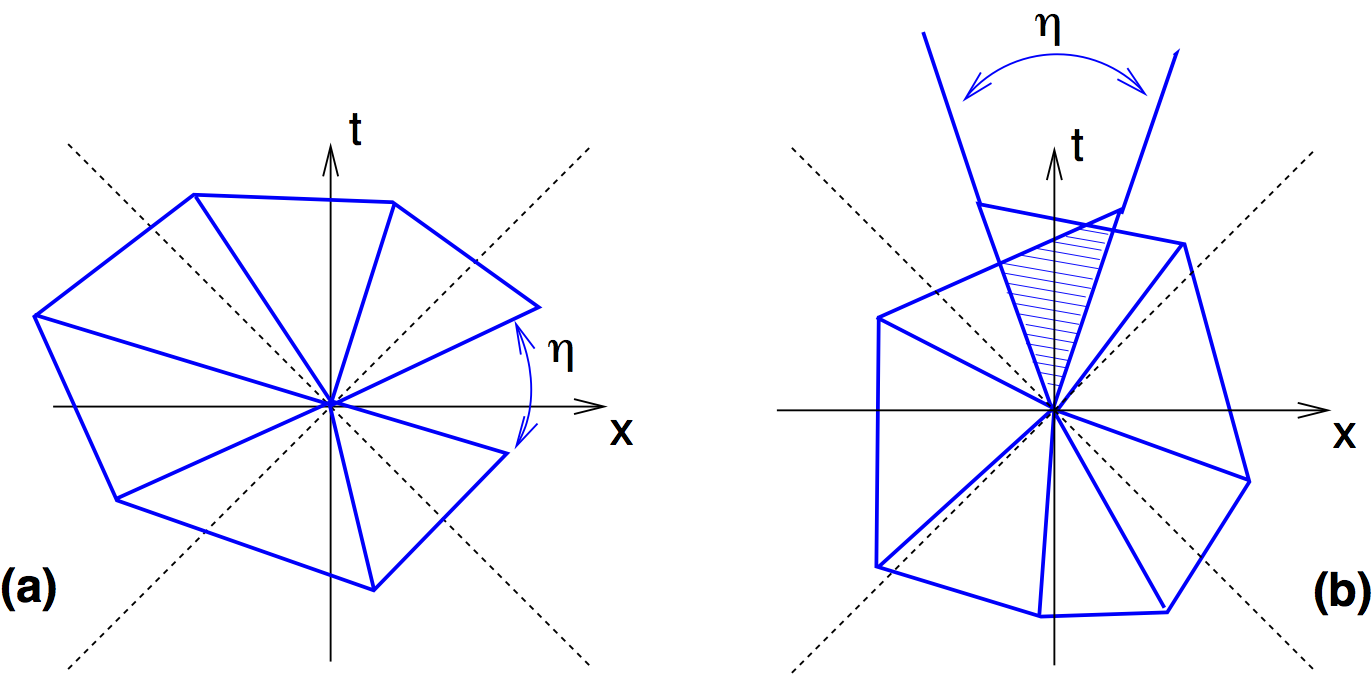
Now What?

Foliation
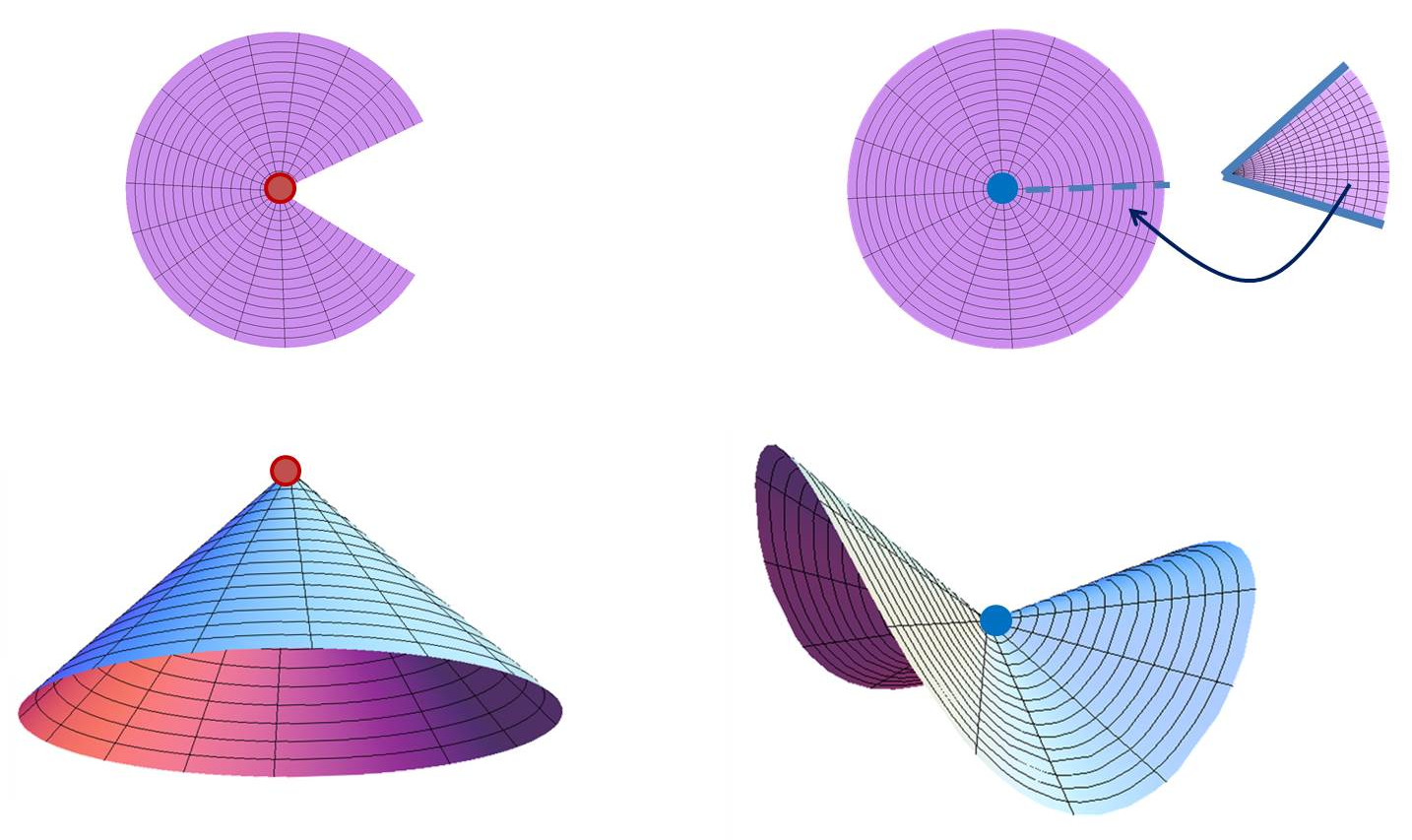
CDT Path Integral
CDT Path Integral
Wick rotation
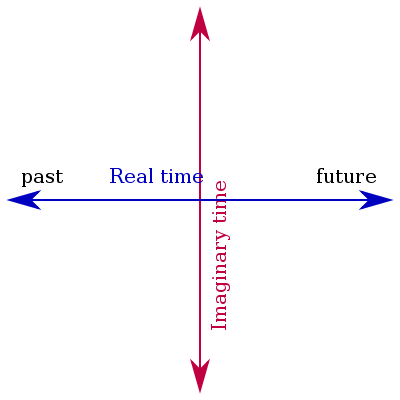
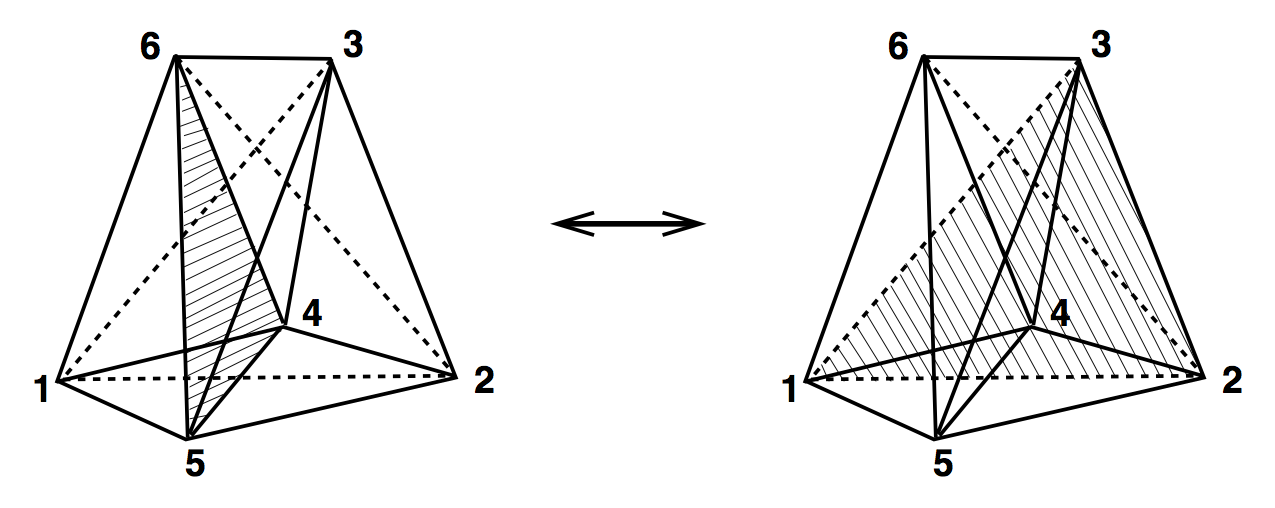
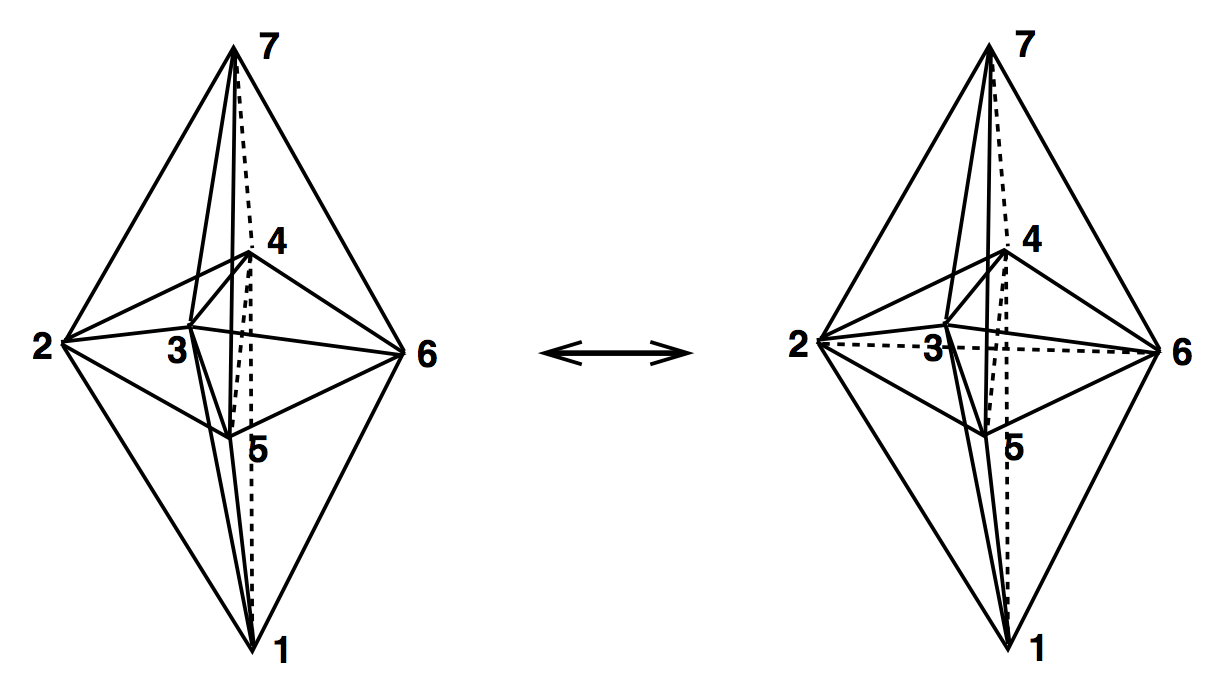
What is an inequivalent triangulation?
3D Ergodic Moves
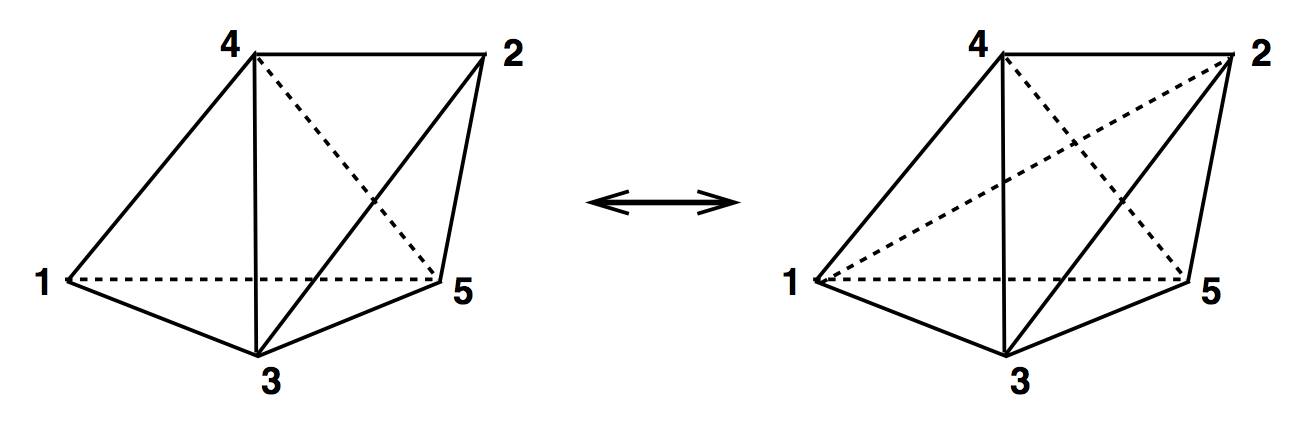

(2,3) & (3,2)
(4,4)
(2,6) & (6,2)
Simplices involved
Move name
(3,1) & (2,2)
2 (1,3) & 2 (3,1)
(1,3) & (3,1)
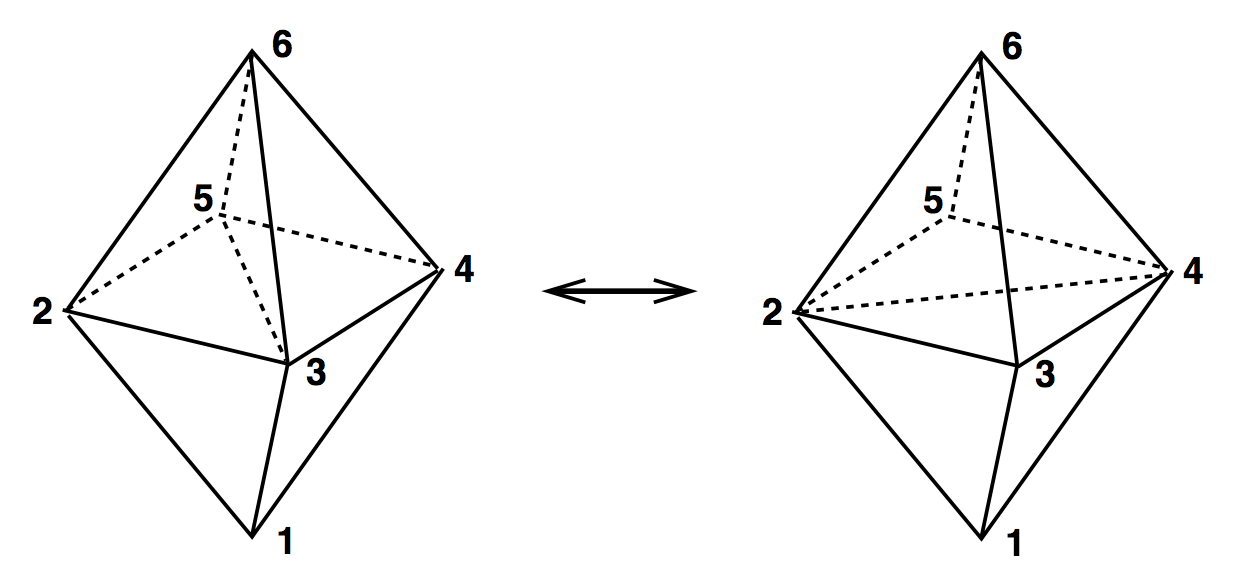
4D Ergodic Moves
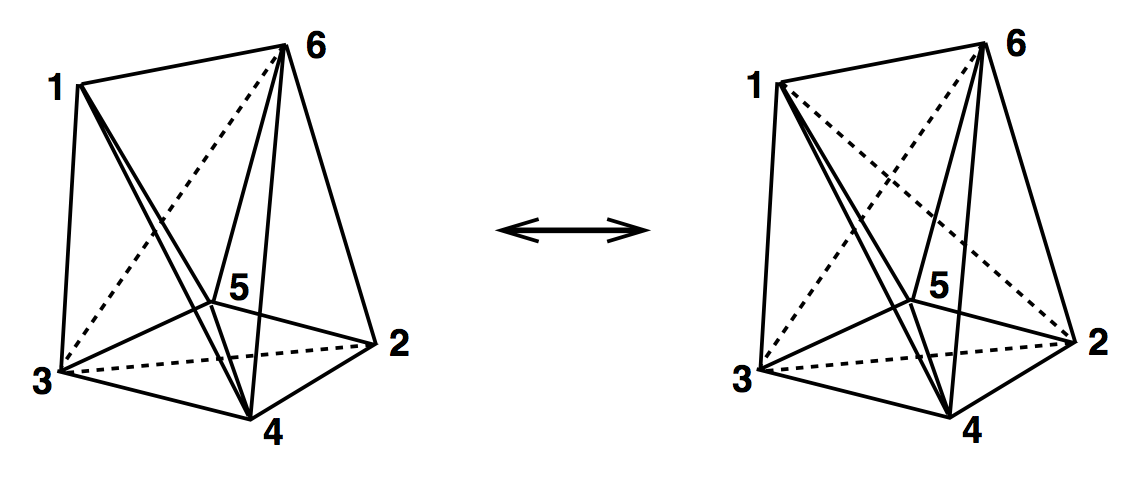
(2,4) & (4,2)
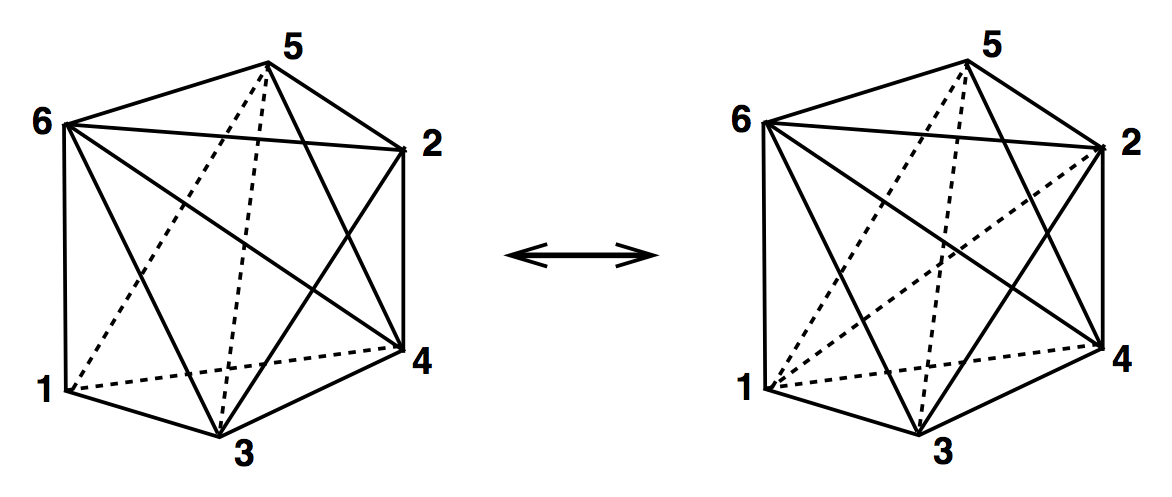
(3,3)
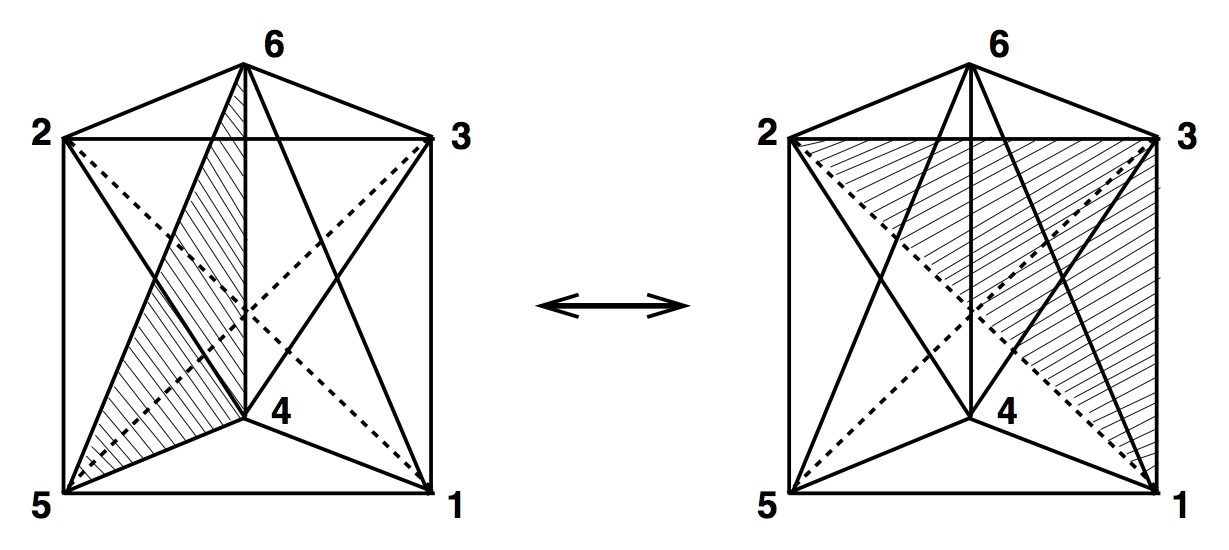
(4,6) & (6,4)
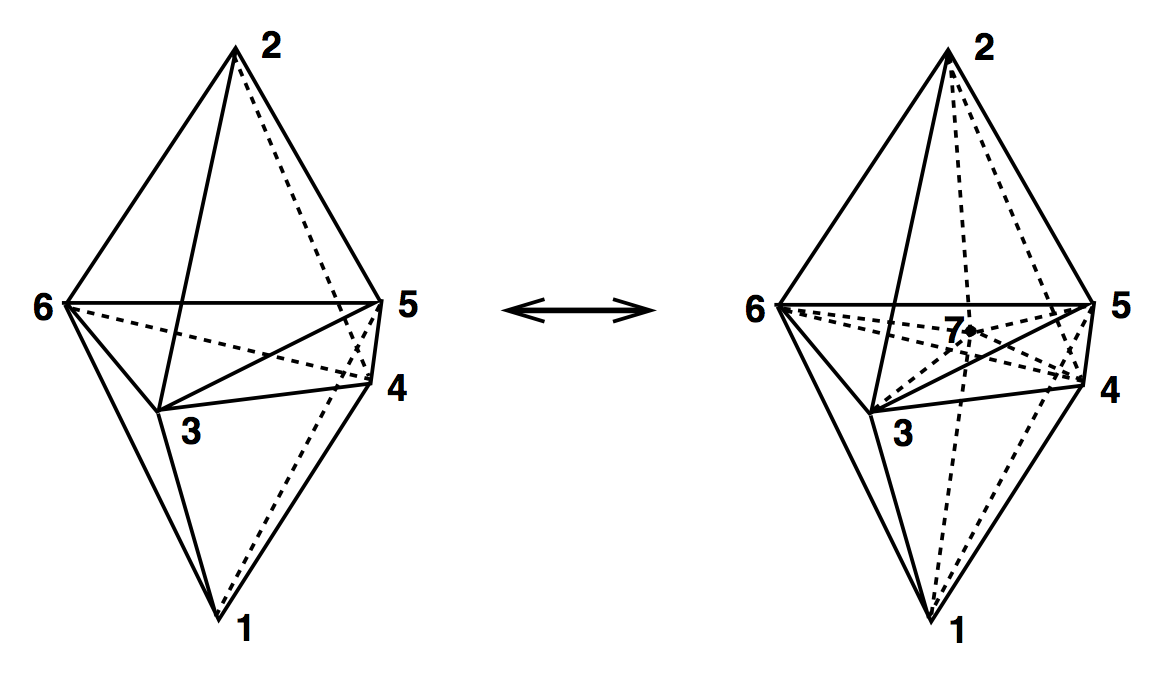
(2,8) & (8,2)
Metropolis-Hastings
Inequivalent Triangulations
Partition Function
Wick rotation
- Pick an ergodic (Pachner) move
- Make that move with a probability of a1a2, where:
CDT, Kommu et. al.
- First independent confirmation of results
- Written in Lisp
- 256 timeslices, 512K simplices about 6 months on an HPC cluster
- Lots and lots of data files
Find the Newtonian Limit, if it exists
- Test case, no limit in 3D
Re-implement CDT
- Rewrite in modern C++
- Use well-known libraries
Use current tools
- LLVM/Clang
- Hosted on GitHub
- Continuous integration with Travis-CI
- C++ Core Guidelines
- Google Style Guide
- GoogleTest/GoogleMock
- CLion
- CMake for cross-platform building
- Literate programming with Doxygen
- Others (Gitter, Ninja, etc.)
Easy to evaluate, use, and contribute
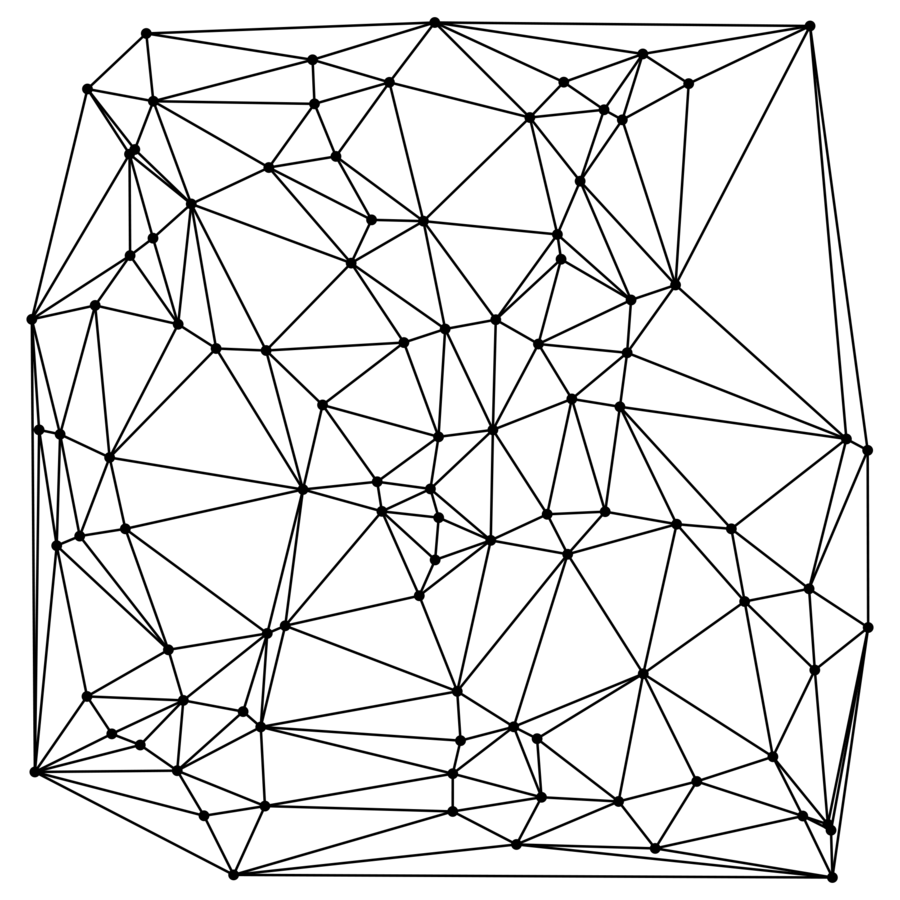
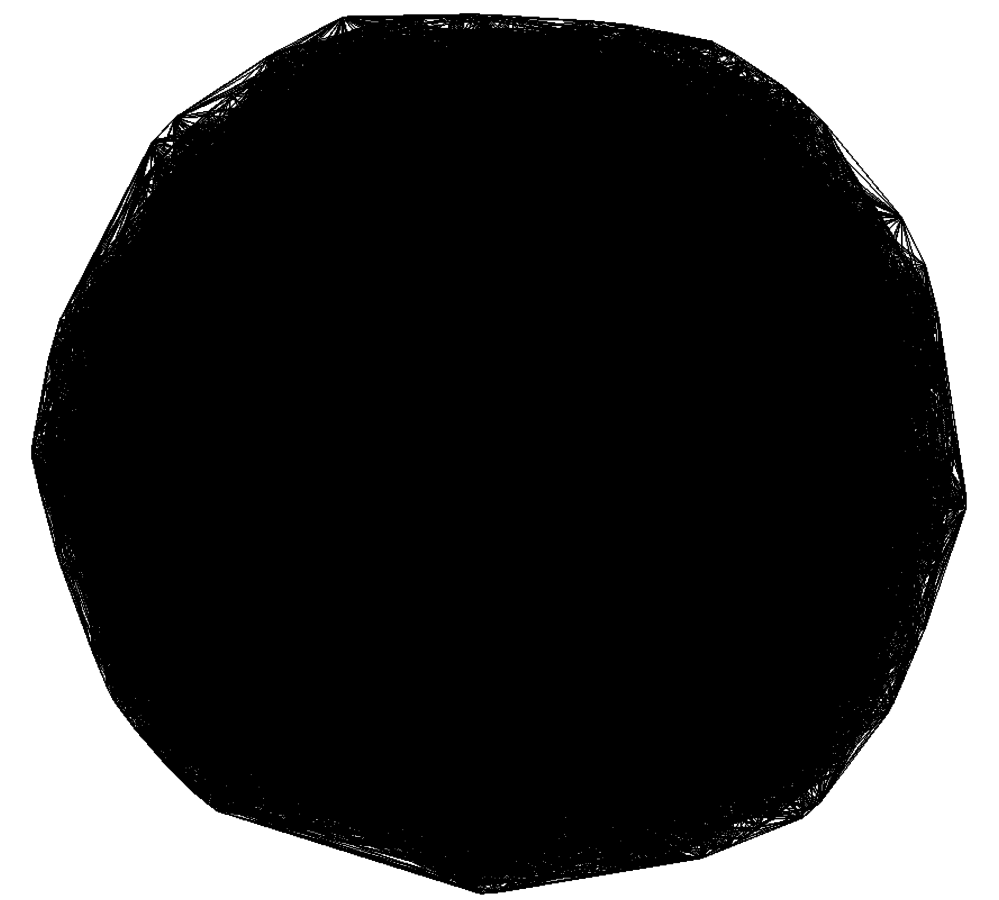
Fast foliated Delaunay Triangulations in CGAL

Fast foliated Delaunay Triangulations in CGAL
256 timeslices, 222,132 vertices, 2,873,253 faces, 1,436,257 simplices
Creation time: 284.596s
(MacBook Pro Retina, Mid 2012)
Remaining Work
Interested? Please Join!
Thank You!
[1] The CGAL Project. CGAL User and Reference Manual. CGAL Editorial Board, 4.6.3 edition, 2015.
[2] Steve Carlip. Why Quantum Gravity is Hard. Conceptual and Technical Challenges for Quantum Gravity, Rome, September 2014.
[3] J. Ambjorn, J. Jurkiewicz, and R. Loll. “Dynamically Triangulating Lorentzian Quantum Gravity.” Nuclear Physics B 610, no. 2001 (May 27, 2001): 347–82.
[4] Rajesh Kommu. “A Validation of Causal Dynamical Triangulations.” arXiv:1110.6875, October 31, 2011. http://arxiv.org/abs/1110.6875.
Mass = Epp quasilocal energy
- In 1+1 simplicial geometry, extrinsic curvature at a vertex is proportional to the number of connected triangles
- In 2+1 simplicial geometry, extrinsic curvature at an edge is proportional to the number of connected tetrahedra
- In 3+1 simplicial geometry, extrinsic curvature at a face is proportional to the number of connected pentachorons (4-simplices)

Non-Euclidean Distance
- Calculate single-source shortest path between the two masses using Bellman-Ford algorithm in O(VE)
- Modify allowed moves to not permit too many successive moves which increase or decrease distance between masses (hold relatively fixed)

Hausdorff Distance(?)
- Calculate Voronoi diagram of Delaunay triangulation
- Find minimal Hausdorff distance in diagram for sets in O((m+n)^6 log(mn)) (Huttenlocher, Kedem, and Kleinberg)
A Trick from GR
The static axisymmetric Weyl metric:
With two-body Chazy-Curzon (circa 1924) solutions of the Einstein field equations:
Leads to a strut:
With a stress:
That can be integrated to get the Newtonian force!
A Trick from GR
Metric
Affine connection
Riemann tensor
Ricci tensor & Ricci scalar
Einstein field equations
Causal Dynamical Triangulations with C++ and CGAL
By Adam Getchell
Causal Dynamical Triangulations with C++ and CGAL
- 420



