New strongly regular graphs from real line packings
John Jasper
Air Force Institute of Technology
International Conference on Approximation Theory and Beyond

The views expressed in this talk are those of the speaker and do not reflect the official policy
or position of the United States Air Force, Department of Defense, or the U.S. Government.
https://slides.com/johnjasper/icatb23/
special optimizers have nice repn's
nice repn's \(\Rightarrow\) rare graphs
Outline
find vectors maximally "spread out"
Background
Definition. Given unit vectors \(\Phi=(\varphi_{i})_{i=1}^{N}\), we define the coherence
\[\mu(\Phi) = \max_{i\neq j}|\langle \varphi_{i},\varphi_{j}\rangle|.\]
Measuring how "spread out" vectors are
\[\mu(\Phi) = \cos(\theta)\]
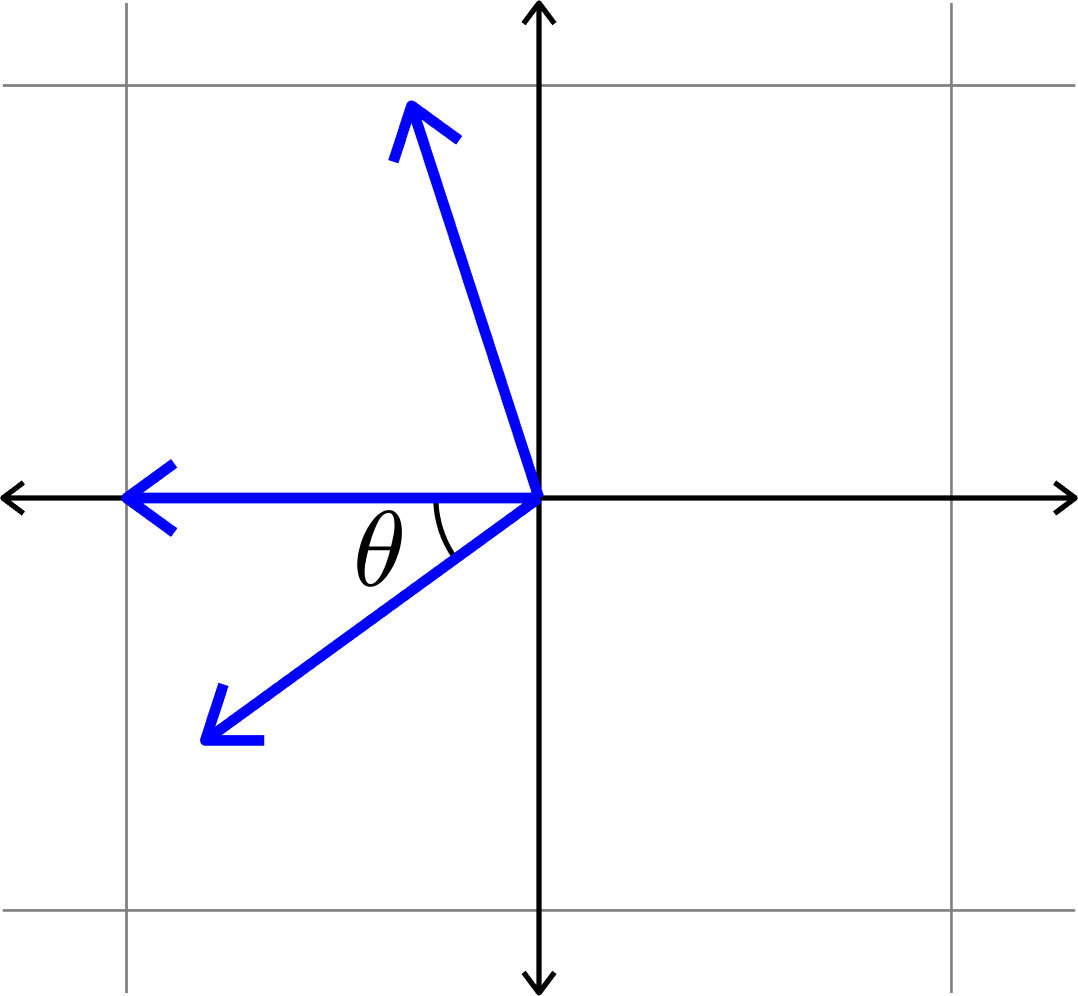
\(\mu(\Phi) = \cos(\theta)\)??

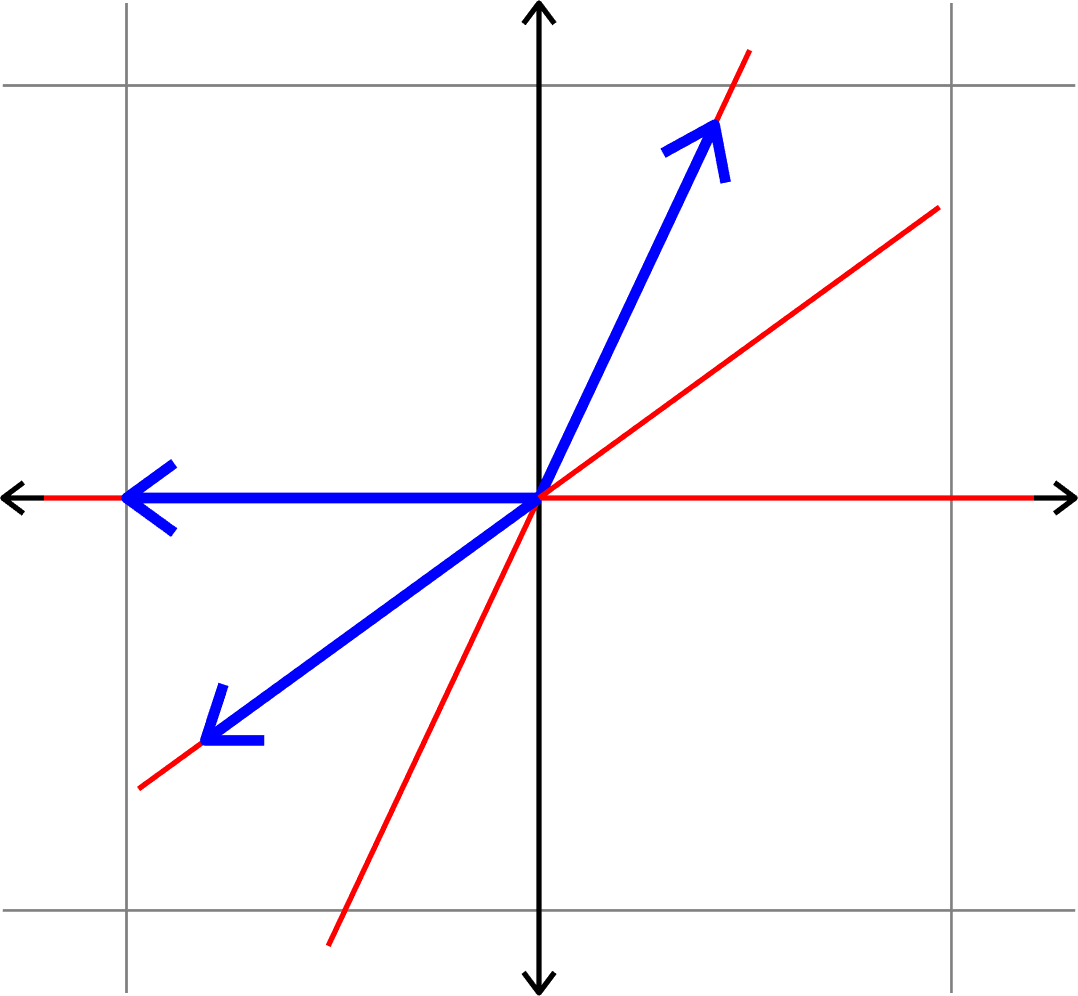

\(\mu(\Phi) = \cos(\theta)\)
Measuring how "spread out" vectors are
Definition. Given unit vectors \(\Phi=(\varphi_{i})_{i=1}^{N}\), we define the coherence
\[\mu(\Phi) = \max_{i\neq j}|\langle \varphi_{i},\varphi_{j}\rangle|.\]
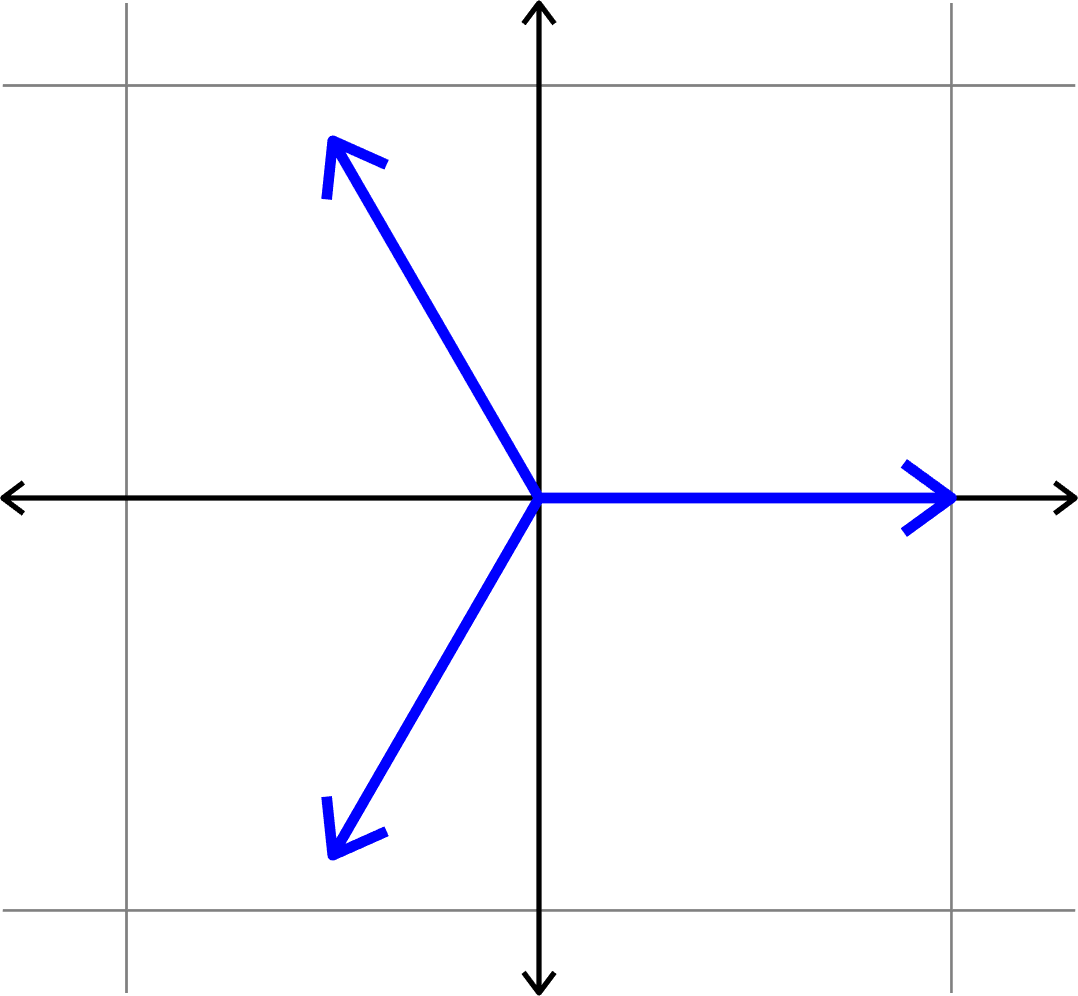
Example.

Measuring how "spread out" vectors are
Definition. Given unit vectors \(\Phi=(\varphi_{i})_{i=1}^{N}\), we define the coherence
\[\mu(\Phi) = \max_{i\neq j}|\langle \varphi_{i},\varphi_{j}\rangle|.\]
Given \((d,N)\) find \(\Phi = (\varphi_{i})_{i=1}^{N}\subset\mathbb{R}^{d}\) such that \(\mu(\Phi)\) is minimal.
Vectors that are as spread out as possible
Theorem (the Welch bound). For unit vectors \(\Phi=(\varphi_{i})_{i=1}^{N}\) in \(\mathbb{R}^d\)
\[\mu(\Phi)\geq \sqrt{\frac{N-d}{d(N-1)}}.\]
Equality holds if and only if both:
- Tight: There is a constant \(A>0\) such that \[\sum_{i=1}^{N}|\langle v,\varphi_{i}\rangle|^{2} = A\|v\|^{2} \quad\text{for all } v.\]
- Equiangular: There is a constant \(\alpha\) such that \[|\langle\varphi_{i},\varphi_{j}\rangle| = \alpha\quad\text{for all }i\neq j.\]
Welch bound equality \(\Longleftrightarrow\) equiangular tight frame (ETF)
ETF Gram matrix
Definition. Let \[\Phi = \big[\varphi_{1}\ \ \varphi_{2}\ \ \cdots\ \ \varphi_{N}\big]\in \mathbb{R}^{d\times N},\]
be a rank \(d\) matrix where each column \(\varphi_{n}\) is unit norm
\[\|\varphi_{n} \|^{2}=1.\]
1) (Tightness) \(\exists\,A>0\) such that \((\Phi^{\top}\Phi)^{2} = A\Phi^{\top}\Phi\).
2) (Equiangular) \(\exists\,B>0\) such that \(|\frac{1}{B}\varphi_{m}^{\top}\varphi_{n}^{}|=1\) for \(m\neq n\).
If both 1) and 2) hold, then \(\{\varphi_{n}\}_{n=1}^{N}\) is an ETF(\(d,N)\).
\[\Phi^{\top}\Phi = \left[\begin{array}{cccc} 1 & \varphi_{1}^{\top}\varphi_{2} & \cdots & \varphi_{1}^{\top}\varphi_{N}\\[1ex] \varphi_{2}^{\top}\varphi_{1} & 1 & \cdots & \varphi_{2}^{\top}\varphi_{N}\\[1ex] \vdots & \vdots & \ddots & \vdots\\[1ex] \varphi_{N}^{\top}\varphi_{1} & \varphi_{N}^{\top}\varphi_{2} & \cdots & 1\end{array}\right]\]
\(1\)'s down the diagonal
1) \(\Phi^{\top}\Phi \propto\) projection
2) \(|\varphi_{m}^{\top}\varphi_{n}^{}|\) constant
\(=\)
Steiner system
\(r\) ones per column
\(r\times (r+1)\) ETF with unimodular entries
"Steiner" ETF
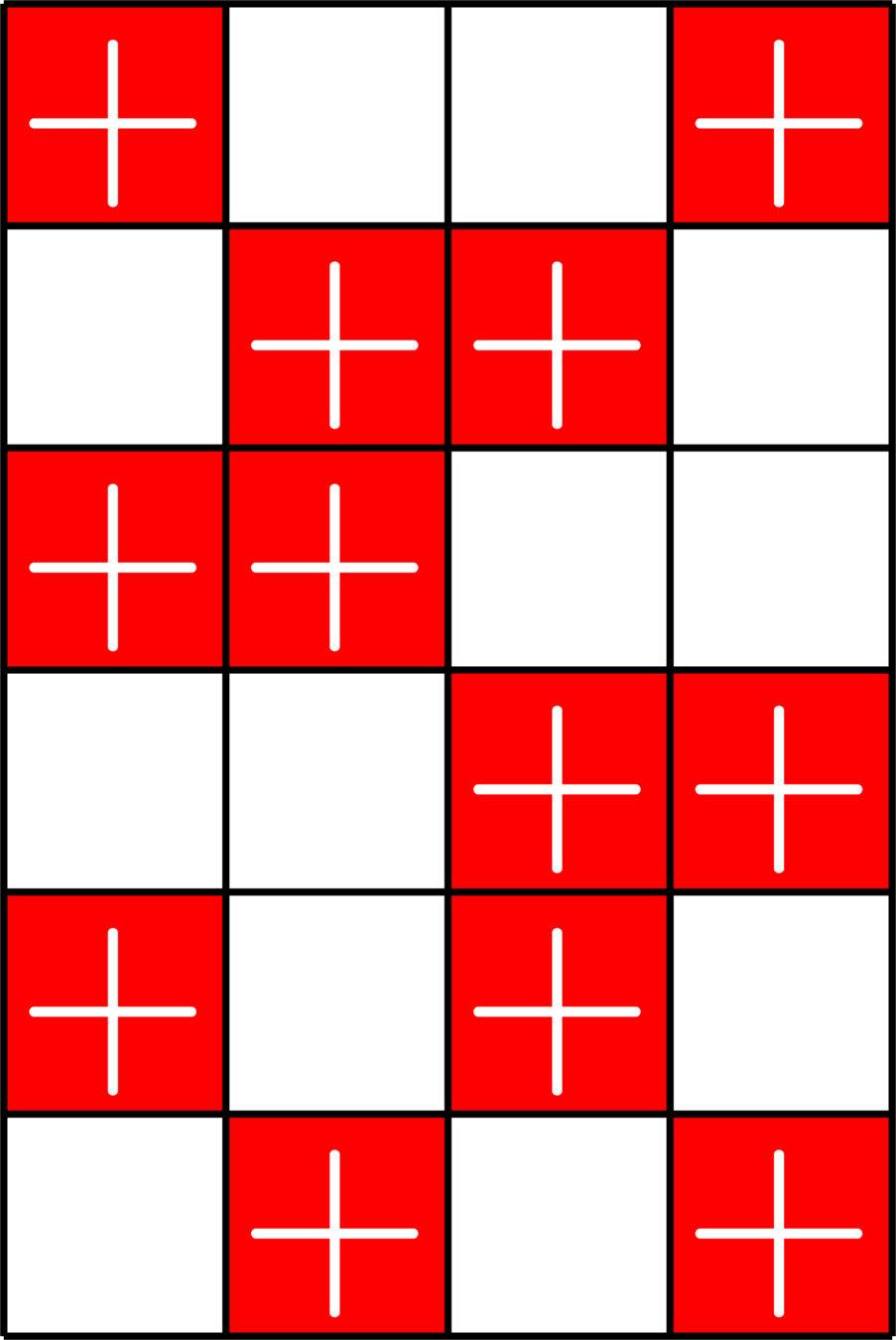
16 vectors in \(\mathbb{R}^{6}\)

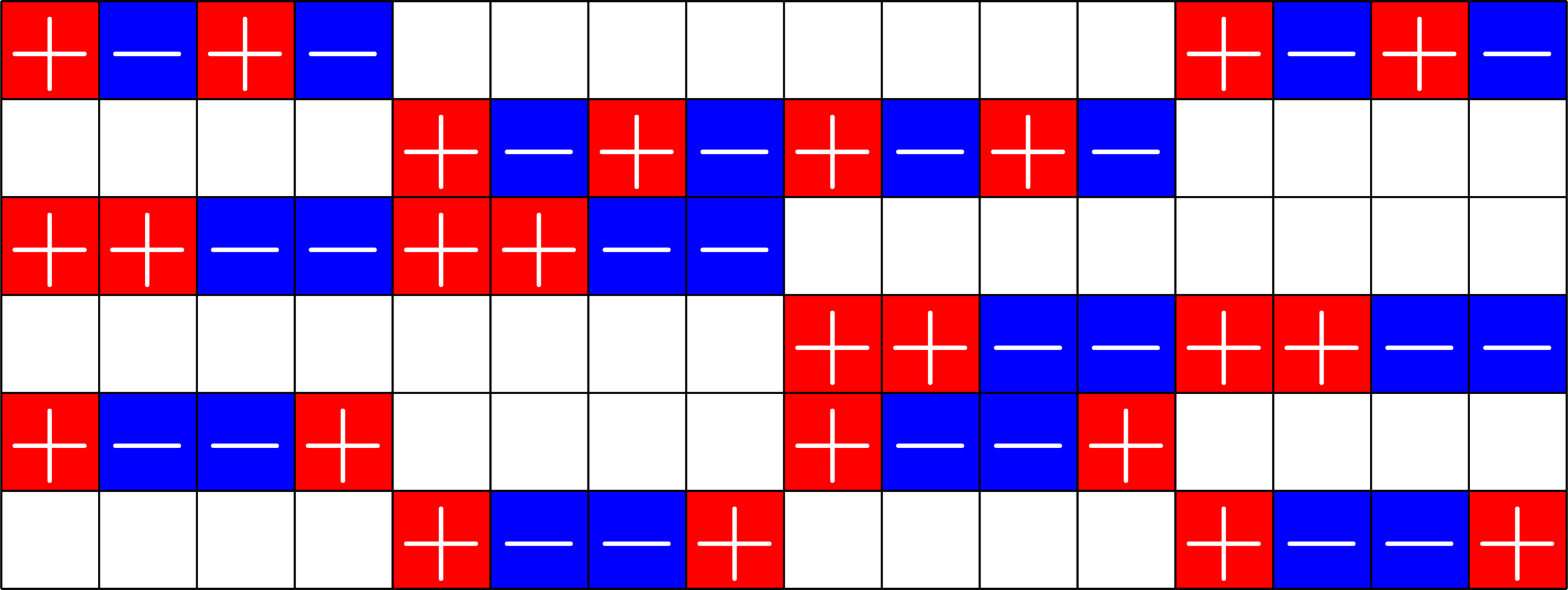
It's easy to go this way
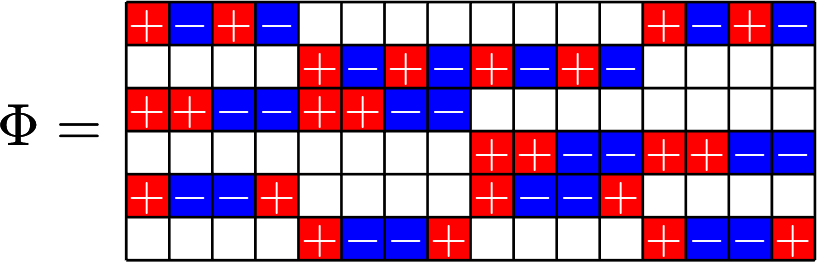
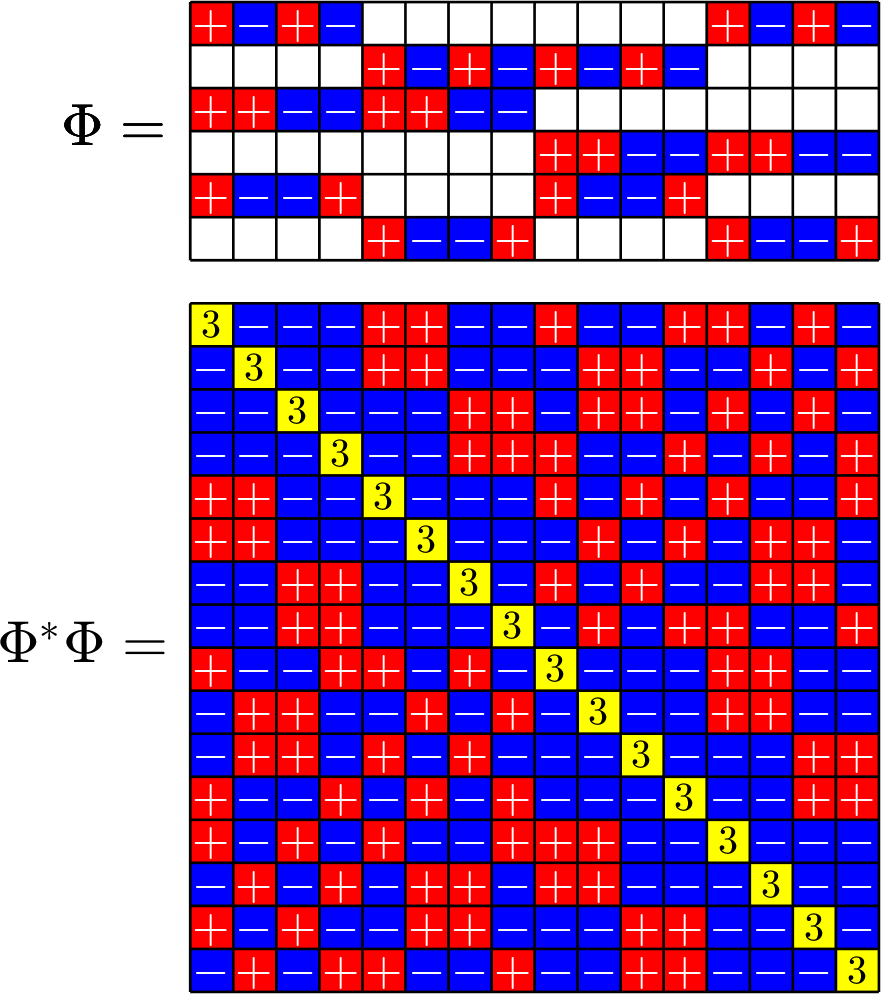
\(\Phi^{\top}\Phi=\)
\(\Phi=\)
Gramians are forgetful
Given \(\Phi^{\top}\Phi\),
\(\Phi\) is only determined up to a unitary


\(\Phi^{\top}\Phi=\)
\(\Phi=\)

Gram matrix of ETF:
Adjacency matrix of graph:
ETFs \(\Rightarrow\) Graphs
- Every vertex has 9 neighbors
- Adjacent vertices have 4 common neighbors
- Non-adjacent vertices have 6 common neighbors

Replace diagonal 1's with 0's
Replace -1's with 1's
Zero out the diagonal
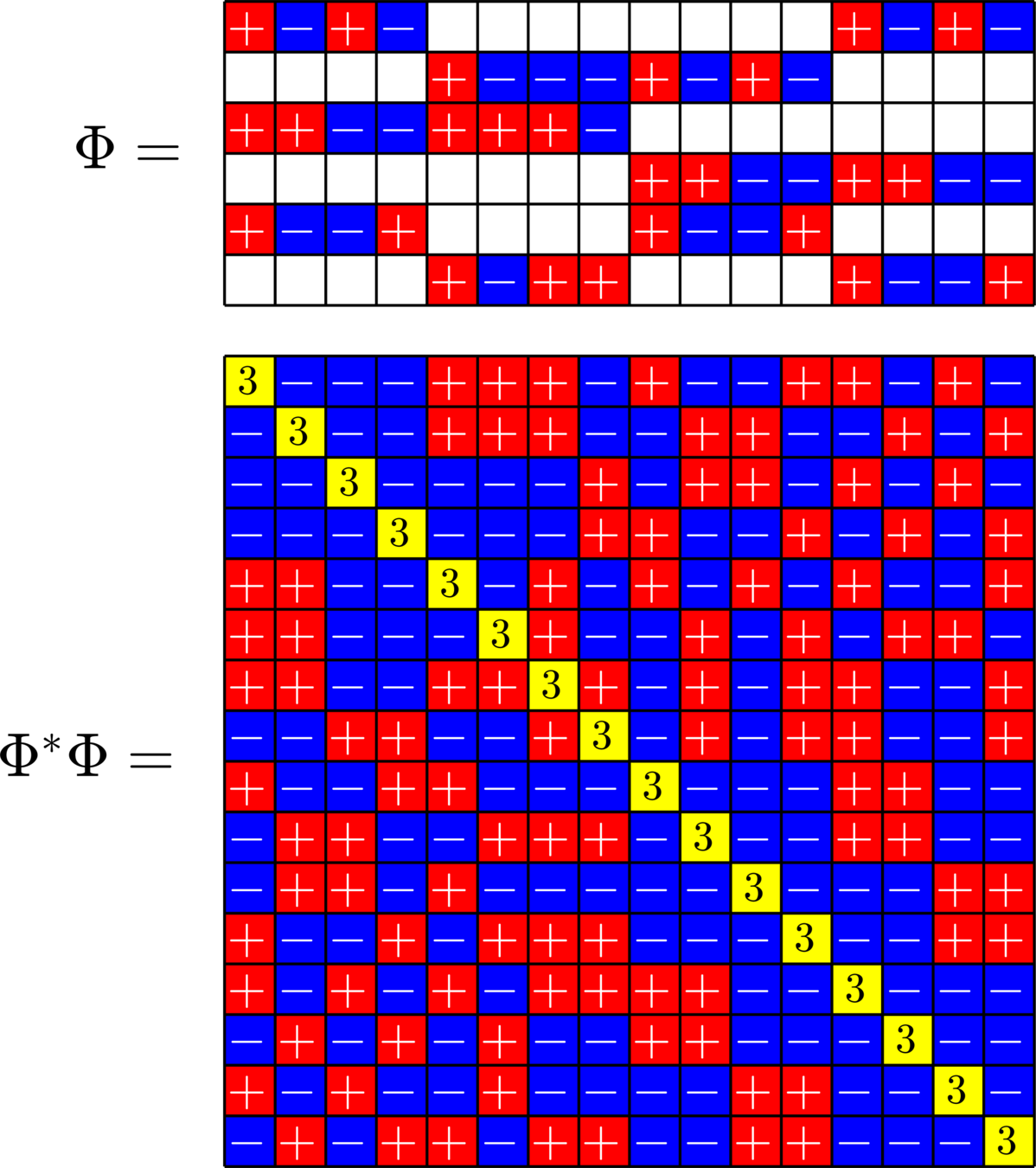
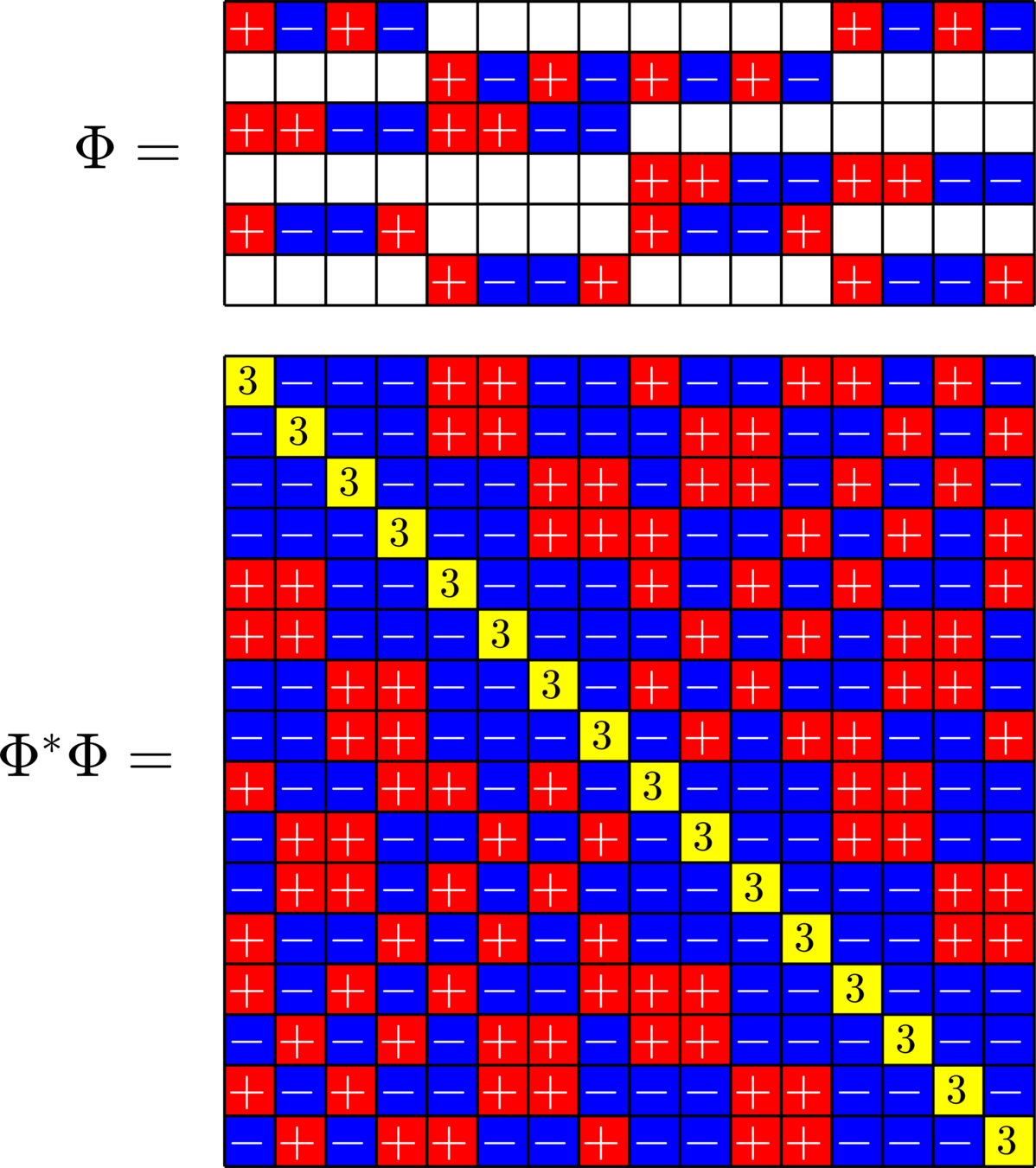
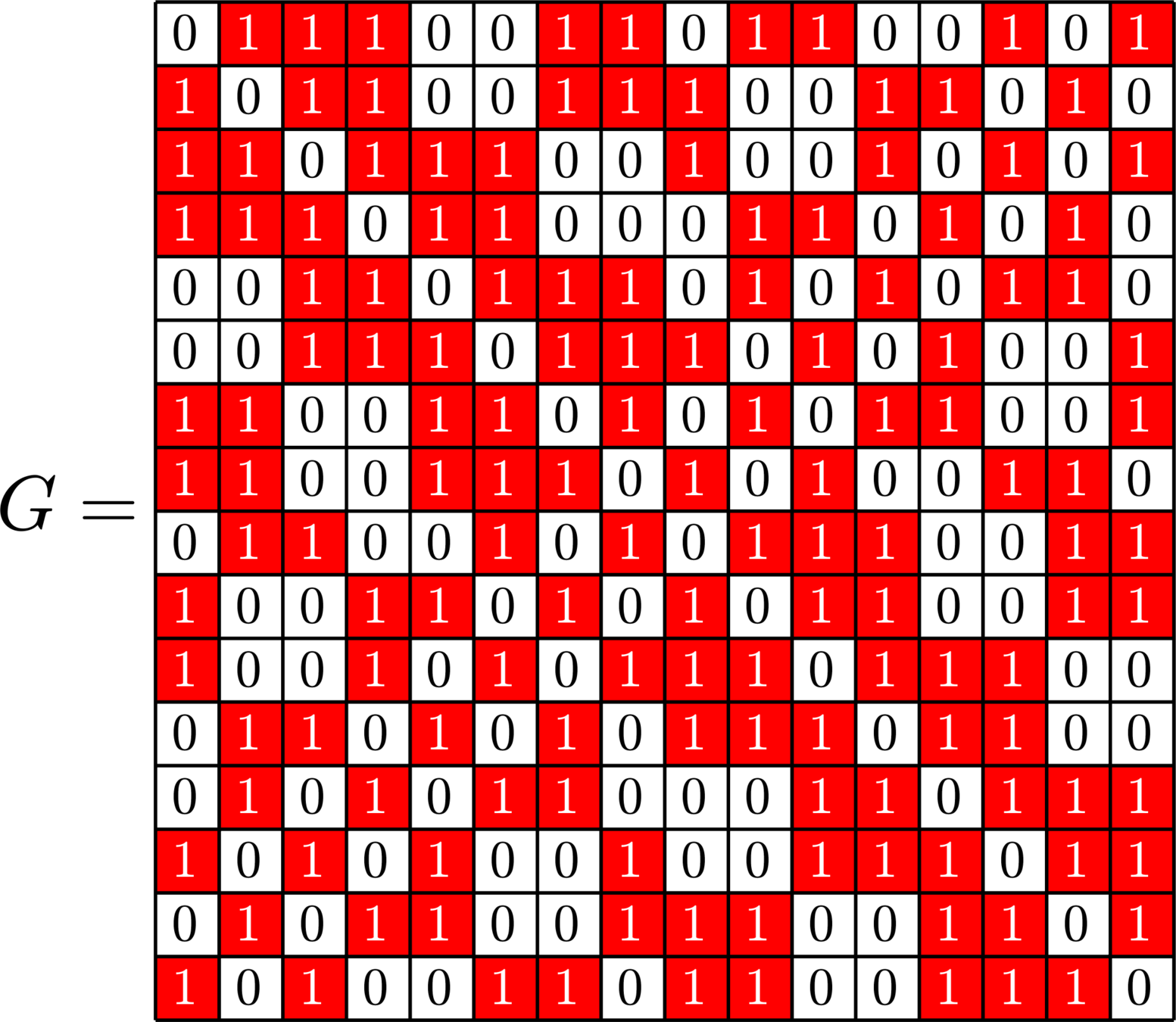
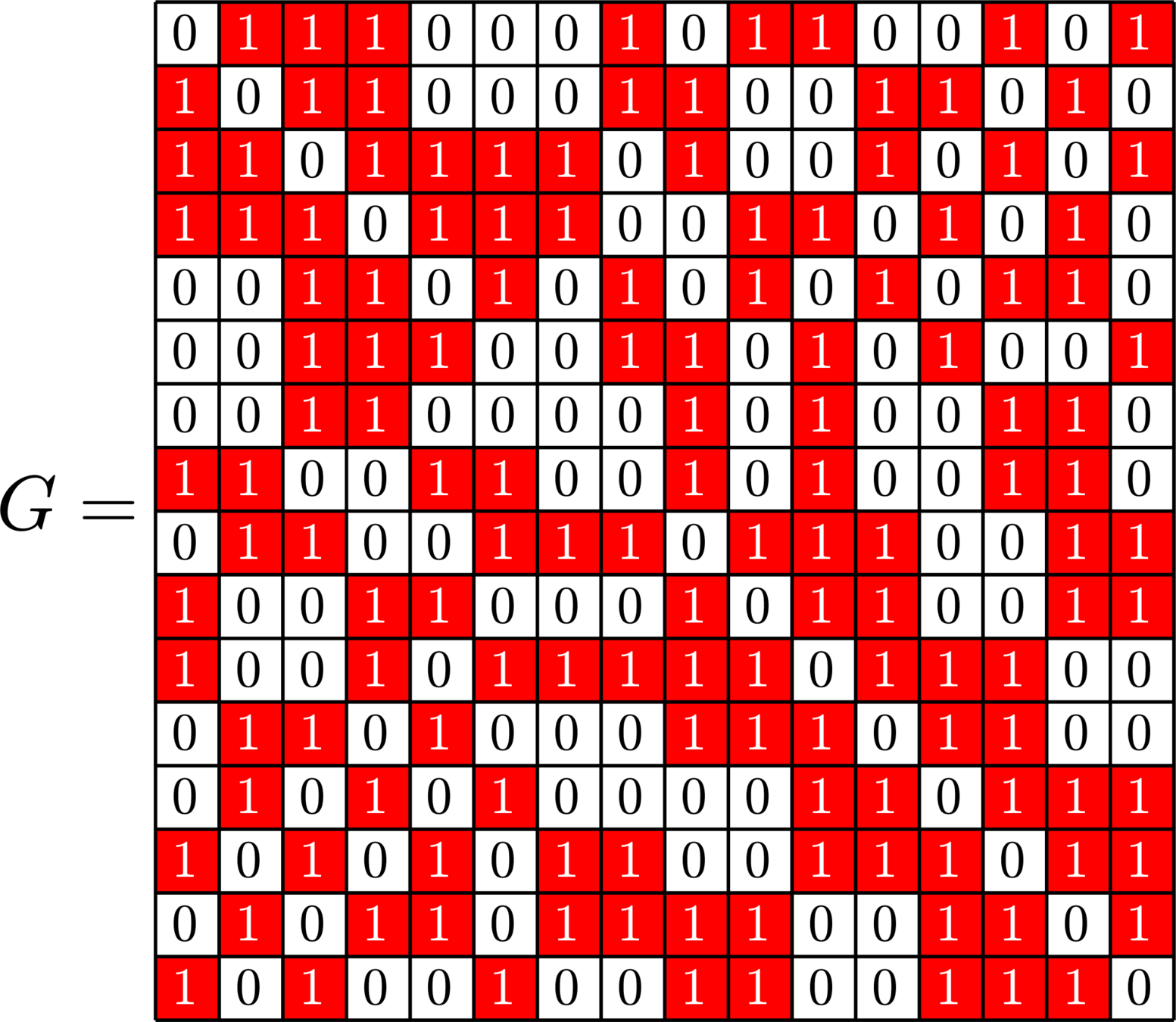

Mult. by \(-1\)
Not regular!
Strongly Regular Graphs
Definition. An \(n\)-vertex graph is called strongly regular if
- every vertex has \(k\) neighbors
- adjacent vertices have \(\lambda\) common neighbors
- nonadjacent vertices have \(\mu\) common neighbors
Such a graph is called an SRG\((n,k,\lambda,\mu)\).
Equivalently, the adjacency matrix \(G\) satisfies
\[G^{2} = k I + \lambda G + \mu(J-I-G)\quad\text{(where \(J\) is the all-ones matrix.)}\]
\(\Phi\) ETF \(\Rightarrow\) "quadratic relation":
\[(\Phi^{\top}\Phi)^{2} = A\,\Phi^{\top}\Phi.\]
But how many \(-1\)'s in each row ???

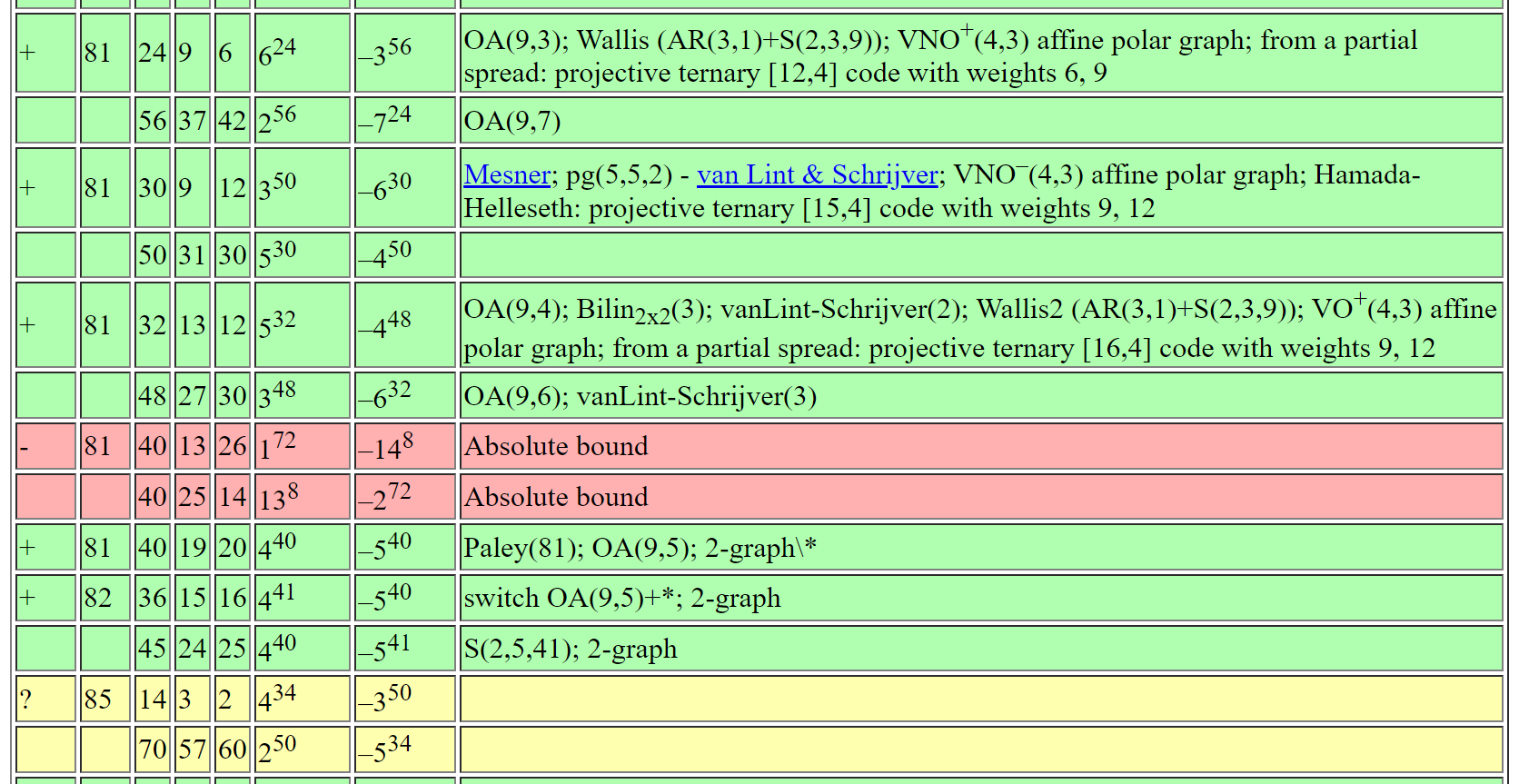

Andries Brouwer's Table of SRGs
= \(\nexists\)
= ???
= \(\exists\)
Goal: \(\mapsto\)
ETF \(\Rightarrow\) SRG
\(\Rightarrow\) the associated graph is regular and thus strongly regular
\(\mathbf{1}\) is an eigenvector of \(\Phi^{\top}\Phi\).
- \(\mathbf{1} := (1,1,\ldots,1)\in\ker\Phi\)
- \(\mathbf{1}\) \(\in\) row space of \(\Phi\), or
}
\(\Leftrightarrow\)
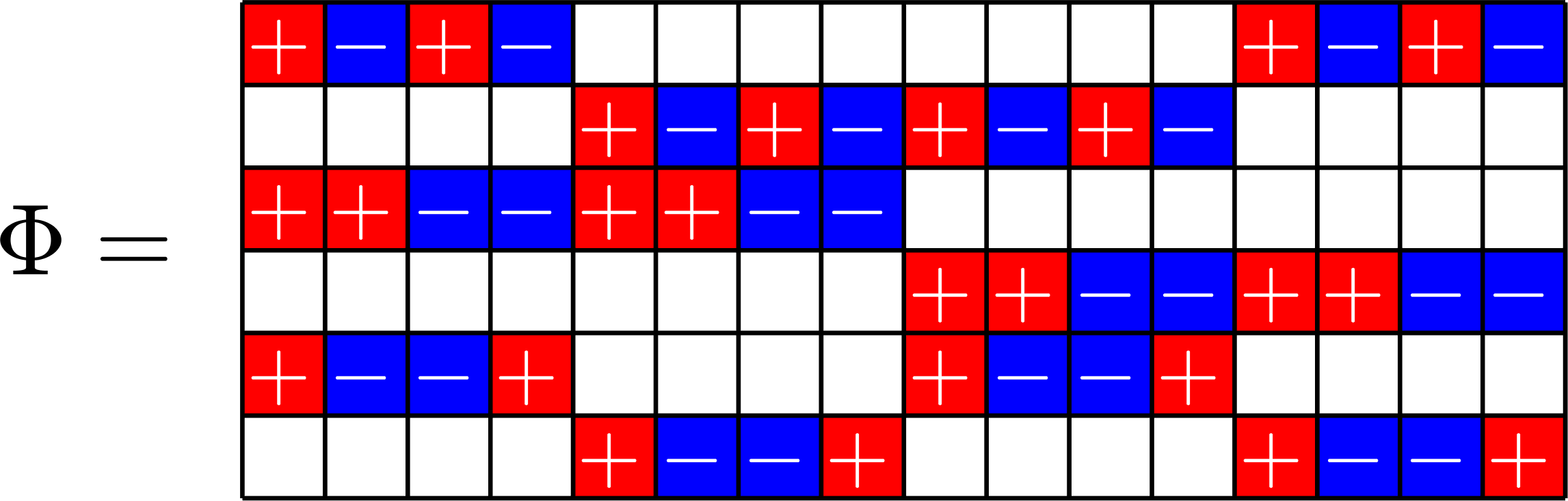
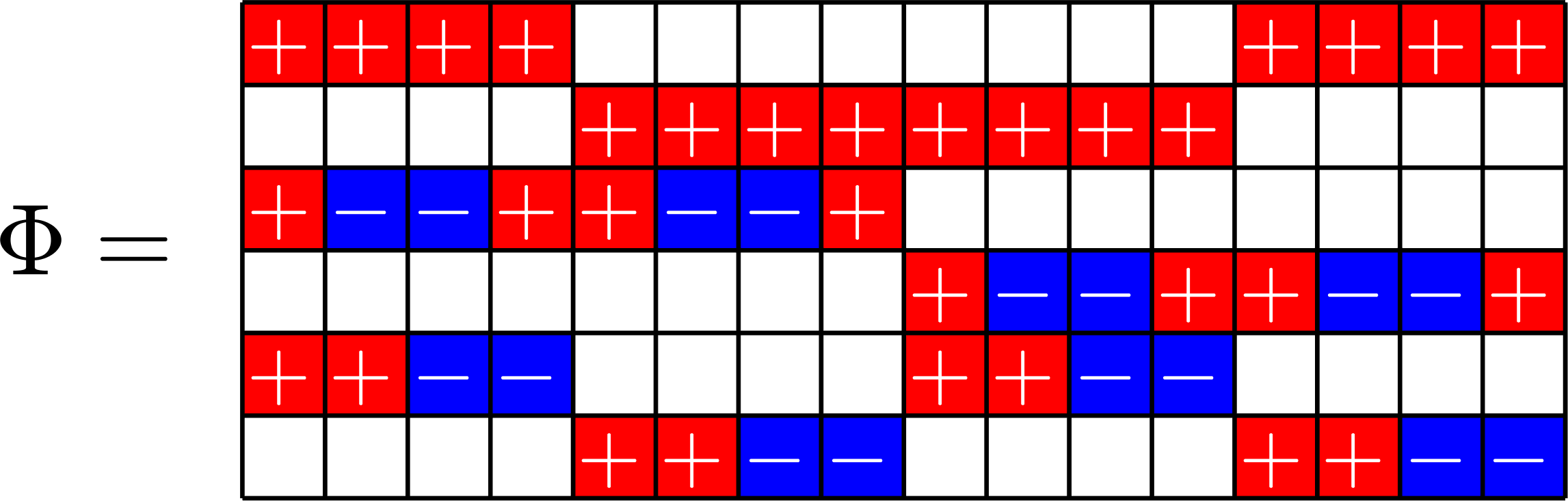
\(\mathbf{1}\) in row space
SRG\((16,5,0,2)\)
\(\mathbf{1}\in\ker\Phi\)
SRG\((16,9,4,6)\)
Example.
Nice ETF representation \(\Rightarrow\) new SRGs!
Suppose \(\Phi\) is an ETF:
Prototype
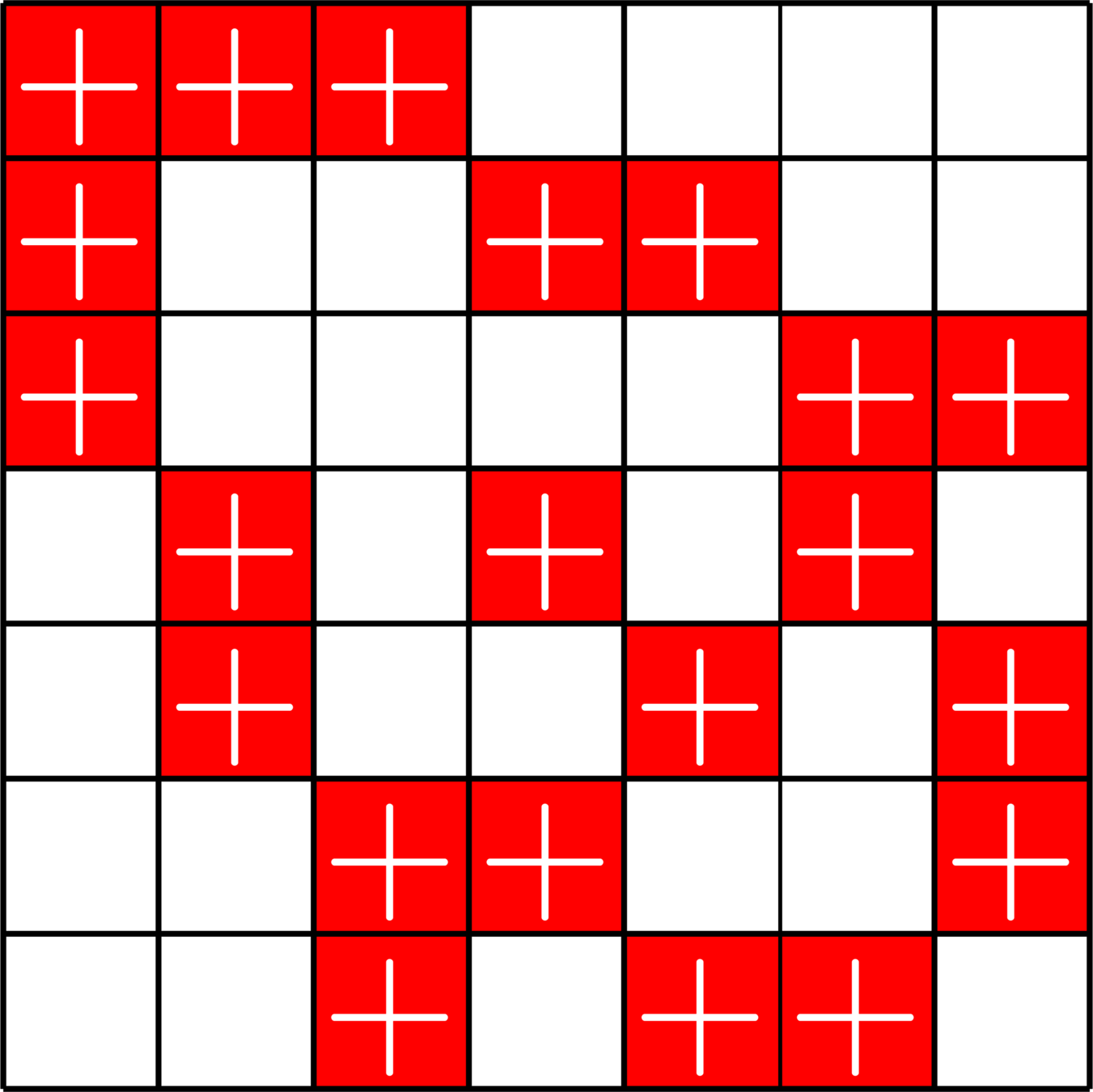
Tremain ETFs


\[\left[\begin{array}{l} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \end{array}\right.\]
\[\left.\begin{array}{l} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \end{array}\right]\]
\(\bigotimes\)
\(\sqrt{2}\)
\(\sqrt{\dfrac{1}{2}}\)
\(\sqrt{\dfrac{3}{2}}\)

Hadamard matrix
Hadamard matrix
Steiner Triple System
Tremain ETFs


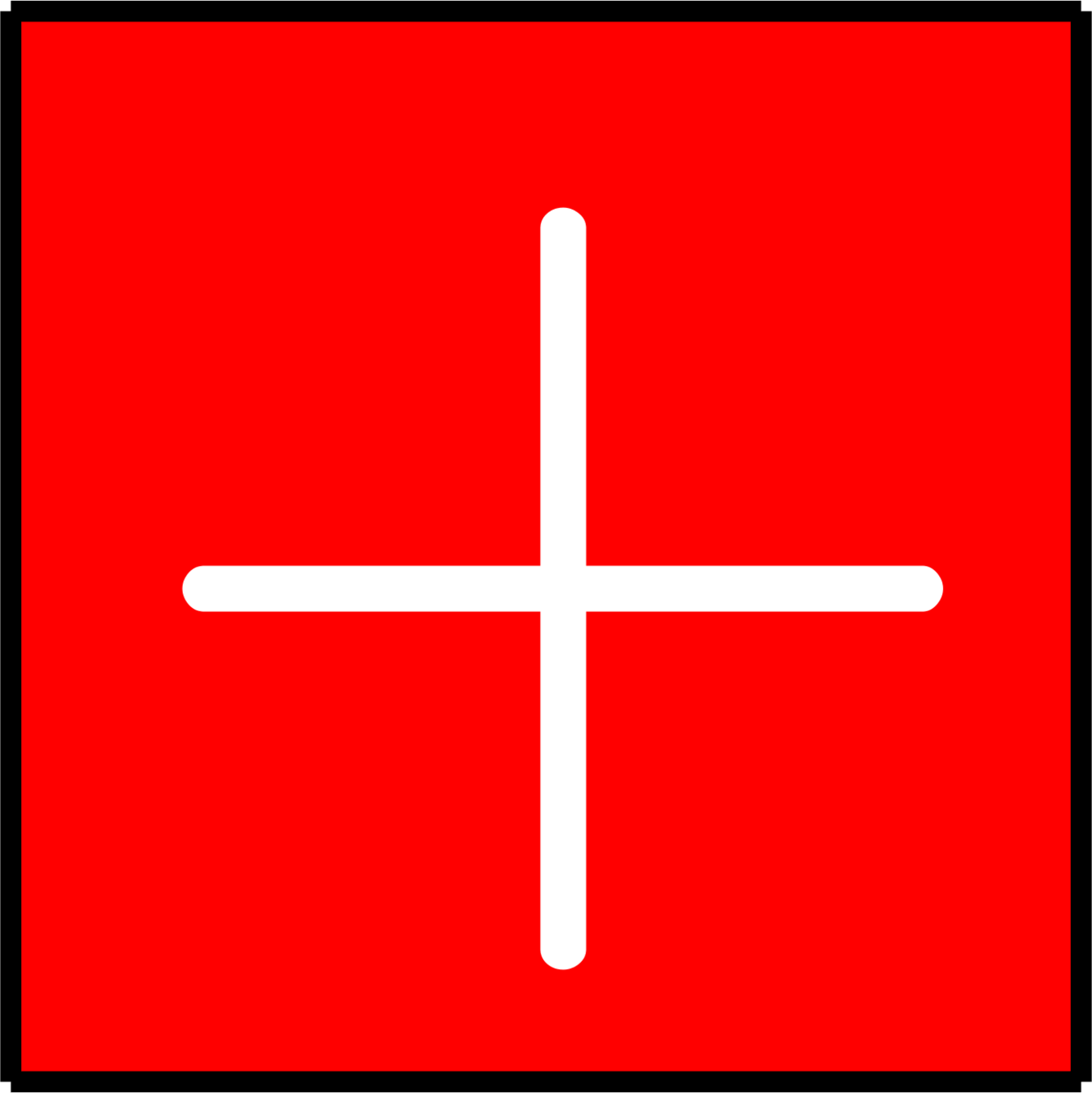
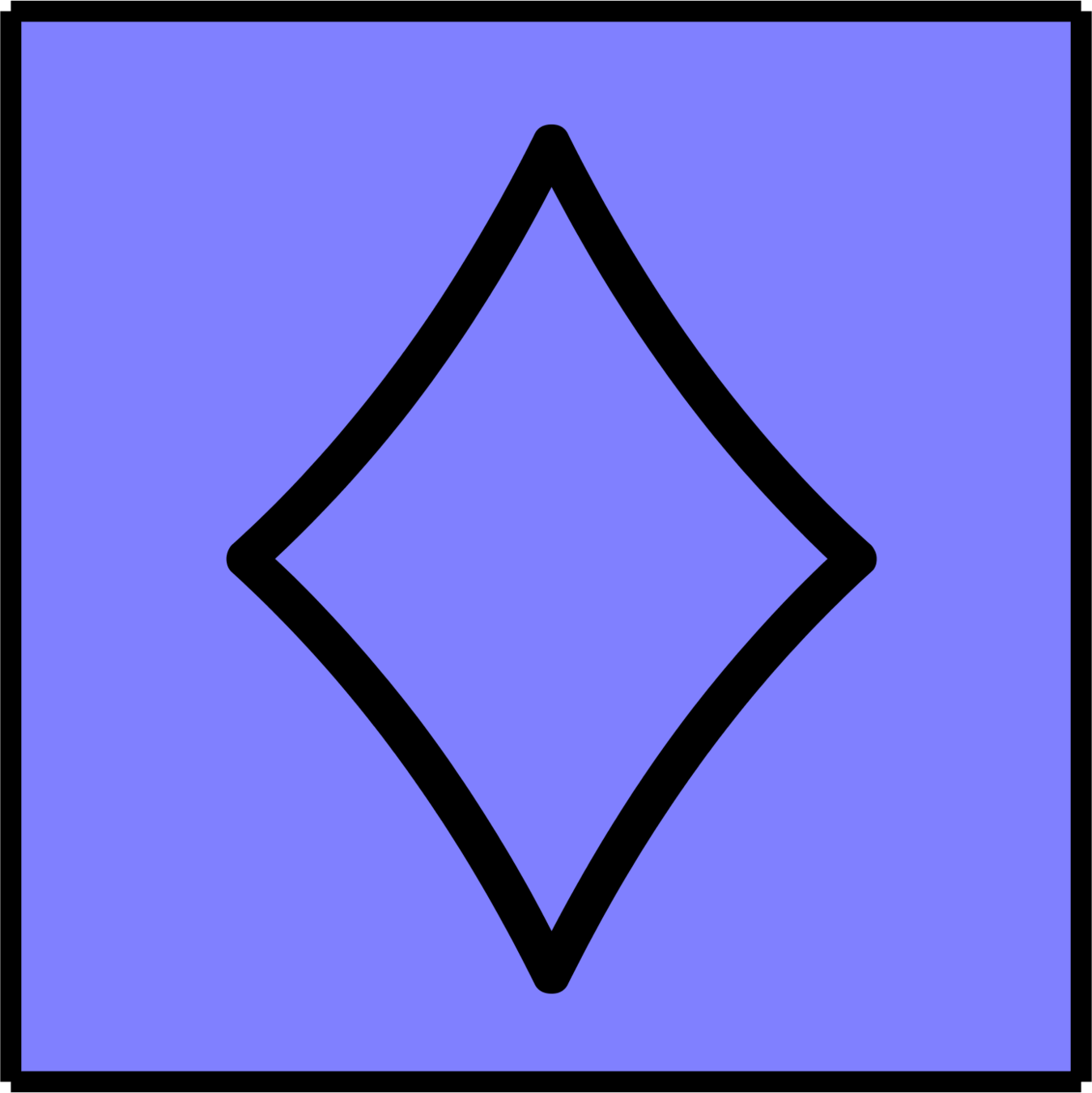
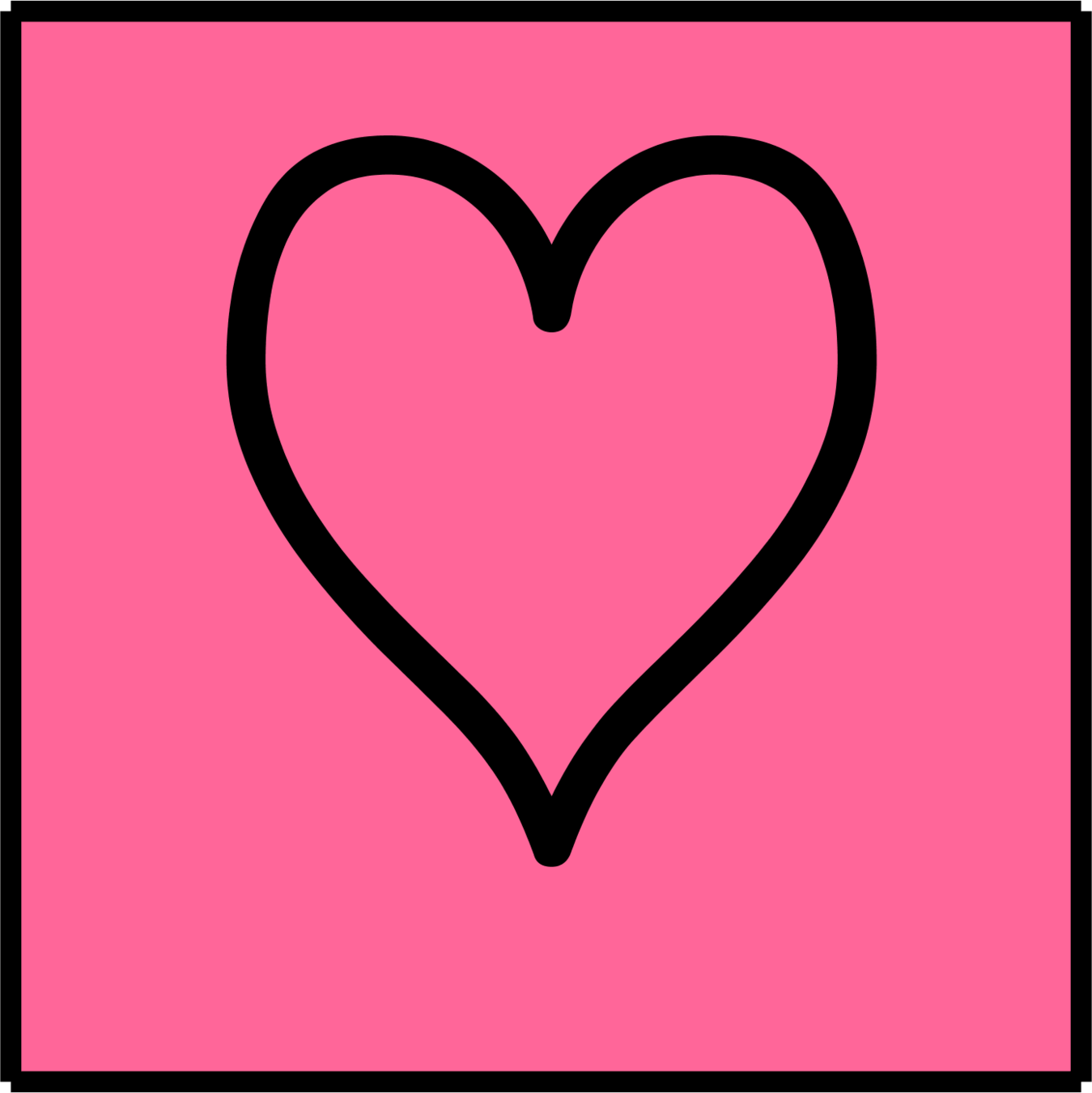

\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)
Tremain ETFs:
Theorem (Fickus, J, Mixon, Peterson '18). If there exists an
\(h\times h\) Hadamard matrix with \(h\equiv 1\) or \(2\) \(\text{mod}\ 3\),
then there exists a \((2,3,2h-1)\)-Steiner system
and by the Tremain construction there exists a real \(d\times N\) ETF where \[d=\frac{1}{3}(h+1)(2h+1),\qquad N=h(2h+1).\]



\(51\times 136\) Tremain ETF
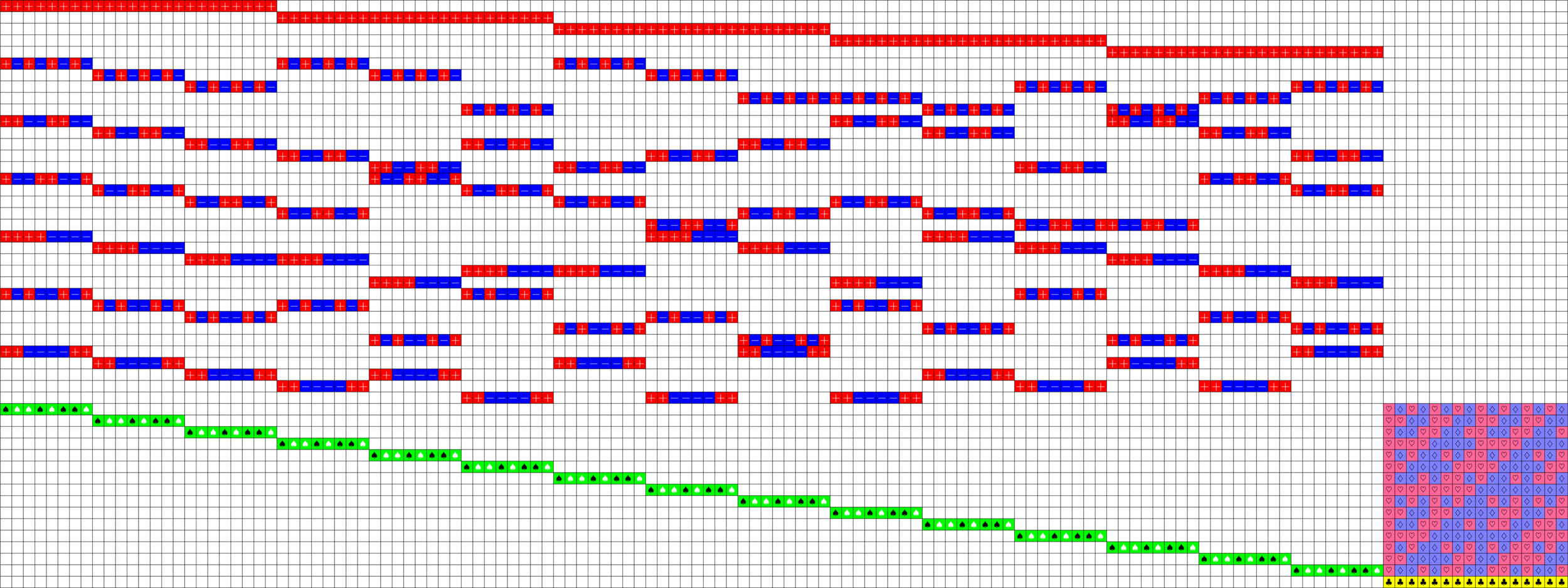







\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)
\(\ =-\sqrt{2}\)
\(\ =-\sqrt{\dfrac{3}{2}}\)
Axial Tremain ETFs:
Theorem (Fickus, J, Mixon, Peterson '18). If there exists an
\(h\times h\) Hadamard matrix with \(h\equiv 2\) \(\text{mod}\ 3\),
then there exists a strongly regular graph with parameters:
\[v=h(2h+1),\ k=\frac{(h+2)(2h-1)}{2},\ \lambda=\frac{(h-1)(h+4)}{2},\ \mu = \frac{h(h+2)}{2}\]


This gave us a new ETF!
From Brouwer's table online:
Let's replicate this success!
New Results
How can I make these vectors sum to zero?






\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)

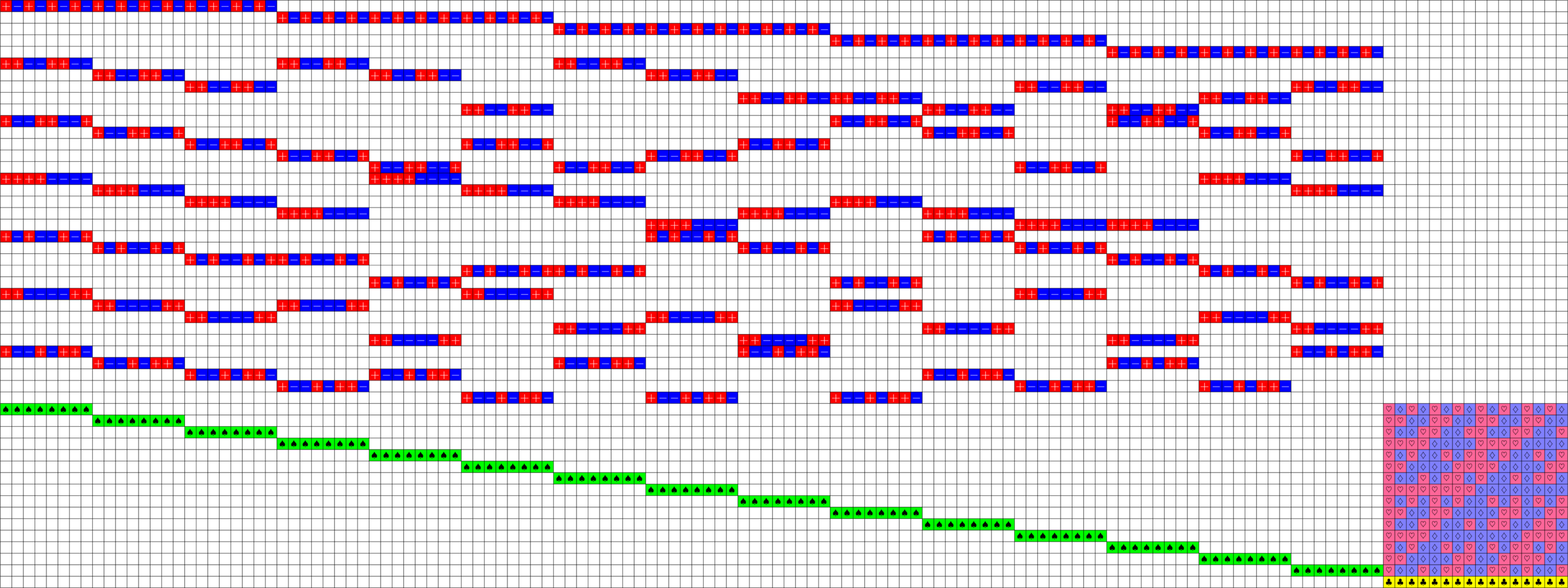
Graph from a \(15\times 36\) ETF with \(\boldsymbol{1}\) the kernel
Back to Brouwer's Table

\(\operatorname{NO}^{-}\)(6,2)

Nice short fat repn ?

\(NO_{6}^{-}(2)\)
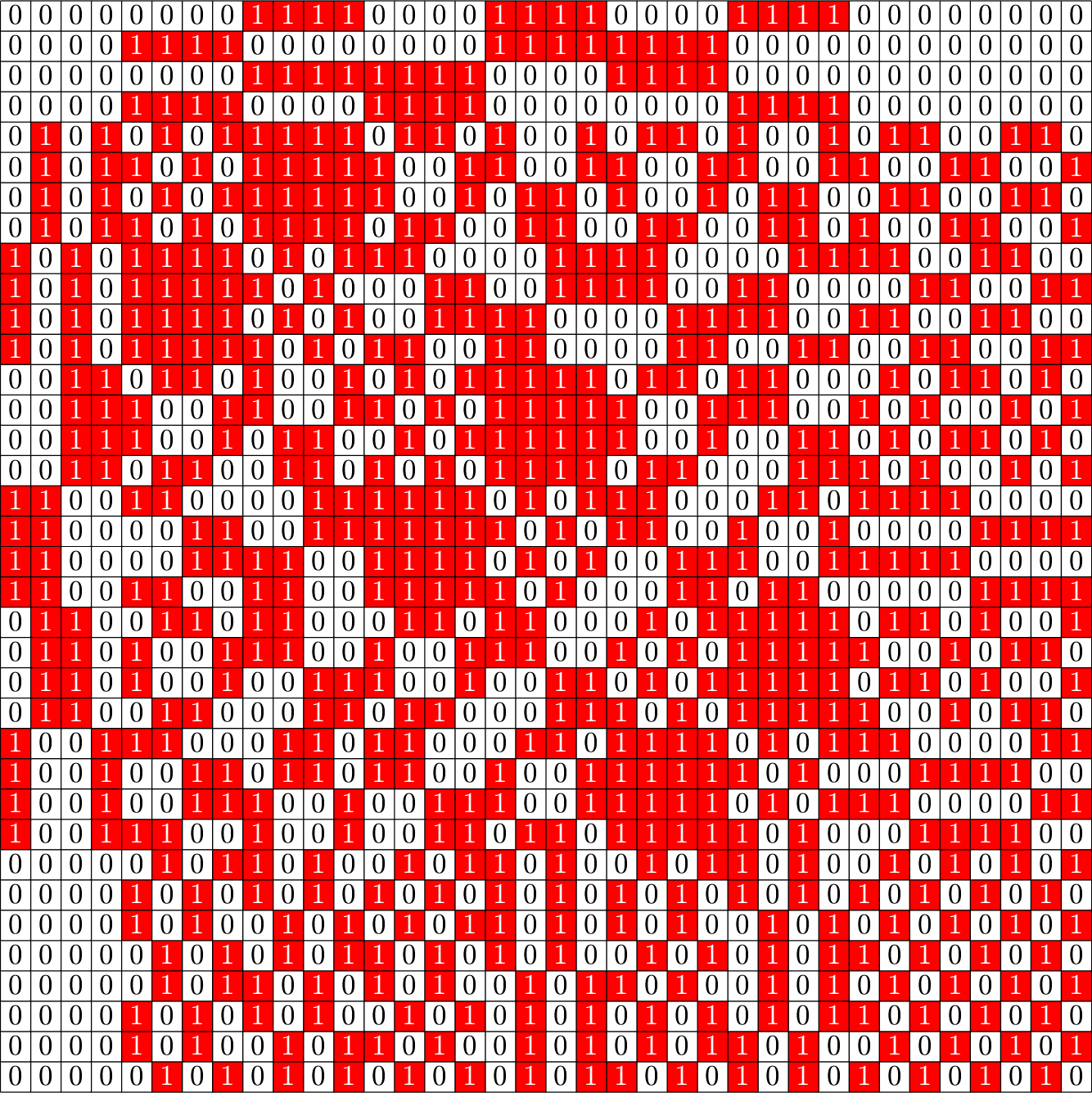

\(\Phi^{\top}\Phi=\)
\(\Phi=\)

Remember: Gramians are forgetful


\(NO_{6}^{-}(2)\)



\(NO_{6}^{-}(2)\)
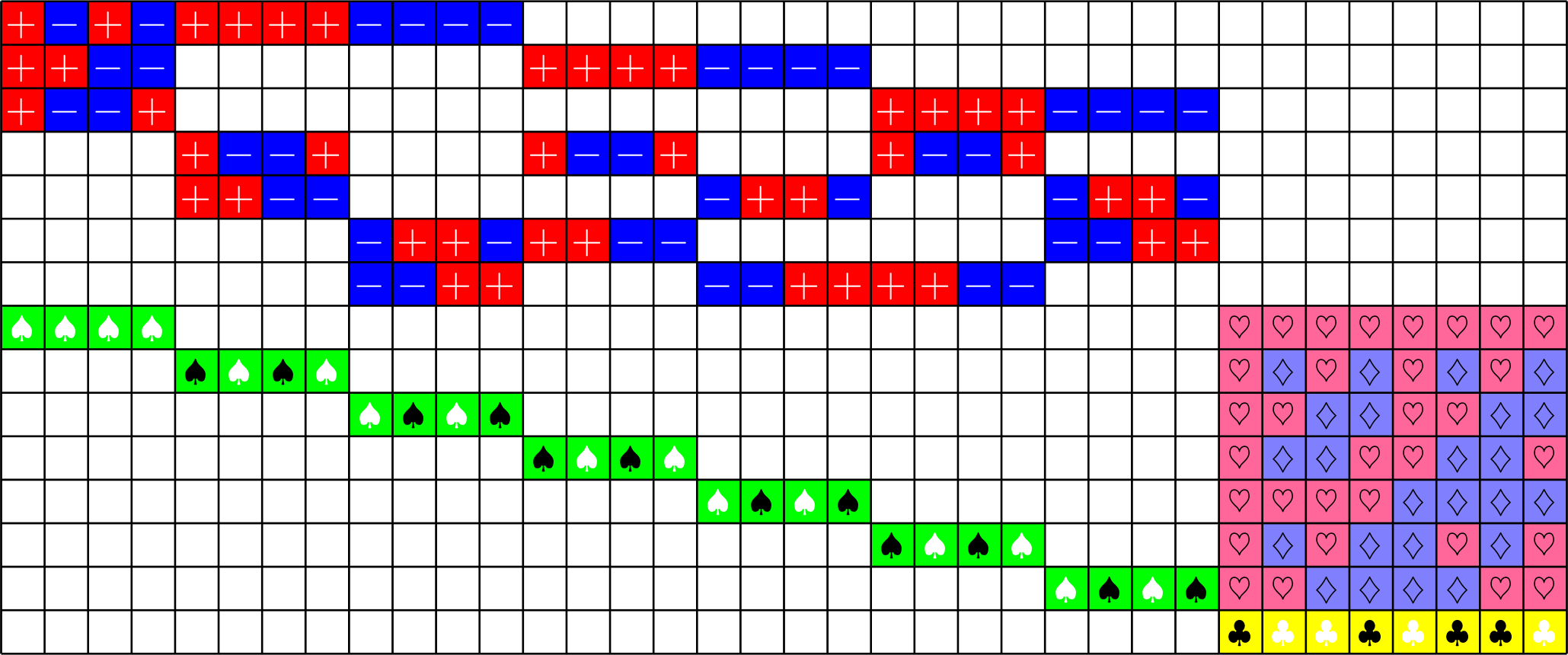






\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)


\(\ =-\sqrt{2}\)
\(\ =-\sqrt{\dfrac{3}{2}}\)
\(NO_{6}^{-}(2)\)

- \(\mathbf{1} = (1,1,\ldots,1)\) is in the kernel
- works for all real Tremain ETFs!
Centered Tremain ETFs:
Theorem (J). If there exists an
\(h\times h\) Hadamard matrix with \(h\equiv 1\) or \(2\) \(\text{mod}\ 3\),
then there exists a strongly regular graph with parameters
\[v=h(2h+1),\quad k=h^2-1,\quad \lambda=\frac{1}{2}(h^2-4),\quad \mu = \frac{1}{2}h(h-1)\]


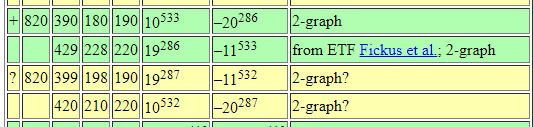
\(\exists\, 20\times 20\) Hadamard matrix \(\Rightarrow\) SRG(820,399,198,190)

From Brouwer's table online:

\(\exists\, 20\times 20\) Hadamard matrix \(\Rightarrow\) SRG(820,399,198,190)
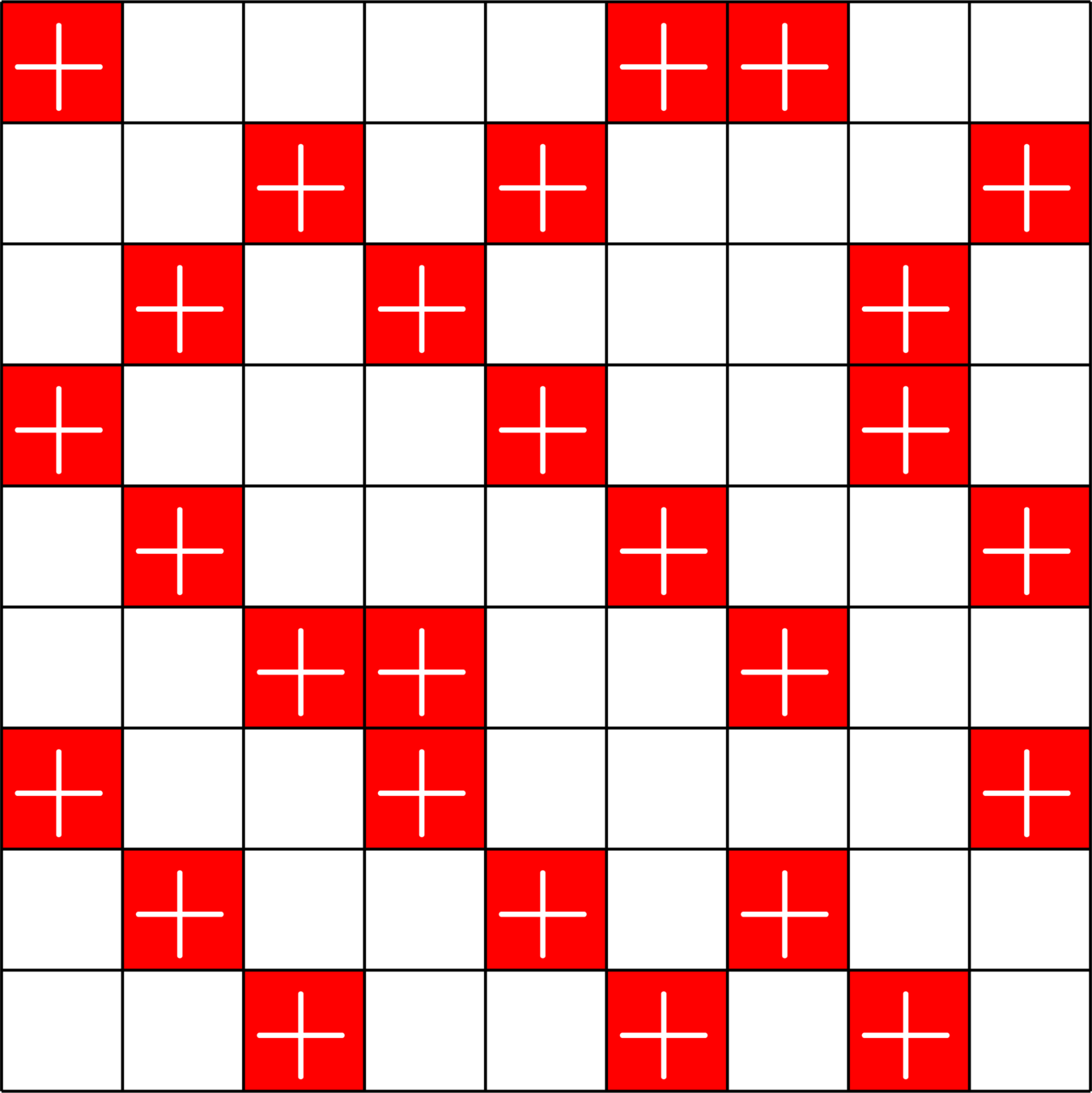
\(\bigotimes\)
\[\left[\begin{array}{l} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \end{array}\right.\]
\[\left.\begin{array}{l} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \end{array}\right]\]
\(I_{3}\otimes\)(\(2\times 3\) ETF)
Hadamard Matrix
Group Divisible Design (GDD)
Group Divisible Design






\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)


\(\ =-\sqrt{2}\)
\(\ =-\sqrt{\dfrac{3}{2}}\)
Group Divisible Design






\(\ =+1\)
\(\ =-1\)
\(\ =\sqrt{2}\)
\(\ =-\sqrt{\dfrac{1}{2}}\)
\(\ =+\sqrt{\dfrac{1}{2}}\)
\(\ =\sqrt{\dfrac{3}{2}}\)


\(\ =-\sqrt{2}\)
\(\ =-\sqrt{\dfrac{3}{2}}\)
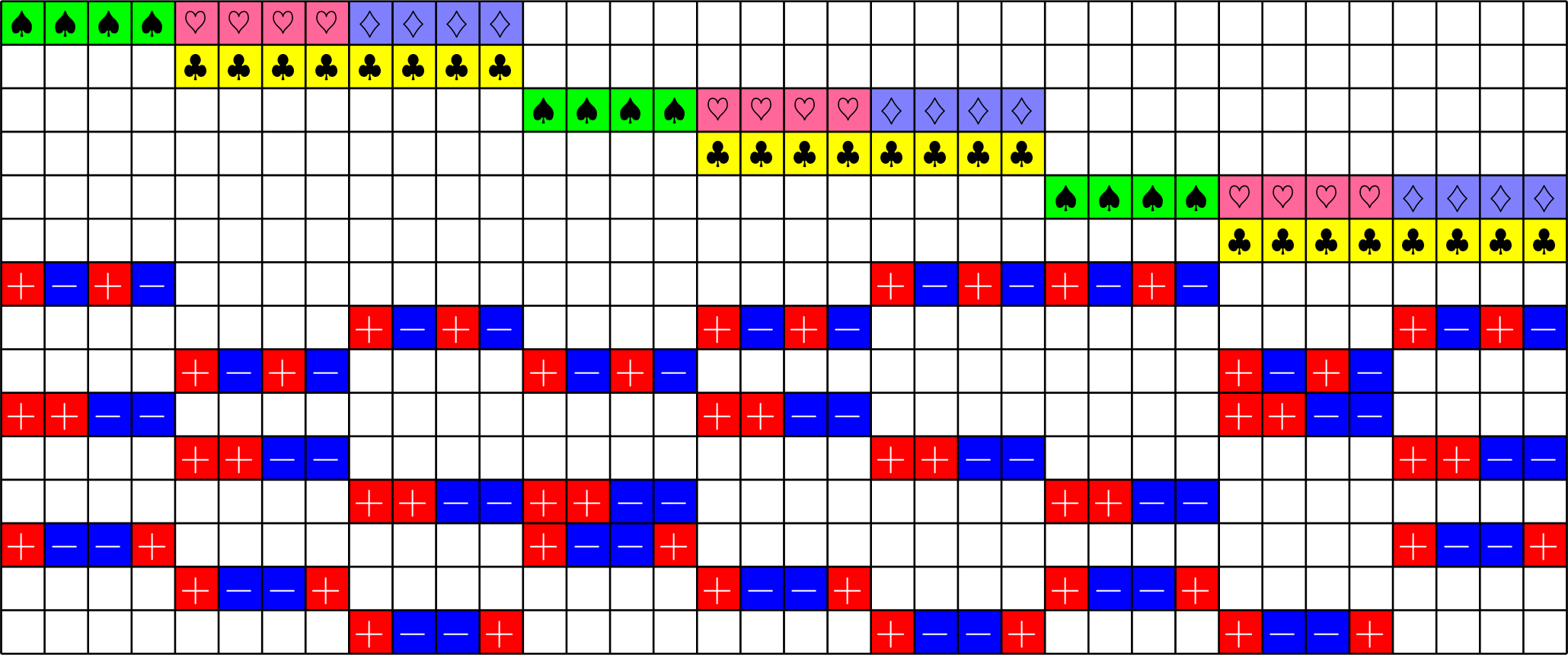
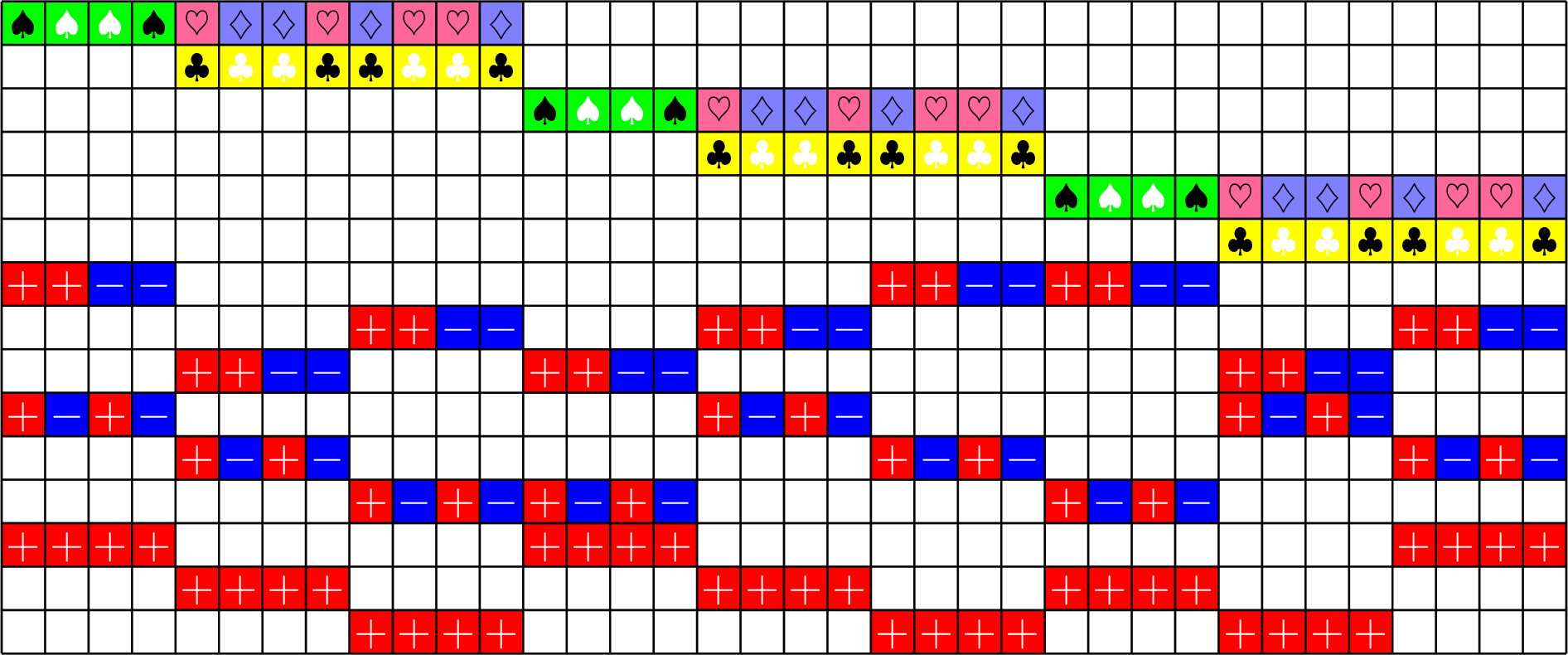
Axial GDD ETFs:
Theorem (J). If there exists an
\(h\times h\) Hadamard matrix with \(h\equiv 1\) \(\text{mod}\ 3\),
then there exists a strongly regular graph with parameters


\(\exists\,16\times 16\) Hadamard matrix \(\Rightarrow\exists\) SRG(528,279,150,144)
From Brouwer's table online:

\[v=h(2h+1),\ \ k=\frac{(h+2)(2h-1)}{2},\ \lambda=\frac{(h-1)(h+4)}{2},\ \mu = \frac{h(h+2)}{2}\]

\(\exists\,16\times 16\) Hadamard matrix \(\Rightarrow\exists\) SRG(528,279,150,144)
Tremain-sized ETFs
2
5
15
51
77
145
187
287
345
477
551
715
3
10
36
136
210
406
528
820
990
1378
1596
2080
\(d\)
\(N\)
Exists
Centered
Axial
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
?
?
?
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
Yes
?
?
?
?
?
?
Finding an axial \(77\times 210\)
- Let \(\Phi\) be the known centered \(77\times210\).
- We want to find \(x = \operatorname{argmin}_{\|x\|_{2} = 1}\|\Phi^{\top}x\|_{\infty}\)
- Instead we find \(x = \operatorname{argmin}_{\|x\|_{2} = 1}\|\Phi^{\top}x\|_{4}\)
- Gradient descent on many seeds
- \(\Phi^{\top}x\) is \(\pm1\) vector \(\Rightarrow\) Profit!
There exists an SRG(210,95,40,45)
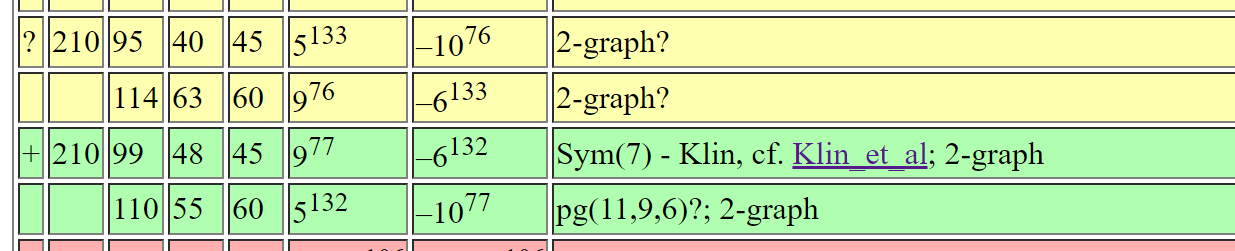
From Brouwer's table online:

There exists an SRG(210,95,40,45)
Thanks!
- This work was partially supported by NSF #1830066
- Webpage: https://tinyurl.com/jasperAFIT
- Slides: https://slides.com/johnjasper

International Conference on Approximation Theory and Beyond
By John Jasper
International Conference on Approximation Theory and Beyond
- 218



