Flag Hilbert–Poincaré series and Igusa zeta functions of hyperplane arrangements
Joshua Maglione
Bielefeld University
math.uni-bielefeld.de/~jmaglione
www.slides.com/joshmaglione/cirm2021
Joint with ...
Igusa's zeta functions
Count roots of \(f\in\Z[X_1,\dots, X_d]\): let \(p\) be a prime,
N_m(p) = \#\left\{X\in(\Z/p^m\Z)^d ~\middle|~ f(X)\equiv 0\mod p^m\right\}
\displaystyle P_f(T) = \sum_{m=0}^\infty p^{-dm}N_m(p)T^m
Igusa's zeta function – \(p\)-adic integral closely related to
Of interest: \(f\) a product of linear polynomials.
Goal: use combinatorial & topological tools to understand asymptotics and arithmetic of \(P_f(T)\) and Igusa zeta function.
Comes up in computing: subgroup, representation, & class counting zeta functions.
Flag Hilbert–Poincaré series
\(\mathcal{A}\) hyperplane arrangement – hyperplanes in \(K^d\)
\(\mathcal{L}(\mathcal{A})\) intersection poset – all nonempty intersections
\pi_{\mathcal{A}}(Y) = \displaystyle\sum_{x\in\mathcal{L}(\mathcal{A})} \mu(x)(-Y)^{\mathrm{rk}(x)} = \mathrm{Poin}(\mathbb{C}^d \setminus \bigcup_{H\in\mathcal{A}}H;\; Y)
Poincaré polynomial:
\(K\!=\!\mathbb{C}\)
\(\hat{1}\)
\(\hat{0}\)
\mathcal{B}_n := \{X_i - X_j ~|~ 1 \leq i < j \leq n\}:
\pi_{\mathcal{B}_4}(Y) = 1 + 6Y + 11Y^2 + 6Y^3
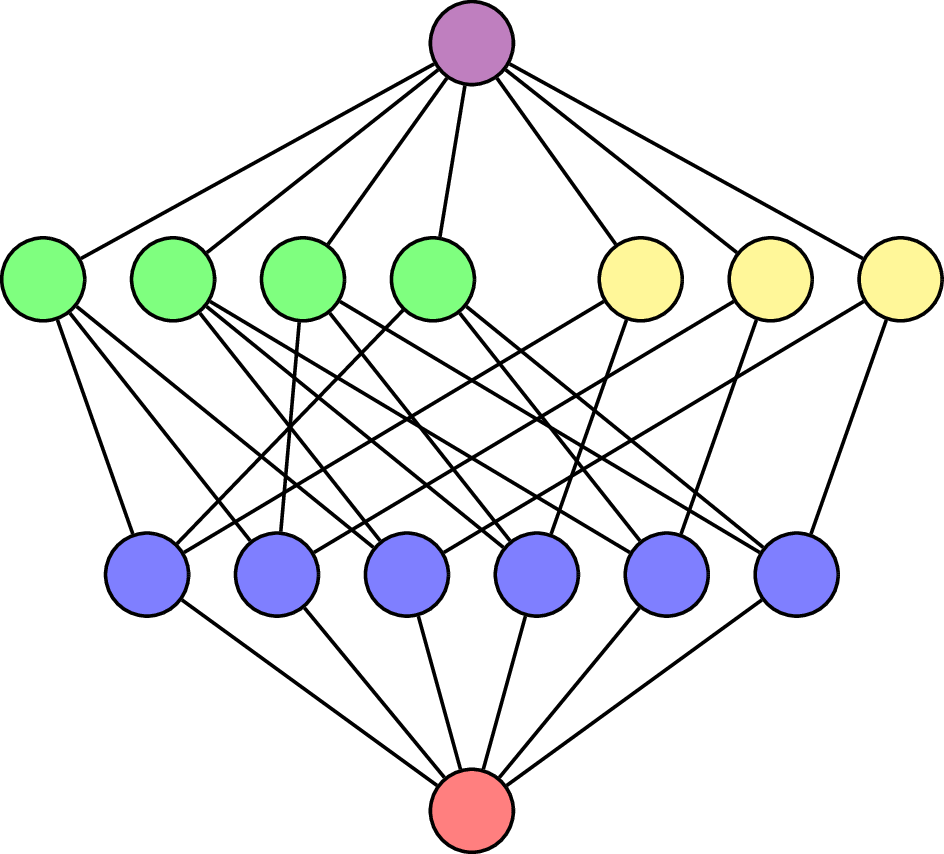
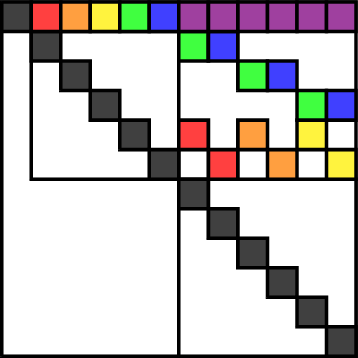
\(\mathcal{L}(\mathcal{B}_4)\)
\(\mathrm{rk}\)
0
1
2
3
For \(F = (x_1 < \cdots < x_\ell)\in\Delta(\mathcal{L}(\mathcal{A}))\), with \(x_0=\hat{0}\), \(x_{\ell+1}=\varnothing\),
\pi_F(Y) = \displaystyle\prod_{k=0}^{\ell} \pi_{\mathcal{A}^{x_k}_{x_{k+1}}}(Y)
With \(x\in \mathcal{L}(\mathcal{A})\), two new arrangements
\begin{aligned}
\mathcal{A}_x &= \left\{H\in\mathcal{A} ~\middle|~ x\subseteq H \right\}, & & (\text{subarrangement})\\
\mathcal{A}^x &= \left\{x\cap H ~\middle|~ H\in\mathcal{A}\setminus\mathcal{A}_x,\; x\cap H\neq \varnothing \right\}. & & (\text{restriction})
\end{aligned}
Example: \(\mathcal{A} = \mathcal{B}_4\)
Order complex: \(\Delta(\mathcal{L}(\mathcal{A}))\) – flags in \(\mathcal{L}(\mathcal{A})\)
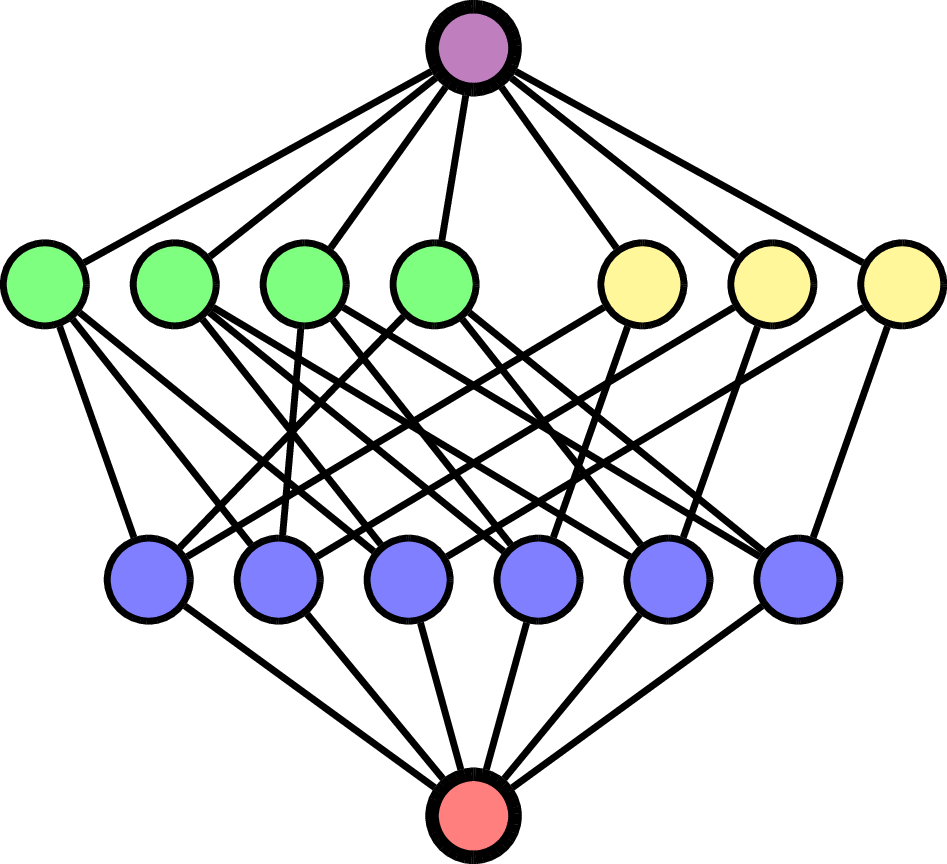
\(F\) empty flag in \(\Delta(\mathcal{L}(\mathcal{A}))\):
\begin{aligned}
\pi_F(Y) &= \pi_{\mathcal{A}_{\varnothing}^{\hat{0}}}(Y) = \pi_{\mathcal{A}}(Y) \\
&= 1 + 6Y + 11Y^2 + 6Y^3
\end{aligned}
\(F = (\quad\;)\):
\begin{aligned}
\pi_F(Y) &= \pi_{\mathcal{A}^{\hat{0}}}(Y) \cdot\pi_{\mathcal{A}_{\varnothing}}(Y) \\
&= (1+Y)\cdot (1 + 3Y + 2Y^2)
\end{aligned}
\begin{aligned}
\pi_F(Y) &= \pi_{\mathcal{A}^{\hat{0}}}(Y) \cdot \pi_{\mathcal{A}_{\phantom{-}}}(Y) \cdot \pi_{\mathcal{A}_{\varnothing}}(Y) \\
&= (1+Y)\cdot (1 + Y) \cdot (1 + Y)
\end{aligned}
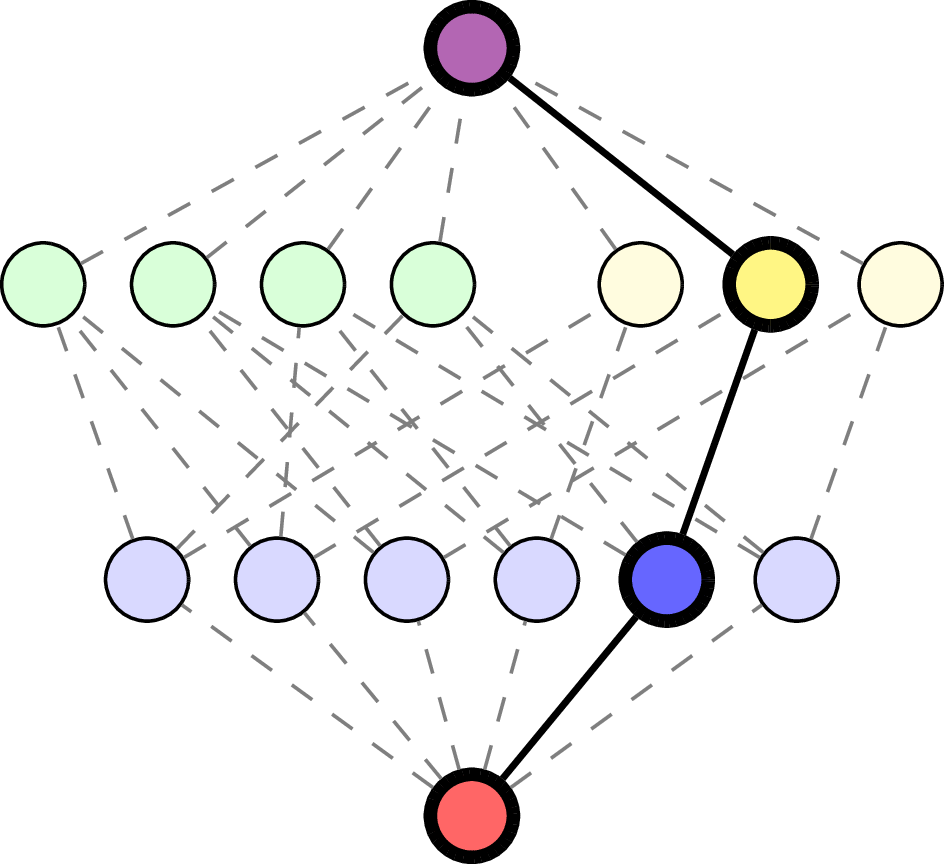
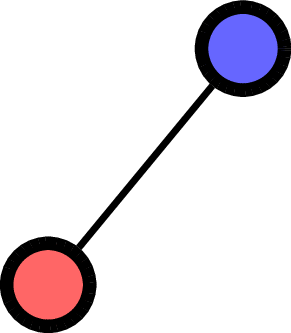
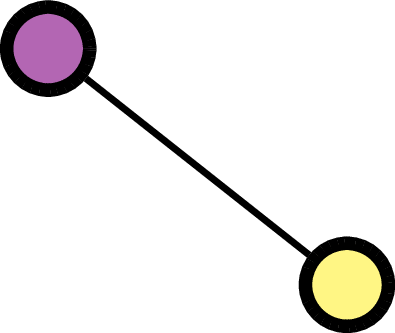
\(F = (\quad\;<\;\quad)\):
Set \(\widetilde{\mathcal{L}}(\mathcal{A}) = \mathcal{L}(\mathcal{A})\setminus\{\hat{0}\}\) and \(\mathcal{A}\) is central if \(\bigcap_{H\in\mathcal{A}} H \neq \varnothing\)
The flag Hilbert–Poincaré series:
\mathsf{fHP}_{\mathcal{A}}(Y, \bm{T}) = \displaystyle\sum_{F\in\Delta(\widetilde{\mathcal{L}}(\mathcal{A}))} \pi_F(Y) \prod_{x\in F} \dfrac{T_x}{1 - T_x}
Thm (M.–Voll 2021). For \(\mathcal{A}\) defined over characteristic \(0\) and central, then
\mathsf{fHP}_{\mathcal{A}}\left(Y^{-1},(T_x^{-1})_{x\in\widetilde{\mathcal{L}}(\mathcal{A})}\right) = (-Y)^{-\mathrm{rk}(\mathcal{A})} T_{\hat{1}} \cdot \mathsf{fHP}_{\mathcal{A}}(Y, \bm{T}).
Self-reciprocity
Idea: \(\mathsf{fHP}_{\mathcal{A}}\) is equivalent to a \(p\)-adic integral.
\(\mathcal{L}(\mathcal{B}_4)\)
Igusa zeta function: a substitution of \(\mathsf{fHP}_{\mathcal{A}}(Y, \bm{T})\).
\begin{aligned}
Y &= -p^{-1}, \\
T_x &= \begin{cases}
p^{-1-s} & x = \phantom{-}, \\
p^{-2-2s} & x = \phantom{-}, \\
p^{-2-3s} & x = \phantom{-}, \\
p^{-3-6s} & x = \phantom{-}.
\end{cases}
\end{aligned}
\dfrac{(1-p^{-1})\left(1 - 5p^{-1} + 6p^{-2} + \cdots - 6p^{-4-5s} + 5p^{-5-5s} - p^{-6-5s}\right)}{(1 - p^{-1-s})^2(1 - p^{-2-3s})(1 - p^{-3-6s})}
Igusa zeta function of \(f_{\mathcal{B}_4}\): for all primes \(p\),
f_{\mathcal{B}_4}(\bm{X}) = (X_1 - X_2)(X_1 - X_3)(X_1 - X_4)(X_2 - X_3)(X_2 - X_4)(X_3 - X_4)
Class counting zeta function: a substitution of \(\mathsf{fHP}_{\mathcal{A}}(Y, \bm{T})\).
\zeta^{\mathrm{cc}}_{\mathbf{G}}(s) = \displaystyle \sum_{m=0}^{\infty} \# \mathrm{cc}(\mathbf{G}(\mathbb{Z}/p^m\mathbb{Z})) p^{-ms}
\(\mathbf{G}\) a nilpotent group scheme of finite type over \(\mathbb{Z}_p\):
Set \(\Gamma = K_{3,2}\) and \(\mathbf{G}_{\Gamma}\) graphical group scheme.
\(\mathbf{G}_{\Gamma}(\mathbb{Z}_p)\) =
\(\leq\mathrm{GL}_{12}(\mathbb{Z}_p)\)
\( =\langle I + E_{1,2} + E_{5,7} + E_{6,8},~\dots, \)
\( I + E_{1,6} + E_{2,8} + E_{3,10} + E_{4,12}\rangle\)
\(\mathcal{C}_n\) coordinate hyperplanes in \(K^n\) and \(\mathcal{L}(\mathcal{C}_n)\cong 2^{[n]}\).
\begin{aligned}
Y &= -p^{-1}, \\
T_I &= \begin{cases}
p^{6-|I|-s} & I = \phantom{-}, \\
p^{7-|I|-s} & I = \phantom{-}, \\
p^{8-|I|-s} & I = \phantom{-}, \\
p^{4-s} & I = \phantom{-}, \\
p^{-|I|} & I = \phantom{-}.
\end{cases}
\end{aligned}
Class counting zeta function for \(\mathbf{G}_{\Gamma}(\mathbb{Z}_p)\) for all primes \(p\):
\dfrac{1 + p^{-s} - p^{1-s} - 2p^{2-s} - p^{3-s} + p^{4-s} + p^{4-2s}}{(1-p^{3-s})(1-p^{5-s})^2}
Details: Rossmann–Voll
A different coarsening
Set each \(T_x=T\) to get coarse flag Hilbert–Poincaré series:
Easier to see examples & other combinatorial properties.
Nice form:
\mathsf{cfHP}_{\mathcal{A}}(Y, T) = \dfrac{\mathcal{N}_{\mathcal{A}}(Y, T)}{(1 - T)^{\mathrm{rk(\mathcal{A})}}}
\mathsf{cfHP}_{\mathcal{A}}(Y, T) = \displaystyle\sum_{F\in\Delta(\widetilde{\mathcal{L}}(\mathcal{A}))} \pi_F(Y) \left(\dfrac{T}{1 - T}\right)^{|F|}
Braid arrangement in \(\mathbb{Q}^n\):
\mathcal{B}_n = \{X_i - X_j ~|~ 1\leq i < j \leq n\}
\mathcal{N}_{\mathcal{A}}(Y, T) = \displaystyle\sum_{F\in\Delta(\widetilde{\mathcal{L}}(\mathcal{A}))} \pi_F(Y) T^{|F|}(1 - T)^{\mathrm{rk}(\mathcal{A})-|F|}
\mathcal{N}_{\mathcal{B}_2}(Y, T) = 1 + Y
\mathcal{N}_{\mathcal{B}_3}(Y, T) = 1 + 3Y + 2Y^2 + (2 + 3Y + Y^2)T
\begin{aligned}
\mathcal{N}_{\mathcal{B}_4}(Y, T) &= 1 + 6Y + 11Y^2 + 6Y^3 \\
&\quad + (11 + 37Y + 37Y^2 + 11Y^3)T \\
&\quad + (6 + 11Y + 6Y^2 + Y^3)T^2
\end{aligned}
All nonnegative coefficients. Computed with \(\mathsf{HypIgu}\).
\begin{aligned}
\mathcal{N}_{\mathsf{E}_7}(Y, T) &= 1 + 63Y + 1617Y^2 + 21735Y^3 + 162939Y^4 + 663957Y^5 \\
&\quad + 1286963Y^6 + 765765Y^7 + (90400 + 1553980Y + 11064984Y^2 \\
&\quad + 42142884Y^3 + 92109360Y^4 + 113759940Y^5 + 70917656Y^6 \\
&\quad + 16725596Y^7)T + (5577043 + 64210477Y + 304475955Y^2 \\
&\quad + 763724661Y^3 + 1080226497Y^4 + 847444143Y^5 + 338480825Y^6 \\
&\quad + 53381039Y^7)T^2 + (37767356 + 323700436Y + 1123040604Y^2 \\
&\quad + 2022363924Y^3 + 2022363924Y^4 + 1123040604Y^5 \\
&\quad + 323700436Y^6 + 37767356Y^7)T^3 + (53381039 + 338480825Y \\
&\quad + 847444143Y^2 + 1080226497Y^3 + 763724661Y^4 + 304475955Y^5 \\
&\quad + 64210477Y^6 + 5577043Y^7)T^4 + (16725596 + 70917656Y \\
&\quad + 113759940Y^2 + 92109360Y^3 + 42142884Y^4 + 11064984Y^5 \\
&\quad + 1553980Y^6 + 90400Y^7)T^5 + (765765 + 1286963Y + 663957Y^2 \\
&\quad + 162939Y^3 + 21735Y^4 + 1617Y^5 + 63Y^6 + Y^7)T^6
\end{aligned}
\mathcal{N}_{\mathsf{E}_7}(1, T) = 2903040(1 + 120T + 1191T^2 + 2416T^3 + 1191T^4 + 120T^5 + T^6)
Do coefficients of \(\mathcal{N}_{\mathcal{A}}(Y, T)\) have combinatorial interpretation?
Coxeter arrangements
The Eulerian polynomial \(E_n(T)\) is combinatorially defined.
\begin{array}{rcccccccccc}
E_1: &&&&&& 1 &&&& \\
E_2: &&&&& 1 && 1 &&& \\
E_3: &&&& 1 && 4 && 1 && \\
E_4: &&& 1 && 11 && 11 && 1 & \\
E_5: && 1 && 26 && 66 && 26 && 1
\end{array}
\mathcal{N}_{\mathcal{A}}(1, T) = \pi_{\mathcal{A}}(1) \cdot E_{\mathrm{rk}(\mathcal{A})}(T).
Thm (M.–Voll 2021). For a Coxeter arrangement \(\mathcal{A}\) with no \(\mathsf{E}_8\) factors:
Conjecture (M.–Voll). For arbitrary \(\mathcal{A}\), the coefficients of \(\mathcal{N}_{\mathcal{A}}(Y, T)\) are nonnegative.
Apply combinatorial & topological tools from hyperplane arrangements to understand asymptotics and arithmetic of
\displaystyle P_f(T) = \sum_{m=0}^\infty p^{-dm}N_m(p)T^m.
Flag Hilbert–Poincaré series have combinatorial features:
Thm. \(\mathcal{A}\) central and characteristic \(0\) \(\implies\) \(\mathsf{fHP}_{\mathcal{A}}\) self-reciprocal.
Thm. \(\mathcal{A}\) Coxeter and no \(\mathsf{E}_8\)-factor \(\implies\) \(\mathcal{N}_{\mathcal{A}}(1, T)=\pi_{\mathcal{A}}(1)\cdot E_{\mathrm{rk}(\mathcal{A})}(T)\).
\(\mathsf{fHP}_{\mathcal{A}}\)
Igusa
cc z.f.
\(\mathsf{cfHP}_{\mathcal{A}}\)
Flag Hilbert--Poincaré series and Igusa zeta functions of hyperplane arrangements
By Josh Maglione
Flag Hilbert--Poincaré series and Igusa zeta functions of hyperplane arrangements
We define a class of multivariate rational functions associated with hyperplane arrangements called flag Hilbert--Poincaré series, and we show how these rational functions are connected to Igusa zeta functions. We report on a general self-reciprocity result and explore other connections within algebraic combinatorics. This is joint work with Christopher Voll.
