Unified quantum resource theory: A framework to understand quantum non-classicality.
Madhav Krishnan V
SID: 12910725
Supervisor : Min-Hsiu Hsieh
Co-Supervisor : Peter Rhode
Non-Classicality
Quantum
Classical
Entanglement
Superpositions
Non-local
Non-Contextual
Discord
Uncertainity
Resource Theories!
What is a resource?
Constraints
Resource
LOCC
Entanglement
Some R.T.s
- Entanglement
- Coherence
- Purity
- Asymmetry
- Thermodynamics
- Non-Contextuality
- Non-Markovianity
- Non-Locality
Unified framework:
Anshu, A. et al. arXiv:1708.00381
1. One-shot assisted concentration of coherence:
Collaborators : Min-Hsiu Hsieh, Eric Chitambar.
2. General one-shot resource concentration:
Collaborators : Min-Hsiu Hsieh, Eric Chitambar.
3. Error filteration using W-state encoding,
Collaborators : Peter Rhode, Austin Lund.
Resource theory of coherence
Free Entities
Expensive Entities
I={ρ=i∑pi∣i⟩⟨i∣}
\mathcal{I} = \left\{ \rho = \sum\limits_i p_i | i \rangle \langle i | \right\}
ρ=i∑pi∣i⟩⟨i∣
\rho\neq \sum\limits_i p_i | i \rangle \langle i |
Fixed basis
{∣i⟩}
\{ | i \rangle \}
∣i⟩⟨i∣
| i \rangle \langle i |
∣i⟩⟨i∣
| i \rangle \langle i |
ΛI:I→I
\Lambda_{\mathcal{I}} : \mathcal{I} \rightarrow \mathcal{I}
Λ:Λ∈/ΛI
\Lambda : \Lambda \notin \Lambda_{\mathcal{I}}
1. One-shot assisted concentration of coherence:
Measure of coherence
- Relative entropy of coherence
Cr(ρ)
C_r(\rho)
Cr(ρ)=δ∈IminS(ρ∥δ)
C_r(\rho) = \min\limits_{\delta\in \mathcal{I}} S( \rho \| \delta)
=S(Δ(ρ))−S(ρ),1
= S(\Delta(\rho)) - S(\rho),^1
where Δ dephases ρ (deletes off-diagonal terms)
\text{where } \Delta \text{ dephases } \rho \text{ (deletes off-diagonal terms)}
1.Winter, A., & Yang, D. : PRL 116.12, 120404, (2016)
Maximally coherent state
∣ΦM⟩=i=1∑MM1∣i⟩
| \Phi_M \rangle = \sum\limits_{i =1 }^M \frac{1}{\sqrt{M}} | i \rangle
Alice
Bob
(∣ψ⟩AB)⊗n
\left(| \psi \rangle^{AB} \right)^{\otimes n}
Λ (unrestricted)
\Lambda \text{ (unrestricted)}
ΛI
\Lambda_{\mathcal{I}}
One-way classical communication
Φ2⊗m
\Phi_2^{\otimes m}
Assisted distillation of coherence
Why assisted?
- Distributed scenario - remote target
- Easy to model
- For qubit, same as unrestricted communication
- Biological systems
- Quantum metrology
- Protocols requiring high coherence
Assisted distillation of coherence
| Optimal rate |
S(Δ(ρB))1
S(\Delta(\rho^B))^1
n→∞
n \rightarrow \infty
n=1
n = 1
1. Chitambar, E., et al. Physical review letters 116.7 (2016): 070402.
?
?
If Δ(ΦM)≻Δ(ψ):
\text{ If } \Delta(\Phi_M) \succ \Delta(\psi) :
Proof Sketch direct
ψ
\psi
ΦM
\Phi_M
ΛI
\Lambda_{\mathcal{I}}
=Smin(Δ(ψ))
=S_{min}(\Delta(\psi))
log2M
\log_2M
M
M
Winter, A., & Yang, D. : PRL 116.12, 120404, (2016)
⋅ψ
\cdot \psi
⋅Λ(
\cdot \Lambda(
⋅ΦM
\cdot \Phi_{\overline{M}}
⋅ψ
\cdot\overline{\psi}
ϵ
\epsilon
ϵ′
\epsilon^{\prime}
with ϵ′ error, optimal
\text{with } \epsilon^{\prime} \text{ error, optimal}
rate M∗, will be max
\text{rate } M^*, \text{ will be max }
over
\text{over}
ball
\text{ball}
around ψ
\text{around } \psi
log2M∗≥ψ∈b∗(ψ,ϵ)maxSmin(Δ(ψ))
\log_2M^* \geq \max\limits_{\overline{\psi} \in b_*(\psi, \epsilon)}S_{min}(\Delta(\overline{\psi}))
ψ
\psi
)
)
Λ
\Lambda
Λ
\Lambda
Proof Sketch: Converse
Starting with an operator identity :
\text{Starting with an operator identity :}
ΦMδΦM=M1ΦM
\Phi_M \delta \Phi_M = \frac{1}{M}\Phi_M
δ∈I
\delta \in \mathcal{I}
δ
\delta
∙ Assume the optimal rate with error ϵ is M, acheived by Λ
\bullet \text{ Assume the optimal rate with error } \epsilon \text{ is } M, \text{ acheived by } \Lambda
log2M≤γ∈Imin{−log2Tr(ψγ)}
\log_2M \leq \min\limits_{\gamma \in \mathcal{I}} \left\{ -\log_2\text{Tr}(\overline{\psi}\gamma) \right\}
ψ depends on Λ,ΦM,ψ
\overline{\psi} \text{ depends on } \Lambda, \Phi_M, \psi
ψ
\overline{\psi}
⋅ψ
\cdot \psi
⋅ψ
\cdot \overline{\psi}
=Smin(Δ(ψ))
= S_{min}(\Delta(\overline{\psi}))
2ϵ
2\epsilon
log2M≤ψ∈b∗′(ψ,2ϵ)maxSmin(Δ(ψ))
\log_2 M \leq \max\limits_{\overline{\psi} \in b_*^{\prime}(\psi, 2\epsilon)} S_{min}(\Delta(\overline{\psi}))
Pure state concentration
Cc(ψ,ϵ):=M∈Nmax{log2M:Λ∈OmaxF2(Λ(ψ),ΦM)≥1−ϵ}
C_c(\psi, \epsilon) := \max\limits_{M \in \mathbb{N}} \left\lbrace \log_2 M : \max\limits_{\Lambda \in \mathcal{O}} F^2(\Lambda(\psi), \Phi_{M}) \geq 1- \epsilon \right\rbrace
ψ∈b∗(ψ,ϵ)maxSmin(Δ(ψ))−δ≤Cc(ψ,ϵ)≤ψ∈b∗′(ψ,2ϵ)maxSmin(Δ(ψ))
\max\limits_{\overline{\psi} \in b_*(\psi, \epsilon)} S_{min}(\Delta(\overline{\psi})) - \delta \leq C_{c}(\psi, \epsilon) \leq \max\limits_{\overline{\psi} \in b^{\prime}_*(\psi, 2\epsilon)} S_{min}(\Delta(\overline{\psi}))
F2(Λ(ψ),ΦM)≥1−ϵ
F^2(\Lambda(\psi), \Phi_{M}) \geq 1- \epsilon
Λ∈OmaxF2(Λ(ψ),ΦM)≥1−ϵ
\max\limits_{\Lambda \in \mathcal{O}} F^2(\Lambda(\psi), \Phi_{M}) \geq 1- \epsilon
Ensemble concentration
Cc(E,ϵ):=M∈Nmax{log2M:{Λi}imaxF2(i∑piΛi(ψi),ΦM)≥1−ϵ}
C_c(\mathfrak{E}, \epsilon) := \max\limits_{M \in \mathbb{N}} \left\lbrace \log_2M : \max\limits_{ \lbrace\Lambda_{i}\rbrace_i} F^{2}\left(\sum\limits_{i}p_i\Lambda_i(\psi_i), \Phi_{M} \right) \geq 1 - \epsilon \right\rbrace
∙ Let E={pi,ψi} be a pure state ensemble
\bullet \text{ Let }\mathfrak{E} = \{ p_i, \psi_i \} \text{ be a pure state ensemble}
E∈b(E,ϵ)maxFminΔ(E)−δ≤Cc(E,ϵ)≤E∈b(E,2ϵ)maxFminΔ(E)
\max\limits_{\overline{\mathfrak{E}} \in b(\mathfrak{E}, \epsilon)}F_{min}^{\Delta}(\overline{\mathfrak{E}}) - \delta \leq C_{c}(\mathfrak{E}, \epsilon) \leq \max\limits_{\overline{\mathfrak{E}} \in b(\mathfrak{E}, 2\epsilon)} F_{min}^{\Delta}(\overline{\mathfrak{E}})
where, FminΔ(E)=ψi∈EminSmin(Δ(ψi))
\text{where, } F^{\Delta}_{min}(\mathfrak{E}) = \min\limits_{\psi_i \in \mathfrak{E}} S_{min}(\Delta(\psi_i))
Asymptotic limit
∙ The coherence of assistance is defined as,
\bullet \text{ The coherence of assistance is defined as,}
Da(ρB)=Eρ={pi,ψi}imaxi∑piS(Δ(ψi))=S(Δ(ρB))1
D_a(\rho^B) = \max\limits_{\mathfrak{E}_{\rho}=\lbrace p_i, \psi_i \rbrace_i} \sum\limits_i p_i S(\Delta(\psi_i)) = S(\Delta(\rho^B))^1
Chitambar, E., et al. Physical review letters 116.7 (2016): 070402.
Ca(ρ,ϵ)=EρmaxCc(Eρ,ϵ)
C_a(\rho, \epsilon) = \max\limits_{\mathfrak{E}_{\rho}} C_{c}(\mathfrak{E}_{\rho}, \epsilon)
ϵ→0limn→∞limn1Ca(ρ⊗n,ϵ)=n→∞limn1Da(ρ⊗n)
\lim\limits_{\epsilon \rightarrow 0}\lim\limits_{n \rightarrow \infty}\frac{1}{n} C_a(\rho^{\otimes n}, \epsilon) = \lim\limits_{n \rightarrow \infty}\frac{1}{n}D_a(\rho^{\otimes n})
References:
-
Streltsov, A., Adesso, G., & Plenio, M. B. (2017). Reviews of Modern Physics, 89(4), 041003. -
Baumgratz, T., Cramer, M., & Plenio, M. B. (2014). Phys. Rev. Lett., 113(14), 140401. -
Winter, A., & Yang, D. (2016). Phys. Rev. Lett., 116(12), 120404. -
Chitambar, E., Streltsov, A., Rana, S., Bera, M. N., Adesso, G., & Lewenstein, M. (2016) Phys. Rev. Lett., 116(7), 070402. -
Buscemi, F., & Datta, N. (2013). IEEE Trans. Inf. Th., 59(3), 1940-1954.
2. General one-shot resource concentration
ΦMδΦM=M1ΦM∀δ∈I
\Phi_M \delta \Phi_M = \frac{1}{M}\Phi_M \hspace{0.5cm} \forall \delta \in \mathcal{I}
For coherence :
Arbitrary R.T. with MRS
Φn=Φ2⊗n,
\Phi^n = \Phi_2^{\otimes n} ,
ΦnδΦn≤2n1Φn∀δ∈F
\Phi^n \delta \Phi^n \leq \frac{1}{2^n}\Phi^n \hspace{0.5cm} \forall \delta \in \mathcal{F}
Leads to distillation upper-bound
Theorem: For any R.T. such that
ΦnγΦn≤2n1Φn∀γ∈F
\Phi^n \gamma \Phi^n \leq \frac{1}{2^n}\Phi^n \hspace{0.5cm} \forall \gamma \in \mathcal{F}
The optimal distillation rate m with error
ρ→Φm
\rho \rightarrow \Phi^m
m≤ρ∈B∗′(ρ,2ϵ)maxGmin(ρ)
m \leq \max\limits_{\overline{\rho} \in B_*^{\prime}(\rho, 2\epsilon)} G_{min}(\overline{\rho})
where,
Gmin(ρ)=γ∈Fmin{−log2Tr(ργ)}
G_{min}(\rho) = \min\limits_{\gamma \in \mathcal{F}} \left\{ -\log_2 \text{Tr}(\rho \gamma) \right\}
ϵ
\epsilon
For
is
| R.Theory | Entanglement | Coherence | Purity |
|---|---|---|---|
Gmin(ψ)
G_{min}(\psi)
Smin(ρψ)
S_{min}(\rho_{\psi})
Smin(Δ(ψ))
S_{min}(\Delta({\psi}))
m
m
Concentration of m copies of MRS from a pure state
ψ
\psi
Gmin(ρ)
G_{min}(\rho)
Smin(Δ(ρ))
S_{min}(\Delta({\rho}))
- General achievability in terms of
Gmin(ρ)
G_{min}(\rho)
Λ(X)=Tr[(I−ψ)X]π+Tr[ψX]Φm
\Lambda(X) = \text{Tr} [(I-\psi)X]\pi + \text{Tr}[\psi X] \Phi^m
A map we are trying out
Ongoing work
- Considering resource non-generating maps
- Understanding which R.T.s can be included in our framework
ϵ
\epsilon
3. Error filteration using W-state encoding,
- Error correction - redundancy
Eg:
α∣0⟩+β∣1⟩→α∣00...0⟩+β∣11...1⟩
\alpha | 0 \rangle + \beta |1 \rangle \rightarrow \alpha | 00...0 \rangle + \beta |11...1 \rangle
GHZ states:
21(∣00...0⟩+∣11...1⟩)
\frac{1}{\sqrt{2}} ( | 00...0 \rangle + |11...1 \rangle )
- Error correction
- Cryptography
- Communication
- Not robust against loss.
W-states
- Superposition of single excitation
Eg:
∣W3⟩=31(∣100⟩+∣010⟩+∣001⟩)
|W^3 \rangle = \frac{1}{\sqrt{3}} (|1 0 0 \rangle + | 0 1 0 \rangle + | 0 0 1 \rangle )
|W_i^N \rangle = \hat\mathrm{QFT_N} | vac \rangle
- Single photon passive linear optics system
- Atomic Ensembles
Tri(∣WN⟩⟨WN∣)=NN−1∣WN−1⟩⟨WN−1∣+N1∣vac⟩⟨vac∣
\text{Tr}_i(|W^N \rangle \langle W^N |) = \frac{N-1}{N} | W^{N-1} \rangle \langle W^{N-1} | + \frac{1}{N} | vac \rangle \langle vac |
Protocol
Random phase error on each mode
eiθ0
e^{i\theta_0}
eiθ1
e^{i\theta_1}
eiθ2
e^{i\theta_2}
0
0
1
1
2
2
Eθ:
\mathcal{E}^{\vec\theta} :
xθ0
\phantom{x}^{\theta_0}
xθ1
\phantom{x}^{\theta_1}
xθ2
\phantom{x}^{\theta_2}
Normally distributed as
N(δ,0)
N(\delta, 0)
∣W⟩
| W \rangle
∣Wθ⟩
| W^{\vec\theta} \rangle
Averaging the error channel gives
Eθ(∣W⟩⟨W∣)=∫p(θ)∣Wθ⟩⟨Wθ∣dθ
\overline{\mathcal{E}^{\vec\theta}} (| W \rangle \langle W |) = \int p(\vec\theta) | W^{\vec\theta} \rangle \langle W^{\vec\theta} | d\theta
=e−δ2∣W⟩⟨W∣+(1−e−δ2)Δ(∣W⟩⟨W∣)
= e^{-\delta^2} | W \rangle \langle W | + (1 - e^{-\delta^2}) \Delta(|W \rangle \langle W |)
Dephasing channel
PH=η+(1−η){e−δ2+N2(1−e−δ2)}
P_{H} =\eta + (1 -\eta) \left\{ e^{-\delta^2} + \frac{2}{N}(1 - e^{-\delta^2}) \right\}
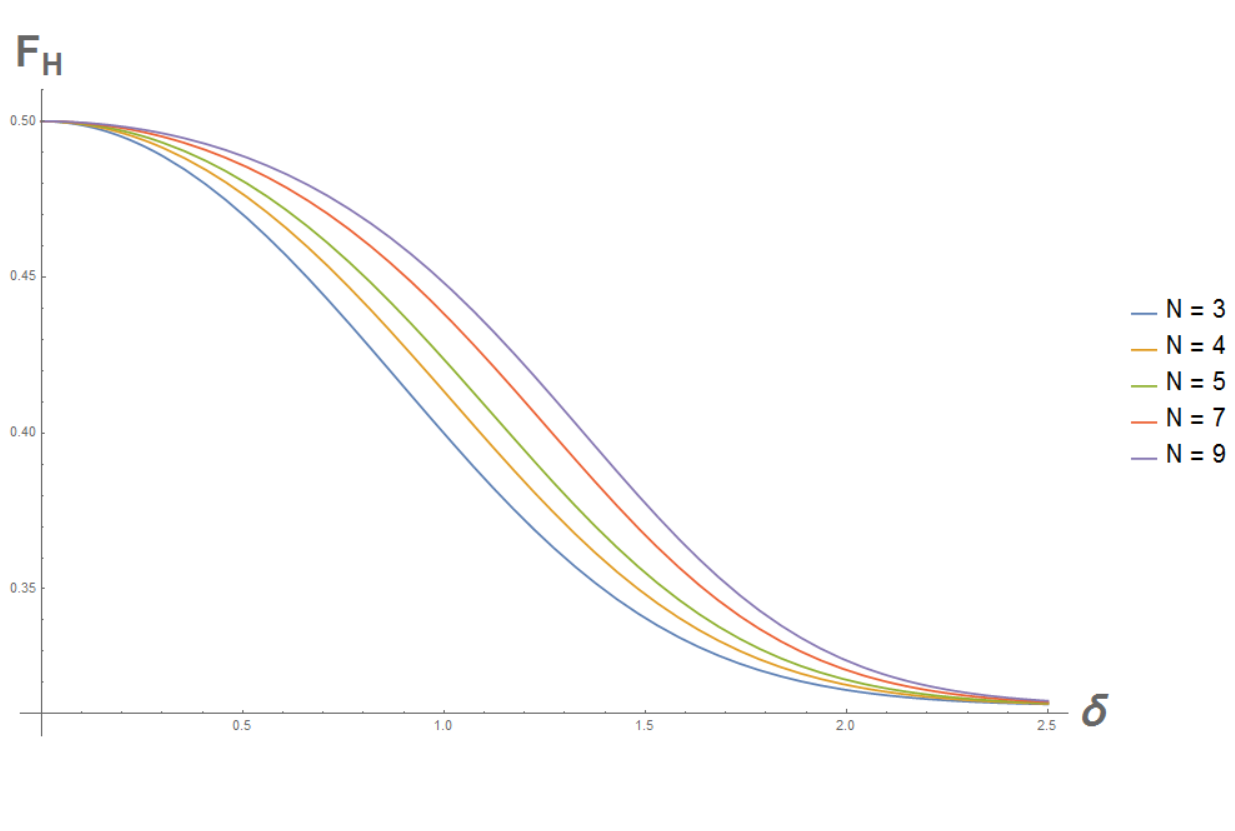
With a loss rate
η
\eta
, the heralding probability will be
For a logical input
∣L⟩=α∣0⟩+β∣1⟩
| L \rangle = \alpha |0 \rangle + \beta | 1 \rangle
FH=(1−η)×e−δ2+N2(1−e−δ2)e−δ2+(1−e−δ2)N1+2∣α∣2∣β∣2
F_H = (1 - \eta) \times \frac{e^{-\delta^2} + (1 - e^{-\delta^2})\frac{1 + 2|\alpha|^2|\beta|^2}{N} }{e^{-\delta^2} + \frac{2}{N}(1 - e^{-\delta^2}) }
, the heralded fidelity is
η=0.5,∣α∣=21
\eta = 0.5, |\alpha| = \frac{1}{\sqrt{2}}
Ongoing work
- Relation to quantum memory architectures
- Reframing in terms of T1, T2 times.
Conclusions
- Calculated bounds forone-shot assisted coherence concentration and recovered known asymptotic limits.
- Proved a generalized resource distillation upper-bound and working on the achievability.
- Developed a protocol for W-state encoded error correction and exploring applications to quantum memories
Future Directions
- Open problems in coherence R.T.
- Generalized resource framework
- Quantum optical non-classicality as a resource theory
- Cluster states - Entanglement swapping
Thank you!
Method of the proof
- The assisted distillation task can be broken down into two parts
- Alice performs POVM on her part of the shared state and sends the result to Bob.
ψAB
\psi^{AB}
{PiA}
\{ P^A_i\}
2. Bob performs an Incoherent operation on his part of the state based on Alice's outcome.
Λi
\Lambda_i
- The best strategy is for Alice to create an optimal ensemble on Bob's side and for Bob to optimally distill this ensemble
- We need to calculate the optimal concentration rate for an ensemble of pure states
E={pi,ψi}
\mathfrak{E} = \{ p_i, \psi_i \}
Unified quantum resource theory: A framework to understand quantum non-classicality. Madhav Krishnan V SID: 12910725 Supervisor : Min-Hsiu Hsieh Co-Supervisor : Peter Rhode
Candidature assesment
By madhav_krishnan
Candidature assesment
Cand assesment
- 127
