Underline = Cmd+u

More symplectic structures

on the space of space curves

Joint with Martin Bauer & Peter Michor

September 2024 @ TU Wien

Sadashige Ishida (ISTA)

Introduction to myself

Sadashige, a PhD student @ ISTA (near Vienna)

Introduction to myself

Geometry & Dynamics in Math, Physics, CS

Sadashige, a PhD student @ ISTA (near Vienna)

Introduction to myself

Geometry & Dynamics in Math, Physics, CS

Sadashige, a PhD student @ ISTA (near Vienna)

Dynamics as \(\infty\)-dim geometry

e.g. fluids, optimal transport, shapes

Introduction to myself

Geometry & Dynamics in Math, Physics, CS

Sadashige, a PhD student @ ISTA (near Vienna)

Dynamics as \(\infty\)-dim geometry

e.g. fluids, optimal transport, shapes

+ their finite-dim approximations

a.k.a. discretization

Introduction to myself

Looking for a postgraduate/research fellow position starting late 2025

Dynamics as \(\infty\)-dim geometry

e.g. fluids, optimal transport, shapes

Geometry & Dynamics in Math, Physics, CS

+ their finite-dim approximations

a.k.a. discretization

Sadashige, a PhD student @ ISTA (near Vienna)

Overview

On the space of space curves, only one symplectic structure was known.

We found more.

Symplectic structure is a sandbox

for Hamiltonian dynamics

Space with

a symplectic structure \(\Omega\)

A function \(H\)

Dynamics \(V_H\)

Symplectic structure is a sandbox

for Hamiltonian dynamics

A symplectic structure \(\Omega\) is a 2-form on a manifold \(X\) s.t.

Symplectic structure is a sandbox

for Hamiltonian dynamics

A symplectic structure \(\Omega\) is a 2-form on a manifold \(X\) s.t.

• closed i.e. \(d\Omega=0\) 

Symplectic structure is a sandbox

for Hamiltonian dynamics

A symplectic structure \(\Omega\) is a 2-form on a manifold \(X\) s.t.

• non-degenerate i.e. the map $$\flat: TX\to T^*X$$ $$  \qquad    v\to \Omega(v, \cdot )$$ is injective

• closed i.e. \(d\Omega=0\) 

Symplectic structure is a sandbox

for Hamiltonian dynamics

Symplectic structure is a sandbox

for Hamiltonian dynamics

For \(H \colon X\to \mathbb{R}\),

Hamiltonian vector field \(V_H\in \Gamma(TM)\) is the one

$$ dH = \Omega(V_H, \cdot). $$

Symplectic structure is a sandbox

for Hamiltonian dynamics

For \(H \colon X\to \mathbb{R}\),

Hamiltonian vector field \(V_H\in \Gamma(TM)\) is the one

$$ dH = \Omega(V_H, \cdot). $$

The Hamiltonian dynamics

$$\dot x=V_H(x).$$

Why Hamiltonian dynamics?

Has nice properties.

E.g.

• \(H\) is preserved in dynamics.
• If \(H\) is invariant under some group action, there is a conserved quantity.

Spaces with symplectic structures

and Hamiltonian dynamics

\(T^*M^n\)

\(T^*\operatorname{SDiff}(M)\)

Celestial mechanics

\(C^\infty(M,\mathbb{C})\)

 Schrödinger equation

 Incompressible fluids

Spaces with symplectic structures

and Hamiltonian dynamics

\(T^*M^n\)

\(T^*\operatorname{SDiff}(M)\)

\(C^\infty(M,\mathbb{C})\)

 Schrödinger equation

 Incompressible fluids

Space curves

Celestial mechanics

The space of space curves

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)=\{ c\colon \mathbb{S}^1\to\mathbb{R}^3, \partial_\theta c\neq 0 \ \forall \theta\in\mathbb{S}^1 \}$$

Parametrized curves

The space of space curves

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)=\{ c\colon \mathbb{S}^1\to\mathbb{R}^3, \partial_\theta c\neq 0 \ \forall \theta\in\mathbb{S}^1 \}$$

Parametrized curves

$$T_c\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)=C^\infty(\mathbb{S}^1,\mathbb{R}^3)$$

Tangent space

The space of space curves

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\operatorname{Diff}^+(\mathbb{S}^1)$$

Unparametrized curves a.k.a. shape space

The space of space curves

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\operatorname{Diff}^+(\mathbb{S}^1)$$

Unparametrized curves a.k.a. shape space

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)$$

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)$$

\[\Bigg\downarrow \pi\]

$$\operatorname{Diff}^+(\mathbb{S}^1)$$

The space of space curves

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\operatorname{Diff}^+(\mathbb{S}^1)$$

Unparametrized curves a.k.a. shape space

Tangent space

$$T_{[c]}\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=T_c\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\ker d\pi_c$$

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)$$

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)$$

\[\Bigg\downarrow \pi\]

$$\operatorname{Diff}^+(\mathbb{S}^1)$$

The space of space curves

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\operatorname{Diff}^+(\mathbb{S}^1)$$

Unparametrized curves a.k.a. shape space

Tangent space

$$T_{[c]}\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=T_c\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\ker d\pi_c$$

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)$$

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)$$

\[\Bigg\downarrow \pi\]

$$\operatorname{Diff}^+(\mathbb{S}^1)$$

\(\{a.\partial_\theta c \mid a\in C^\infty(\mathbb{S}^1)\}\)

curve tangent

The space of space curves

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\operatorname{Diff}^+(\mathbb{S}^1)$$

Unparametrized curves a.k.a. shape space

Tangent space

$$T_{[c]}\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)=T_c\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)/\ker d\pi_c$$

$$\eqsim\{h \in T_c \operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)\mid h\perp \partial_\theta c \}$$

$$\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)$$

$$\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)$$

\[\Bigg\downarrow \pi\]

$$\operatorname{Diff}^+(\mathbb{S}^1)$$

\(\{a.\partial_\theta c \mid a\in C^\infty(\mathbb{S}^1)\}\)

curve tangent

Symplectic structure

\[\Omega_c\colon T_c\operatorname{Imm}\times T_c\operatorname{Imm}\to \mathbb{R}\]

\[\Omega_c(h,k)=\int_{\mathbb{S}^1}\langle D_s c\times h, k\rangle ds\]

where

\[D_s = \frac{\partial_\theta }{|\partial_\theta c|}, \quad ds = |\partial_\theta c| d\theta \]

Closed (\(d\Omega=0\)),

Degenerate with

\(\ker \Omega_{[c]}= \ker d\pi_c= \{a. D_s c\mid a \in C^\infty(\mathbb{S}^1)\}\)

Symplectic?

On \(\operatorname{Imm}(\mathbb{S}^1,\mathbb{R}^3)\),

Canonical symplectic structure on \(\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)\)

The Marsden-Weinstein structure

\[\Omega_{[c]}\colon T_{[ c]}\operatorname{UImm}\times T_{[ c]}\operatorname{UImm}\to \mathbb{R},\]

Canonical symplectic structure on \(\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)\)

\[\Omega_{[c]}\colon T_{[ c]}\operatorname{UImm}\times T_{[ c]}\operatorname{UImm}\to \mathbb{R},\]

The Marsden-Weinstein structure

\[\Omega_{[c]}([h],[k])=\int_{\mathbb{S}^1}\langle D_s c\times h, k\rangle ds\]

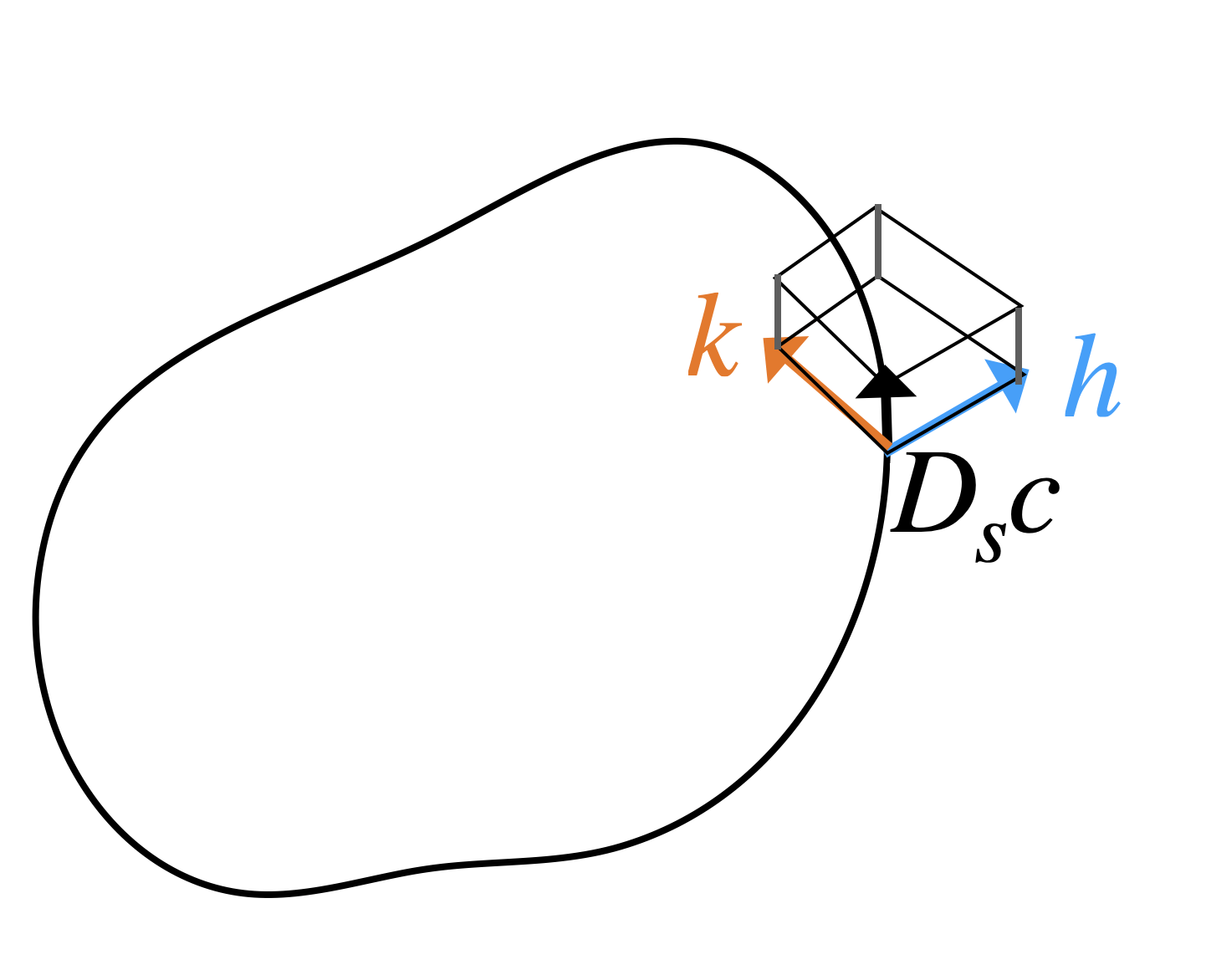
where

\[D_s = \frac{\partial_\theta }{|\partial_\theta c|}, \quad ds = |\partial_\theta c| d\theta \]

unit tangent

Canonical symplectic structure on \(\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)\)

\[\Omega_{[c]}\colon T_{[ c]}\operatorname{UImm}\times T_{[ c]}\operatorname{UImm}\to \mathbb{R},\]

Symplectic!

The Marsden-Weinstein structure

\[\Omega_{[c]}([h],[k])=\int_{\mathbb{S}^1}\langle D_s c\times h, k\rangle ds\]

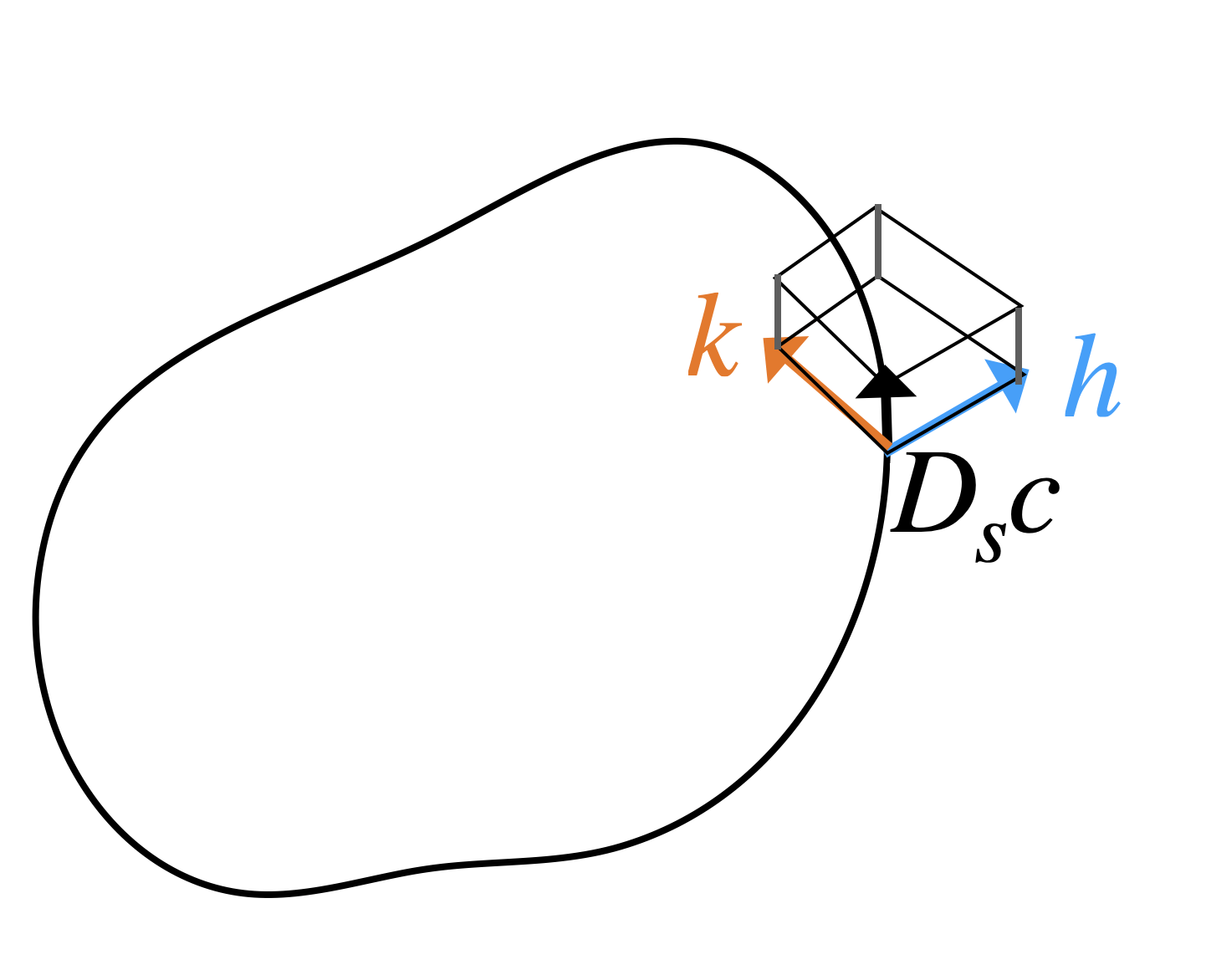
where

\[D_s = \frac{\partial_\theta }{|\partial_\theta c|}, \quad ds = |\partial_\theta c| d\theta \]

unit tangent

Canonical symplectic structure on \(\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)\)

\[\Omega_{[c]}\colon T_{[ c]}\operatorname{UImm}\times T_{[ c]}\operatorname{UImm}\to \mathbb{R},\]

Closed \(d\Omega=0\),

Symplectic!

The Marsden-Weinstein structure

\[\Omega_{[c]}([h],[k])=\int_{\mathbb{S}^1}\langle D_s c\times h, k\rangle ds\]

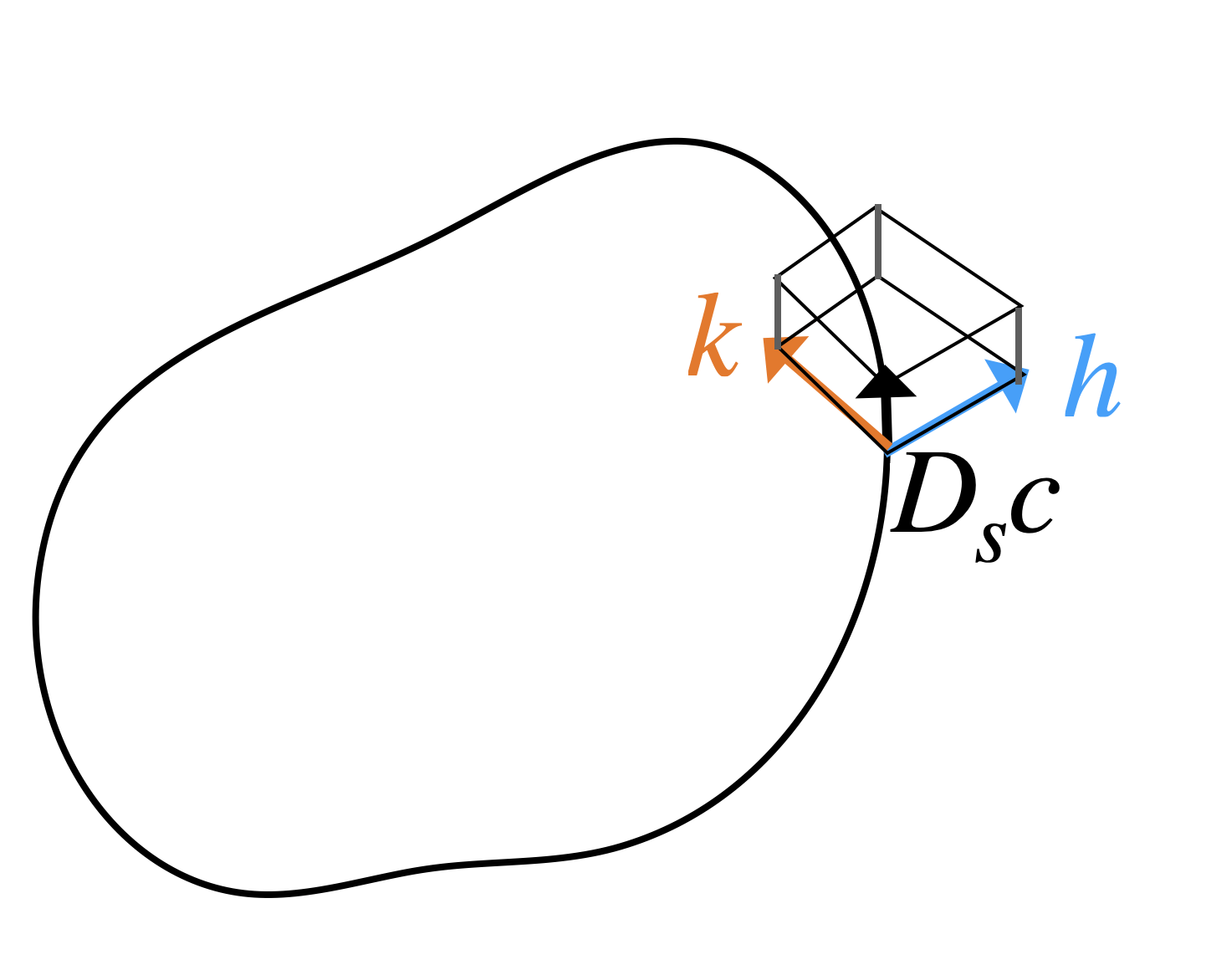
where

\[D_s = \frac{\partial_\theta }{|\partial_\theta c|}, \quad ds = |\partial_\theta c| d\theta \]

unit tangent

Canonical symplectic structure on \(\operatorname{UImm}(\mathbb{S}^1,\mathbb{R}^3)\)

\[\Omega_{[c]}\colon T_{[ c]}\operatorname{UImm}\times T_{[ c]}\operatorname{UImm}\to \mathbb{R},\]

Closed \(d\Omega=0\),

Symplectic!

The Marsden-Weinstein structure

\[\Omega_{[c]}([h],[k])=\int_{\mathbb{S}^1}\langle D_s c\times h, k\rangle ds\]

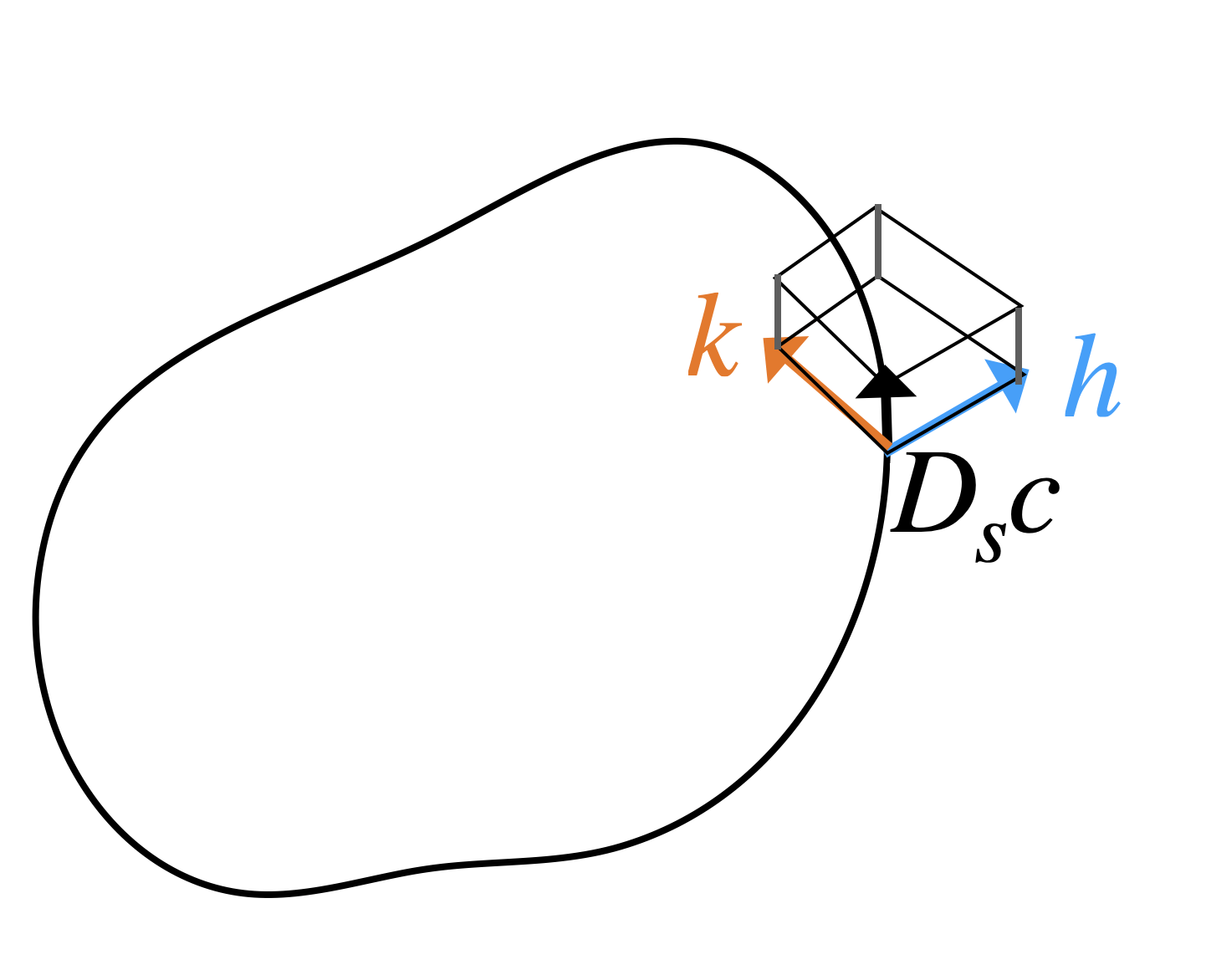
where

\[D_s = \frac{\partial_\theta }{|\partial_\theta c|}, \quad ds = |\partial_\theta c| d\theta \]

Non-degenerate \(\ker \Omega_{[c]}=[0]=\{a.D_s c \mid a \in C^\infty(\mathbb{S}^1)\}\)

unit tangent

Hamiltonian flow

E.g. 

Hamiltonian \(H = \operatorname{Length}([c])\)

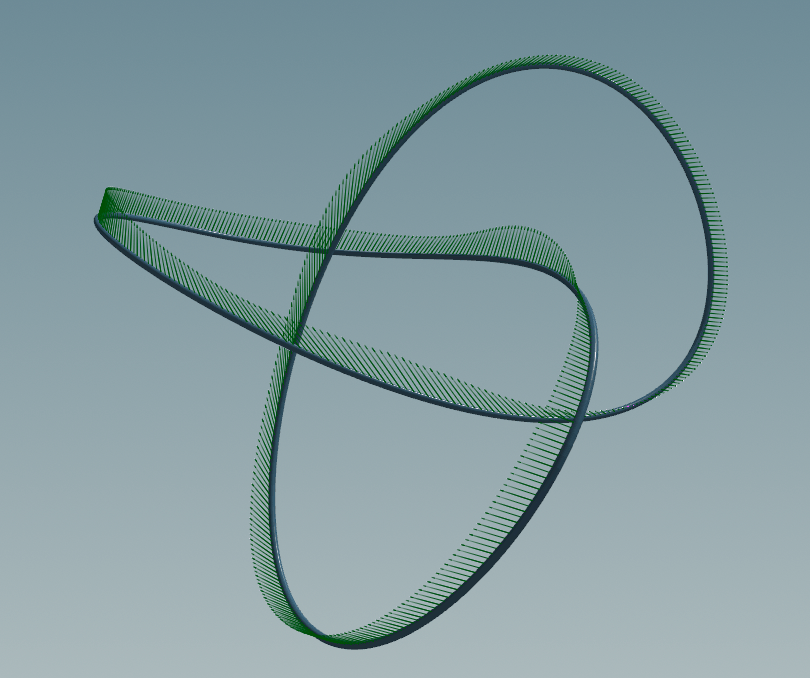
Binormal flow

Dynamics \(V_H=[D_s c \times D_s^2 c]\)

Hamiltonian flow

E.g. 

Hamiltonian \(H = \operatorname{Length}([c])\)

Binormal flow

Dynamics \(V_H=[D_s c \times D_s^2 c]\)

A completely integrable system

More symplectic structures?

More symplectic structures?

Remember

A symplectic structure \(\Omega\) defines a Hamiltonian dynamics \(V_H\) via $$dH=\Omega(V_H,\cdot).$$

More symplectic structures?

A different \(\Omega\) defines different \(V_H\).

Remember

A symplectic structure \(\Omega\) defines a Hamiltonian dynamics \(V_H\) via $$dH=\Omega(V_H,\cdot).$$

More symplectic structures?

A different \(\Omega\) defines different \(V_H\).

But only one \(\Omega\) is known on \(\operatorname{UImm}\).

Remember

A symplectic structure \(\Omega\) defines a Hamiltonian dynamics \(V_H\) via $$dH=\Omega(V_H,\cdot).$$

More symplectic structures?

A different \(\Omega\) defines different \(V_H\).

We found more.

But only one \(\Omega\) is known on \(\operatorname{UImm}\).

Remember

A symplectic structure \(\Omega\) defines a Hamiltonian dynamics \(V_H\) via $$dH=\Omega(V_H,\cdot).$$

Hint 1. Trend in shape analysis

Hint 1. Trend in shape analysis

a Riemannian metric on \(\operatorname{Imm}\)

$$ g(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

Hint 1. Trend in shape analysis

a Riemannian metric on \(\operatorname{Imm}\)

$$ g(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

a suitable operator

$$L\colon T\operatorname{Imm}\to T\operatorname{Imm}. $$

Hint 1. Trend in shape analysis

a Riemannian metric on \(\operatorname{Imm}\)

$$ g(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

New metric $$g^L(h,k)\coloneqq g(h,Lk) $$

a suitable operator

$$L\colon T\operatorname{Imm}\to T\operatorname{Imm}. $$

e.g. \(L_c=\operatorname{id}_c\),

$$g^{\operatorname{id}}_c(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

Hint 1. Trend in shape analysis

e.g. \(L_c=\operatorname{id}_c\),

$$g^{\operatorname{id}}_c(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

e.g. \(L_c=\lambda(c)\) with a conformal factor \(\lambda\colon \operatorname{Imm}\to \mathbb{R}_{>0}\)

$$g^{\lambda}_c(h,k)=\lambda(c)\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

Hint 1. Trend in shape analysis

e.g. \(L_c=\operatorname{id}_c\),

$$g^{\operatorname{id}}_c(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

e.g. Sobolev-type differential operator  \(L_c=\operatorname{id}-D_s^2\)

$$g^{\operatorname{id}-D_s^2}_c(h,k)=\int_{\mathbb{S}^1} \langle h,k\rangle -\langle h, D_s^2 k \rangle ds = \int_{\mathbb{S}^1} \langle h,k\rangle + \langle D_s h, D_s k \rangle ds $$

Hint 1. Trend in shape analysis

e.g. \(L_c=\lambda(c)\) with a conformal factor \(\lambda\colon \operatorname{Imm}\to \mathbb{R}_{>0}\)

$$g^{\lambda}_c(h,k)=\lambda(c)\int_{\mathbb{S}^1} \langle h,k\rangle ds$$

Hint 2. Liouville form

\(\Omega\) is exact

i.e. there is a 1-form \(\Theta\) s.t. \(\Omega=d\Theta\).

Hint 2. Liouville form

\(\Omega\) is exact

\(\Theta\) is given by 

$$\Theta_{[c]}([h])=\frac{1}{3}\int_{\mathbb{S}^1}\langle D_s c\times c, h\rangle  ds=g^{\operatorname{id}}\left(\frac{1}{3}D_s c\times c, h\right).$$

i.e. there is a 1-form \(\Theta\) s.t. \(\Omega=d\Theta\).

Hint 1 + Hint 2

Let's define a 1-form

$$\Theta_{[c]}^{\color{blue}L}([h])\coloneqq g^{\color{blue}L}\left(\frac{1}{3}D_s c\times c, h\right)= \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, {\color{blue}L} h\rangle ds $$

Hint 1 + Hint 2

and a 2-form $$\Omega^L\coloneqq d\Theta^L$$

Let's define a 1-form

$$\Theta_{[c]}^{\color{blue}L}([h])\coloneqq g^{\color{blue}L}\left(\frac{1}{3}D_s c\times c, h\right)= \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, {\color{blue}L} h\rangle ds $$

$$\Omega^L\coloneqq d\Theta^L$$

Does \(\Omega^L\) define a symplectic structure on \(\operatorname{UImm}\)?.

Hint 1 + Hint 2

with  \(\Theta_{[c]}^L([h])\coloneqq \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, L h\rangle ds \)

Closeness. OK because it is exact  \(d\Omega^L=dd\Theta^L=0\)

$$\Omega^L\coloneqq d\Theta^L$$

Does \(\Omega^L\) define a symplectic structure on \(\operatorname{UImm}\)?.

Hint 1 + Hint 2

with  \(\Theta_{[c]}^L([h])\coloneqq \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, L h\rangle ds \)

Closeness. OK because it is exact  \(d\Omega^L=dd\Theta^L=0\)

$$\Omega^L\coloneqq d\Theta^L$$

Does \(\Omega^L\) define a symplectic structure on \(\operatorname{UImm}\)?.

Hint 1 + Hint 2

with  \(\Theta_{[c]}^L([h])\coloneqq \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, L h\rangle ds \)

Non-degeneracy on \(\operatorname{UImm}\) ?

i.e. \(\ker \Omega_c^L = [0] = \{a. D_s c \mid a \in C^\infty(\mathbb{S}^1)\}\)

Closeness. OK because it is exact  \(d\Omega^L=dd\Theta^L=0\)

$$\Omega^L\coloneqq d\Theta^L$$

Does \(\Omega^L\) define a symplectic structure on \(\operatorname{UImm}\)?.

Non-degeneracy on \(\operatorname{UImm}\) ?

i.e. \(\ker \Omega_c^L = [0] = \{a. D_s c \mid a \in C^\infty(\mathbb{S}^1)\}\)

Need to check case by case.

Hint 1 + Hint 2

with  \(\Theta_{[c]}^L([h])\coloneqq \frac{1}{3} \int_{\mathbb{S}^1} \langle D_s c \times c, L h\rangle ds \)

Symplectic structure

induced by a conformal factor

$$\Omega^\lambda\coloneqq d\Theta^\lambda$$

Theorem

With a conformal factor \(\lambda\colon \operatorname{UImm}\to \mathbb{R}_{>0} \),

is a symplectic structure on \(\operatorname{UImm}\) 

Symplectic structure

induced by a conformal factor

$$\Omega^\lambda\coloneqq d\Theta^\lambda$$

Theorem

With a conformal factor \(\lambda\colon \operatorname{UImm}\to \mathbb{R}_{>0} \),

is a symplectic structure on \(\operatorname{UImm}\) 

if \(\Theta^\lambda\) is NOT invariant under scaling (\(c\to c+t c\) with \(t \in \mathbb{R}^\times\)).

Symplectic structure

induced by a conformal factor

$$\Omega^\lambda\coloneqq d\Theta^\lambda$$

Theorem

With a conformal factor \(\lambda\colon \operatorname{UImm}\to \mathbb{R}_{>0} \),

is a symplectic structure on \(\operatorname{UImm}\) 

If \(\Theta^\lambda\) is invariant under scaling, \(\Omega^\lambda\) is NOT symplectic on \(\operatorname{UImm}\),

but becomes symplectic in another space! (Check out our paper)

if \(\Theta^\lambda\) is NOT invariant under scaling (\(c\to c+t c\) with \(t \in \mathbb{R}^\times\)).

Open problem

Is there a non-conformal \(L\) making \(\Omega^L\coloneqq d\Theta^L\) symplectic?

Open problem

Is there a non-conformal \(L\) making \(\Omega^L\coloneqq d\Theta^L\) symplectic?

Conjecture

Squared curvature \(L|_\theta=1+\kappa^2_c(\theta)\) defines a symplectic structure.

Open problem

Is there a non-conformal \(L\) making \(\Omega^L\coloneqq d\Theta^L\) symplectic?

Conjecture

Squared curvature \(L|_\theta=1+\kappa^2_c(\theta)\) defines a symplectic structure.

Result in preparation

Squared scale \(L|_\theta=|c(\theta)|^2\) defines a symplectic structure.

Simulation of Hamiltonian dynamics

Remember, different symplectic structures induce different dynamics from the same Hamiltonian.

(Marsden-Weinstein)

\(\Omega_c^{\operatorname{id}}\)

\(\Omega_c^{\operatorname{Length}(c)^{-1/10}}\)

\(\Omega_c^{\operatorname{Length}(c)^{2}}\)

Simulation of Hamiltonian dynamics

Hamiltonian dynamics \(V_H\) of total squared-scale  $$H(c)=\int_{\mathbb{S}^1}|c|^2 ds$$

\(H\) is preserved in all of them.

Future work A

Do the same machinery to find more symplectic structures on other \(\infty\)-dim manifolds.

Future work

Future work

Our paper has a disclaimer

We do not guarantee any correctness of (even short-time behavior) dynamics in our simulation

Future work

Symplectic structure on the space of discrete space curves?

Toward a symplectic integrator for space curves

Our paper has a disclaimer

We do not guarantee any correctness of (even short-time behavior) dynamics in our simulation

Future work

Symplectic structure on the space of discrete space curves?

Toward a symplectic integrator for space curves

Looking for a collaborator!

Our paper has a disclaimer

We do not guarantee any correctness of (even short-time behavior) dynamics in our simulation

Thanks!

Preprint: arXiv 2407.19908

Sadashige.Ishida@ist.ac.at