Chapter 24
\text{Chapter 24}
Chapter 24
\text{Chapter 24}
Chapter 24
\text{Chapter 24}
Chapter 24
\text{Chapter 24}
An electron is released from rest at the origin in a uniform electric field that points in the
\text{An electron is released from rest at the origin in a uniform electric field that points in the }
positive x direction and has a magnitude of 850 N/C. What is the change in the electric
\text{positive $x$ direction and has a magnitude of $850 \mathrm{~N} / \mathrm{C}$. What is the change in the electric }
potential energy of the electron-field system when the electron moves a distance of 2.5 m ?
\text{potential energy of the electron-field system when the electron moves a distance of $2.5 \mathrm{~m}$ ?}
B) +3.4×10−16 J
\text{B) $+3.4 \times 10^{-16} \mathrm{~J}$}
A) −3.4×10−16 J
\text{A) $-3.4 \times 10^{-16} \mathrm{~J}$}
C) −1.4×10−16 J
\text{C) $-1.4 \times 10^{-16} \mathrm{~J}$}
D) +1.4×10−16 J
\text{D) $+1.4 \times 10^{-16} \mathrm{~J}$}
E) −5.4×10−16 J
\text{E) $-5.4 \times 10^{-16} \mathrm{~J}$}
An electron is released from rest at the origin in a uniform electric field that points in the
\text{An electron is released from rest at the origin in a uniform electric field that points in the }
positive x direction and has a magnitude of 850 N/C. What is the change in the electric
\text{positive $x$ direction and has a magnitude of $850 \mathrm{~N} / \mathrm{C}$. What is the change in the electric }
potential energy of the electron-field system when the electron moves a distance of 2.5 m ?
\text{potential energy of the electron-field system when the electron moves a distance of $2.5 \mathrm{~m}$ ?}
ΔU=−Wfield =−∫ifF⋅ds=−∫ifqE⋅ds
\Delta U=-W_{\text {field }}=-\int_{i}^f \vec{F} \cdot d \vec{s}=-\int_i^f q \vec{E} \cdot d \vec{s}
ΔU=−Wfield =−∫if(−e)E⋅ds⋅cos1800=−∫ifeE⋅ds=−eEd
\Delta U=-W_{\text {field }}=-\int_i^f (-e) {E} \cdot d{s}\cdot \cos 180^0=-\int_i^f e E \cdot d{s}=-eEd
ΔU=−1.6×10−19(850)(2.5)=−3.4×10−16 J
\Delta U=-1.6 \times 10^{-19}(850)(2.5)=-3.4 \times 10^{-16} \mathrm{~J}
⋅
\cdot
⋅
\cdot
ds
d\vec{s}
E
\vec{E}
−
-
−
-
initial
\text{initial}
final
\text{final}
Answer A
\text{Answer A}
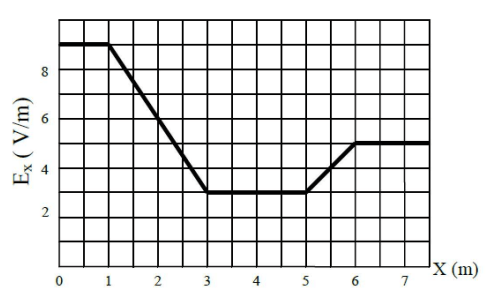
The figure shows a plot for the electric field Ex as a function of x. Find the magnitude of the
\text{The figure shows a plot for the electric field $E_x$ as a function of $x$. Find the magnitude of the }
potential difference between the points x=2.00 m and x=6.00 m.
\text{potential difference between the points $x=2.00 \mathrm{~m}$ and $x=6.00 \mathrm{~m}$.}
A) 14.5 V
\text{A) 14.5 V}
B) 12.5 V
\text{B) 12.5 V}
C) 10.0 V
\text{C) 10.0 V}
D) 16.5 V
\text{D) 16.5 V}
E) 11.0 V
\text{E) 11.0 V}
The figure shows a plot for the electric field Ex as a function of x. Find the magnitude of the
\text{The figure shows a plot for the electric field $E_x$ as a function of $x$. Find the magnitude of the }
potential difference between the points x=2.00 m and x=6.00 m.
\text{potential difference between the points $x=2.00 \mathrm{~m}$ and $x=6.00 \mathrm{~m}$.}

ΔV=−∫ifE⋅ds
\Delta V=-\int_i^{f} \vec{E} \cdot d \vec{s}
ΔV=−
\Delta V=-
Area under curve
\text{Area under curve}
Be careful: the area under the curve can be positive or negative
\text{Be careful: the area under the curve can be positive or negative}
ΔV=−∫x1x2Ex⋅dx
\Delta V=-\int_{x_1}^{x_2} {E}_x \cdot d{x}
(Warning: The question is incomplete: they need to precise that Ey=Ez=0)
\text{(Warning: The question is incomplete: they need to precise that $E_y=E_z=0$)}
ΔV=−14.5V
\Delta V=-14.5 V
Answer A
\text{Answer A}
In the figure, particles with charges q1=+10μC and q2=−30μC are fixed in place with
\text{In the figure, particles with charges $q_1=+10 \mu \mathrm{C}$ and $q_2=-30 \mu \mathrm{C}$ are fixed in place with }
a separation of d=24 cm.
\text{a separation of $d=24 \mathrm{~cm}$. }
What is the value of Q that will make the potential equal zero at point P.
\text{What is the value of $Q$ that will make the potential equal zero at point $P$.}
⋅
\cdot
⋅
\cdot
⋅
\cdot
Q
Q
P
P
d
d
d
d
d
d
q1
q_1
q2
q_2
×
\times
A) 7.1μC
\text{A) $7.1 \hspace{1mm}\mu \mathrm{C}$}
B) 5.1μC
\text{B) $5.1 \hspace{1mm}\mu \mathrm{C}$}
C) 10μC
\text{C) $10 \hspace{1mm}\mu \mathrm{C}$}
D) 3.5μC
\text{D) $3.5 \hspace{1mm}\mu \mathrm{C}$}
E) 4.5μC
\text{E) $4.5 \hspace{1mm}\mu \mathrm{C}$}
In the figure, particles with charges q1=+10μC and q2=−30μC are fixed in place with
\text{In the figure, particles with charges $q_1=+10 \mu \mathrm{C}$ and $q_2=-30 \mu \mathrm{C}$ are fixed in place with }
a separation of d=24 cm.
\text{a separation of $d=24 \mathrm{~cm}$. }
What is the value of Q that will make the potential equal zero at point P.
\text{What is the value of $Q$ that will make the potential equal zero at point $P$.}
⋅
\cdot
⋅
\cdot
⋅
\cdot
Q
Q
P
P
d
d
d
d
d
d
q1
q_1
q2
q_2
×
\times
V=r1kq1+r2kq2+r3kQ=0
V=\frac{k q_1}{r_1}+\frac{k q_2}{r_2}+\frac{k Q}{r_3}=0
dkq1+2dkq2+2dkQ=0
\frac{k q_1}{d}+\frac{k q_2}{2d}+\frac{k Q}{\sqrt{2}d}=0
Q=2(−q1−2q2)=2(−10−(−30)/2)
Q=\sqrt{2}(-q_1-\frac{q_2}{2})=\sqrt{2}(-10-(-30)/2)
Q=2(5)=7.1μC
Q=\sqrt{2}(5)=7.1\mu C
2d
\sqrt{2}d
Answer A
\text{Answer A}
In a certain region of space, the electric potential is given by: V=−2.0xyz2, where V is in volts,
\text{In a certain region of space, the electric potential is given by: $V=-2.0 x y z^2$, where $\mathrm{V}$ is in volts,}
and x,y, and z are in meters. What is the magnitude of the electric field at the point with position
\text{and $x, y$, and $z$ are in meters.
What is the magnitude of the electric field at the point with position}
vector (2.0^−2.0^+4.0k^)?
\text{vector $(2.0 \hat{\imath}-2.0 \hat{\jmath}+4.0 \hat{k}) ?$}
A) 111 V/m
\text{A) $111 \mathrm{~V} / \mathrm{m}$}
B) 90.8 V/m
\text{B) $90.8 \mathrm{~V} / \mathrm{m}$}
C) 16.1 V/m
\text{C) $16.1 \mathrm{~V} / \mathrm{m}$}
D) 743 V/m
\text{D) $743 \mathrm{~V} / \mathrm{m}$}
E) 571 V/m
\text{E) $571 \mathrm{~V} / \mathrm{m}$}
In a certain region of space, the electric potential is given by: V=−2.0xyz2, where V is in volts,
\text{In a certain region of space, the electric potential is given by: $V=-2.0 x y z^2$, where $\mathrm{V}$ is in volts,}
and x,y, and z are in meters. What is the magnitude of the electric field at the point with position
\text{and $x, y$, and $z$ are in meters.
What is the magnitude of the electric field at the point with position}
vector (2.0^−2.0^+4.0k^)?
\text{vector $(2.0 \hat{\imath}-2.0 \hat{\jmath}+4.0 \hat{k}) ?$}
Ex=−∂x∂V=2yz2=2(−2)(4)2=−64 N/CEy=−∂y∂V=2xz2=+64 N/CEz=−∂z∂V=4xyz=4(2)(−2)(4)=−64 N/CE=Ex2+Ey2+Ez2=111 V
\begin{aligned}
& E_x=-\frac{\partial V}{\partial x}=2 y z^2=2(-2)(4)^2=-64 \mathrm{~N} / \mathrm{C} \\
& E_y=-\frac{\partial V}{\partial y}=2 x z^2=+64 \mathrm{~N} / \mathrm{C} \\
& E_z=-\frac{\partial V}{\partial z}=4 x y z=4(2)(-2)(4)=-64 \mathrm{~N} / \mathrm{C} \\
& E=\sqrt{E_x^2+E_y^2+E_z^2}=111 \mathrm{~V}
\end{aligned}
Answer A
\text{Answer A}
Four identical charged particles, each of charge q=30μC, are fixed at the corner of a square
\text{Four identical charged particles, each of charge $q=30 \mu \mathrm{C}$, are fixed at the corner of a square}
of length 10.0 cm. How much work is required, by an external agent, to move one of them to infinity?
\text{of length $10.0 \mathrm{~cm}$. How much work is required, by an external agent, to move one of them to infinity?}
A) -219 J
\text{A) -219 J}
B) +219 J
\text{B) +219 J}
C) -510 J
\text{C) -510 J}
E) -105 J
\text{E) -105 J}
D) +510 J
\text{D) +510 J}
Four identical charged particles, each of charge q=30μC, are fixed at the corner of a square
\text{Four identical charged particles, each of charge $q=30 \mu \mathrm{C}$, are fixed at the corner of a square}
of length 10.0 cm. How much work is required, by an external agent, to move one of them to infinity?
\text{of length $10.0 \mathrm{~cm}$. How much work is required, by an external agent, to move one of them to infinity?}
ΔK=−ΔU+Wapp
\Delta K=-\Delta U+W_{\text {app }}
0
0
Wapp =ΔU=qΔV=q(Vf−Vi)=−q(dkq+dkq+2dkq)
W_{\text {app }}=\Delta U=q\Delta V =q(V_f-V_i)=-q(\frac{kq}{d}+\frac{kq}{d}+\frac{kq}{\sqrt{2}d})
0
0
Wapp =−dkq2(2+21)=0.1(9×109)(30×10−6)2(2+21)
W_{\text {app }}=-\frac{kq^2}{d}(2+\frac{1}{\sqrt{2}})=\frac{\left(9 \times 10^9\right)\left(30 \times 10^{-6}\right)^2}{0.1}\left(2+\frac{1}{\sqrt{2}}\right)
Wapp =−219 J
W_{\text {app }}=-219 \mathrm{~J}
⋅
\cdot
⋅
\cdot
⋅
\cdot
⋅
\cdot
d
d
d
d
d
d
d
d
2d
\sqrt{2}d
Answer A
\text{Answer A}
Two metal spheres 1 and 2 with radii r1=1.0 cm and r2=2.0 cm carry charges q1=+22nC,
\text{Two metal spheres 1 and 2 with radii $r_1=1.0 \mathrm{~cm}$ and $r_2=2.0 \mathrm{~cm}$ carry charges $q_1=+22 \hspace{1mm}\mathrm{nC}$,}
and q2=−10nC, respectively. Initially both spheres are far apart. Then the spheres are connected
\text{and $q_2=-10\hspace{1mm} \mathrm{ nC}$, respectively. Initially both spheres are far apart. Then the spheres are connected}
by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?
\text{by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?}
A) +18nC
\text{A) $+18 \hspace{1mm}$nC}
B) −18nC
\text{B) $-18 \hspace{1mm}$nC}
C) +12nC
\text{C) $+12 \hspace{1mm}$nC}
D) −12nC
\text{D) $-12 \hspace{1mm}$nC}
E) +14nC
\text{E) $+14 \hspace{1mm}$nC}
Two metal spheres 1 and 2 with radii r1=1.0 cm and r2=2.0 cm carry charges q1=+22nC,
\text{Two metal spheres 1 and 2 with radii $r_1=1.0 \mathrm{~cm}$ and $r_2=2.0 \mathrm{~cm}$ carry charges $q_1=+22 \hspace{1mm}\mathrm{nC}$,}
and q2=−10nC, respectively. Initially both spheres are far apart. Then the spheres are connected
\text{and $q_2=-10\hspace{1mm} \mathrm{ nC}$, respectively. Initially both spheres are far apart. Then the spheres are connected}
by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?
\text{by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?}
22 nC
22 \text{ nC}
q1
q_1
q2
q_2
Connected
\text{Connected}
disonnected
\text{disonnected}
V1=V2
V_1=V_2
since the spheres are conducting (metalic)
\text{since the spheres are conducting (metalic)}
they will have the same electric potential
\text{they will have the same electric potential }
when connected
\text{when connected}
−10 nC
-10 \text{ nC}
Two metal spheres 1 and 2 with radii r1=1.0 cm and r2=2.0 cm carry charges q1=+22nC,
\text{Two metal spheres 1 and 2 with radii $r_1=1.0 \mathrm{~cm}$ and $r_2=2.0 \mathrm{~cm}$ carry charges $q_1=+22 \hspace{1mm}\mathrm{nC}$,}
and q2=−10nC, respectively. Initially both spheres are far apart. Then the spheres are connected
\text{and $q_2=-10\hspace{1mm} \mathrm{ nC}$, respectively. Initially both spheres are far apart. Then the spheres are connected}
by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?
\text{by a thin wire, how much charge is lost by sphere 1 when the electrostatic equilibrium is reached?}
22 nC
22 \text{ nC}
−10 nC
-10 \text{ nC}
q1
q_1
q2
q_2
Connected
\text{Connected}
disonnected
\text{disonnected}
V1=V2
V_1=V_2
since the spheres are conducting (metalic)
\text{since the spheres are conducting (metalic)}
they will have the same electric potential
\text{they will have the same electric potential }
when connected
\text{when connected}
R1kq1=R2kq2
\begin{aligned}
\frac{k q_1}{R_1} & =\frac{k q_2}{R_2}
\end{aligned}
V1=V2
V_1 =V_2
⇒
\Rightarrow
The total charge is conserved:
\text{The total charge is conserved:}
q1+q2=22−10=12 nC=q0
q_1+q_2=22-10=12 \text{ nC}=q_0
R1kq1=R2kq2
\begin{aligned}
\frac{k q_1}{R_1} & =\frac{k q_2}{R_2}
\end{aligned}
⇒
\Rightarrow
q2=q1R1R2
\begin{aligned}
{q_2} =q_1\frac{ R_2}{R_1}
\end{aligned}
⇒
\Rightarrow
q2=(q0−q2)R1R2
\begin{aligned}
{q_2} =(q_0-q_2)\frac{ R_2}{R_1}
\end{aligned}
⇒
\Rightarrow
q2=q01+R1R2R1R2=q0R1+R2R2=121+22=8 nC,
\begin{aligned}
{q_2} =q_0\frac{\frac{ R_2}{R_1}}{1+\frac{ R_2}{R_1}}=q_0\frac{ R_2}{R_1+ R_2}=12 \frac{2}{1+2}=8 \text{ nC},
\end{aligned}
q1=12−8=4 nC
q_1=12-8=4 \text{ nC}
The charge lost by sphere 1 is: qlost=22−4=18 nC
\text{The charge lost by sphere $1$ is: } q_\text{lost}=22-4=18 \text{ nC}
FIG. 10 gives the electric potential V as a function of distance x . Rank the four regions according
\text{FIG. 10 gives the electric potential V as a function of distance x . Rank the four regions according }
to the magnitude of the x -component of the electric field within them, GREATEST FIRST.
\text{to the magnitude of the x -component of the electric field within them, GREATEST FIRST.}
A) 2, 4, then 1 and 3 tie
\text{A) 2, 4, then 1 and 3 tie}
B) 4, 2, then 1 and 3 tie
\text{B) 4, 2, then 1 and 3 tie}
C) 4,2,3,1
\text{C) $4,2,3,1$}
D) 4,3,1,2
\text{D) $4,3,1,2$}
E) 2,1,3,4
\text{E) $2,1,3,4$}

FIG. 10 gives the electric potential V as a function of distance x . Rank the four regions according
\text{FIG. 10 gives the electric potential V as a function of distance x . Rank the four regions according }
to the magnitude of the x -component of the electric field within them, GREATEST FIRST.
\text{to the magnitude of the x -component of the electric field within them, GREATEST FIRST.}
A) 2, 4, then 1 and 3 tie
\text{A) 2, 4, then 1 and 3 tie}
B) 4, 2, then 1 and 3 tie
\text{B) 4, 2, then 1 and 3 tie}
C) 4,2,3,1
\text{C) $4,2,3,1$}
D) 4,3,1,2
\text{D) $4,3,1,2$}
E) 2,1,3,4
\text{E) $2,1,3,4$}

The electric field component Ex is the slope of V(x)
\text{The electric field component $E_x$ is the slope of $V(x)$}
Text
A)+260V
A) +260 V
B)−260V
B) -260 V
C)−195V
C) -195 V
D)+195V
D) +195 V
E)+325V
E) +325 V
A uniform electric field of magnitude 325 V/m is directed in the negative y direction. The
\text{A uniform electric field of magnitude $325 \mathrm{~V} / \mathrm{m}$ is directed in the negative $y$ direction. The }
coordinates of point A are (−0.200,−0.300)m, and those of point B are (0.400,0.500)m.
\text{coordinates of point A are $(-0.200,-0.300) \mathrm{m}$, and those of point B are $(0.400,0.500) \mathrm{m}$.}
Calculate the electric potential difference VB−VA.
\text{Calculate the electric potential difference $V_B-V_A$.}
ΔVVB=−E⋅Δr−VA=−E⋅(rB−rA)=325^⋅(0.6^+0.8^)=+260 V
\begin{aligned}
\Delta \mathrm{V} & =-\overrightarrow{\mathrm{E}} \cdot \Delta \overrightarrow{\mathrm{r}} \\
V_B & -V_A=-\overrightarrow{\mathrm{E}} \cdot\left(\overrightarrow{\mathrm{r}}_{\mathrm{B}}-\overrightarrow{\mathrm{r}}_{\mathrm{A}}\right) \\
& =325 \hat{\jmath} \cdot(0.6 \hat{\imath}+0.8 \hat{\jmath})=+260 \mathrm{~V}
\end{aligned}
Text
A)+260V
A) +260 V
B)−260V
B) -260 V
C)−195V
C) -195 V
D)+195V
D) +195 V
E)+325V
E) +325 V
A uniform electric field of magnitude 325 V/m is directed in the negative y direction. The
\text{A uniform electric field of magnitude $325 \mathrm{~V} / \mathrm{m}$ is directed in the negative $y$ direction. The }
coordinates of point A are (−0.200,−0.300)m, and those of point B are (0.400,0.500)m.
\text{coordinates of point A are $(-0.200,-0.300) \mathrm{m}$, and those of point B are $(0.400,0.500) \mathrm{m}$.}
Calculate the electric potential difference VB−VA.
\text{Calculate the electric potential difference $V_B-V_A$.}
Proof:
\text{Proof:}
ΔV=−∫E⋅ds
\Delta V=-\int \vec{E}\cdot \vec{ds}
ΔV=−∫E⋅ds=−E⋅∫ds
\Delta V=-\int \vec{E}\cdot \vec{ds}=- \vec{E}\cdot \int\vec{ds}
=−E⋅Δr
=- \vec{E}\cdot \Delta \vec{r}
C h a p t e r 2 4 \text{Chapter 24} C h a p t e r 2 4 \text{Chapter 24} C h a p t e r 2 4 \text{Chapter 24} C h a p t e r 2 4 \text{Chapter 24}
Chapter 24
By smstry
Chapter 24
- 213



