Chapter 8
\text{Chapter 8}
Chapter 8
\text{Chapter 8}
Chapter 8
\text{Chapter 8}
Chapter 8
\text{Chapter 8}
Dr. Adel Abbout
\textit{Dr. Adel Abbout}
A 2.0 kg block is thrown upward from the ground. At what height above the ground will
\text{A $2.0 \mathrm{~kg}$ block is thrown upward from the ground. At what height above the ground will }
the gravitational potential energy of the Earth-block system have increased by 490 J ?
\text{the gravitational potential energy of the Earth-block system have increased by $490 \mathrm{~J}$ ?}
A) 12 mB) 50 mC) 25 mD) 8.0 mE) 18 m
\text{A) $12 \mathrm{~m}$}\\
\text{B) $50 \mathrm{~m}$}\\
\text{C) $25 \mathrm{~m}$}\\
\text{D) $8.0 \mathrm{~m}$}\\
\text{E) $18 \mathrm{~m}$}
A 2.0 kg block is thrown upward from the ground. At what height above the ground will
\text{A $2.0 \mathrm{~kg}$ block is thrown upward from the ground. At what height above the ground will }
the gravitational potential energy of the Earth-block system have increased by 490 J ?
\text{the gravitational potential energy of the Earth-block system have increased by $490 \mathrm{~J}$ ?}
A) 12 mB) 50 mC) 25 mD) 8.0 mE) 18 m
\text{A) $12 \mathrm{~m}$}\\
\text{B) $50 \mathrm{~m}$}\\
\text{C) $25 \mathrm{~m}$}\\
\text{D) $8.0 \mathrm{~m}$}\\
\text{E) $18 \mathrm{~m}$}
ΔU=mgy
\Delta U=mgy
where y is measure from the ground as reference.
\text{where }y \text{ is measure from the ground as reference.}
y=mgΔU=2.0×9.8490≈25 m
\displaystyle y=\frac{\Delta U}{mg}=\frac{490}{2.0\times 9.8}\approx 25\text{ m}
Answer C)
\text{Answer C)}
An ideal spring with a 20 N/m spring constant is compressed by a 10 N force. The potential
\text{An ideal spring with a $20 \mathrm{~N} / \mathrm{m}$ spring constant is compressed by a $10 \mathrm{~N}$ force. The potential }
A) 0.50 JB) 2.5 JC) 5.0 JD) 10 JE) 200 J
\text{A) $0.50 \mathrm{~J}$}\\
\text{B) $2.5 \mathrm{~J}$}\\
\text{C) $5.0 \mathrm{~J}$}\\
\text{D) $10 \mathrm{~J}$}\\
\text{E) $200 \mathrm{~J}$}
energy stored in the spring is:
\text{energy stored in the spring is: }
An ideal spring with a 20 N/m spring constant is compressed by a 10 N force. The potential
\text{An ideal spring with a $20 \mathrm{~N} / \mathrm{m}$ spring constant is compressed by a $10 \mathrm{~N}$ force. The potential }
A) 0.50 JB) 2.5 JC) 5.0 JD) 10 JE) 200 J
\text{A) $0.50 \mathrm{~J}$}\\
\text{B) $2.5 \mathrm{~J}$}\\
\text{C) $5.0 \mathrm{~J}$}\\
\text{D) $10 \mathrm{~J}$}\\
\text{E) $200 \mathrm{~J}$}
Answer B)
\text{Answer B)}
energy stored in the spring is:
\text{energy stored in the spring is: }
U=21kx2
\displaystyle U=\frac{1}{2}k x_\text{}^2
The elastic potential energy is:
\text{The elastic potential energy is:}
(When the spring is relaxed, its energy is 0)
\text{(When the spring is relaxed, its energy is 0)}
F=kx
\displaystyle F=k x
(magnitude)
\text{(magnitude)}
U=21kF2=2×20102=2.5 J
\displaystyle U=\frac{1}{2}\frac{F^2}{k} =\frac{10^2}{2\times20}=2.5 \text{ J}
A 2.0 kg object is connected to one end of an unstretched spring which is attached to the ceiling
\text{A $2.0 \mathrm{~kg}$ object is connected to one end of an unstretched spring which is attached to the ceiling }
A) 0.80 mB) 0.10 mC) 0.40 mD) 0.20 mE) 0.50 m
\text{A) $0.80 \mathrm{~m}$}\\
\text{B) $0.10 \mathrm{~m}$}\\
\text{C) $0.40 \mathrm{~m}$}\\
\text{D) $0.20 \mathrm{~m}$}\\
\text{E) $0.50 \mathrm{~m}$}
by the other end and then the object is allowed to drop. The spring constant of the spring
\text{by the other end and then the object is allowed to drop. The spring constant of the spring }
is 196 N/m. How far does it drop before coming to rest momentarily?
\text{is $196 \mathrm{~N} / \mathrm{m}$. How far does it drop before coming to rest momentarily?}

m
m
m
m
reference
\text{reference}
k
k
(not compressed)
\text{(not compressed)}
A 2.0 kg object is connected to one end of an unstretched spring which is attached to the ceiling
\text{A $2.0 \mathrm{~kg}$ object is connected to one end of an unstretched spring which is attached to the ceiling }
by the other end and then the object is allowed to drop. The spring constant of the spring
\text{by the other end and then the object is allowed to drop. The spring constant of the spring }
is 196 N/m. How far does it drop before coming to rest momentarily?
\text{is $196 \mathrm{~N} / \mathrm{m}$. How far does it drop before coming to rest momentarily?}
We take the same reference for the potential energy and the elastic one.
\text{We take the same reference for the potential energy and the elastic one.}
To avoid problems, take it at the position where the spring is not stretched.
\text{To avoid problems, take it at the position }\\
\text{where the spring is not stretched.}
The mechanical energy is conserved:
\text{The mechanical energy is conserved:}
Ki+Ui=Kf+Uf
K_\text{i}+U_\text{i}=K_\text{f}+U_\text{f}
21mvi2+mgy0+21kxi2=21mvf2+mgy+21kxf2
\displaystyle \frac{1}{2}mv_\text{i}^2+mgy_0+\frac{1}{2} k x_\text{i}^2=\frac{1}{2}mv_\text{f}^2+mgy+\frac{1}{2} k x_\text{f}^2
xi=y0=0
x_\text{i}=y_0=0
vi=vf=0
v_\text{i}=v_\text{f}=0
xf=y
x_\text{f}=y
(reference)
(\text{reference})
(at rest)
(\text{at rest})
(same distance)
\text{(same distance)}
0=mgy+21ky2
\displaystyle 0=mgy+\frac{1}{2} k y^2
0=mgy+21ky2
\displaystyle 0=mgy+\frac{1}{2} k y^2
y=−k2mg=−1962×2×9.8
\displaystyle y=-\frac{2mg}{k}=-\frac{2\times2\times9.8}{196}
⇒
\Rightarrow
=−0.20 m
=-0.20\text{ m}

m
m
m
m
reference
\text{reference}
k
k
y
y
Answer D)
\text{Answer D)}
(not compressed)
\text{(not compressed)}
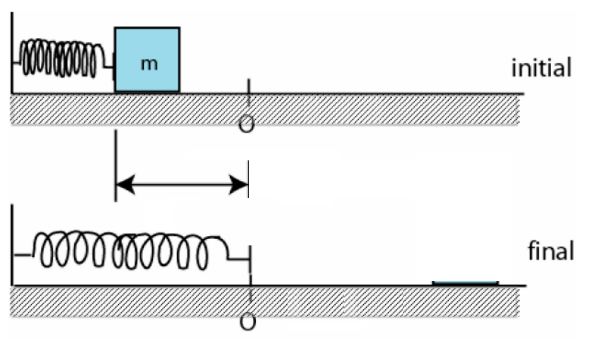
An ideal spring (compressed by 7.00 cm and initially at rest,) fires a 15.0 g block horizontally
\text{An ideal spring (compressed by $7.00 \mathrm{~cm}$ and initially at rest,) fires a 15.0 $\mathrm{g}$ block horizontally }
across a frictionless table top. The spring has a spring constant of 20.0 N/m.
\text{across a frictionless table top. The spring has a spring constant of $20.0 \mathrm{~N} / \mathrm{m}$. }
The speed of the block as it leaves the spring is:
\text{The speed of the block as it leaves the spring is: }
A) 2.56 m/sB) 1.90 m/sC) 3.64 m/sD) 8.12 m/sE) 5.25 m/s
\text{A) $2.56 \mathrm{~m} / \mathrm{s}$}\\
\text{B) $1.90 \mathrm{~m} / \mathrm{s}$}\\
\text{C) $3.64 \mathrm{~m} / \mathrm{s}$}\\
\text{D) $8.12 \mathrm{~m} / \mathrm{s}$}\\
\text{E) $5.25 \mathrm{~m} / \mathrm{s}$}
An ideal spring (compressed by 7.00 cm and initially at rest,) fires a 15.0 g block horizontally
\text{An ideal spring (compressed by $7.00 \mathrm{~cm}$ and initially at rest,) fires a 15.0 $\mathrm{g}$ block horizontally }
across a frictionless table top. The spring has a spring constant of 20.0 N/m.
\text{across a frictionless table top. The spring has a spring constant of $20.0 \mathrm{~N} / \mathrm{m}$. }
The speed of the block as it leaves the spring is:
\text{The speed of the block as it leaves the spring is: }
m
m
m
m
7.00 cm
7.00 \text{ cm}
The external forces T and FN are not working
\text{The external forces }\vec{T}\text{ and }\vec{F}_N \text{ are not working}
The mechanical energy of (spring+mass) is conserved
\text{The mechanical energy of (spring+mass) is \textcolor{red}{conserved}}
Ki+Ui=Kf+Uf
K_\text{i}+U_\text{i}=K_\text{f}+U_\text{f}
21mvi2+21kxi2=21mvf2+21kxf2
\displaystyle \frac{1}{2}mv_\text{i}^2+\frac{1}{2} k x_\text{i}^2=\frac{1}{2}mv_\text{f}^2+\frac{1}{2} k x_\text{f}^2
vi=0
v_\text{i}=0
xf=0
x_\text{f}=0
(at rest)
(\text{at rest})
(not stretched)
\text{(not stretched)}
We have
\text{We have }
and
\text{ and }
21kxi2=21mvf2
\displaystyle \frac{1}{2} k x_\text{i}^2=\frac{1}{2}mv_\text{f}^2
v=ximk
\displaystyle v=x_\text{i}\sqrt{\frac{k}{m}}
v=0.07×15×10−320≈2.56 m/s
\displaystyle v=0.07\times\sqrt{\frac{20}{15 \times 10^{-3}}}\approx2.56\text{ m/s}
Answer A)
\text{Answer A)}
(kinetic and elastic energies)
\text{(kinetic and elastic energies) }
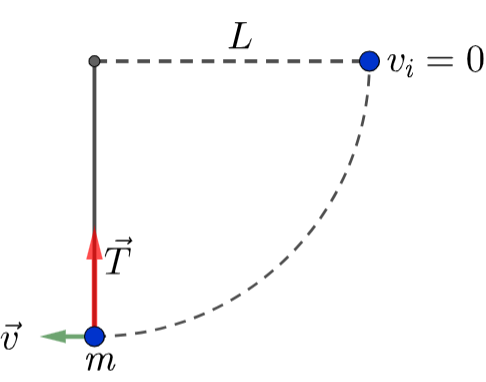
An object of mass m, attached to a light cord of length L, is held horizontally from a fixed
\text{An object of mass $m$, attached to a light cord of length $L$, is held horizontally from a fixed}
support as shown in Fig 1 . The object is then released from rest.
\text{ support as shown in Fig 1 . The object is then released from rest.}
What is the tension force in the cord when the object is at the lowest point of its swing?
\text{ What is the tension force in the cord when the object is at the lowest point of its swing?}
A) 2mgB) mgC) 3mgD) mg/2E) mgL
\text{A) $2 \mathrm{ mg}$}\\
\hspace{-2mm}\text{B) $\mathrm{ mg}$}\\
\text{C) $3 \mathrm{ mg}$}\\
\hspace{2mm}\text{D) $\mathrm{ mg} / 2$}\\
\text{E) $\mathrm{ mgL}$}
An object of mass m, attached to a light cord of length L, is held horizontally from a fixed
\text{An object of mass $m$, attached to a light cord of length $L$, is held horizontally from a fixed}
support as shown in Fig 1 . The object is then released from rest.
\text{ support as shown in Fig 1 . The object is then released from rest.}
What is the tension force in the cord when the object is at the lowest point of its swing?
\text{ What is the tension force in the cord when the object is at the lowest point of its swing?}

ΔEmec=W(T)
\Delta E_{mec}=W(\vec{T})
since T is continously perpendicular to the path, its work is therefore 0
\text{since }\vec{T} \text{ is continously perpendicular to the path,}\\ \text{ its work is therefore }0
The mechanical energy is therefore conserved
\text{The mechanical energy is therefore \textcolor{red}{conserved}}
Ki+Ui=Kf+Uf
K_\text{i}+U_\text{i}=K_\text{f}+U_\text{f}
21mvi2+mgy=21mvf2
\displaystyle \frac{1}{2}mv_\text{i}^2+mgy=\frac{1}{2}mv_\text{f}^2
The reference for the potential energy is taken at the lowes point
\text{The reference for the potential energy is taken at the lowes point}
y=L
y=L
vi=0
v_\text{i}=0
and
\text{ and }
⇒
\Rightarrow
vf=2gL
\displaystyle v_\text{f}=\sqrt{2gL}
(system is earth+mass)
\text{(system is earth+mass)}
at the lowest point, we have
\text{at the lowest point, we have}
mg+T=ma
m\vec{g}+\vec{T}=m\vec{a}
where the acceleration is centripetal. By projecting on the radial axis, we get:
\text{where the acceleration is centripetal. By projecting on the radial axis, we get:}
−mg+T=ma=mRv2
\displaystyle -m{g}+{T}=m{a}=m\frac{v^2}{R}
⇒
\Rightarrow
T=mg+mRv2=mg+2mgL/L=3mg
\displaystyle {T}=mg+m\frac{v^2}{R}=mg+2mgL/L=3mg
Answer C)
\text{Answer C)}
T=3mg
\displaystyle {T}=3mg
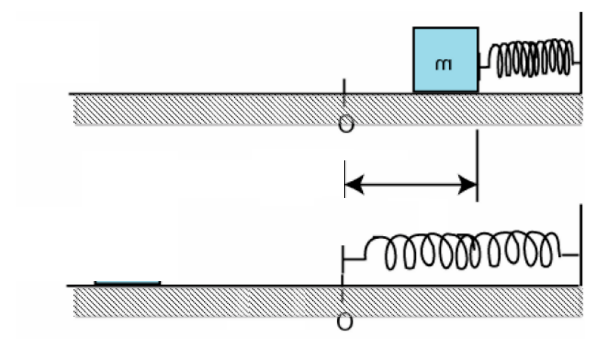
A block of mass 2.0 kg is initially moving to the right on a horizontal frictionless surface at
\text{A block of mass $2.0 \mathrm{~kg}$ is initially moving to the right on a horizontal frictionless surface at }
a speed 5.0 m/s. It then compresses a spring of spring constant 100 N/m.
\text{ a speed $5.0 \mathrm{~m} / \mathrm{s}$. It then compresses a spring of spring constant $100 \mathrm{~N} / \mathrm{m}$.}
At the instant when the kinetic energy of the block is equal to the potential energy of the spring,
\text{ At the instant when the kinetic energy of the block is equal to the potential energy of the spring,}
the spring is compressed a distance of:
\text{ the spring is compressed a distance of:}
A) 0.25 mB) 0.50 mC) 1.0 mD) 0.75 mE) 0.10 m
\text{A) $0.25 \mathrm{~m}$}\\
\text{B) $0.50 \mathrm{~m}$}\\
\hspace{-2mm}\text{C) $1.0 \mathrm{~m}$}\\
\text{D) $0.75 \mathrm{~m}$}\\
\text{E) $0.10 \mathrm{~m}$}
A block of mass 2.0 kg is initially moving to the right on a horizontal frictionless surface at
\text{A block of mass $2.0 \mathrm{~kg}$ is initially moving to the right on a horizontal frictionless surface at }
a speed 5.0 m/s. It then compresses a spring of spring constant 100 N/m.
\text{ a speed $5.0 \mathrm{~m} / \mathrm{s}$. It then compresses a spring of spring constant $100 \mathrm{~N} / \mathrm{m}$.}
At the instant when the kinetic energy of the block is equal to the potential energy of the spring,
\text{ At the instant when the kinetic energy of the block is equal to the potential energy of the spring,}
the spring is compressed a distance of:
\text{ the spring is compressed a distance of:}
m
{m}
m
{m}
?
?
k
k
initial
\text{initial}
final
\text{final}
The mechanical energy of the system mass+spring is conserved.
\text{The mechanical energy of the system mass+spring is \textcolor{red}{conserved.}}
Ki+Ui=Kf+Uf
K_\text{i}+U_\text{i}=K_\text{f}+U_\text{f}
the initial elastic potential energy is Ui=0
\text{the initial elastic potential energy is }U_\text{i}=0
Kf=Uf⇒Kf+Uf=2Uf
K_\text{f}=U_\text{f}\Rightarrow K_\text{f}+U_\text{f}=2U_\text{f}
1
1
…
\dots
1
1
Ki=2Uf
K_\text{i}=2U_\text{f}
⇒
\Rightarrow
21mvi2=kxf2
\displaystyle \frac{1}{2}mv_\text{i}^2= k x_\text{f}^2
xf=2kmvi
\displaystyle x_\text{f}=\sqrt{\frac{m}{2k}}v_\text{i}
xf=2×1002.0×5.0=0.50 m
\displaystyle x_\text{f}=\sqrt{\frac{2.0}{2\times100}}\times 5.0=0.50 \text{ m}
xf=0.50 m
\displaystyle x_\text{f}=0.50 \text{ m}
Answer B)
\text{Answer B)}
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
A 3.00 kg block is dropped from a height of 40 cm onto a spring of spring constant k (see Fig 2).
\text{A $3.00 \mathrm{~kg}$ block is dropped from a height of $40 \mathrm{~cm}$ onto a spring of spring constant $\mathrm{k}$ (see Fig 2).}
If the maximum distance the spring is compressed =0.130 m, find k.
\text{ If the maximum distance the spring is compressed $=0.130 \mathrm{~m}$, find $\mathrm{k}$.}

m
m
A) 1840 N/mB) 980 N/mC) 490 N/mD) 1250 N/mE) 2800 N/m
\text{A) $1840 \mathrm{~N} / \mathrm{m}$}\\
\hspace{-2mm}\text{B) $980 \mathrm{~N} / \mathrm{m}$}\\
\hspace{-2mm}\text{C) $490 \mathrm{~N} / \mathrm{m}$}\\
\text{D) $1250 \mathrm{~N} / \mathrm{m}$}\\
\text{E) $2800 \mathrm{~N} / \mathrm{m}$}
A 3.00 kg block is dropped from a height of 40 cm onto a spring of spring constant k (see Fig 2).
\text{A $3.00 \mathrm{~kg}$ block is dropped from a height of $40 \mathrm{~cm}$ onto a spring of spring constant $\mathrm{k}$ (see Fig 2).}
If the maximum distance the spring is compressed =0.130 m, find k.
\text{ If the maximum distance the spring is compressed $=0.130 \mathrm{~m}$, find $\mathrm{k}$.}
system=earth+mass+spring
\text{system=earth+mass+spring}
mechanical energy is conserved
\text{mechanical energy is \textcolor{red}{conserved}}
Ki+Uip+Uie=Kf+Ufp+Ufe
K_\text{i}+U_\text{i}^p+U_\text{i}^e=K_\text{f}+U_\text{f}^p+U_\text{f}^e
21mvi2+mgy0+21kxi2=21mvf2+mgy+21kxf2
\displaystyle \frac{1}{2}mv_\text{i}^2+mgy_0+\frac{1}{2} k x_\text{i}^2=\frac{1}{2}mv_\text{f}^2+mgy+\frac{1}{2} k x_\text{f}^2
=
=
0
0
=
=
0
0
=
=
0
0
mg(y0−y)=21kxf2
\displaystyle mg(y_0-y)=\frac{1}{2} k x_\text{f}^2
(the final speed is 0)
(\text{the final speed is }0)
y0−y is the total distance: y0−y=d+xf
y_0-y \text{ is the total distance: }y_0-y=d+x_\text{f}
k=xf22mg(d+xf)
\displaystyle k=\frac{2mg(d+x_\text{f})}{x_\text{f}^2}
k=0.13022×3.00×9.8×(0.40+0.130)≈1844 N/m
\displaystyle k=\frac{2\times 3.00\times 9.8\times(0.40+0.130)}{0.130^2}\approx1844 \text{ N/m}
Ansewr A)
\text{Ansewr A)}

m
m

m
m
m
m
x
x
Figure 2
\text{Figure 2}
d
d
A projectile of mass 0.20 kg is fired with an initial speed of 20 m/s at an angle of 60 degrees
\text{A projectile of mass $0.20 \mathrm{~kg}$ is fired with an initial speed of $20 \mathrm{~m} / \mathrm{s}$ at an angle of 60 degrees}
above the horizontal. The kinetic energy of the projectile at its highest point is:
\text{ above the horizontal. The kinetic energy of the projectile at its highest point is:}
A) 0 JB) 40 JC) 30 JD) 5.0 JE) 10 J
\hspace{-1mm}\text{A) $0 \mathrm{~J}$}\\
\text{B) $40 \mathrm{~J}$}\\
\text{C) $30 \mathrm{~J}$}\\
\hspace{1mm}\text{D) $5.0 \mathrm{~J}$}\\
\text{E) $10 \mathrm{~J}$}
A projectile of mass 0.20 kg is fired with an initial speed of 20 m/s at an angle of 60 degrees
\text{A projectile of mass $0.20 \mathrm{~kg}$ is fired with an initial speed of $20 \mathrm{~m} / \mathrm{s}$ at an angle of 60 degrees}
above the horizontal. The kinetic energy of the projectile at its highest point is:
\text{ above the horizontal. The kinetic energy of the projectile at its highest point is:}
Conservation of the mechanical energy:
\text{Conservation of the mechanical energy:}
Ki+Ui=Kf+Uf
K_\text{i}+U_\text{i}=K_\text{f}+U_\text{f}
21mvi2+mgy0=Kf+mgy
\displaystyle \frac{1}{2}mv_\text{i}^2+mgy_0=K_\text{f}+mgy
=
=
0
0
The maximum height H=2gvi2sin(θ)2
\text{The maximum height }\displaystyle H=\frac{v_i^2 \sin(\theta)^2}{2g}
(Formula sheet)
(\text{Formula sheet})
21mvi2−mgH=Kf
\displaystyle \frac{1}{2}mv_\text{i}^2-mgH=K_\text{f}
21mvi2(1−sin2θ)=Kf
\displaystyle \frac{1}{2}mv_\text{i}^2 (1-\sin^2\theta)=K_\text{f}
Kf=21mvi2cos2θ
\displaystyle K_\text{f}=\frac{1}{2}mv_\text{i}^2 \cos^2\theta
Kf=210.20×202×221=10 J
\displaystyle K_\text{f}=\frac{1}{2} 0.20\times20^2\times \frac{1}{2^2}=10 \text{ J}
Answer E)
\text{Answer E)}
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
⇒
\Rightarrow
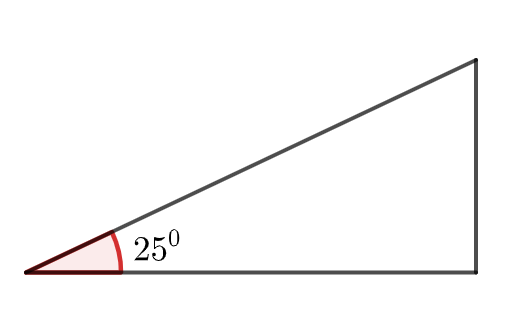
A 2.2 kg block starts from rest on a rough inclined plane that makes an angle of 25∘ with the
\text{A $2.2 \mathrm{~kg}$ block starts from rest on a rough inclined plane that makes an angle of $25^{\circ}$ with the}
horizontal. The coefficient of kinetic friction is 0.25 . As the block slides 2.0 m down the plane,
\text{horizontal. The coefficient of kinetic friction is 0.25 . As the block slides $2.0 \mathrm{~m}$ down the plane, }
the mechanical energy of the Earth-block system changes by:
\text{the mechanical energy of the Earth-block system changes by:}
A) −11 JB) +0 JC) +9.8 JD) −18 JE) −9.8 J
\text{A) $-11 \mathrm{~J}$}\\
\hspace{-2mm}\text{B) $+0 \mathrm{~J}$}\\
\hspace{0mm}\text{C) $+9.8 \mathrm{~J}$}\\
\text{D) $-18 \mathrm{~J}$}\\
\hspace{1mm}\text{E) $-9.8 \mathrm{~J}$}
A 2.2 kg block starts from rest on a rough inclined plane that makes an angle of 25∘ with the
\text{A $2.2 \mathrm{~kg}$ block starts from rest on a rough inclined plane that makes an angle of $25^{\circ}$ with the}
horizontal. The coefficient of kinetic friction is 0.25 . As the block slides 2.0 m down the plane,
\text{horizontal. The coefficient of kinetic friction is 0.25 . As the block slides $2.0 \mathrm{~m}$ down the plane, }
the mechanical energy of the Earth-block system changes by:
\text{the mechanical energy of the Earth-block system changes by:}
ΔEmec+ΔEth=0
\Delta E_{\mathrm{mec}}+\Delta E_{\mathrm{th}}=0
ΔEint=0
\Delta E_{\mathrm{int}}=0
W(FN)=0
W(\vec{F}_N)=0
The external force is FN, so
\text{The external force is }\vec{F}_N, \text{ so}
ΔEth=fd=μkNd=μkmgdcosθ
\Delta E_{\mathrm{th}}=f d=\mu_k N d=\mu_kmgd \cos\theta
FN
\vec{F}_N
mg
m\vec{g}
f
\vec{f}
ΔEmec=−ΔEth=−μkmgdcosθ
\Delta E_{\mathrm{mec}}=-\Delta E_{\mathrm{th}}=-\mu_kmgd \cos\theta
=−0.25×2.2×9.8×2×cos(250)
=-0.25\times 2.2\times9.8\times2\times\cos(25^0)
=−0.25×2.2×9.8×2×cos(250)
=-0.25\times 2.2\times9.8\times2\times\cos(25^0)
=−9.8 J
=-9.8 \text{ J}
Answer E)
\text{Answer E)}
A 6.0 kg box starts up a 30 degrees incline with 158 J of kinetic energy. How far will it slide up
\text{A $6.0 \mathrm{~kg}$ box starts up a 30 degrees incline with $158 \mathrm{~J}$ of kinetic energy. How far will it slide up }
the incline if the coefficient of kinetic friction between box and incline is 0.40 ?
\text{the incline if the coefficient of kinetic friction between box and incline is 0.40 ?}
A) 5.2 mB) 2.2 mC) 1.2 mD) 4.2 mE) 3.2 m
\text{A) $5.2 \mathrm{~m}$}\\
\text{B) $2.2 \mathrm{~m}$}\\
\text{C) $1.2 \mathrm{~m}$}\\
\text{D) $4.2 \mathrm{~m}$}\\
\text{E) $3.2 \mathrm{~m}$}
A 6.0 kg box starts up a 30 degrees incline with 158 J of kinetic energy. How far will it slide up
\text{A $6.0 \mathrm{~kg}$ box starts up a 30 degrees incline with $158 \mathrm{~J}$ of kinetic energy. How far will it slide up }
the incline if the coefficient of kinetic friction between box and incline is 0.40 ?
\text{the incline if the coefficient of kinetic friction between box and incline is 0.40 ?}
ΔEmec+ΔEth=0
\Delta E_{\mathrm{mec}}+\Delta E_{\mathrm{th}}=0
ΔK+ΔU+ΔEth=0
\Delta K+\Delta U +\Delta E_{\mathrm{th}}=0
ΔEth=fd=μkNd=μkmgdcosθ
\Delta E_{\mathrm{th}}=f d=\mu_k N d=\mu_kmgd \cos\theta

FN
\vec{F}_N
mg
m\vec{g}
f
\vec{f}
v
\vec{v}
ΔK=Kf−Ki=0−Ki=−158 J
\Delta K=K_\text{f}-K_\text{i}=0-K_\text{i}=-158 \text{ J}
ΔU=mg(y−y0)=mgdsinθ
\Delta U =mg(y-y_0)=mgd \sin\theta
ΔK+ΔU+ΔEth=0
\Delta K+\Delta U +\Delta E_{\mathrm{th}}=0
⇒
\Rightarrow
ΔK+mgh+μkmgdcosθ=0
\Delta K+mg h+\mu_kmgd \cos\theta=0
d=−mg(sinθ+μkcosθ)ΔK=6.0×9.8×(sin300+0.40cos300)158≈3.2 m
\displaystyle d=-\frac{\Delta K}{mg(\sin\theta+\mu_k \cos\theta)}=\frac{158}{6.0\times 9.8\times(\sin30^0+0.40\cos30^0)}\approx3.2 \text{ m}
⇒
\Rightarrow
ΔK+mgdsinθ+μkmgdcosθ=0
\Delta K+mg d \sin\theta+\mu_kmgd \cos\theta=0
Conservation of energy
\text{Conservation of energy}
Answer E)
\text{Answer E)}
I=Ihoop +Irod Ihoop =21mR2
\begin{aligned}
I=I_{\text {hoop }}+ & I_{\text {rod }} \\
& I_{\text {hoop }}=\frac{1}{2} \mathrm{mR}^2
\end{aligned}
C h a p t e r 8 \text{Chapter 8} C h a p t e r 8 \text{Chapter 8} C h a p t e r 8 \text{Chapter 8} C h a p t e r 8 \text{Chapter 8} D r . A d e l A b b o u t \textit{Dr. Adel Abbout}
Chapter 8-Phys101
By smstry
Chapter 8-Phys101
- 175



