\text{Chapter 6}
\text{Chapter 6}
\text{Chapter 6}
\text{Chapter 6}
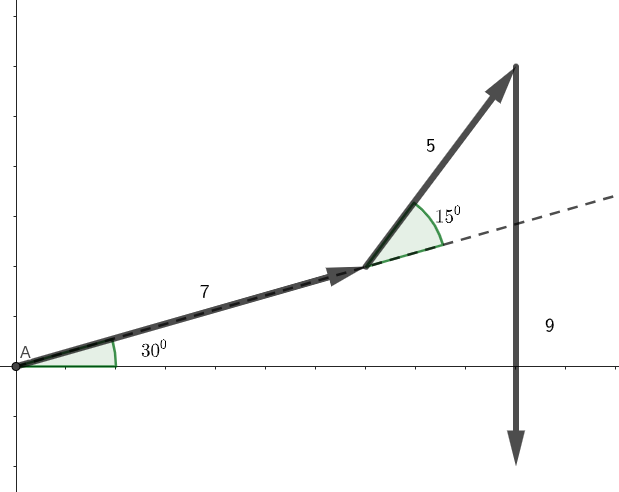
\text{A man walks 7 km in the direction $15^0$ north of east, and then deviates $15^0$ north of his direction}
\text{ and walks for 5 km, then goes due south for 9 km}
\text{What is the distance of its destiniation from the origin?}

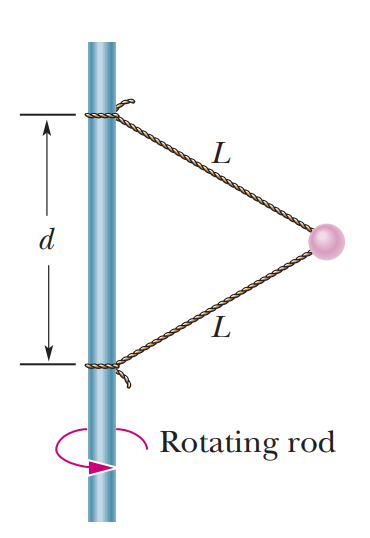


\vec{a}
\vec{T}_1
\vec{T}_2


\displaystyle \vec{T}_1+\vec{T}_2+m\vec{g}=m\vec{a}
\vec{a}
T_1\sin\theta-T_2\sin\theta-mg=0
\displaystyle \text{from the triangle, we have} \sin\theta=\frac{d/2}{L}=0.85/1.70=\frac{1}{2}
\text{The angle is therefore }\theta=30^0
\displaystyle \cos\theta=\frac{\sqrt{3}}{2}
\text{we project on y-axis}
\vec{T}_1
\vec{T}_2
\displaystyle T_1\frac{1}{2}-T_2\frac{1}{2}=mg
\displaystyle T_1-T_2=2mg
\displaystyle T_2=T_1-2mg
\displaystyle T_2=35-2\times1.34\times 9.8=8.7N
\Rightarrow
\Rightarrow
\Rightarrow

\vec{a}
T_1\sin\theta-T_2\sin\theta-mg=0
\displaystyle \cos\theta=\frac{\sqrt{3}}{2}
\displaystyle \sin\theta=\frac{{1}}{2}
\displaystyle {T}_1\frac{\sqrt{3}}{2}+{T}_2\frac{\sqrt{3}}{2}=m{a}
\vec{T}_2
\vec{T}_1
\text{I take my x-axis towords the center of rotation,} \\ \text{ and then project the equation}
\displaystyle {T}_1\cos\theta+{T}_2\cos\theta=m{a}
\displaystyle m{a}=\frac{\sqrt{3}}{2}(35+8.7)=37.8 N
\sum \vec{F}=m\vec{a}
{F}_\text{net}=m{a}
\displaystyle a=\frac{F_\text{net}}{m}
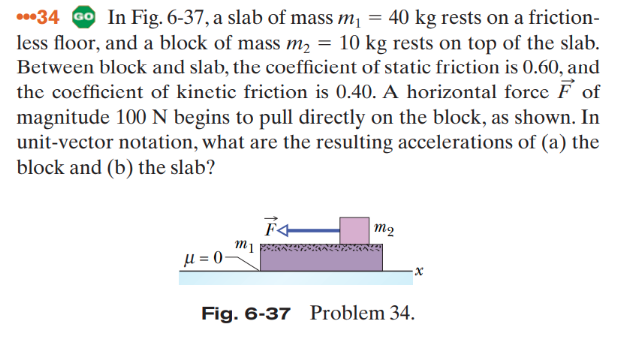
\overrightarrow{f}

\overrightarrow{N}
m_2\vec{g}
m_1\vec{g}
\overrightarrow{f}
\overrightarrow{N'}
m_2\vec{g}
\overrightarrow{F}
\overrightarrow{f}
\text{free body diagram on }m_1
\text{free body diagram on }m_2
\overrightarrow{N}
m_2\vec{g}
m_1\vec{g}
\overrightarrow{f}
\overrightarrow{N'}
m_2\vec{g}
\overrightarrow{F}
\overrightarrow{f}
\text{free body diagram on }m_1
\text{free body diagram on }m_2
\text{Mass }m_2:
m_2g=N'
f_\text{max}=\mu_sN'=\mu_s m_2 g=0.60\times 10\times 9.8=58.8N
F>f_\text{max}\Rightarrow m_2 \text{ will move}\Rightarrow\text{We use }\mu_k
\text{the projection on the x-axis gives}
\vec{F}+\vec{f}+\vec{N'}+m_2\vec{g}=m_2\vec{a}
x
F-f=m_2a\Rightarrow F-\mu_km_2g=m_2a\Rightarrow a=F/m_2-\mu_kg=100/10-0.4\times 9.8\approx6.1\text{ $m/s^2$}
\text{Mass }m_1:
\text{(Be careful: }f \text{ is not related to }N \text{ because this } N \text{ is from the surface below)}
\vec{f}+\vec{N}+m_2\vec{g}+m_1\vec{g}=m_1\vec{a}
\text{the projection on the x-axis gives}
{f}=m_1{a}\Rightarrow
\mu_k m_2 g=m_1{a}\Rightarrow a=\mu_k g m_2/m_1 =0.40\times 10/40 \times 9.8\approx1 \text{$m/s^2$}


\text{A loaded penguin sled weighing $80 \mathrm{~N}$ rests on a plane inclined at an angle of $20^{\circ}$ to the horizontal.}
\text{Between the sled and the plane, the coefficient of static friction is 0.25 , and the coefficient of }
\text{kinetic friction is 0.15 .}
\text{a. What is the least magnitude of the force $F$, }
\text{ parallel to the plane, that will prevent the sled }
m\vec{g}+\vec{N}+\vec{f}+\vec{F}=m\vec{a}
\text{The force F prevents from slipping \textcolor{red}{down} so the friction $\vec{f}$ should be \textcolor{red}{upward}}
\text{We project on x-axis (along the incline)}
-mg \sin(20^0)+f_\text{max}+F=0
f_\text{max}=\mu_sN=\mu_s mg \cos(20^0)
F=mg(\sin(20^0)-\mu_s\cos(20))=80( 0.34-0.25\times0.94)=8.6 N
\text{from slipping down the plane?}

\text{A loaded penguin sled weighing $80 \mathrm{~N}$ rests on a plane inclined at an angle of $20^{\circ}$ to the horizontal.}
\text{Between the sled and the plane, the coefficient of static friction is 0.25 , and the coefficient of }
\text{kinetic friction is 0.15 .}
m\vec{g}+\vec{N}+\vec{f}+\vec{F}=m\vec{a}
\text{The force F wants to start the mass moving up so the friction $\vec{f}$ should be \textcolor{red}{downward}}
\text{We project on x-axis (along the incline)}
-mg \sin(20^0)-f_\text{max}+F=0
f_\text{max}=\mu_sN=\mu_s mg \cos(20^0)
F=mg(\sin(20^0)+\mu_s\cos(20))=80( 0.34 0.25\times0.94)=46.2 N
\text{b) What is the minimum magnitude of $F$ that will start }
\text{the sled moving up the plane?}

\text{A loaded penguin sled weighing $80 \mathrm{~N}$ rests on a plane inclined at an angle of $20^{\circ}$ to the horizontal.}
\text{Between the sled and the plane, the coefficient of static friction is 0.25 , and the coefficient of }
\text{kinetic friction is 0.15 .}
m\vec{g}+\vec{N}+\vec{f}+\vec{F}=m\vec{a}
\text{The force F moves the mass up at a constant $v$, the friction $\vec{f}$ should be \textcolor{red}{downward}}
\text{We project on x-axis (along the incline)}
-mg \sin(20^0)-f+F=0
f=\mu_k N=\mu_k mg \cos(20^0)
F=mg(\sin(20^0)+\mu_k\cos(20))=80( 0.34 +0.15\times0.94)=38.6N
\text{c) What value of $F$ is required to move the sled up ?}
\text{the plane at constant velocity?}

Chapter 6-Phys101
By smstry
Chapter 6-Phys101
- 259



