Chapter 28
\text{Chapter 28}
Chapter 28
\text{Chapter 28}
Chapter 28
\text{Chapter 28}
Chapter 28
\text{Chapter 28}
A wire 80.0 cm long carries a 0.820 A current in the positive direction of a y axis through
\text{A wire $80.0 \mathrm{~cm}$ long carries a $0.820 \mathrm{~A}$ current in the positive direction of a y axis through }
a magnetic field B=(4.00i^+12.0j^)mT.
\text{a magnetic field $\vec{B}=(4.00 \hat{i}+12.0 \hat{j}) m T$. }
In unit vector notation, what is the magnetic force on the wire?
\text{In unit vector notation, what is the magnetic force on the wire?}
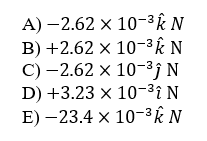
A wire 80.0 cm long carries a 0.820 A current in the positive direction of a y axis through
\text{A wire $80.0 \mathrm{~cm}$ long carries a $0.820 \mathrm{~A}$ current in the positive direction of a y axis through }
a magnetic field B=(4.00i^+12.0j^)mT.
\text{a magnetic field $\vec{B}=(4.00 \hat{i}+12.0 \hat{j}) m T$. }
In unit vector notation, what is the magnetic force on the wire?
\text{In unit vector notation, what is the magnetic force on the wire?}
F=il×B=0.82[0.8^×(4^+12^)10−3]=0.82×10−3[3.2×(−k^)]=−2.624×10−3k^N
\begin{aligned}
\vec{\mathrm{F}} & =\mathrm{i} \vec{l} \times \vec{B} \\
& =0.82\left[0.8 \hat{\jmath} \times(4 \hat{\imath}+12 \hat{\jmath}) 10^{-3}\right] \\
& =0.82 \times 10^{-3}[3.2 \times(-\hat{k})]=-2.624 \times 10^{-3} \hat{k} N
\end{aligned}
Reminder:
\text{Reminder:}
i^×j^=k^
\hat{i}\times\hat{j}=\hat{k}
j^×j^=0
\hat{j}\times\hat{j}=0
At one instant an electron is moving in the xy plane, the components of its velocity being
\text{At one instant an electron is moving in the $\mathrm{xy}$ plane, the components of its velocity being}
vx=5.0×105 m/s and vy=3.0×105 m/s. A magnetic field of 0.80 T is in the positive
\text{$\mathrm{v}_{\mathrm{x}}=5.0 \times 10^5 \mathrm{~m} / \mathrm{s}$ and $\mathrm{v}_{\mathrm{y}}=3.0 \times 10^5 \mathrm{~m} / \mathrm{s}$. A magnetic field of $0.80 \mathrm{~T}$ is in the positive }
x-direction. At that instant the magnitude of the magnetic force on the electron is:
\text{$\mathrm{x}$-direction. At that instant the magnitude of the magnetic force on the electron is:}

At one instant an electron is moving in the xy plane, the components of its velocity being
\text{At one instant an electron is moving in the $\mathrm{xy}$ plane, the components of its velocity being}
vx=5.0×105 m/s and vy=3.0×105 m/s. A magnetic field of 0.80 T is in the positive
\text{$\mathrm{v}_{\mathrm{x}}=5.0 \times 10^5 \mathrm{~m} / \mathrm{s}$ and $\mathrm{v}_{\mathrm{y}}=3.0 \times 10^5 \mathrm{~m} / \mathrm{s}$. A magnetic field of $0.80 \mathrm{~T}$ is in the positive }
x-direction. At that instant the magnitude of the magnetic force on the electron is:
\text{$\mathrm{x}$-direction. At that instant the magnitude of the magnetic force on the electron is:}
vF=(5×105^+3×105^)sm,B=0.8^=qv×B=e(−0.8×3×105)k^=3.84×10−14 Nk^
\begin{aligned}
\vec{v} & =\Big(5 \times 10^5 \hat{\imath}+3 \times 10^5 \hat{\jmath}\Big) \frac{\mathrm{m}}{\mathrm{s}}, \quad \overrightarrow{\mathrm{B}}=0.8 \hat{\imath} \\
\overrightarrow{\mathrm{F}} & =\mathrm{q} \overrightarrow{\mathrm{v}} \times \overrightarrow{\mathrm{B}} \\
& =\mathrm{e}\left(-0.8 \times 3 \times 10^5\right) \hat{\mathrm{k}}=3.84 \times 10^{-14} \mathrm{~N} \hat{\mathrm{k}}
\end{aligned}
At one instant an electron is moving in the xy plane, the components of its velocity being
\text{At one instant an electron is moving in the $\mathrm{xy}$ plane, the components of its velocity being}
vx=5.0×105 m/s and vy=3.0×105 m/s. A magnetic field of 0.80 T is in the positive
\text{$\mathrm{v}_{\mathrm{x}}=5.0 \times 10^5 \mathrm{~m} / \mathrm{s}$ and $\mathrm{v}_{\mathrm{y}}=3.0 \times 10^5 \mathrm{~m} / \mathrm{s}$. A magnetic field of $0.80 \mathrm{~T}$ is in the positive }
x-direction. At that instant the magnitude of the magnetic force on the electron is:
\text{$\mathrm{x}$-direction. At that instant the magnitude of the magnetic force on the electron is:}
vF=(5×105^+3×105^)sm,B=0.8^=qv×B=e(−0.8×3×105)k^=3.84×10−14 Nk^
\begin{aligned}
\vec{v} & =\Big(5 \times 10^5 \hat{\imath}+3 \times 10^5 \hat{\jmath}\Big) \frac{\mathrm{m}}{\mathrm{s}}, \quad \overrightarrow{\mathrm{B}}=0.8 \hat{\imath} \\
\overrightarrow{\mathrm{F}} & =\mathrm{q} \overrightarrow{\mathrm{v}} \times \overrightarrow{\mathrm{B}} \\
& =\mathrm{e}\left(-0.8 \times 3 \times 10^5\right) \hat{\mathrm{k}}=3.84 \times 10^{-14} \mathrm{~N} \hat{\mathrm{k}}
\end{aligned}
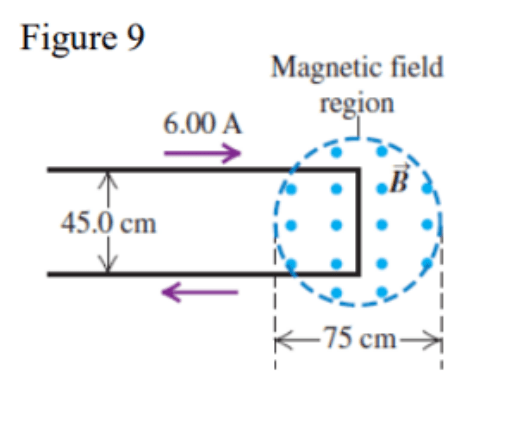
A long wire carrying a 6.00 A current reverses direction by means of two right-angle bends,
\text{A long wire carrying a $6.00 \mathrm{~A}$ current reverses direction by means of two right-angle bends, }
as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of
\text{as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of }
magnitude 0.70 T confined to a circular region of diameter 75 cm.
\text{magnitude $0.70 \mathrm{~T}$ confined to a circular region of diameter $75 \mathrm{~cm}$.}
What is the net magnetic force on the wire?
\text{What is the net magnetic force on the wire?}
E) 1.9 N, to the left
\text{E) 1.9 $\mathrm{~N}$, to the left}
B) 1.9 N, to the right
\text{B) $1.9 \mathrm{~N}$, to the right}
C) zero
\text{C) zero}
D) 1.6 N, to the right
\text{D) $1.6 \mathrm{~N}$, to the right}
A) 1.6 N, to the left
\text{A) $1.6 \mathrm{~N}$, to the left}

A long wire carrying a 6.00 A current reverses direction by means of two right-angle bends,
\text{A long wire carrying a $6.00 \mathrm{~A}$ current reverses direction by means of two right-angle bends, }
as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of
\text{as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of }
magnitude 0.70 T confined to a circular region of diameter 75 cm.
\text{magnitude $0.70 \mathrm{~T}$ confined to a circular region of diameter $75 \mathrm{~cm}$.}
What is the net magnetic force on the wire?
\text{What is the net magnetic force on the wire?}
The forces on the upper and lower sides cancel on the right side:
\text{The forces on the upper and lower sides cancel on the right side:}
(L×B) is to the left
\text{$(\vec{L} \times \vec{B})$ is to the left}
F=iLB=6×0.45×0.7=1.9 N
\begin{aligned}
& \mathrm{F}=\mathrm{iLB} \\
& =6 \times 0.45 \times 0.7=1.9 \mathrm{~N}
\end{aligned}
(Answer E)
\text{(Answer E)}
A long wire carrying a 6.00 A current reverses direction by means of two right-angle bends,
\text{A long wire carrying a $6.00 \mathrm{~A}$ current reverses direction by means of two right-angle bends, }
as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of
\text{as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of }
magnitude 0.70 T confined to a circular region of diameter 75 cm.
\text{magnitude $0.70 \mathrm{~T}$ confined to a circular region of diameter $75 \mathrm{~cm}$.}
What is the net magnetic force on the wire?
\text{What is the net magnetic force on the wire?}
(Answer E)
\text{(Answer E)}
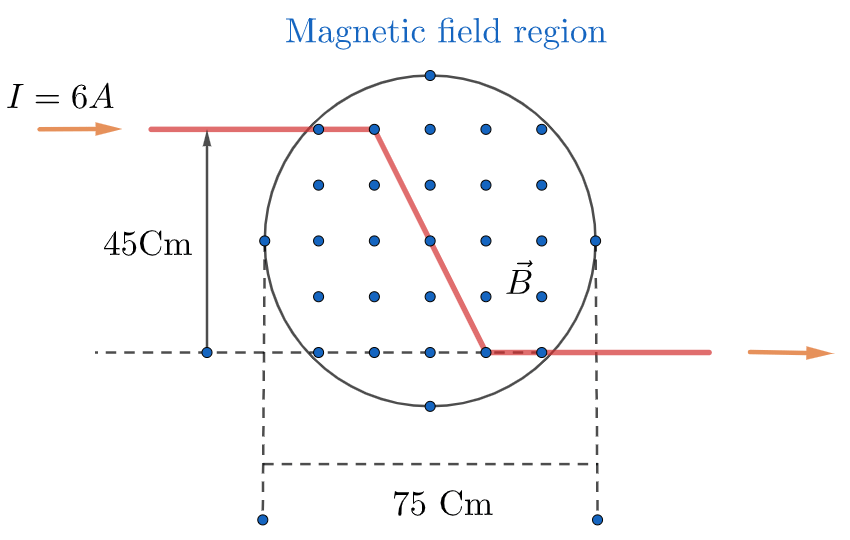
What if we change the configuration as in this figure ?
\text{What if we change the configuration as in this figure ?}
A long wire carrying a 6.00 A current reverses direction by means of two right-angle bends,
\text{A long wire carrying a $6.00 \mathrm{~A}$ current reverses direction by means of two right-angle bends, }
as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of
\text{as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of }
magnitude 0.70 T confined to a circular region of diameter 75 cm.
\text{magnitude $0.70 \mathrm{~T}$ confined to a circular region of diameter $75 \mathrm{~cm}$.}
What is the net magnetic force on the wire?
\text{What is the net magnetic force on the wire?}

What if we change the configuration as in this figure ?
\text{What if we change the configuration as in this figure ?}
The vector L (in green) is the sum of the three portions inside the magnetic field region
\text{The vector $\vec{L}$ (in green) is the sum of the three portions inside the magnetic field region }
F=iLB=i2RB=6×0.75×0.7=3.15N
F=iL B=i2RB=6 \times 0.75\times 0.7=3.15 N
Can you find the direction of the force?
\text{Can you find the direction of the force?}
sinθ=0.45/0.75=0.6
\sin \theta=0.45/0.75=0.6
θ
\theta
A long wire carrying a 6.00 A current reverses direction by means of two right-angle bends,
\text{A long wire carrying a $6.00 \mathrm{~A}$ current reverses direction by means of two right-angle bends, }
as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of
\text{as shown in FIGURE 9. The part of the wire where the bend occurs is in a magnetic field of }
magnitude 0.70 T confined to a circular region of diameter 75 cm.
\text{magnitude $0.70 \mathrm{~T}$ confined to a circular region of diameter $75 \mathrm{~cm}$.}
What is the net magnetic force on the wire?
\text{What is the net magnetic force on the wire?}

What if we change the configuration as in this figure ?
\text{What if we change the configuration as in this figure ?}
The vector L (in green) is the sum of the three portions inside the magnetic field region
\text{The vector $\vec{L}$ (in green) is the sum of the three portions inside the magnetic field region }
F=iLB=i2RB=6×0.75×0.7=3.15N
F=iL B=i2RB=6 \times 0.75\times 0.7=3.15 N
Can you find the direction of the force?
\text{Can you find the direction of the force?}
sinθ=0.45/0.75=0.6
\sin \theta=0.45/0.75=0.6
θ
\theta
The force F makes −(900+36.9=126.90) with the x-axis
\text{The force $\vec{F}$ makes $-(90^0+36.9=126.9^0)$ with the x-axis}
θ=36.90
\theta=36.9^0
F
\vec{F}
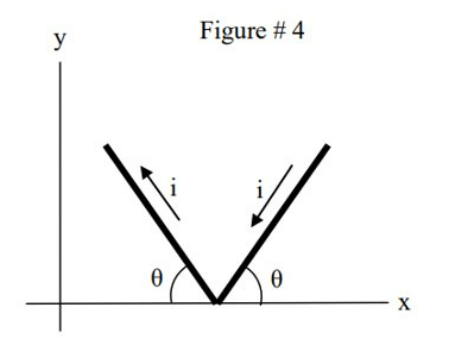
A wire is bent as shown in Figure 4. It lies in a uniform magnetic field B=4.0k^T.
\text{A wire is bent as shown in Figure 4. It lies in a uniform magnetic field $\vec{B}=4.0 \hat{\mathrm{k}} \mathrm{T}$. }
Each wire section is 2.0 m long and makes an angle of θ=60∘ with the x-axis, and the wire
\text{Each wire section is $2.0 \mathrm{~m}$ long and makes an angle of $\theta=60^{\circ}$ with the $\mathrm{x}$-axis, and the wire }
carries a current of 2.0 A. What is the the net magnetic force on the wire?
\text{carries a current of $2.0 \mathrm{~A}$. What is the the net magnetic force on the wire?}
(the positive z-axis is out of the page).
\text{(the positive $\mathrm{z}$-axis is out of the page).}
A) +16 j^N
\text{A) $ +16\text{ } \hat{\mathrm{j}} \mathrm{N}$}
B) +28 j^N
\text{B) $+28 \text{ } \hat{\mathrm{j}} \mathrm{N}$}
C) −28 i^N
\text{C) $-28 \text{ } \hat{\mathrm{i}} \mathrm{N}$}
D) −16 j^N
\text{D) $-16 \text{ } \hat{\mathrm{j}} \mathrm{N}$}
E) Zero
\text{E) Zero}
A wire is bent as shown in Figure 4. It lies in a uniform magnetic field B=4.0k^T.
\text{A wire is bent as shown in Figure 4. It lies in a uniform magnetic field $\vec{B}=4.0 \hat{\mathrm{k}} \mathrm{T}$. }
Each wire section is 2.0 m long and makes an angle of θ=60∘ with the x-axis, and the wire
\text{Each wire section is $2.0 \mathrm{~m}$ long and makes an angle of $\theta=60^{\circ}$ with the $\mathrm{x}$-axis, and the wire }
carries a current of 2.0 A. What is the the net magnetic force on the wire?
\text{carries a current of $2.0 \mathrm{~A}$. What is the the net magnetic force on the wire?}
(the positive z-axis is out of the page).
\text{(the positive $\mathrm{z}$-axis is out of the page).}

L
\vec{L}
The smart way to solve this problem is to use the total L
\text{The smart way to solve this problem is to use the total $\vec{L}$}
L=−Li^
\vec{L}=-L\hat{i}
F=iL×B=iLB(−i^×k^)=+iLBj^
\vec{F}=i\vec{L}\times \vec{B}=i L B (-\hat{i}\times\hat{k})=+i L B\hat{j}
F=+iLBj^=i2lcosθBj^=2×2×2×0.5×4j=^16j^
\vec{F}=+i L B\hat{j}=i2l\cos\theta B \hat{j}=2\times 2\times 2\times 0.5\times 4\hat{j=}16\hat{j}
l
l
l
l
The bent wire shown below lies in a uniform magnetic field. The wire carries a current of 2.0 A,
\text{The bent wire shown below lies in a uniform magnetic field. The wire carries a current of $2.0 \mathrm{~A}$, }
and the two straight sections each has a length of 2.0 m.
\text{and the two straight sections each has a length of $2.0 \mathrm{~m}$.}
What is the net magnetic force on the wire if the magnetic field is B=4.0i^(T) ?
\text{What is the net magnetic force on the wire if the magnetic field is $\overrightarrow{\mathbf{B}}=4.0 \hat{\mathbf{i}}(\mathrm{T})$ ?}
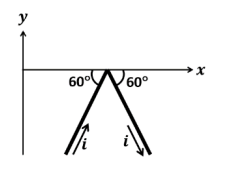
A) zero B) +28k^ C) −28k^ D) +16k^ E) −16k^
\begin{aligned}
& \text { A) }\text{zero} \\
& \text { B) }+28 \hat{k} \\
& \text { C) }-28 \hat{k} \\
& \text { D) }+16 \hat{k} \\
& \text { E) }-16 \hat{k}
\end{aligned}
Can you solve it using the same method?
\text{Can you solve it using the same method?}
A particle with mass m and charge q, moving with a velocity v enters a region of uniform
\text{A particle with mass $\mathrm{m}$ and charge $\mathrm{q}$, moving with a velocity $\mathbf{v}$ enters a region of uniform}
magnetic field B, as shown in FIGURE 7. The particle strikes the wall at a distance d from
\text{magnetic field $\mathbf{B}$, as shown in FIGURE 7. The particle strikes the wall at a distance $\mathrm{d}$ from }
the entrance slit. If the particle’s velocity stays the same but its charge to mass ratio is
\text{the entrance slit. If the particle's velocity stays the same but its charge to mass ratio is }
doubled, at what distance from the entrance slit will the particle strike the wall?
\text{doubled, at what distance from the entrance slit will the particle strike the wall?}
A) d/2
\text{A) $d / 2$}
B) d2
\text{B) $\mathrm{d} \sqrt{2 }$}
C) d
\text{C) $\mathrm{d}$}
D) d/2
\text{D) $\mathrm{d} / \sqrt{2} $}
E) 2 d
\text{E) $2 \mathrm{~d}$}
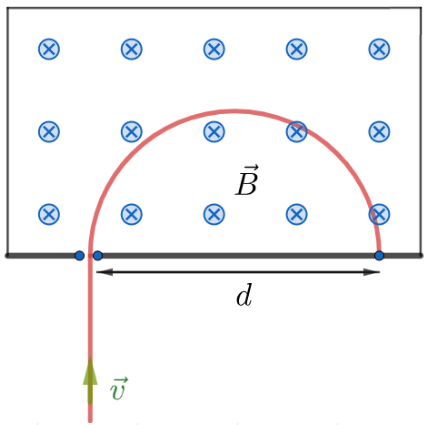
A particle with mass m and charge q, moving with a velocity v enters a region of uniform
\text{A particle with mass $\mathrm{m}$ and charge $\mathrm{q}$, moving with a velocity $\mathbf{v}$ enters a region of uniform}
magnetic field B, as shown in FIGURE 7. The particle strikes the wall at a distance d from
\text{magnetic field $\mathbf{B}$, as shown in FIGURE 7. The particle strikes the wall at a distance $\mathrm{d}$ from }
the entrance slit. If the particle’s velocity stays the same but its charge to mass ratio is
\text{the entrance slit. If the particle's velocity stays the same but its charge to mass ratio is }
doubled, at what distance from the entrance slit will the particle strike the wall?
\text{doubled, at what distance from the entrance slit will the particle strike the wall?}
A) d/2
\text{A) $d / 2$}
B) d2
\text{B) $\mathrm{d} \sqrt{2 }$}
C) d
\text{C) $\mathrm{d}$}
D) d/2
\text{D) $\mathrm{d} / \sqrt{2} $}
E) 2 d
\text{E) $2 \mathrm{~d}$}

RR′RR′=qBmv=Bv(mq)=Bv(mq)′=(mq)′(mq)=21⇒R′=2R⇒d′=2d
\begin{aligned}
\mathrm{R} & =\frac{m v}{q B}=\frac{v}{B}\left(\frac{q}{m}\right) \\
\mathrm{R}^{\prime} & =\frac{v}{B}\left(\frac{q}{m}\right)^{\prime} \\
\frac{\mathrm{R}^{\prime}}{\mathrm{R}} & =\frac{\left(\frac{q}{m}\right)}{\left(\frac{q}{m}\right)^{\prime}}=\frac{1}{2} \Rightarrow \mathrm{R}^{\prime}=\frac{\mathrm{R}}{2} \Rightarrow d^\prime=\frac{d}{2}
\end{aligned}
The coil in the figure has its plane parallel to the xz plane and carries current i=1.0A in
\text{The coil in the figure has its plane parallel to the $x z$ plane and carries current $i=1.0 A$ in }
the direction indicated. The coil has 8.0 turns and a cross sectional area of 4.0×10−3 m2
\text{the direction indicated. The coil has 8.0 turns and a cross sectional area of $4.0 \times 10^{-3} \mathrm{~m}^2$ }
and lies in an external uniform magnetic field that is given by B=(−2.0mT)^.
\text{and lies in an external uniform magnetic field that is given by $\vec{B}=(-2.0 m T) \hat{\imath}$. }
Find the torque (in units of μN.m) on the coil due to the magnetic field.
\text{Find the torque (in units of $\mu \mathrm{N} . \mathrm{m})$ on the coil due to the magnetic field.}
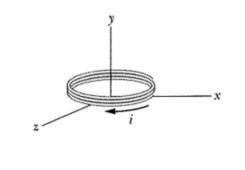
A) −64k^ B) +64k^ C) +12^ D) −12^ E) −64^
\begin{aligned}
& \text { A) }-64 \hat{k} \\
& \text { B) }+64 \hat{k} \\
& \text { C) }+12 \hat{\imath} \\
& \text { D) }-12 \hat{\imath} \\
& \text { E) }-64 \hat{\jmath}
\end{aligned}
The coil in the figure has its plane parallel to the xz plane and carries current i=1.0A in
\text{The coil in the figure has its plane parallel to the $x z$ plane and carries current $i=1.0 A$ in }
the direction indicated. The coil has 8.0 turns and a cross sectional area of 4.0×10−3 m2
\text{the direction indicated. The coil has 8.0 turns and a cross sectional area of $4.0 \times 10^{-3} \mathrm{~m}^2$ }
and lies in an external uniform magnetic field that is given by B=(−2.0mT)^.
\text{and lies in an external uniform magnetic field that is given by $\vec{B}=(-2.0 m T) \hat{\imath}$. }
Find the torque (in units of μN.m) on the coil due to the magnetic field.
\text{Find the torque (in units of $\mu \mathrm{N} . \mathrm{m})$ on the coil due to the magnetic field.}

τ=μ×B
\vec{\tau}=\vec{\mu} \times \vec{B}
μ=NAi(−^)
\vec{\mu}=N A i(-\hat{\jmath})
(we used the right hand rule to get the direction.)
\text{(we used the right hand rule to get the direction.)}
τ=NAi(−^)×B(−^)τ=NAiB(−k^)τ=−8(4×10−3)(1)(2×10−3)τ=(−64 μN⋅m)k^
\begin{aligned}
& \vec{\tau}=N A i(-\hat{\jmath}) \times B(-\hat{\imath}) \\
& \vec{\tau}=N A i B(-\hat{k}) \\
& \vec{\tau}=-8\left(4 \times 10^{-3}\right)(1)\left(2 \times 10^{-3}\right) \\
& \vec{\tau}=(-64 \text{ } \mu \mathrm{N\cdot m}) \hat{k}
\end{aligned}
j^×i^=−k^
\hat{j} \times \hat{i}=-\hat{k}
Answer A
\text{Answer A}
The coil in FIGURE 11 carries a current i=2.0 A in the direction indicated, has 3.0 turns
\text{The coil in FIGURE 11 carries a current $i=2.0 \mathrm{~A}$ in the direction indicated, has 3.0 turns }
and a cross section area of 4.0×10−3 m2, and lies in a uniform magnetic field
\text{and a cross section area of $4.0 \times 10^{-3} \mathrm{~m}^2$, and lies in a uniform magnetic field }
B =(2.0i^−3.0j^−4.0k^)10−3 T.
\text{$\overrightarrow{\boldsymbol{B}}$ $=(2.0 \hat{\boldsymbol{i}}-3.0 \hat{\boldsymbol{j}}-4.0 \hat{\boldsymbol{k}}) 10^{-3} \mathrm{~T}$. }
What is the torque (in unit-vector notation) on the coil due to the magnetic field?
\text{What is the torque (in unit-vector notation) on the coil due to the magnetic field?}
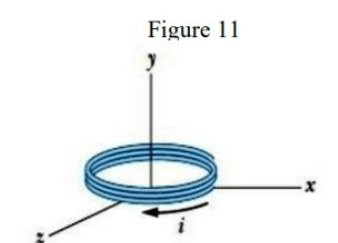
A) (96i^+48k^)10−6 N⋅m
\text{
A) $(96 \hat{\boldsymbol{i}}+48 \hat{\boldsymbol{k}}) 10^{-6} \mathrm{~N} \cdot \mathrm{m}$
}
B) (45i^+62k^)10−6 N⋅m
\text{
B) $(45 \hat{i}+62 \hat{\boldsymbol{k}}) 10^{-6} \mathrm{~N} \cdot \mathrm{m}$
}
C) (23i^+40k^)10−6 N.m
\text{
C) $(23 \hat{\boldsymbol{i}}+40 \hat{\boldsymbol{k}}) 10^{-6} \mathrm{~N} . \mathrm{m}$
}
D) (10i^+8.0k^)10−6 N.m
\text{
D) $(10 \hat{i}+8.0 \hat{\boldsymbol{k}}) 10^{-6} \mathrm{~N} . \mathrm{m}$
}
E) (45i^+72k^)10−6 N⋅m
\text{
E) $(45 \hat{\boldsymbol{i}}+72 \hat{\boldsymbol{k}}) 10^{-6} \mathrm{~N} \cdot \mathrm{m}$
}
The coil in FIGURE 11 carries a current i=2.0 A in the direction indicated, has 3.0 turns
\text{The coil in FIGURE 11 carries a current $i=2.0 \mathrm{~A}$ in the direction indicated, has 3.0 turns }
and a cross section area of 4.0×10−3 m2, and lies in a uniform magnetic field
\text{and a cross section area of $4.0 \times 10^{-3} \mathrm{~m}^2$, and lies in a uniform magnetic field }
B =(2.0i^−3.0j^−4.0k^)10−3 T.
\text{$\overrightarrow{\boldsymbol{B}}$ $=(2.0 \hat{\boldsymbol{i}}-3.0 \hat{\boldsymbol{j}}-4.0 \hat{\boldsymbol{k}}) 10^{-3} \mathrm{~T}$. }
What is the torque (in unit-vector notation) on the coil due to the magnetic field?
\text{What is the torque (in unit-vector notation) on the coil due to the magnetic field?}

τμτ=μ×B=NiA=3×2×2×10−3×(−)=−24×10−3=−24×10−3×(2.0−3.0−4.0k)×10−3=−24×2×10−6×(×)+24×10−6×4(×k)=(96+48k)×10−6 N.m
\begin{aligned}
\vec{\tau} & =\vec{\mu} \times \vec{B} \\
\vec{\mu} & =N_i \vec{A}=3 \times 2 \times 2 \times 10^{-3} \times(-\vec{\jmath})=-24 \times 10^{-3} \vec{\jmath} \\
\vec{\tau} & =-24 \times 10^{-3} \vec{\jmath} \times(2.0 \vec{\imath}-3.0 \vec{\jmath}-4.0 \vec{k}) \times 10^{-3} \\
& =-24 \times 2 \times 10^{-6} \vec{\jmath} \times(\vec{\jmath} \times \vec{\imath})+24 \times 10^{-6} \times 4(\vec{\jmath} \times \vec{k}) \\
& =(96 \vec{\imath}+48 \vec{k}) \times 10^{-6} \mathrm{~N} . \mathrm{m}
\end{aligned}
Consider a square current loop with a side length of 25 cm that carries a current I=5.6 A as
\text{Consider a square current loop with a side length of $25 \mathrm{~cm}$ that carries a current $I=5.6 \mathrm{~A}$ as }
shown below. A constant 0.25T magnetic field makes angle θ=30∘ with the direction normal
\text{shown below. A constant $0.25\mathrm{T}$ magnetic field makes angle $\theta=30^{\circ}$ with the direction normal }
to the plane of the loop. Find the magnitude of the torque on the loop.
\text{to the plane of the loop. Find the magnitude of the torque on the loop.}
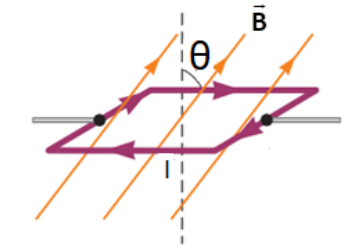
A) 4.4×10−2 N.m
\text{
A) $4.4 \times 10^{-2} \mathrm{~N} . \mathrm{m}$
}
B) 2.2×10−2 N.m
\text{
B) $2.2 \times 10^{-2} \mathrm{~N} . \mathrm{m}$
}
C) 3.7×10−2 N.m
\text{
C) $3.7 \times 10^{-2} \mathrm{~N} . \mathrm{m}$
}
D) 1.0×10−2 N.m
\text{
D) $1.0 \times 10^{-2} \mathrm{~N} . \mathrm{m}$
}
E) 2.8×10−2 N.m
\text{E) $2.8 \times 10^{-2} \mathrm{~N} . \mathrm{m}$}
A magnetic dipole with a dipole moment ∣μ∣=2.3×10−6Am2 is placed in a uniform magnetic
\text{A magnetic dipole with a dipole moment $|\vec{\mu}|=2.3 \times 10^{-6} \mathrm{Am}^2$ is placed in a uniform magnetic }
field of magnitude 0.97 T. Find the work required to change the orientation of the dipole from
\text{field of magnitude $0.97 \mathrm{~T}$. Find the work required to change the orientation of the dipole from }
its initial state to the final state as shown below.
\text{its initial state to the final state as shown below.}
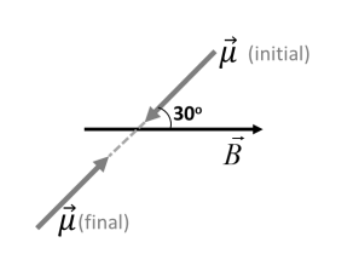
A) 3.9 μJ
\text{
A) $3.9 \text{ }\mu \mathrm{J}$
}
B) 7.6 μJ
\text{
B) $7.6 \text{ }\mu \mathrm{J}$
}
C) 1.5 μJ
\text{C) $1.5 \text{ }\mu \mathrm{J}$
}
D) 5.3 μJ
\text{D) $5.3 \text{ }\mu \mathrm{J}$
}
E) 0.010 μJ
\text{E) $0.010 \text{ }\mu \mathrm{J}$}
A magnetic dipole with a dipole moment ∣μ∣=2.3×10−6Am2 is placed in a uniform magnetic
\text{A magnetic dipole with a dipole moment $|\vec{\mu}|=2.3 \times 10^{-6} \mathrm{Am}^2$ is placed in a uniform magnetic }
field of magnitude 0.97 T. Find the work required to change the orientation of the dipole from
\text{field of magnitude $0.97 \mathrm{~T}$. Find the work required to change the orientation of the dipole from }
its initial state to the final state as shown below.
\text{its initial state to the final state as shown below.}

A) 3.9 μJ
\text{
A) $3.9 \text{ }\mu \mathrm{J}$
}
B) 7.6 μJ
\text{
B) $7.6 \text{ }\mu \mathrm{J}$
}
C) 1.5 μJ
\text{C) $1.5 \text{ }\mu \mathrm{J}$
}
D) 5.3 μJ
\text{D) $5.3 \text{ }\mu \mathrm{J}$
}
E) 0.010 μJ
\text{E) $0.010 \text{ }\mu \mathrm{J}$}
Wa=ΔU=−μf⋅B+μi⋅B
W_a=\Delta U=-\vec{\mu_\text{f}}\cdot \vec{B}+\vec{\mu_\text{i}}\cdot \vec{B}
U=−μ⋅B
U=-\vec{\mu}\cdot \vec{B}
=−μBcos300+μBcos1500
=-\mu B \cos 30^0+\mu B \cos150^0
=−2μBcos(30)
=-2\mu B \cos(30)
=−2×2.3×10−6×0.97×3/2
=-2\times2.3 \times 10^{-6}\times 0.97\times \sqrt{3}/2
=−3.9 μJ
=-3.9\text{ } \mu J

Figure 9 shows a 20-turn rectangular coil of dimensions 10 cm by 5 cm. It is hinged along
\text{Figure 9 shows a 20-turn rectangular coil of dimensions $10 \mathrm{~cm}$ by $5 \mathrm{~cm}$. It is hinged along }
one long side (z-axis), and carries a current i=0.1 A. A uniform magnetic field of B=0.5i^ T
\text{one long side (z-axis), and carries a current $i=0.1 \mathrm{~A}$. A uniform magnetic field of $\vec{B}=0.5 \hat{i} \mathrm{~T}$ }
is present in the region. What is the torque acting on the coil about the hinge line?
\text{is present in the region. What is the torque acting on the coil about the hinge line?}
A) −5×10−3k^Nm
\text{
A) $-5 \times 10^{-3} \hat{k} \mathrm{Nm}$
}
B) +5×10−3k^Nm
\text{
B) $+5 \times 10^{-3} \hat{k} \mathrm{Nm}$
}
C) −2×10−3j^Nm
\text{
C) $-2 \times 10^{-3} \hat{j} \mathrm{Nm}$
}
D) +2×10−3j^Nm
\text{
D) $+2 \times 10^{-3} \hat{j} \mathrm{Nm}$
}
E) −2×10−3k^Nm
\text{
E) $-2 \times 10^{-3} \hat{k} \mathrm{Nm}$}

Figure 9 shows a 20-turn rectangular coil of dimensions 10 cm by 5 cm. It is hinged along
\text{Figure 9 shows a 20-turn rectangular coil of dimensions $10 \mathrm{~cm}$ by $5 \mathrm{~cm}$. It is hinged along }
one long side (z-axis), and carries a current i=0.1 A. A uniform magnetic field of B=0.5i^ T
\text{one long side (z-axis), and carries a current $i=0.1 \mathrm{~A}$. A uniform magnetic field of $\vec{B}=0.5 \hat{i} \mathrm{~T}$ }
is present in the region. What is the torque acting on the coil about the hinge line?
\text{is present in the region. What is the torque acting on the coil about the hinge line?}
μ=NiA=20×0.1×10×10−2×5×10−2j^=10−2j^τ=μ×B=10−2j^×0.5i^=−5×10−3k
\begin{aligned}
& \vec{\mu}=N i \vec{A}=20 \times 0.1 \times 10 \times 10^{-2} \times 5 \times 10^{-2} \hat{j}=10^{-2} \hat{j} \\
& \vec{\tau}=\vec{\mu} \times \vec{B}=10^{-2} \hat{j} \times 0.5 \hat{i}=-5 \times 10^{-3} \vec{k}
\end{aligned}
C h a p t e r 2 8 \text{Chapter 28} C h a p t e r 2 8 \text{Chapter 28} C h a p t e r 2 8 \text{Chapter 28} C h a p t e r 2 8 \text{Chapter 28}
Chapter 28
By smstry
Chapter 28
- 126



