Chapter 18
\text{Chapter 18}
Chapter 18
\text{Chapter 18}
Chapter 18
\text{Chapter 18}
Chapter 18
\text{Chapter 18}
Dr. Adel Abbout
\text{Dr. Adel Abbout}
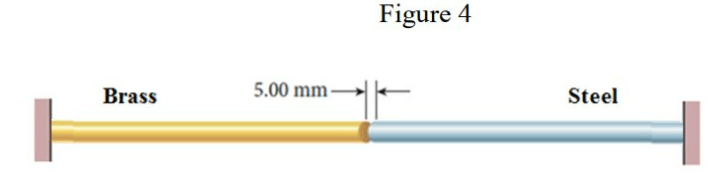
The ends of the two brass and steel rods, each 1.00 m long; as shown in the Figure 4, are separated
\text{The ends of the two brass and steel rods, each $1.00 \mathrm{~m}$ long; as shown in the Figure 4, are separated}
by 5.00 mm at 25.0∘C. Assuming that the outside ends of both rods rest firmly against rigid supports,
\text{by $5.00 \mathrm{~mm}$ at $25.0^{\circ} \mathrm{C}$. Assuming that the outside ends of both rods rest firmly against rigid supports,}
at what temperature will the inside ends of the rods just touch?
\text{at what temperature will the inside ends of the rods just touch?}\\
(αsteel =13.0×10−6/∘C;αBrass =19.0×10−6/C∘).
\text{$\left(\alpha_{\text {steel }}=13.0 \times 10^{-6} /{ }^{\circ} \mathrm{C} ; \alpha_{\text {Brass }}=19.0 \times 10^{-6} / \mathrm{C}^{\circ}\right)$.}

The ends of the two brass and steel rods, each 1.00 m long; as shown in the Figure 4, are separated
\text{The ends of the two brass and steel rods, each $1.00 \mathrm{~m}$ long; as shown in the Figure 4, are separated}
by 5.00 mm at 25.0∘C. Assuming that the outside ends of both rods rest firmly against rigid supports,
\text{by $5.00 \mathrm{~mm}$ at $25.0^{\circ} \mathrm{C}$. Assuming that the outside ends of both rods rest firmly against rigid supports,}
at what temperature will the inside ends of the rods just touch?
\text{at what temperature will the inside ends of the rods just touch?}\\
(αsteel =13.0×10−6/∘C;αBrass =19.0×10−6/C∘).
\text{$\left(\alpha_{\text {steel }}=13.0 \times 10^{-6} /{ }^{\circ} \mathrm{C} ; \alpha_{\text {Brass }}=19.0 \times 10^{-6} / \mathrm{C}^{\circ}\right)$.}

ΔLBrass +ΔLSteel =d
\Delta L_{\text {Brass }}+\Delta L_{\text {Steel }}=d
ΔT=L(αBrass +αSteel )d=1(19+13)×10−65×10−3=156.3
\Delta T=\frac{d}{L\left(\alpha_{\text {Brass }}+\alpha_{\text {Steel }}\right)}=\frac{5 \times 10^{-3}}{1(19+13) \times 10^{-6}}=156.3
Tf=Ti+ΔT=25+156.3=181.25∘C
T_f=T_i+\Delta T=25+156.3=181.25^{\circ} \mathrm{C}
LαBrass ΔT+LαSteel ΔT=d
L \alpha_\text {Brass }\Delta T+L\alpha_\text {Steel }\Delta T=d
The touching condition is
\text{The touching condition is}
What is the change in density of an aluminum cube of mass 200 g and of edge length 5.0 cm when
\text{What is the change in density of an aluminum cube of mass $200 \mathrm{~g}$ and of edge length $5.0 \mathrm{~cm}$ when }
heated from 10∘C to 80∘C (coefficient of linear expansion of aluminum 23×10−6/∘C).
\text{heated from $10^{\circ} \mathrm{C}$ to $80^{\circ} \mathrm{C}$ (coefficient of linear expansion of aluminum $\left.23 \times 10^{-6} /{ }^{\circ} \mathrm{C}\right)$.}

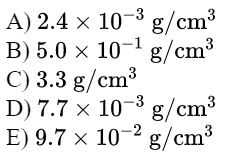
What is the change in density of an aluminum cube of mass 200 g and of edge length 5.0 cm when
\text{What is the change in density of an aluminum cube of mass $200 \mathrm{~g}$ and of edge length $5.0 \mathrm{~cm}$ when }
heated from 10∘C to 80∘C (coefficient of linear expansion of aluminum 23×10−6/∘C).
\text{heated from $10^{\circ} \mathrm{C}$ to $80^{\circ} \mathrm{C}$ (coefficient of linear expansion of aluminum $\left.23 \times 10^{-6} /{ }^{\circ} \mathrm{C}\right)$.}
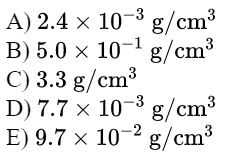

ρ1=53200=58 g/cm3
\rho_1=\frac{200}{5^3}=\frac{8}{5} \mathrm{~g} / \mathrm{cm}^3
ρ2=(5+LαΔT)3200=(5+8.05×10−3)3200=1.5922 g/cm3
\rho_2=\frac{200}{(5+\mathrm{L} \alpha \Delta \mathrm{T})^3}=\frac{200}{\left(5+8.05 \times 10^{-3}\right)^3}=1.5922 \mathrm{~g} / \mathrm{cm}^3
Δρ=∣ρ2−ρ1∣=7.703×10−3 g/cm3
\Delta \rho=\left|\rho_2-\rho_1\right|=7.703 \times 10^{-3} \mathrm{~g} / \mathrm{cm}^3
Answer D
\text{Answer D}
What is the change in density of an aluminum cube of mass 200 g and of edge length 5.0 cm when
\text{What is the change in density of an aluminum cube of mass $200 \mathrm{~g}$ and of edge length $5.0 \mathrm{~cm}$ when }
heated from 10∘C to 80∘C (coefficient of linear expansion of aluminum 23×10−6/∘C).
\text{heated from $10^{\circ} \mathrm{C}$ to $80^{\circ} \mathrm{C}$ (coefficient of linear expansion of aluminum $\left.23 \times 10^{-6} /{ }^{\circ} \mathrm{C}\right)$.}

More elegant way (not required to be learned for the exam)
\text{More elegant way (not required to be learned for the exam)}
ρ=Vm
\rho=\frac{m}{V}
logρ=logm−logV
\log \rho=\log m -\log V
we do now the derivatives
\text{we do now the derivatives}
ρdρ=mdm−VdV
\frac{d\rho}{\rho}=\frac{dm}{m}-\frac{dV}{V}
the mass doesn’t change, so dm=0
\text{the mass doesn't change, so }dm=0
we change dρ by Δρ
\text{we change $d\rho$ by $\Delta \rho$}
ρΔρ=−VΔV=−3αΔT
\frac{\Delta \rho}{\rho}=-\frac{\Delta V}{V}=-3\alpha \Delta T
Δρ=−3αρΔT
{\Delta \rho}=-3\alpha \rho\Delta T
⇒
\Rightarrow
⇒
\Rightarrow
∣Δρ∣=3×23×10−6×58×70=7.728×10−3cm3g
{|\Delta \rho|}=3\times 23\times10^{-6}\times \frac{8}{5}\times70=7.728\times 10^{-3} \text{$\displaystyle \frac{g}{cm^3}$}
Do you want to strengthen your background?
\text{Do you want to strengthen your background?}
(Because ΔV=V3αΔT)
(\text{Because }\Delta V=V3\alpha \Delta T)
A glass container whose volume is 1.00 L is completely filled with a liquid at this at 0.00∘C.
\text{A glass container whose volume is $1.00 \mathrm{~L}$ is completely filled with a liquid at this at $0.00^{\circ} \mathrm{C}$.}
When the filled container is warmed to 55.0∘C, a volume of 8.95 cm3 of the liquid overflows.
\text{When the filled container is warmed to $55.0^{\circ} \mathrm{C}$, a volume of 8.95 $\mathrm{cm}^3$ of the liquid overflows.}
If the coefficient of linear expansion of glass is 5.67×10−6 /C∘, then find the coefficient of volume
\text{If the coefficient of linear expansion of glass is $5.67 \times 10^{-6}$ $/ \mathrm{C}^{\circ}$, then find the coefficient of volume}
expansion of the liquid.
\text{expansion of the liquid. }
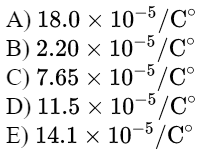
A glass container whose volume is 1.00 L is completely filled with a liquid at this at 0.00∘C.
\text{A glass container whose volume is $1.00 \mathrm{~L}$ is completely filled with a liquid at this at $0.00^{\circ} \mathrm{C}$.}
When the filled container is warmed to 55.0∘C, a volume of 8.95 cm3 of the liquid overflows.
\text{When the filled container is warmed to $55.0^{\circ} \mathrm{C}$, a volume of 8.95 $\mathrm{cm}^3$ of the liquid overflows.}
If the coefficient of linear expansion of glass is 5.67×10−6 /C∘, then find the coefficient of volume
\text{If the coefficient of linear expansion of glass is $5.67 \times 10^{-6}$ $/ \mathrm{C}^{\circ}$, then find the coefficient of volume}
expansion of the liquid.
\text{expansion of the liquid. }
in this problem, the liquid will expand as well as the glass container
\text{in this problem, the liquid will expand as well as the glass container}
for the glass:
\text{for the glass:}
ΔVglass =V0ΔTβglass
\Delta V_{\text {glass }}=V_0 \Delta T\beta_{\text {glass }}
for the liquid:
\text{for the liquid:}
ΔVliquid =V0ΔTβliquid
\Delta V_{\text {liquid }}=V_0 \Delta T\beta_{\text {liquid }}
Vliquid′−Vglass′=(Vliquid0+ΔVliquid)−(Vglass0+ΔVglass)=(ΔVliquid−ΔVglass)
V'_\text{liquid}-V'_\text{glass}=(V^0_\text{liquid}+\Delta V_\text{liquid})-(V^0_\text{glass}+\Delta V_\text{glass})=(\Delta V_\text{liquid}-\Delta V_\text{glass})
because Vglass=Vliquid=V0
\text{because }V_{glass}=V_\text{liquid}=V^0
VOverflow=Vliquid ′−Vglass ′=ΔVliquid −ΔVglass =V0ΔT(βliquid −βglass )
V_\text{Overflow}=V_{\text {liquid }}^{\prime}-V_{\text {glass }}^{\prime}=\Delta V_{\text {liquid }}-\Delta V_{\text {glass }}=V_0 \Delta T\left(\beta_{\text {liquid }}-\beta_{\text {glass }}\right)
βliquid =V0×ΔTΔVoverflow +βglass =103×558.95+3×5.67×10−6
\beta_{\text {liquid }}=\frac{\Delta V_{\text {overflow }}}{V_0 \times \Delta T}+\beta_{\text {glass }}=\frac{8.95}{10^3 \times 55}+3 \times 5.67 \times 10^{-6}
βliquid =17.97×10−5=18.0×10−5/C∘
\beta_{\text {liquid }}=17.97 \times 10^{-5}=18.0 \times 10^{-5} / C^{\circ}
Answer A
\text{Answer A}
Suppose that on a linear temperature scale X, water boils at −53.5∘X and freezes at −170∘X.
\text{Suppose that on a linear temperature scale $\mathrm{X}$, water boils at $-53.5^{\circ} \mathrm{X}$ and freezes at $-170^{\circ} \mathrm{X}$.}
What is a temperature of 340 K on the X scale? (0∘C=273 K)
\text{What is a temperature of $340 \mathrm{~K}$ on the $\mathrm{X}$ scale? $\left(0^{\circ} \mathrm{C}=273 \mathrm{~K}\right)$ }
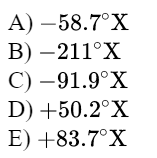
Suppose that on a linear temperature scale X, water boils at −53.5∘X and freezes at −170∘X.
\text{Suppose that on a linear temperature scale $\mathrm{X}$, water boils at $-53.5^{\circ} \mathrm{X}$ and freezes at $-170^{\circ} \mathrm{X}$.}
What is a temperature of 340 K on the X scale? (0∘C=273 K)
\text{What is a temperature of $340 \mathrm{~K}$ on the $\mathrm{X}$ scale? $\left(0^{\circ} \mathrm{C}=273 \mathrm{~K}\right)$ }
373 K
\text{373 K}
273 K
\text{273 K}
−53.50X
\text{$-53.5^0X$}
−1700X
\text{$-170^0X$}
340 K
\text{340 K}
x
x
−53.5−(−170)−53.5−x=373−273373−340
\frac{-53.5-x}{-53.5-(-170)}=\frac{373-340}{373-273}
−116.553.5+x=10033
\frac{53.5+x}{-116.5}=\frac{33}{100}
x=−10033116.5−53.5=−91.8
x=-\frac{33}{100}116.5-53.5=-91.8
Answer C
\text{Answer C}
similar ratios should be equal
\text{similar ratios should be equal}
A 150 g of water at 30.0∘C is poured over a 60.0 g cube of ice at a temperature of −5.00∘C. How many
\text{A $150 \mathrm{~g}$ of water at $30.0^{\circ} \mathrm{C}$ is poured over a $60.0 \mathrm{~g}$ cube of ice at a temperature of $-5.00^{\circ} \mathrm{C}$. How many}
gram of ice has melted when the ice-water mixture has reached thermal equilibrium
\text{gram of ice has melted when the ice-water mixture has reached thermal equilibrium }
specific heat of ice cice =2220 J/kg.K; heat of fusion of ice LF =333 kJ/kg)?
\text{specific heat of ice $\mathrm{c}_{\text {ice }}=2220 \mathrm{~J} / \mathrm{kg} . \mathrm{K}$; heat of fusion of ice $\mathrm{L}_{\mathrm{F}}$ $=333 \mathrm{~kJ} / \mathrm{kg}) ?$}

A 150 g of water at 30.0∘C is poured over a 60.0 g cube of ice at a temperature of 5.00∘C. How many
\text{A $150 \mathrm{~g}$ of water at $30.0^{\circ} \mathrm{C}$ is poured over a $60.0 \mathrm{~g}$ cube of ice at a temperature of $5.00^{\circ} \mathrm{C}$. How many}
gram of ice has melted when the ice-water mixture has reached thermal equilibrium
\text{gram of ice has melted when the ice-water mixture has reached thermal equilibrium }
specific heat of ice cice =2220 J/kg.K; heat of fusion of ice LF =333 kJ/kg)?
\text{specific heat of ice $\mathrm{c}_{\text {ice }}=2220 \mathrm{~J} / \mathrm{kg} . \mathrm{K}$; heat of fusion of ice $\mathrm{L}_{\mathrm{F}}$ $=333 \mathrm{~kJ} / \mathrm{kg}) ?$}
If we have a mixture of ice-water at equilibrium then, T=0
\text{If we have a mixture of ice-water at equilibrium then, $T=0$}
water at 300
\text{water at $30^0$}
ice at −50
\text{ice at $-5^0$}
Water at 00
\text{Water at $0^0$}
→
\rightarrow
→
\rightarrow
→
\rightarrow
Q1
Q_1
Q2
Q_2
cooling
\text{cooling}
melting
\text{melting}
heating
\text{heating}
water at 00
\text{water at $0^0$}
ice at 00
\text{ice at $0^0$}
Qwater+Qice=0
Q_\text{water}+Q_\text{ice}=0
mwcwΔTw+(miciΔTi+mi′LF)=0
m_\text{w}c_\text{w}\Delta T_\text{w}+ (m_{i}c_{i}\Delta T_i+m'_iL_F)=0
heating
\text{heating}
melting
\text{melting}
mi′ is the mass of ice that has melted
\text{$m'_i$ is the mass of ice that has melted}
m′=−LFmwcwΔTw+miciΔTi=−333×103150×4190×(0−30)+60×2220×(0−(−5))
m'=-\frac{m_\text{w}c_\text{w}\Delta T_\text{w}+ m_{i}c_{i}\Delta T_i}{L_F}=-\frac{150\times 4190\times(0-30)+60\times2220\times(0-(-5))}{333\times 10^3}
m′=54.6 g
m'=54.6 \text{ g}
Answer B
\text{Answer B}
Qw
{Q_\text{w}}
(only exchange, no heat is lost)
(\text{only exchange, no heat is lost})
mi
m_i
mi
m_i
mi′
m'_i
One kg of ice is mixed with one kg of water at 10.0∘C. When thermal equilibrium is reached,
\text{One $\mathrm{kg}$ of ice is mixed with one $\mathrm{kg}$ of water at $10.0^{\circ} \mathrm{C}$. When thermal equilibrium is reached, }
the mixture contains total 2.00 kg of ice at 0.00∘C. Determine the initial temperature of the ice.
\text{the mixture contains total $2.00 \mathrm{~kg}$ of ice at $0.00^{\circ} \mathrm{C}$. Determine the initial temperature of the ice.}
The specific heat of ice 2092 J/kg∘C.
\text{The specific heat of ice $2092 \mathrm{~J} / \mathrm{kg}^{\circ} \mathrm{C}$.}

One kg of ice is mixed with one kg of water at 10.0∘C. When thermal equilibrium is reached,
\text{One $\mathrm{kg}$ of ice is mixed with one $\mathrm{kg}$ of water at $10.0^{\circ} \mathrm{C}$. When thermal equilibrium is reached, }
the mixture contains total 2.00 kg of ice at 0.00∘C. Determine the initial temperature of the ice.
\text{the mixture contains total $2.00 \mathrm{~kg}$ of ice at $0.00^{\circ} \mathrm{C}$. Determine the initial temperature of the ice.}
The specific heat of ice 2092 J/kg∘C.
\text{The specific heat of ice $2092 \mathrm{~J} / \mathrm{kg}^{\circ} \mathrm{C}$.}
QWater +Qice =0
Q_{\text {Water }}+ Q_{\text {ice }}=0
ice at T
\text{ice at T}
ice at 00
\text{ice at $0^0$}
Heat is not lost and gets only exchanged
\text{Heat is not lost and gets only exchanged}
water at 100
\text{water at $10^0$}
water at 00
\text{water at $0^0$}
ice at 00
\text{ice at $0^0$}
→
\rightarrow
→
\rightarrow
→
\rightarrow
Qice=mici(0−T)
Q_\text{ice}=m_i c_{i} (0-T)
Qwater=Q1+Q2=mwcw(0−10)+mwLs
Q_\text{water}=Q_1+Q_2=m_wc_w(0-10)+m_wL_s
Ls=−LF=−333 kJ/c
L_s=-L_F=\textcolor{red}{-}333 \text{ kJ/c}
Q1
Q_1
Q2
Q_2
cooling
\text{cooling}
solidification
\text{solidification}
heating
\text{heating}
−mwcw10−mwLF=miciT
-m_wc_w 10-m_wL_F=m_ic_i T
T=−micimwcw10+mwLF=−1×20921×10×4187+333×103=−179.20C
T=-\frac{m_wc_w 10+m_wL_F}{m_ic_i}=-\frac{1\times10\times4187+333\times 10^3}{1\times 2092}=-179.2 ^0C
Answer A
\text{Answer A}
A block of mass 125 g at a temperature of 90.0∘C is placed in a cup containing 0.326 kg of water
\text{A block of mass $125 \mathrm{~g}$ at a temperature of $90.0^{\circ} \mathrm{C}$ is placed in a cup containing 0.326 $\mathrm{kg}$ of water}
at 20.0∘C. The block and the water reach an equilibrium temperature of 22.4∘C. Neglecting the
\text{ at $20.0^{\circ} \mathrm{C}$. The block and the water reach an equilibrium temperature of $22.4^{\circ} \mathrm{C}$. Neglecting the }
heat capacity of the cup, find the specific heat of the block.
\text{heat capacity of the cup, find the specific heat of the block. }

A block of mass 125 g at a temperature of 90.0∘C is placed in a cup containing 0.326 kg of water
\text{A block of mass $125 \mathrm{~g}$ at a temperature of $90.0^{\circ} \mathrm{C}$ is placed in a cup containing 0.326 $\mathrm{kg}$ of water}
at 20.0∘C. The block and the water reach an equilibrium temperature of 22.4∘C. Neglecting the
\text{ at $20.0^{\circ} \mathrm{C}$. The block and the water reach an equilibrium temperature of $22.4^{\circ} \mathrm{C}$. Neglecting the }
heat capacity of the cup, find the specific heat of the block.
\text{heat capacity of the cup, find the specific heat of the block. }
Qw+QB=0
Q_w+Q_B=0
No heat is lost. only exhange of heat accurs.
\text{No heat is lost. only exhange of heat accurs.}
mwcwΔTw+mBcBΔTB=0
m_w c_w\Delta T_w+m_B c_B\Delta T_B=0
cB=mBΔTBmwcwΔTw=mB×(90−22.4)mw×cw×(22.4−20)=0.125×67.60.326×4187×(2.4)=387.7 J/kg⋅C∘
c_B=\frac{m_w c_w\Delta T_w}{m_B \Delta T_B}=\frac{m_w \times c_w \times(22.4-20)}{m_B \times(90-22.4)}=\frac{0.326 \times 4187 \times(2.4)}{0.125 \times 67.6}=387.7 \mathrm{~J} / \mathrm{kg} \cdot \mathrm{C}^{\circ}
Find the temperature T4
\text{Find the temperature $T_4$ }

Find the temperature T4
\text{Find the temperature $T_4$ }

In the steady state, the conduction rates through
\text{In the steady state, the conduction rates through }
all the layers are the same.
\text{all the layers are the same.}
kaALaT1−T2=kdALdT4−T5
k_a A \frac{T_1-T_2}{L_a}=k_d A \frac{T_4-T_5}{L_d}
kaAKaT1−T2=5LaA2LaT4−T5
k_a A \frac{T_1-T_2}{K_a}=5 L_a A \frac{T_4-T_5}{2 L_a}
T1−T2=25( T4−T5)
\mathrm{T}_1-\mathrm{T}_2=\frac{5}{2}\left(\mathrm{~T}_4-\mathrm{T}_5\right)
25∘C−20∘C=25( T4−(−10∘C))
25^{\circ} \mathrm{C}-20^{\circ} \mathrm{C}=\frac{5}{2}\left(\mathrm{~T}_4-\left(-10^{\circ} \mathrm{C}\right)\right)
T4=−8∘C
T_4=-8^{\circ} \mathrm{C}
Pa=Pd
P_a=P_d
A cubic tank filled with 5.0 kg of water is insulated from all sides except its top which is covered with
\text{{A cubic tank filled with $5.0 \mathrm{~kg}$ of water is insulated from all sides except its top which is covered with }}
a square glass sheet of length 2.0 m and thickness 3.0 cm. The water is initially at 20∘C. It is exposed
\text{a square glass sheet of length $2.0 \mathrm{~m}$ and thickness $3.0 \mathrm{~cm}$. The water is initially at $20^{\circ} \mathrm{C}$. It is exposed }
for 20 seconds to the outside environment where the temperature is 55∘C. Find the change in the
\text{for 20 seconds to the outside environment where the temperature is $55^{\circ} \mathrm{C}$. Find the change in the }
temperature of water (assume that heat is distributed uniformly in the water). (Kglass =1.0 W/m.K)
\text{temperature of water (assume that heat is distributed uniformly in the water). $\left(\mathrm{K}_{\text {glass }}=1.0 \mathrm{~W} / \mathrm{m} . \mathrm{K}\right)$}

A cubic tank filled with 5.0 kg of water is insulated from all sides except its top which is covered with
\text{{A cubic tank filled with $5.0 \mathrm{~kg}$ of water is insulated from all sides except its top which is covered with }}
a square glass sheet of length 2.0 m and thickness 3.0 cm. The water is initially at 20∘C. It is exposed
\text{a square glass sheet of length $2.0 \mathrm{~m}$ and thickness $3.0 \mathrm{~cm}$. The water is initially at $20^{\circ} \mathrm{C}$. It is exposed }
for 20 seconds to the outside environment where the temperature is 55∘C. Find the change in the
\text{for 20 seconds to the outside environment where the temperature is $55^{\circ} \mathrm{C}$. Find the change in the }
temperature of water (assume that heat is distributed uniformly in the water). (Kglass =1.0 W/m.K)
\text{temperature of water (assume that heat is distributed uniformly in the water). $\left(\mathrm{K}_{\text {glass }}=1.0 \mathrm{~W} / \mathrm{m} . \mathrm{K}\right)$}
Q=LKA(TH−TL)t=mωcωΔT
\mathrm{Q}=\frac{\mathrm{KA}\left(\mathrm{T}_{\mathrm{H}}-\mathrm{T}_{\mathrm{L}}\right) \mathrm{t}}{\mathrm{L}}=\mathrm{m}_\omega c_\omega \Delta \mathrm{T}
ΔT=mωcωLKA(TH−TL)t=3×10−2×5.0×4186(1.0)(4)(55−20)(20)
\Delta \mathrm{T}=\frac{\mathrm{KA}\left(\mathrm{T}_{\mathrm{H}}-\mathrm{T}_{\mathrm{L}}\right) \mathrm{t}}{\mathrm{m}_\omega \mathrm{c}_\omega \mathrm{L}}=\frac{(1.0)(4)(55-20)(20)}{3 \times 10^{-2} \times 5.0 \times 4186}
⇒ΔT=4.5∘C
\Rightarrow \Delta \mathrm{T}=4.5^{\circ} \mathrm{C}
Additional problems
\text{Additional problems}
A steel rod is 4.000 cm in diameter at 35∘C. A brass ring has an inner diameter of 3.992 cm
\text{A steel rod is $4.000 \mathrm{~cm}$ in diameter at $35^{\circ} \mathrm{C}$. A brass ring has an inner diameter of $3.992 \mathrm{~cm}$}
at 35∘C. At what common temperature will the brass ring slide onto steal rod?
\text{at $35^{\circ} \mathrm{C}$. At what common temperature will the brass ring slide onto steal rod?}
[αsteel =11×10−6/K−1,αbrass =19×10−6/K−1] (Ans: 286∘C )
\text{$\left[\alpha_{\text {steel }}=11 \times 10^{-6} / \mathrm{K}^{-1}, \alpha_{\text {brass }}=19 \times 10^{-6} / \mathrm{K}^{-1}\right]$ (Ans: $286^{\circ} \mathrm{C}$ )}
when we increase temperature, each length will encrease following the same law
\text{when we increase temperature, each length will encrease following the same law}
ΔL=LαΔT
\Delta L=L\alpha \Delta T
The ring will slide when it will have the same diameter as that of the rod
\text{The ring will slide when it will have the same diameter as that of the rod}
D1=D2
D_1=D_2
D10+ΔD1=D20+ΔD2
D_1^0+\Delta D_1=D_2^0+\Delta D_2
⇒
\Rightarrow
Ds0+Ds0αsΔT=Db0+Db0αbΔT
D_s^0+D_s^0 \alpha_s \Delta T=D_b^0+ D_b^0 \alpha_b \Delta T
⇒
\Rightarrow
⇒
\Rightarrow
ΔT=(Ds0αs−Db0αb)Db0−Ds0=(4×11−3.992×19)×10−63.992−4.000
\Delta T=\frac{D_b^0-D_s^0}{(D_s^0 \alpha_s- D_b^0 \alpha_b)}=\frac{3.992-4.000}{(4\times 11-3.992\times19)\times 10^{-6}}
=2510
=251^0
T2=2860
T_2=286^0
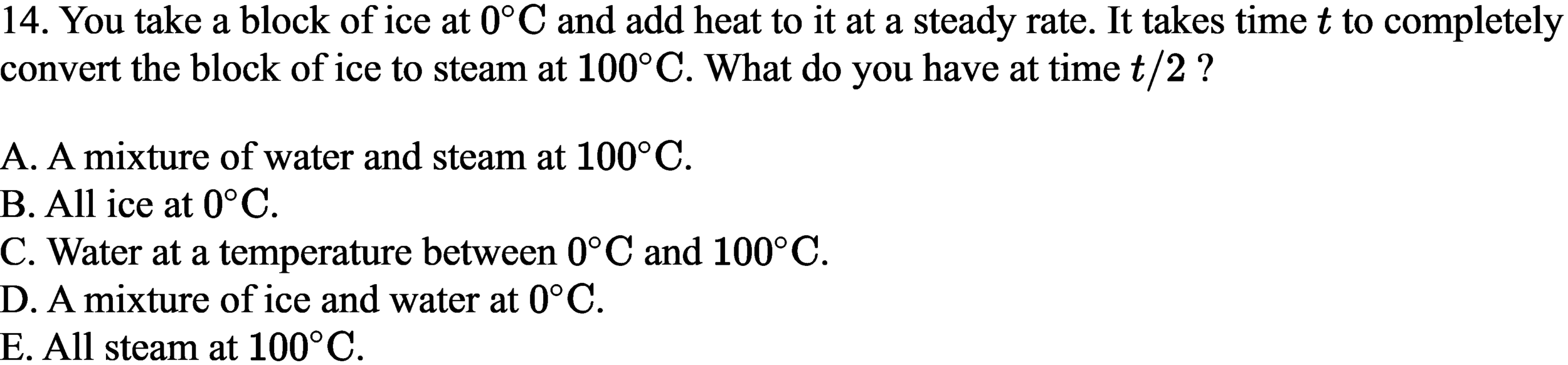
C h a p t e r 1 8 \text{Chapter 18} C h a p t e r 1 8 \text{Chapter 18} C h a p t e r 1 8 \text{Chapter 18} C h a p t e r 1 8 \text{Chapter 18} D r . A d e l A b b o u t \text{Dr. Adel Abbout}
Chapter 18
By smstry
Chapter 18
- 424



